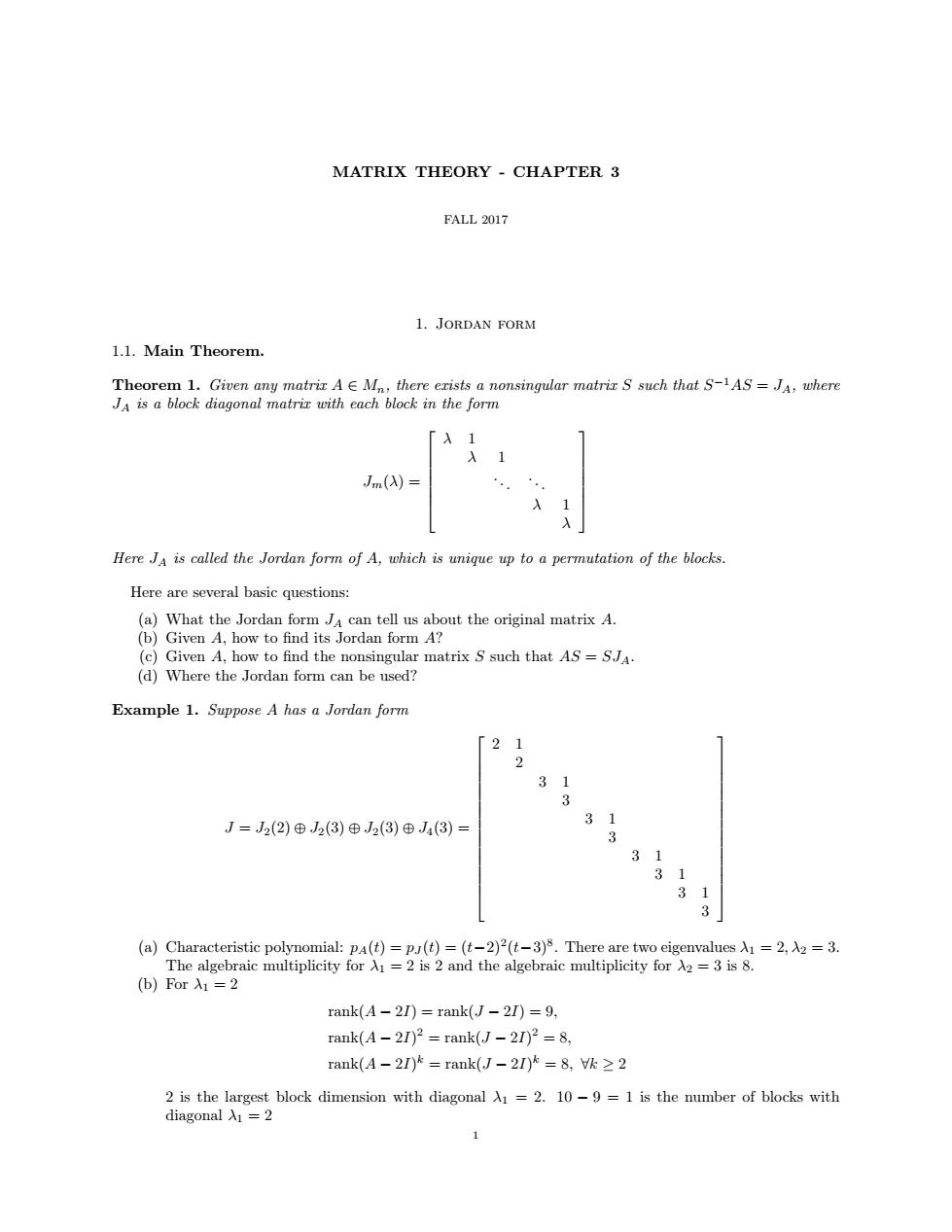
MATRIX THEORY CHAPTER 3 FALL 2017 1.JORDAN FORM 1.1.Main Theorem 「A1 入1 Jm(A)= Here JA is called the Jordan form of A,which is unique up to a permutation of the blocks Here are several basic questions (a)What the Jordan form JA can tell us about the original matrix A. (b)Given A,how to find its Jordan form A? to nn Example 1.Suppose A has a Jordan form 「21 J=2(2)⊕2(3)⊕2(3)⊕J(3) 31 3 31 1 31 3 (a)Characte The algeb =2,2=3 (b)For A1 =2 rank(A-2)=rank(J-2)=9, rank(A-21)2 rank(J-2)2=8, rank(A-21)=rank(J)=8,k
MATRIX THEORY - CHAPTER 3 FALL 2017 1. Jordan form 1.1. Main Theorem. Theorem 1. Given any matrix A ∈ Mn, there exists a nonsingular matrix S such that S −1AS = JA, where JA is a block diagonal matrix with each block in the form Jm(λ) = λ 1 λ 1 . . . . . . λ 1 λ Here JA is called the Jordan form of A, which is unique up to a permutation of the blocks. Here are several basic questions: (a) What the Jordan form JA can tell us about the original matrix A. (b) Given A, how to find its Jordan form A? (c) Given A, how to find the nonsingular matrix S such that AS = SJA. (d) Where the Jordan form can be used? Example 1. Suppose A has a Jordan form J = J2(2) ⊕ J2(3) ⊕ J2(3) ⊕ J4(3) = 2 1 2 3 1 3 3 1 3 3 1 3 1 3 1 3 (a) Characteristic polynomial: pA(t) = pJ (t) = (t−2)2 (t−3)8 . There are two eigenvalues λ1 = 2, λ2 = 3. The algebraic multiplicity for λ1 = 2 is 2 and the algebraic multiplicity for λ2 = 3 is 8. (b) For λ1 = 2 rank(A − 2I) = rank(J − 2I) = 9, rank(A − 2I) 2 = rank(J − 2I) 2 = 8, rank(A − 2I) k = rank(J − 2I) k = 8, ∀k ≥ 2 2 is the largest block dimension with diagonal λ1 = 2. 10 − 9 = 1 is the number of blocks with diagonal λ1 = 2 1

2 FALL 2017 (c)Forλ2=3 rank(A-3I)=rank(J-31)=7, rank(A-31)2 rank(J3)2=4, rank(A-31)rank(J-3)3=3. rank(A-31)=rank(-31)=2. rank(A-31)rank()=2.k 24 12 7-=3 is the number of blocks with dia al a with dimer 4-3=1 is the number of blocks with diagonal 3 with dimension 3x3. gonal 3 with dimension 4x 4. (d)Suppose S (Ao7 =307 Ao3=303 Aas =305 Aa2=2a2+a4 Aa4=304+03 a6=306十a5 4a10=3a10+a8 There are 4 eigenvectors Notice that 4 is the number of blocks in the Jordan form 1.2.Properties.Jordan form is the standard form or simple representative in each class given by similar equivalence. AS=SJ.J=diag(J()..J(e)),J()=ding ()...() 入九1 ()= (a)First,J is block diagonal with each diagonal block (co sponding to oneλ.Second.each ()is block diagonal such that the small diagonal blocks all have on the diagonal,1 upper next to diagona and 0 at o of small blockssth multiplicity nCorresponding to eachthe number (b)The number and dimensions for those small blocks corresponding to each is fully determined by rank(A-)'=r,s=1,2, n-r1 =number of blocks corresponding to Ai with dimension 2 1 x 1 r-ra=number of blocks corresponding to with dimension2x2 ra-ra=number of blocks corresponding to with dimension3 (n-r)-(r-ra)=number of blocks corresponding to with dimension 1x1 (r1-r2)-(r2-rs)=number of blocks corresponding to A;with dimension 2 x 2 first si such that r)Then corresponding to this,the (c)Jordan form determines all the pr operties of A that does not change under similar transformation. For example,eigenvalues,characte ristic polynomial,minimal polynomial. Then those colmn vectors,which corresponds to the first term of each smal
2 FALL 2017 (c) For λ2 = 3 rank(A − 3I) = rank(J − 3I) = 7, rank(A − 3I) 2 = rank(J − 3I) 2 = 4, rank(A − 3I) 3 = rank(J − 3I) 3 = 3, rank(A − 3I) 4 = rank(J − 3I) 3 = 2, rank(A − 3I) k = rank(J − 3I) k = 2, ∀k ≥ 4 4 is the largest block dimension with diagonal 3. 10 − 7 = 3 is the number of blocks with diagonal 3. 7 − 4 = 3 is the number of blocks with diagonal 3 with dimension ≥ 2 × 2. 4 − 3 = 1 is the number of blocks with diagonal 3 with dimension ≥ 3 × 3. 3 − 2 = 1 is the number of blocks with diagonal 3 with dimension ≥ 4 × 4. (d) Suppose S = [α1, ..., α10] such that AS = SJ. Then ( Aα1 = 2α1 Aα2 = 2α2 + α1 ( Aα3 = 3α3 Aα4 = 3α4 + α3 ( Aα5 = 3α5 Aα6 = 3α6 + α5 Aα7 = 3α7 Aα8 = 3α8 + α7 Aα9 = 3α9 + α8 Aα10 = 3α10 + α8 There are 4 eigenvectors α1, α3, α5, α7. Notice that 4 is the number of blocks in the Jordan form. 1.2. Properties. Jordan form is the standard form or simple representative in each class given by similar equivalence. AS = SJ, J = diag{J(λ1), · · · J(λk)}, J(λi) = diag{Jl1 (λi), · · · , Jli (λi)} Jj (λi) = λi 1 λi 1 . . . . . . λi 1 λi (a) First, J is block diagonal with each diagonal block J(λi) corresponding to one λi . Second, each J(λi) is block diagonal such that the small diagonal blocks all have λi on the diagonal, 1 upper next to diagonal and 0 at other positions. (b) The total dimension of J(λi) is the algebraic multiplicity ni . Corresponding to each λi , the number of small blocks is the geometric multiplicity li . (b) The number and dimensions for those small blocks corresponding to each λi is fully determined by rank(A − λi) s = rs, s = 1, 2, ....... n − r1 = number of blocks corresponding to λi with dimension ≥ 1 × 1 r1 − r2 = number of blocks corresponding to λi with dimension ≥ 2 × 2 r2 − r3 = number of blocks corresponding to λi with dimension ≥ 3 × 3 . . . (n − r1) − (r1 − r2) = number of blocks corresponding to λi with dimension 1 × 1 (r1 − r2) − (r2 − r3) = number of blocks corresponding to λi with dimension 2 × 2 . . . The computation stops at the first si such that rsi = r(si+1). Then corresponding to this λi , the largest block has dimension si × si . (c) Jordan form determines all the properties of A that does not change under similar transformation. For example, eigenvalues, characteristic polynomial, minimal polynomial. (d) Denote S = [α1, ..., αn]. Then those column vectors, which corresponds to the first term of each small block in Jordan form, are eigenvectors
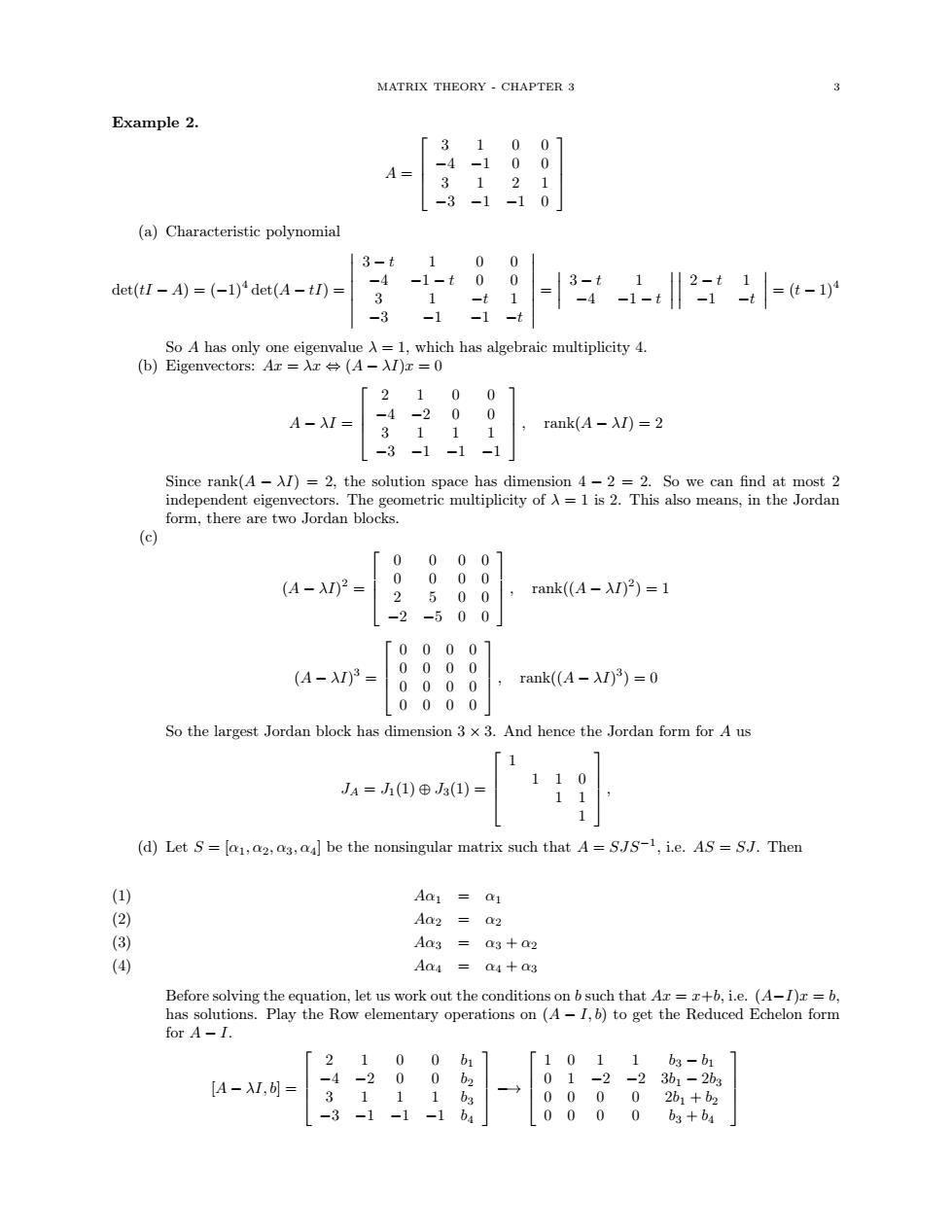
MATRIX THEORY CHAPTER 3 3 Example 2. (a)Characteristic polynomial 3-t det(tI-A)=(-1)det(A-tI)= -1 lw- -1-t0 So A has only one eigenvalue A=1,which has algebraic multiplicity 4. (b)Eigenvectors:Ar=(AAl)r=0 1 A-AI= 311 rank(A-AI)=2 -3-1-1-1 Since rank(A-Al)=2.the solution space has dimension 4-2=2.So we can find at most 2 independent eigenvecorin bleekcometrie multipucity or 2.This also means,in the Jordan form,there are two (e) 88881 「0 (4-λI)2= 500 rank((A-AD)2)=1 2 -2-500 「00001 (4-A)3 888 rank((A-AI))=0 0000 So the largest Jordan block has dimension 3 x 3.And hence the Jordan form for A us 「1 JA=(1)3(1) 110 11 1 (d)Let S=[a1,a2,a3,aa]be the nonsingular matrix such that A=S.JS-1,i.e.AS=SJ.Then Ao1 =01 四 A02=02 Aa3 =03+02 Aa4 04+03 Before solving the equation,let us work out the conditions on bsuch that Ar=r+b,i.e.(A-I)=b, Ply the Row etary peo(togt th Reede 2 0 0 10 [A-AI,6]= 01-2-2361-2h
MATRIX THEORY - CHAPTER 3 3 Example 2. A = 3 1 0 0 −4 −1 0 0 3 1 2 1 −3 −1 −1 0 (a) Characteristic polynomial det(tI − A) = (−1)4 det(A − tI) = 3 − t 1 0 0 −4 −1 − t 0 0 3 1 −t 1 −3 −1 −1 −t = 3 − t 1 −4 −1 − t 2 − t 1 −1 −t = (t − 1)4 So A has only one eigenvalue λ = 1, which has algebraic multiplicity 4. (b) Eigenvectors: Ax = λx ⇔ (A − λI)x = 0 A − λI = 2 1 0 0 −4 −2 0 0 3 1 1 1 −3 −1 −1 −1 , rank(A − λI) = 2 Since rank(A − λI) = 2, the solution space has dimension 4 − 2 = 2. So we can find at most 2 independent eigenvectors. The geometric multiplicity of λ = 1 is 2. This also means, in the Jordan form, there are two Jordan blocks. (c) (A − λI) 2 = 0 0 0 0 0 0 0 0 2 5 0 0 −2 −5 0 0 , rank((A − λI) 2 ) = 1 (A − λI) 3 = 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 , rank((A − λI) 3 ) = 0 So the largest Jordan block has dimension 3 × 3. And hence the Jordan form for A us JA = J1(1) ⊕ J3(1) = 1 1 1 0 1 1 1 , (d) Let S = [α1, α2, α3, α4] be the nonsingular matrix such that A = SJS−1 , i.e. AS = SJ. Then (1) Aα1 = α1 (2) Aα2 = α2 (3) Aα3 = α3 + α2 (4) Aα4 = α4 + α3 Before solving the equation, let us work out the conditions on b such that Ax = x+b, i.e. (A−I)x = b, has solutions. Play the Row elementary operations on (A − I, b) to get the Reduced Echelon form for A − I. [A − λI, b] = 2 1 0 0 b1 −4 −2 0 0 b2 3 1 1 1 b3 −3 −1 −1 −1 b4 −→ 1 0 1 1 b3 − b1 0 1 −2 −2 3b1 − 2b3 0 0 0 0 2b1 + b2 0 0 0 0 b3 + b4
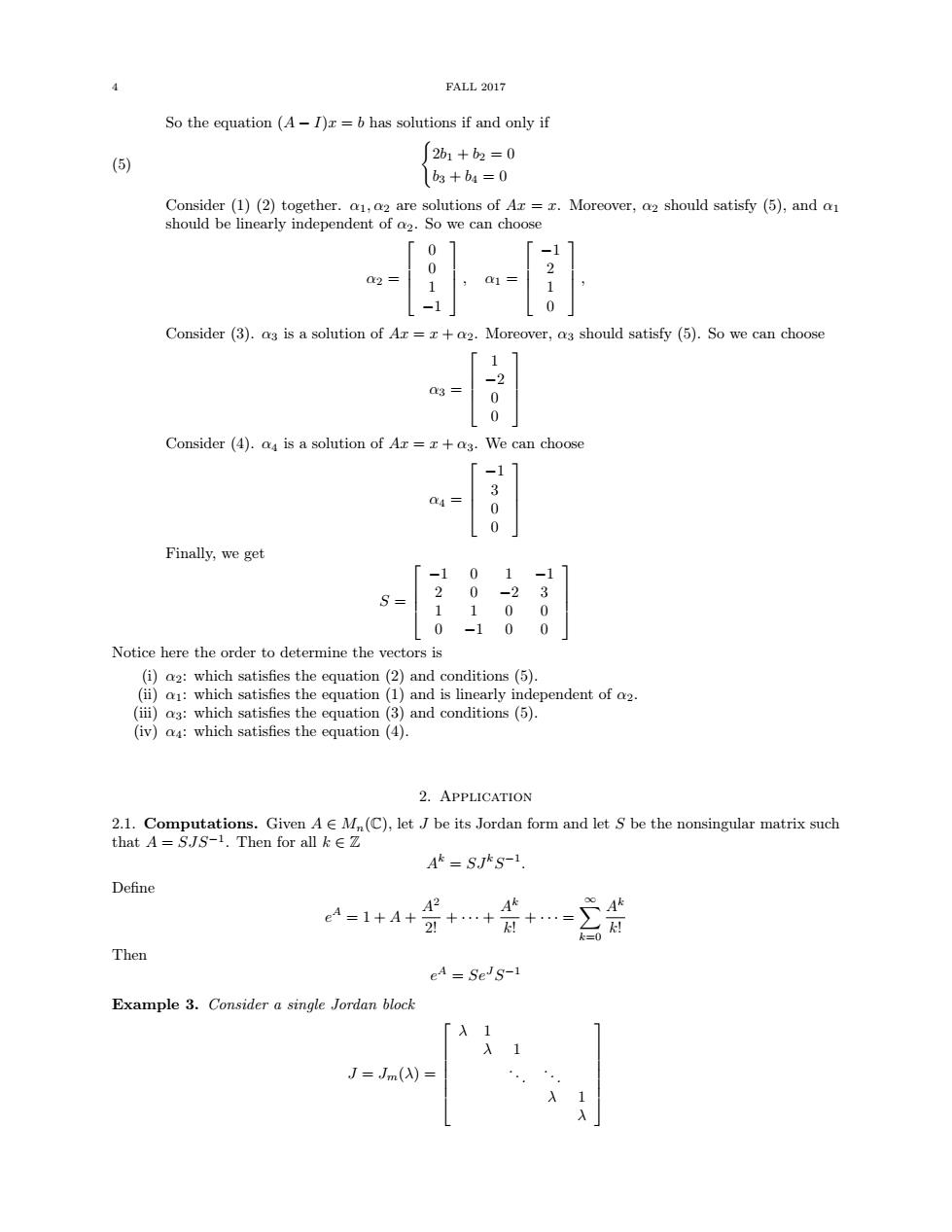
4 FALL 2017 So the equation (A-I)z=b has solutions if and only if 2b1+2=0 b3+b4=0 2 01 -11 0 Consider (3).os is a solution of Ar=r+a2.Moreover,a3 should satisfy (5).So we can choose 17 a3= 0 Consider (4).a is a solution of Ar=+.We can choose -1 Finally,we get s- Notice here the order to determine the vectors is ependent of o (iv):which satisfies the equation (4). 2.APPLICATION 名品各C,bte Ak SS-1 Define 1+4+++若+…一2甜 Then eA Se/S-1 Example 3.Consider a single Jordan block 1 J=Jm(X)=
4 FALL 2017 So the equation (A − I)x = b has solutions if and only if (5) ( 2b1 + b2 = 0 b3 + b4 = 0 Consider (1) (2) together. α1, α2 are solutions of Ax = x. Moreover, α2 should satisfy (5), and α1 should be linearly independent of α2. So we can choose α2 = 0 0 1 −1 , α1 = −1 2 1 0 , Consider (3). α3 is a solution of Ax = x + α2. Moreover, α3 should satisfy (5). So we can choose α3 = 1 −2 0 0 Consider (4). α4 is a solution of Ax = x + α3. We can choose α4 = −1 3 0 0 Finally, we get S = −1 0 1 −1 2 0 −2 3 1 1 0 0 0 −1 0 0 Notice here the order to determine the vectors is (i) α2: which satisfies the equation (2) and conditions (5). (ii) α1: which satisfies the equation (1) and is linearly independent of α2. (iii) α3: which satisfies the equation (3) and conditions (5). (iv) α4: which satisfies the equation (4). 2. Application 2.1. Computations. Given A ∈ Mn(C), let J be its Jordan form and let S be the nonsingular matrix such that A = SJS−1 . Then for all k ∈ Z A k = SJkS −1 . Define e A = 1 + A + A2 2! + · · · + Ak k! + · · · = X∞ k=0 Ak k! Then e A = SeJ S −1 Example 3. Consider a single Jordan block J = Jm(λ) = λ 1 λ 1 . . . . . . λ 1 λ
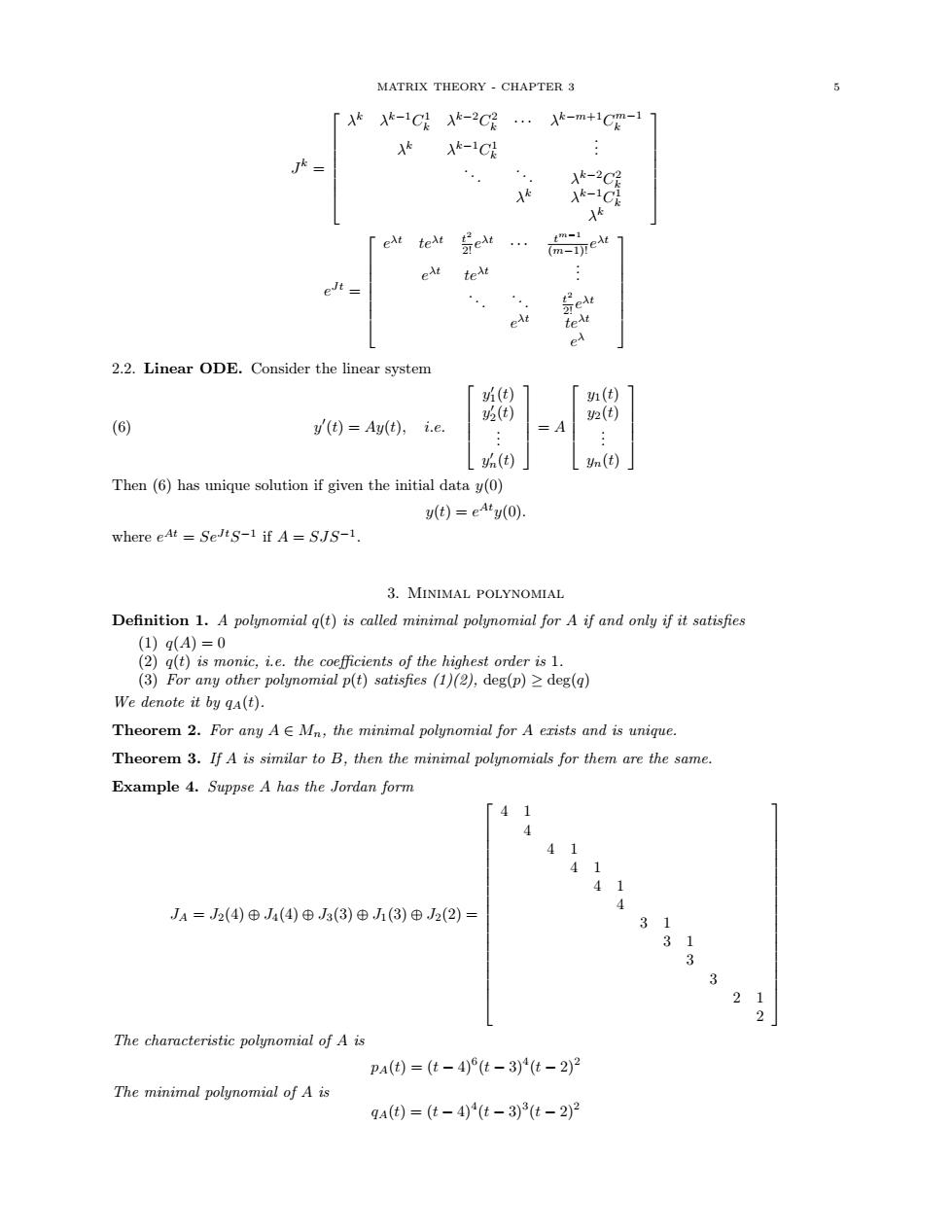
MATRIX THEORY-CHAPTER 3 -1C以λ-2C喉…-m+1CW-11 kAk-1C以 J 1k [ext text号et: 2.2.Linear ODE.Consider the linear system ()=A(),i.e =A 6() un(t) Then(6)has unique solution if given the initial data) =e4o). where eAt SeJts-1 if A=SJS-1. 3.MINIMAL POLYNOMIAL Definition 1.A polymomial (t)is called minimal polynomial for A if and only if it satisfies 智PEah时Aeom (3)For any other polymomial p(t)satisfies(1)(2),deg(p)z deg(q) We denote it by qa(t). Theorem 2.For any AMa,the minimal polynomial for A erists and is unique. Theorem 3.If A is similar to B,then the minimal polyomials for them are the same. Example 4.Suppse A has the Jordan form 「4 1 41 JA=2(⊕(④⊕(3)⊕(3)⊕J2(2 31 3 21 The characteristic polynomial of A is pA()=(t-4)(t-3)(t-2)2 The minimal polynomial of A is gA()=(t-4)1(t-3)3(t-2)2
MATRIX THEORY - CHAPTER 3 5 J k = λ k λ k−1C 1 k λ k−2C 2 k · · · λ k−m+1C m−1 k λ k λ k−1C 1 k . . . . . . . . . λ k−2C 2 k λ k λ k−1C 1 k λ k e Jt = e λt teλt t 2 2! e λt · · · tm−1 (m−1)! e λt e λt teλt . . . . . . . . . t 2 2! e λt e λt teλt e λ 2.2. Linear ODE. Consider the linear system (6) y 0 (t) = Ay(t), i.e. y 0 1 (t) y 0 2 (t) . . . y 0 n (t) = A y1(t) y2(t) . . . yn(t) Then (6) has unique solution if given the initial data y(0) y(t) = e Aty(0). where e At = SeJtS −1 if A = SJS−1 . 3. Minimal polynomial Definition 1. A polynomial q(t) is called minimal polynomial for A if and only if it satisfies (1) q(A) = 0 (2) q(t) is monic, i.e. the coefficients of the highest order is 1. (3) For any other polynomial p(t) satisfies (1)(2), deg(p) ≥ deg(q) We denote it by qA(t). Theorem 2. For any A ∈ Mn, the minimal polynomial for A exists and is unique. Theorem 3. If A is similar to B, then the minimal polynomials for them are the same. Example 4. Suppse A has the Jordan form JA = J2(4) ⊕ J4(4) ⊕ J3(3) ⊕ J1(3) ⊕ J2(2) = 4 1 4 4 1 4 1 4 1 4 3 1 3 1 3 3 2 1 2 The characteristic polynomial of A is pA(t) = (t − 4)6 (t − 3)4 (t − 2)2 The minimal polynomial of A is qA(t) = (t − 4)4 (t − 3)3 (t − 2)2
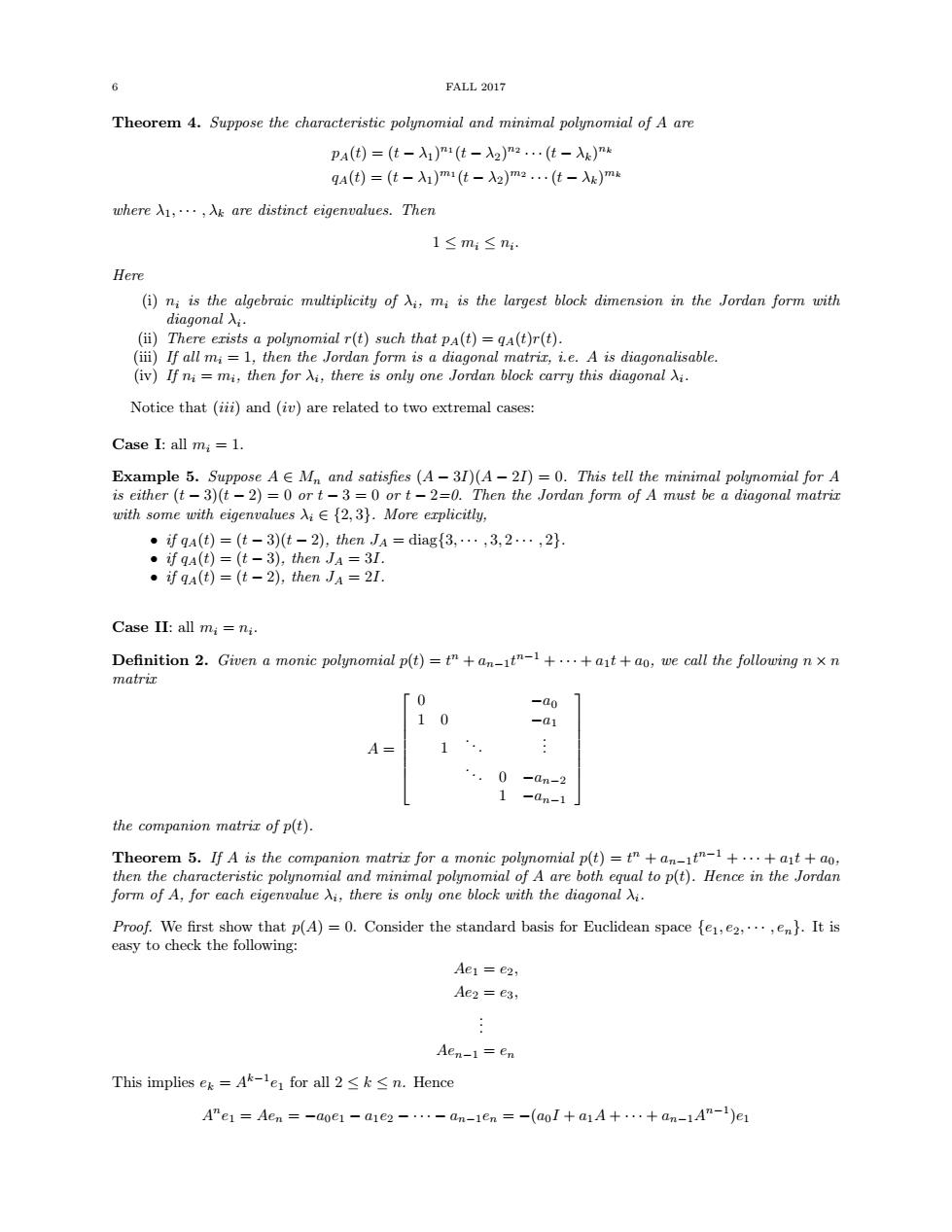
6 FALL 2017 Theorem 4.Suppose the characteristic polynomial and minimal polymomial of A are pA()=(t-)(t-2)…t-A) ga(t)=(t-A1)mi(t-X2)m2...(tk)m here入L,..,入are distinct eigenvalues.Then 1≤m≤n Here (i)n is the algebraic multiplicity of m is the largest block dimension in the Jordan form with (ch)( rir.ie.A is diagonalisable (iv)if n=mi,then fori there is only one Jordan block carry this diagonal Notice that (iii)and (iv)are related to two extremal cases: Case I:all mi=1. ith some with =-3t-2,到then JA=diag{8…,3,2…,2 Case IⅡ:allm=n Definition 2.Given a monic polynomial p(t)=+++t+o we call the following n xn matri证 「0 “. 1二- the companion matriz of p(t). Theorem 5.If A is the companion matriz for a monic polynomial p(t)=t"+an-t"-+..+at+ao then the characteristic polynomial and minimal polynomial of A are both equal to p(t).Hence in the Jordan form of A,for each eigenvalue Ai:there is only one block with the diagonal Ai. easy to check the Ae1=e2, Ae2 e3: Aen-1=en This implies-Ak-le1 for all2≤k≤n.Hence A"e1 Aen =-aoel-are2 -...an-1en =-(aoI+aA+...+an-1A"-!)e1
6 FALL 2017 Theorem 4. Suppose the characteristic polynomial and minimal polynomial of A are pA(t) = (t − λ1) n1 (t − λ2) n2 · · ·(t − λk) nk qA(t) = (t − λ1) m1 (t − λ2) m2 · · ·(t − λk) mk where λ1, · · · , λk are distinct eigenvalues. Then 1 ≤ mi ≤ ni . Here (i) ni is the algebraic multiplicity of λi, mi is the largest block dimension in the Jordan form with diagonal λi. (ii) There exists a polynomial r(t) such that pA(t) = qA(t)r(t). (iii) If all mi = 1, then the Jordan form is a diagonal matrix, i.e. A is diagonalisable. (iv) If ni = mi, then for λi, there is only one Jordan block carry this diagonal λi. Notice that (iii) and (iv) are related to two extremal cases: Case I: all mi = 1. Example 5. Suppose A ∈ Mn and satisfies (A − 3I)(A − 2I) = 0. This tell the minimal polynomial for A is either (t − 3)(t − 2) = 0 or t − 3 = 0 or t − 2=0. Then the Jordan form of A must be a diagonal matrix with some with eigenvalues λi ∈ {2, 3}. More explicitly, • if qA(t) = (t − 3)(t − 2), then JA = diag{3, · · · , 3, 2 · · · , 2}. • if qA(t) = (t − 3), then JA = 3I. • if qA(t) = (t − 2), then JA = 2I. Case II: all mi = ni . Definition 2. Given a monic polynomial p(t) = t n + an−1t n−1 + · · · + a1t + a0, we call the following n × n matrix A = 0 −a0 1 0 −a1 1 . . . . . . . . . 0 −an−2 1 −an−1 the companion matrix of p(t). Theorem 5. If A is the companion matrix for a monic polynomial p(t) = t n + an−1t n−1 + · · · + a1t + a0, then the characteristic polynomial and minimal polynomial of A are both equal to p(t). Hence in the Jordan form of A, for each eigenvalue λi, there is only one block with the diagonal λi. Proof. We first show that p(A) = 0. Consider the standard basis for Euclidean space {e1, e2, · · · , en}. It is easy to check the following: Ae1 = e2, Ae2 = e3, . . . Aen−1 = en This implies ek = Ak−1 e1 for all 2 ≤ k ≤ n. Hence A n e1 = Aen = −a0e1 − a1e2 − · · · − an−1en = −(a0I + a1A + · · · + an−1A n−1 )e1
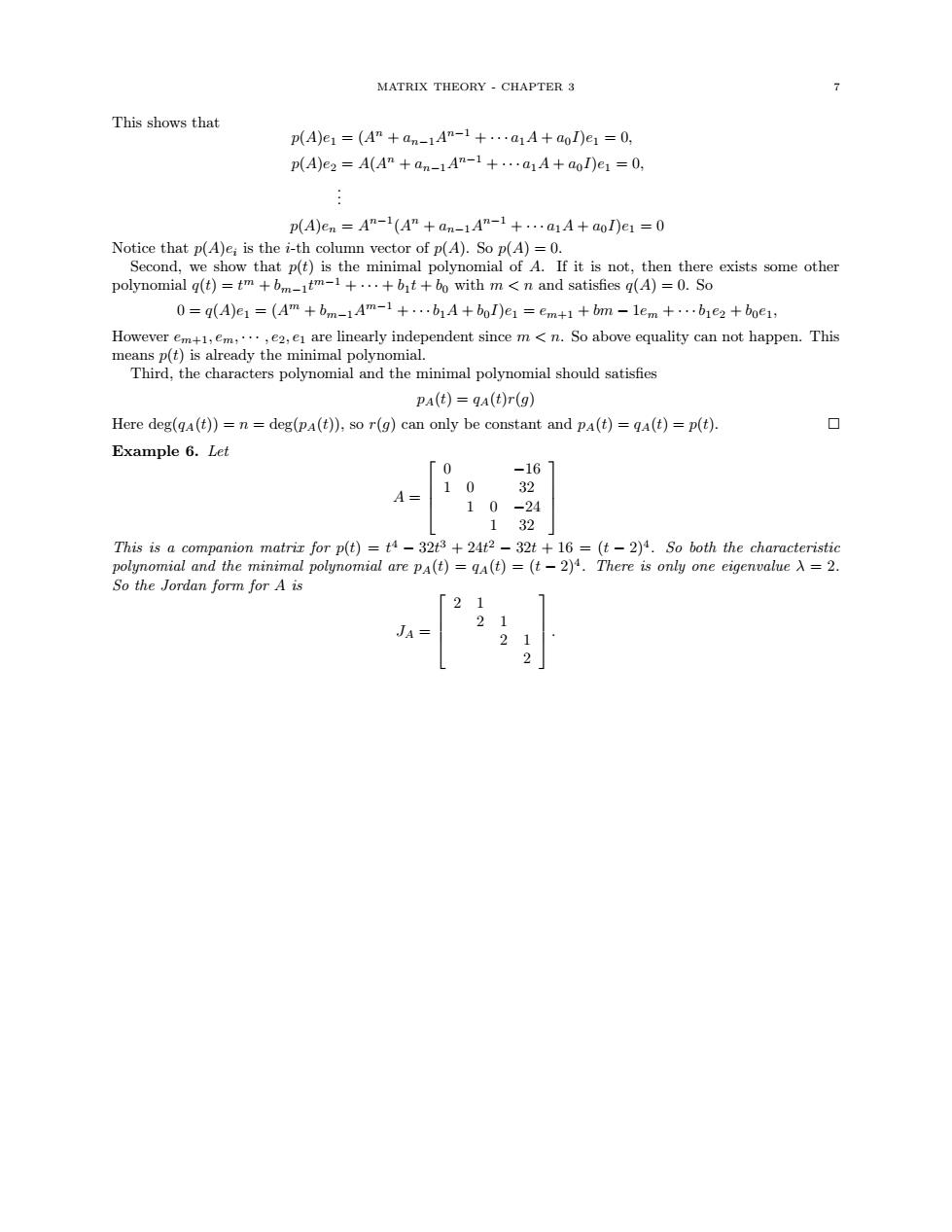
MATRIX THEORY CHAPTER 3 This shows that p(A)e1=(A"an-1Am-1+...aA+aol)e=0. p(A)e2 A(A"an-1A"-1+...aA+ao)e=0 p(A)e. -A-1(4+a- 4n-11 .…a1A+a0I)e1=0 Second,we show that p(t)is the minimal polynomial of A.itnot.then these exists some other 0=q(A)e1 =(Am+om-1Am-1+...A+bol)e1 em+i+om-lem+...oe2+boe1: However em+,em.,e2,e are linearly independent since m<n.So above equality can not happen.This PA(t)=gA(r(g) Here deg(A(t))=n=deg(pA()).sor(g)can only be constant and pA(t)=(t)-p(t). Example 6.Let 0 6 A= 10-24 132 This is a co anion matrir for p(t)=t-32t3+24t2-32t+16 =(t-2)4.So both the characteristic mAmn0=-m的mA= 2 21 21 2
MATRIX THEORY - CHAPTER 3 7 This shows that p(A)e1 = (A n + an−1A n−1 + · · · a1A + a0I)e1 = 0, p(A)e2 = A(A n + an−1A n−1 + · · · a1A + a0I)e1 = 0, . . . p(A)en = A n−1 (A n + an−1A n−1 + · · · a1A + a0I)e1 = 0 Notice that p(A)ei is the i-th column vector of p(A). So p(A) = 0. Second, we show that p(t) is the minimal polynomial of A. If it is not, then there exists some other polynomial q(t) = t m + bm−1t m−1 + · · · + b1t + b0 with m < n and satisfies q(A) = 0. So 0 = q(A)e1 = (A m + bm−1A m−1 + · · · b1A + b0I)e1 = em+1 + bm − 1em + · · · b1e2 + b0e1, However em+1, em, · · · , e2, e1 are linearly independent since m < n. So above equality can not happen. This means p(t) is already the minimal polynomial. Third, the characters polynomial and the minimal polynomial should satisfies pA(t) = qA(t)r(g) Here deg(qA(t)) = n = deg(pA(t)), so r(g) can only be constant and pA(t) = qA(t) = p(t). Example 6. Let A = 0 −16 1 0 32 1 0 −24 1 32 This is a companion matrix for p(t) = t 4 − 32t 3 + 24t 2 − 32t + 16 = (t − 2)4 . So both the characteristic polynomial and the minimal polynomial are pA(t) = qA(t) = (t − 2)4 . There is only one eigenvalue λ = 2. So the Jordan form for A is JA = 2 1 2 1 2 1 2 .�