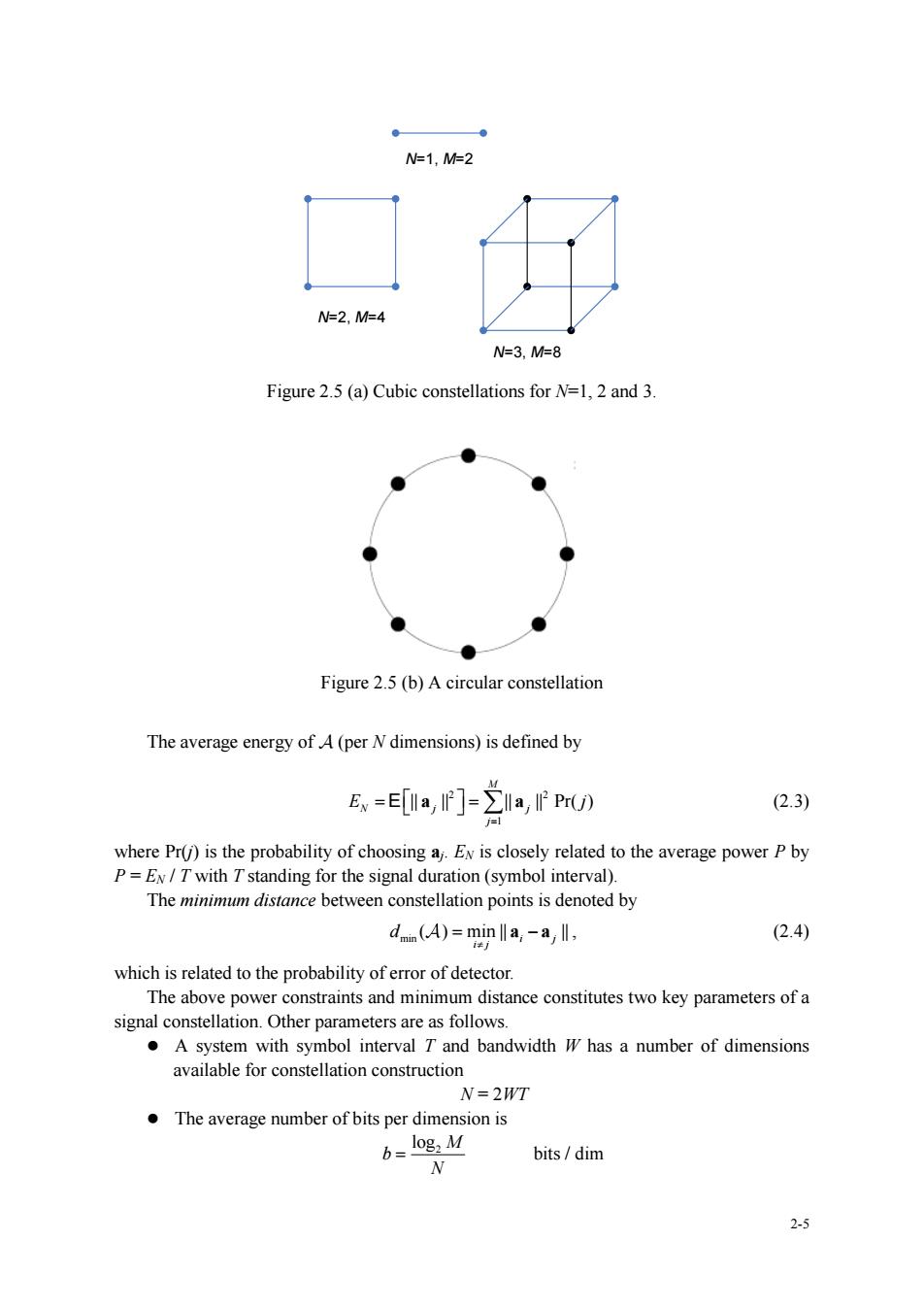
M1,M2 2,M4 W3,M=8 Figure 2.5(a)Cubic constellations for N=1,2 and 3. ●】 ● Figure 2.5(b)A circular constellation The average energy ofA(per N dimensions)is defined by E、=eIa,]-2Ia,rPmU) (2.3) where Pr()is the probability of choosinga EN is closely related to the average power P by P=E/T with Tstanding for the signal duration(symbol interval). The minimum distance between constellation points is denoted by dn(A)=min‖a,-a, (2.4) which is related to the probability of error of detector. The above power constraints and minimum distance constitutes two key parameters of a signal constellation.Other parameters are as follows. .A system with symbol interval T and bandwidth W has a number of dimensions avail ble N=2WT The average number of bits per dimension is b=log:M bits/dim 32-5 N=1, M=2 N=2, M=4 N=3, M=8 Figure 2.5 (a) Cubic constellations for N=1, 2 and 3. Figure 2.5 (b) A circular constellation The average energy of  (per N dimensions) is defined by 2 2 1 || || || || Pr( ) M N j j j E j = = =   E  a a  (2.3) where Pr(j) is the probability of choosing aj. EN is closely related to the average power P by P = EN / T with T standing for the signal duration (symbol interval). The minimum distance between constellation points is denoted by min ( ) min || || i j i j d  = − a a , (2.4) which is related to the probability of error of detector. The above power constraints and minimum distance constitutes two key parameters of a signal constellation. Other parameters are as follows. ⚫ A system with symbol interval T and bandwidth W has a number of dimensions available for constellation construction N = 2WT ⚫ The average number of bits per dimension is 2 log M b N = bits / dim