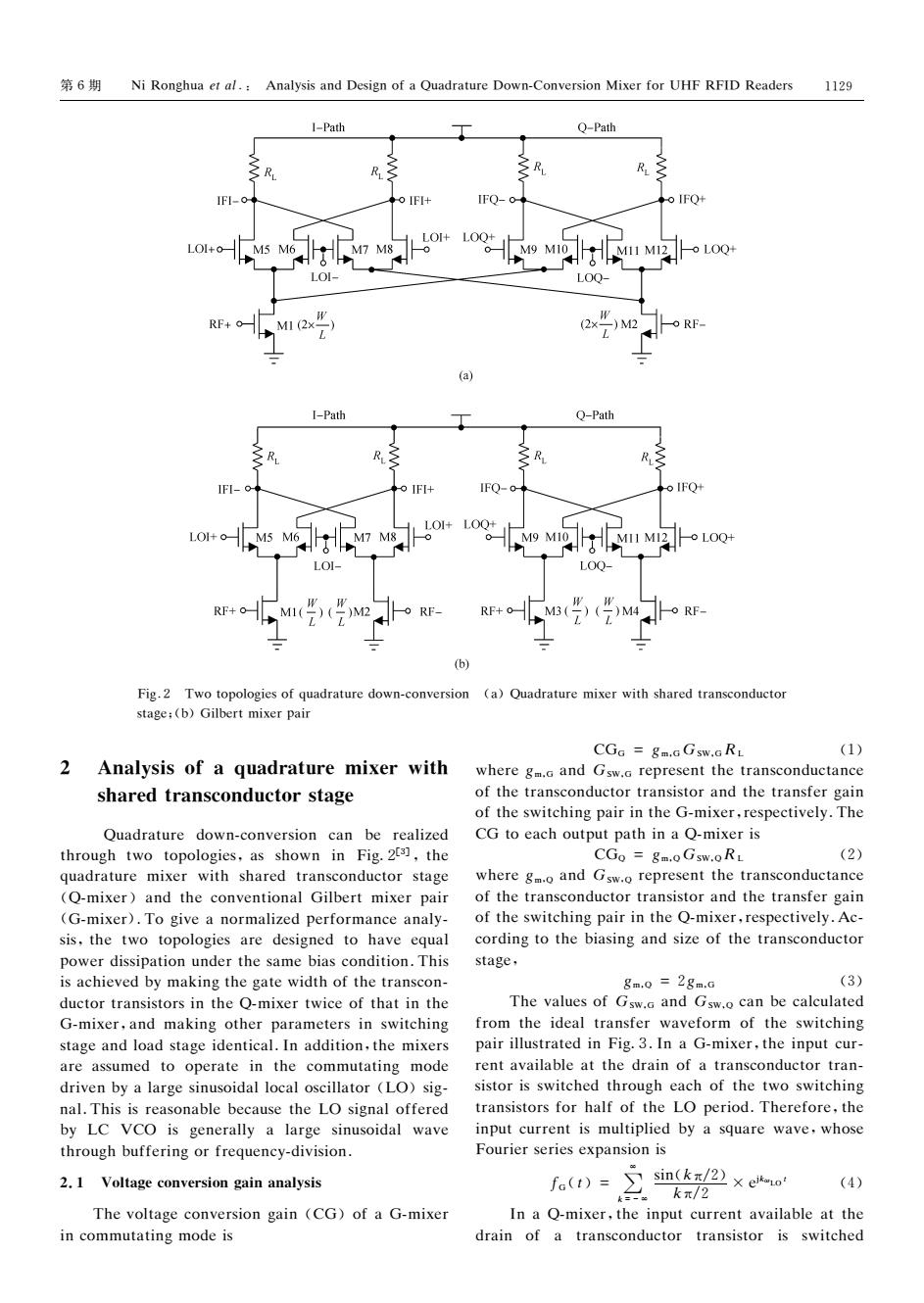
6 Ni Ronghua et al.:Analysis and Design of a Quadrature Down-Conversion Mixer for UHF RFID Readers 1129 1-Path Q-Path IFI- IFQ-o LOQ- RF. M1(2x 2x -)M2 (a) I-Path Q-Path IFI-c IFI+ o IFO MI1 MI2o LOQ+ RF+C M RF (b) Fig.2 Two topologies of quadrature down-conversion (a)Quadrature mixer with shared transconductor stage;(b)Gilbert mixer pair CGa gm.G Gsw.G RL (1) 2 Analysis of a quadrature mixer with where gm.a and Gsw.a represent the transconductance shared transconductor stage of the transconductor transistor and the transfer gain of the switching pair in the G-mixer,respectively.The Quadrature down-conversion can be realized CG to each output path in a Q-mixer is through two topologies,as shown in Fig.2t3],the CGo =gm.oGsw.oRL (2) quadrature mixer with shared transconductor stage where gm.o and Gsw.o represent the transconductance (Q-mixer)and the conventional Gilbert mixer pair of the transconductor transistor and the transfer gain (G-mixer).To give a normalized performance analy-of the switching pair in the Q-mixer,respectively.Ac- sis,the two topologies are designed to have equal cording to the biasing and size of the transconductor power dissipation under the same bias condition.This stage. is achieved by making the gate width of the transcon- gm.0=2gm.0 (3) ductor transistors in the Q-mixer twice of that in the The values of Gsw.a and Gsw.o can be calculated G-mixer,and making other parameters in switching from the ideal transfer waveform of the switching stage and load stage identical.In addition,the mixers pair illustrated in Fig.3.In a G-mixer,the input cur- are assumed to operate in the commutating mode rent available at the drain of a transconductor tran- driven by a large sinusoidal local oscillator (LO)sig- sistor is switched through each of the two switching nal.This is reasonable because the LO signal offered transistors for half of the LO period.Therefore,the by LC VCO is generally a large sinusoidal wave input current is multiplied by a square wave,whose through buffering or frequency-division. Fourier series expansion is 2.1 Voltage conversion gain analysis fa(t)=∑sin(/2×eo 2.kx/2 (4) The voltage conversion gain (CG)of a G-mixer In a Q-mixer,the input current available at the in commutating mode is drain of a transconductor transistor is switched第６期 犖犻犚狅狀犵犺狌犪犲狋犪犾．： 犃狀犪犾狔狊犻狊犪狀犱犇犲狊犻犵狀狅犳犪犙狌犪犱狉犪狋狌狉犲犇狅狑狀犆狅狀狏犲狉狊犻狅狀犕犻狓犲狉犳狅狉犝犎犉犚犉犐犇 犚犲犪犱犲狉狊 犉犻犵．２ 犜狑狅狋狅狆狅犾狅犵犻犲狊狅犳狇狌犪犱狉犪狋狌狉犲犱狅狑狀犮狅狀狏犲狉狊犻狅狀 （犪）犙狌犪犱狉犪狋狌狉犲犿犻狓犲狉狑犻狋犺狊犺犪狉犲犱狋狉犪狀狊犮狅狀犱狌犮狋狅狉 狊狋犪犵犲；（犫）犌犻犾犫犲狉狋犿犻狓犲狉狆犪犻狉 ２ 犃狀犪犾狔狊犻狊狅犳犪狇狌犪犱狉犪狋狌狉犲犿犻狓犲狉狑犻狋犺 狊犺犪狉犲犱狋狉犪狀狊犮狅狀犱狌犮狋狅狉狊狋犪犵犲 犙狌犪犱狉犪狋狌狉犲 犱狅狑狀犮狅狀狏犲狉狊犻狅狀 犮犪狀 犫犲 狉犲犪犾犻狕犲犱 狋犺狉狅狌犵犺狋狑狅 狋狅狆狅犾狅犵犻犲狊，犪狊狊犺狅狑狀 犻狀 犉犻犵２［３］，狋犺犲 狇狌犪犱狉犪狋狌狉犲 犿犻狓犲狉 狑犻狋犺狊犺犪狉犲犱狋狉犪狀狊犮狅狀犱狌犮狋狅狉狊狋犪犵犲 （犙犿犻狓犲狉）犪狀犱狋犺犲犮狅狀狏犲狀狋犻狅狀犪犾犌犻犾犫犲狉狋 犿犻狓犲狉狆犪犻狉 （犌犿犻狓犲狉）．犜狅犵犻狏犲犪狀狅狉犿犪犾犻狕犲犱狆犲狉犳狅狉犿犪狀犮犲犪狀犪犾狔 狊犻狊，狋犺犲狋狑狅狋狅狆狅犾狅犵犻犲狊犪狉犲 犱犲狊犻犵狀犲犱狋狅 犺犪狏犲犲狇狌犪犾 狆狅狑犲狉犱犻狊狊犻狆犪狋犻狅狀狌狀犱犲狉狋犺犲狊犪犿犲犫犻犪狊犮狅狀犱犻狋犻狅狀．犜犺犻狊 犻狊犪犮犺犻犲狏犲犱犫狔犿犪犽犻狀犵狋犺犲犵犪狋犲狑犻犱狋犺狅犳狋犺犲狋狉犪狀狊犮狅狀 犱狌犮狋狅狉狋狉犪狀狊犻狊狋狅狉狊犻狀狋犺犲犙犿犻狓犲狉狋狑犻犮犲狅犳狋犺犪狋犻狀狋犺犲 犌犿犻狓犲狉，犪狀犱 犿犪犽犻狀犵狅狋犺犲狉狆犪狉犪犿犲狋犲狉狊犻狀狊狑犻狋犮犺犻狀犵 狊狋犪犵犲犪狀犱犾狅犪犱狊狋犪犵犲犻犱犲狀狋犻犮犪犾．犐狀犪犱犱犻狋犻狅狀，狋犺犲犿犻狓犲狉狊 犪狉犲犪狊狊狌犿犲犱狋狅 狅狆犲狉犪狋犲犻狀 狋犺犲 犮狅犿犿狌狋犪狋犻狀犵 犿狅犱犲 犱狉犻狏犲狀犫狔犪犾犪狉犵犲狊犻狀狌狊狅犻犱犪犾犾狅犮犪犾狅狊犮犻犾犾犪狋狅狉（犔犗）狊犻犵 狀犪犾．犜犺犻狊犻狊狉犲犪狊狅狀犪犫犾犲犫犲犮犪狌狊犲狋犺犲犔犗狊犻犵狀犪犾狅犳犳犲狉犲犱 犫狔 犔犆 犞犆犗 犻狊 犵犲狀犲狉犪犾犾狔 犪犾犪狉犵犲 狊犻狀狌狊狅犻犱犪犾 狑犪狏犲 狋犺狉狅狌犵犺犫狌犳犳犲狉犻狀犵狅狉犳狉犲狇狌犲狀犮狔犱犻狏犻狊犻狅狀． ２．１ 犞狅犾狋犪犵犲犮狅狀狏犲狉狊犻狅狀犵犪犻狀犪狀犪犾狔狊犻狊 犜犺犲狏狅犾狋犪犵犲犮狅狀狏犲狉狊犻狅狀犵犪犻狀 （犆犌）狅犳犪犌犿犻狓犲狉 犻狀犮狅犿犿狌狋犪狋犻狀犵犿狅犱犲犻狊 犆犌犌 ＝犵犿，犌犌犛犠，犌犚犔 （１） 狑犺犲狉犲犵犿，犌 犪狀犱犌犛犠，犌 狉犲狆狉犲狊犲狀狋狋犺犲狋狉犪狀狊犮狅狀犱狌犮狋犪狀犮犲 狅犳狋犺犲狋狉犪狀狊犮狅狀犱狌犮狋狅狉狋狉犪狀狊犻狊狋狅狉犪狀犱狋犺犲狋狉犪狀狊犳犲狉犵犪犻狀 狅犳狋犺犲狊狑犻狋犮犺犻狀犵狆犪犻狉犻狀狋犺犲犌犿犻狓犲狉，狉犲狊狆犲犮狋犻狏犲犾狔．犜犺犲 犆犌狋狅犲犪犮犺狅狌狋狆狌狋狆犪狋犺犻狀犪犙犿犻狓犲狉犻狊 犆犌犙 ＝犵犿，犙犌犛犠，犙犚犔 （２） 狑犺犲狉犲犵犿，犙 犪狀犱犌犛犠，犙 狉犲狆狉犲狊犲狀狋狋犺犲狋狉犪狀狊犮狅狀犱狌犮狋犪狀犮犲 狅犳狋犺犲狋狉犪狀狊犮狅狀犱狌犮狋狅狉狋狉犪狀狊犻狊狋狅狉犪狀犱狋犺犲狋狉犪狀狊犳犲狉犵犪犻狀 狅犳狋犺犲狊狑犻狋犮犺犻狀犵狆犪犻狉犻狀狋犺犲犙犿犻狓犲狉，狉犲狊狆犲犮狋犻狏犲犾狔．犃犮 犮狅狉犱犻狀犵狋狅狋犺犲犫犻犪狊犻狀犵犪狀犱狊犻狕犲狅犳狋犺犲狋狉犪狀狊犮狅狀犱狌犮狋狅狉 狊狋犪犵犲， 犵犿，犙 ＝２犵犿，犌 （３） 犜犺犲狏犪犾狌犲狊狅犳犌犛犠，犌 犪狀犱犌犛犠，犙 犮犪狀犫犲犮犪犾犮狌犾犪狋犲犱 犳狉狅犿狋犺犲犻犱犲犪犾狋狉犪狀狊犳犲狉 狑犪狏犲犳狅狉犿 狅犳狋犺犲狊狑犻狋犮犺犻狀犵 狆犪犻狉犻犾犾狌狊狋狉犪狋犲犱犻狀犉犻犵３．犐狀犪犌犿犻狓犲狉，狋犺犲犻狀狆狌狋犮狌狉 狉犲狀狋犪狏犪犻犾犪犫犾犲犪狋狋犺犲犱狉犪犻狀狅犳犪狋狉犪狀狊犮狅狀犱狌犮狋狅狉狋狉犪狀 狊犻狊狋狅狉犻狊狊狑犻狋犮犺犲犱狋犺狉狅狌犵犺犲犪犮犺狅犳狋犺犲狋狑狅狊狑犻狋犮犺犻狀犵 狋狉犪狀狊犻狊狋狅狉狊犳狅狉犺犪犾犳狅犳狋犺犲犔犗 狆犲狉犻狅犱．犜犺犲狉犲犳狅狉犲，狋犺犲 犻狀狆狌狋犮狌狉狉犲狀狋犻狊犿狌犾狋犻狆犾犻犲犱犫狔犪狊狇狌犪狉犲 狑犪狏犲，狑犺狅狊犲 犉狅狌狉犻犲狉狊犲狉犻犲狊犲狓狆犪狀狊犻狅狀犻狊 犳犌（狋）＝ ∑ ∞ 犽＝－∞ 狊犻狀（犽π／２） 犽π／２ ×犲犼犽ω犔犗狋 （４） 犐狀犪犙犿犻狓犲狉，狋犺犲犻狀狆狌狋犮狌狉狉犲狀狋犪狏犪犻犾犪犫犾犲犪狋狋犺犲 犱狉犪犻狀 狅犳 犪 狋狉犪狀狊犮狅狀犱狌犮狋狅狉 狋狉犪狀狊犻狊狋狅狉 犻狊 狊狑犻狋犮犺犲犱 １１２９