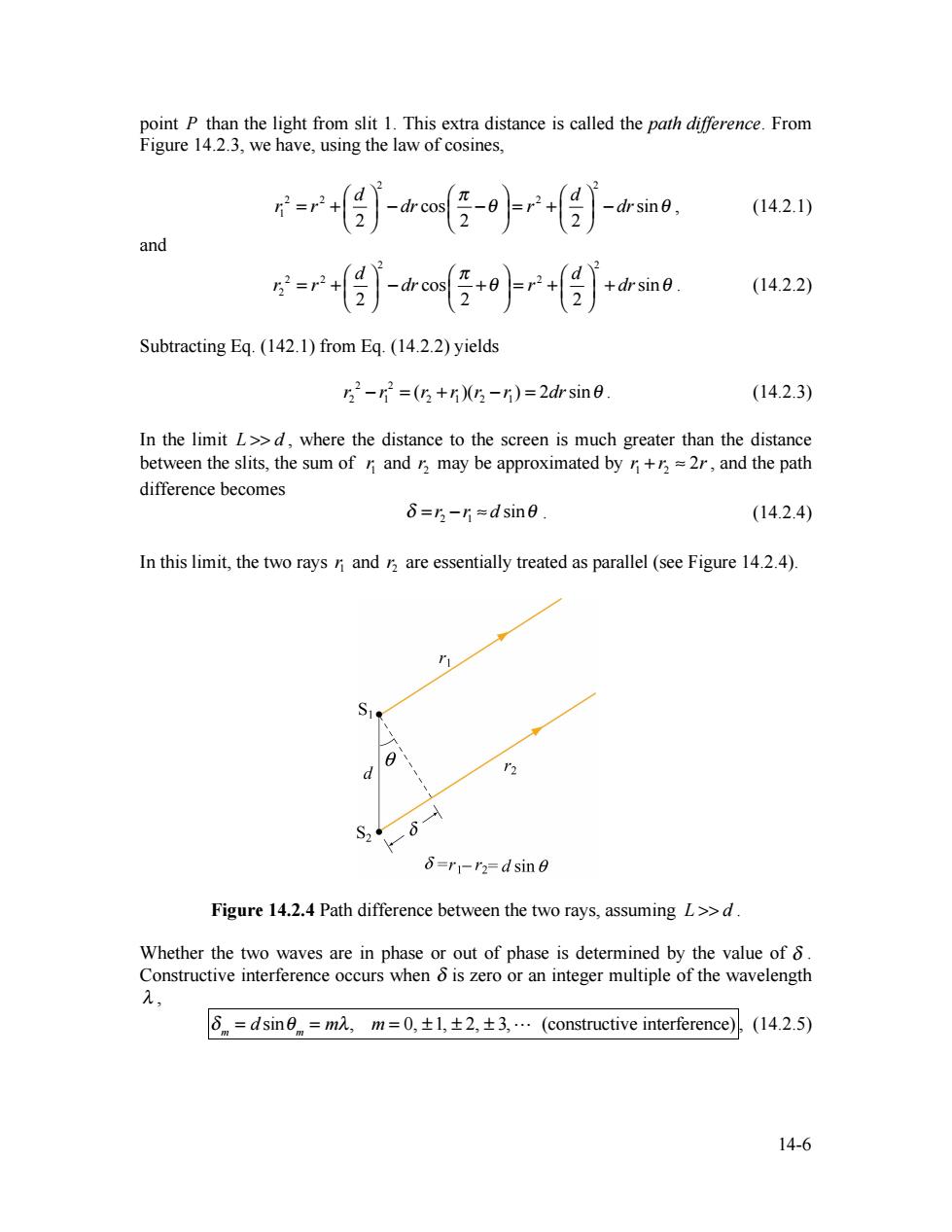
point P than the light from slit 1.This extra distance is called the path difference.From Figure 14.2.3,we have,using the law of cosines, =r侣j-a任-+m. (14.2.1) and =r+-m任+r+侵 (14.2.2) Subtracting Eq.(142.1)from Eq.(14.2.2)yields 52-r2=(5+r)5-r)=2 drsin6. (14.2.3) In the limit L>>d,where the distance to the screen is much greater than the distance between the slits,the sum of n and r may be approximated by r+r=2r,and the path difference becomes δ=5-片=dsin6. (14.2.4) In this limit,the two rays ni and r are essentially treated as parallel (see Figure 14.2.4). S1 d '2 S2 8=r-r2=dsine Figure 14.2.4 Path difference between the two rays,assuming L>>d. Whether the two waves are in phase or out of phase is determined by the value of 8. Constructive interference occurs when is zero or an integer multiple of the wavelength 2, δn=dsin9n=m2,m=O,±l,±2，±3，.(constructive interference) (14.2.5) 14-614-6 point P than the light from slit 1. This extra distance is called the path difference. From Figure 14.2.3, we have, using the law of cosines, 2 2 2 2 2 1 cos sin 2 2 2 d d r r dr r dr π θ θ ⎛ ⎞ ⎛ ⎞ ⎛ ⎞ = + ⎜ ⎟ − ⎜ − ⎟ = + ⎜ ⎟ − ⎝ ⎠ ⎝ ⎠ ⎝ ⎠ , (14.2.1) and 2 2 2 2 2 2 cos sin 2 2 2 d d r r dr r dr π θ θ ⎛ ⎞ ⎛ ⎞ ⎛ ⎞ = + ⎜ ⎟ − ⎜ + ⎟ = + ⎜ ⎟ + ⎝ ⎠ ⎝ ⎠ ⎝ ⎠ . (14.2.2) Subtracting Eq. (142.1) from Eq. (14.2.2) yields 2 2 2 1 2 1 2 1 r − r = (r + r )(r − r ) = 2drsinθ . (14.2.3) In the limit L >> d , where the distance to the screen is much greater than the distance between the slits, the sum of 1 r and 2r may be approximated by 1 2 r + r ≈ 2r, and the path difference becomes 2 1 δ = r − r ≈ d sinθ . (14.2.4) In this limit, the two rays 1 r and 2r are essentially treated as parallel (see Figure 14.2.4). Figure 14.2.4 Path difference between the two rays, assuming L >> d . Whether the two waves are in phase or out of phase is determined by the value of δ . Constructive interference occurs when δ is zero or an integer multiple of the wavelength λ , δ m = d sinθ m = mλ, m = 0, ± 1, ± 2, ± 3, (constructive interference) , (14.2.5)