正在加载图片...
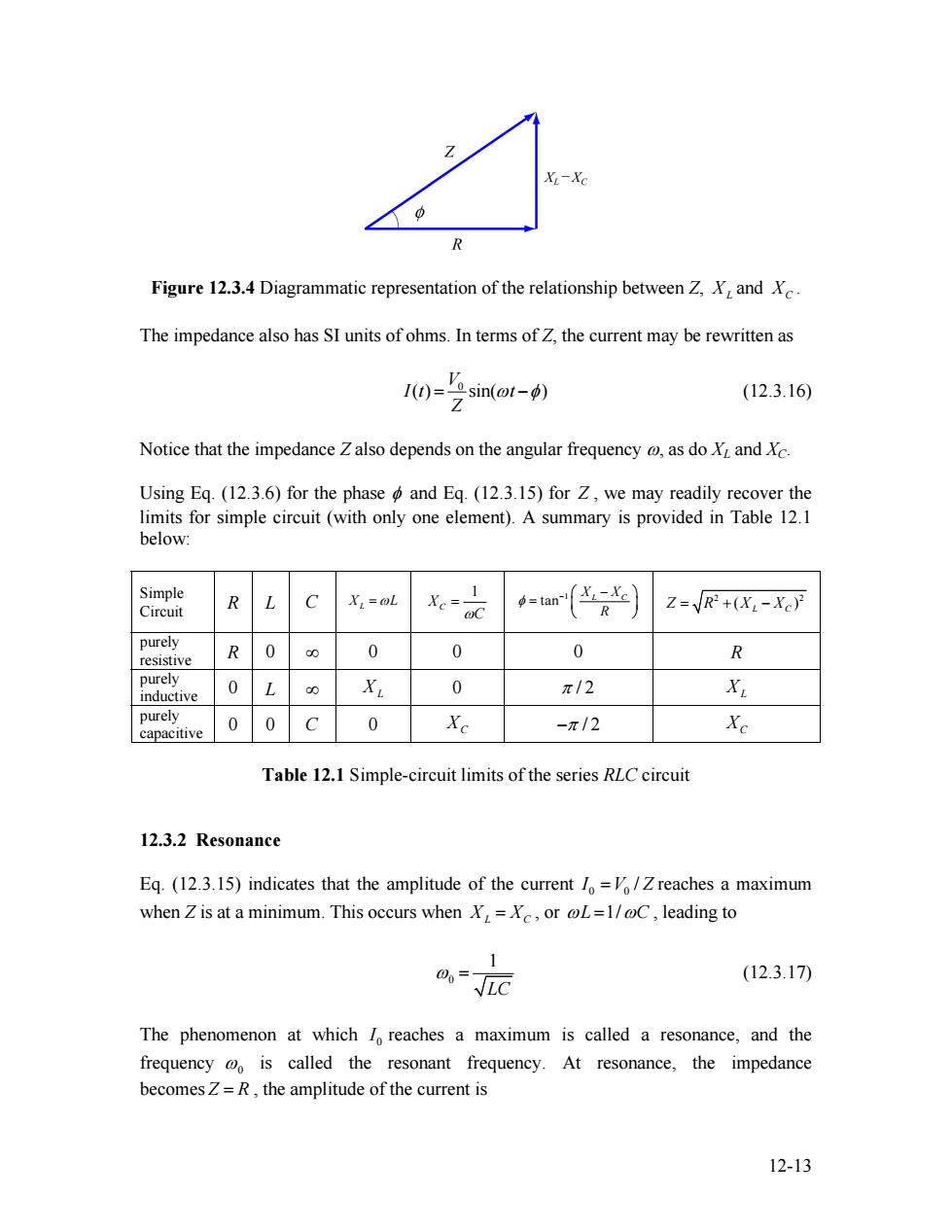
XL-Xc R Figure 12.3.4 Diagrammatic representation of the relationship between Z,X,and X The impedance also has SI units of ohms.In terms of Z,the current may be rewritten as I0=台-sin(o1-) (12.3.16 Notice that the impedance Z also depends on the angular frequency @as do XL and Xc. Using Eq.(12.3.6)for the phase and Eq.(12.3.15)for Z,we may readily recover the limits for simple circuit(with only one element).A summary is provided in Table 12.1 below: Simple C Circuit R L XL=0L Xc= OC Z=yR2+(XL-Xc) purely R 0 00 0 0 0 resistive R purely 0 inductive L 00 XL 0 π/2 X purely capacitive 0 0 C 0 Xc -π/2 Xc Table 12.1 Simple-circuit limits of the series RLC circuit 12.3.2 Resonance Eq.(12.3.15)indicates that the amplitude of the current 1=/Zreaches a maximum when Z is at a minimum.This occurs when X.=Xc,or L=1/@C,leading to 1 0o-JIC (12.3.17) The phenomenon at which reaches a maximum is called a resonance,and the frequency @o is called the resonant frequency.At resonance,the impedance becomes Z=R,the amplitude of the current is 12-13Figure 12.3.4 Diagrammatic representation of the relationship between Z, XL and XC . The impedance also has SI units of ohms. In terms of Z, the current may be rewritten as 0 ( ) sin( ) V I t t Z = ω −φ (12.3.16) Notice that the impedance Z also depends on the angular frequency ω, as do XL and XC. Using Eq. (12.3.6) for the phase φ and Eq. (12.3.15) for Z , we may readily recover the limits for simple circuit (with only one element). A summary is provided in Table 12.1 below: Simple Circuit R L C XL =ωL 1 X C ωC = 1 tan X X L C R φ − ⎛ ⎞ − = ⎜ ⎟ ⎝ ⎠ 2 2 ( ) Z R = + XL C − X purely resistive R 0 ∞ 0 0 0 R purely inductive 0 L ∞ XL 0 π / 2 XL purely capacitive 0 0 C 0 XC −π / 2 XC Table 12.1 Simple-circuit limits of the series RLC circuit 12.3.2 Resonance Eq. (12.3.15) indicates that the amplitude of the current 0 0 I =V / Z reaches a maximum when Z is at a minimum. This occurs when XL = XC , or ωL =1/ωC , leading to 0 1 LC ω = (12.3.17) The phenomenon at which 0 I reaches a maximum is called a resonance, and the frequency ω0 is called the resonant frequency. At resonance, the impedance becomes Z = R , the amplitude of the current is 12-13