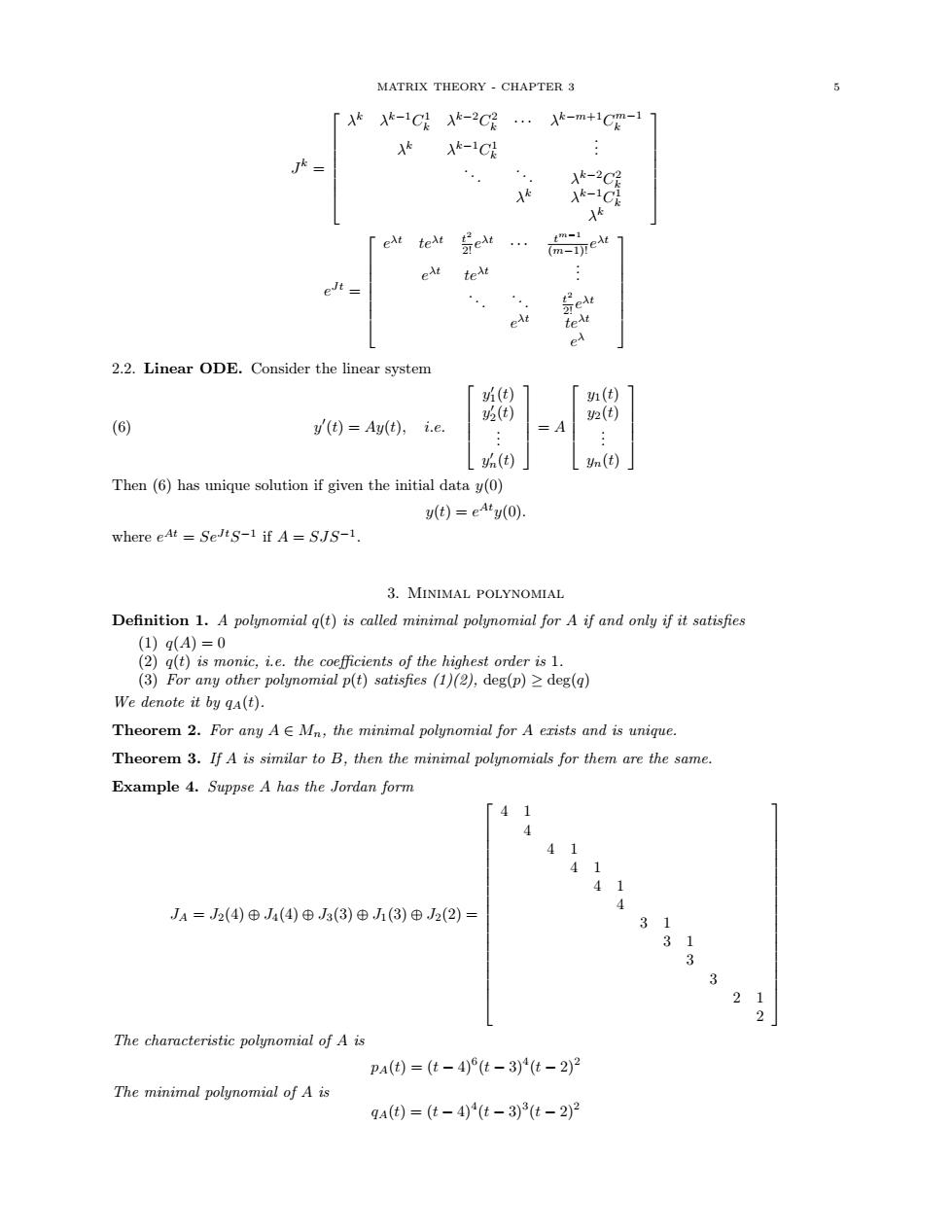
MATRIX THEORY-CHAPTER 3 -1C以λ-2C喉…-m+1CW-11 kAk-1C以 J 1k [ext text号et: 2.2.Linear ODE.Consider the linear system ()=A(),i.e =A 6() un(t) Then(6)has unique solution if given the initial data) =e4o). where eAt SeJts-1 if A=SJS-1. 3.MINIMAL POLYNOMIAL Definition 1.A polymomial (t)is called minimal polynomial for A if and only if it satisfies 智PEah时Aeom (3)For any other polymomial p(t)satisfies(1)(2),deg(p)z deg(q) We denote it by qa(t). Theorem 2.For any AMa,the minimal polynomial for A erists and is unique. Theorem 3.If A is similar to B,then the minimal polyomials for them are the same. Example 4.Suppse A has the Jordan form 「4 1 41 JA=2(⊕（④⊕(3）⊕(3)⊕J2(2 31 3 21 The characteristic polynomial of A is pA()=(t-4)(t-3)(t-2)2 The minimal polynomial of A is gA()=(t-4)1(t-3)3(t-2)2 MATRIX THEORY - CHAPTER 3 5 J k =         λ k λ k−1C 1 k λ k−2C 2 k · · · λ k−m+1C m−1 k λ k λ k−1C 1 k . . . . . . . . . λ k−2C 2 k λ k λ k−1C 1 k λ k         e Jt =         e λt teλt t 2 2! e λt · · · tm−1 (m−1)! e λt e λt teλt . . . . . . . . . t 2 2! e λt e λt teλt e λ         2.2. Linear ODE. Consider the linear system (6) y 0 (t) = Ay(t), i.e.      y 0 1 (t) y 0 2 (t) . . . y 0 n (t)      = A      y1(t) y2(t) . . . yn(t)      Then (6) has unique solution if given the initial data y(0) y(t) = e Aty(0). where e At = SeJtS −1 if A = SJS−1 . 3. Minimal polynomial Definition 1. A polynomial q(t) is called minimal polynomial for A if and only if it satisfies (1) q(A) = 0 (2) q(t) is monic, i.e. the coefficients of the highest order is 1. (3) For any other polynomial p(t) satisfies (1)(2), deg(p) ≥ deg(q) We denote it by qA(t). Theorem 2. For any A ∈ Mn, the minimal polynomial for A exists and is unique. Theorem 3. If A is similar to B, then the minimal polynomials for them are the same. Example 4. Suppse A has the Jordan form JA = J2(4) ⊕ J4(4) ⊕ J3(3) ⊕ J1(3) ⊕ J2(2) =                     4 1 4 4 1 4 1 4 1 4 3 1 3 1 3 3 2 1 2                     The characteristic polynomial of A is pA(t) = (t − 4)6 (t − 3)4 (t − 2)2 The minimal polynomial of A is qA(t) = (t − 4)4 (t − 3)3 (t − 2)2