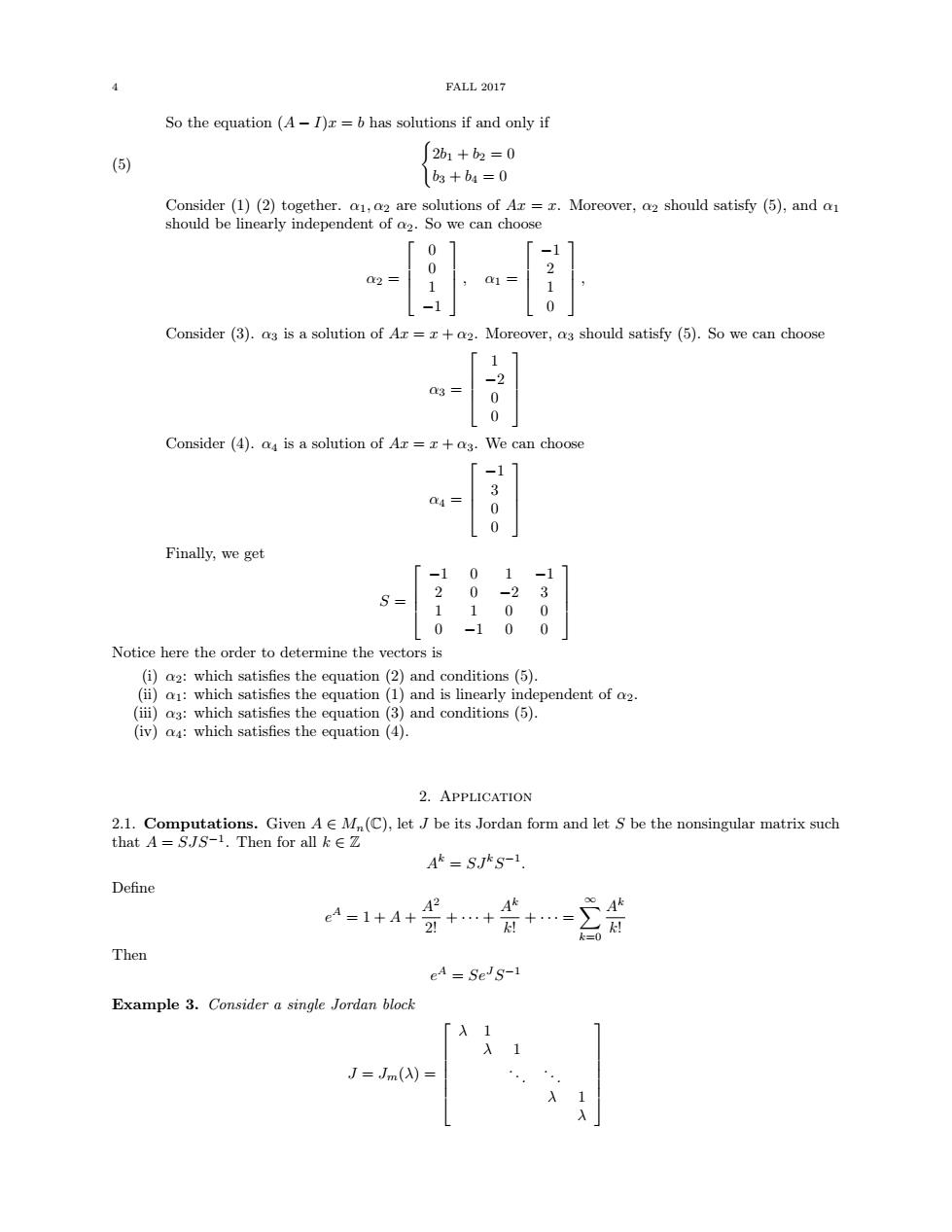
4 FALL 2017 So the equation (A-I)z=b has solutions if and only if 2b1+2=0 b3+b4=0 2 01 -11 0 Consider (3).os is a solution of Ar=r+a2.Moreover,a3 should satisfy (5).So we can choose 17 a3= 0 Consider (4).a is a solution of Ar=+.We can choose -1 Finally,we get s- Notice here the order to determine the vectors is ependent of o (iv):which satisfies the equation (4). 2.APPLICATION 名品各C,bte Ak SS-1 Define 1+4+++若+…一2甜 Then eA Se/S-1 Example 3.Consider a single Jordan block 1 J=Jm(X)=4 FALL 2017 So the equation (A − I)x = b has solutions if and only if (5) ( 2b1 + b2 = 0 b3 + b4 = 0 Consider (1) (2) together. α1, α2 are solutions of Ax = x. Moreover, α2 should satisfy (5), and α1 should be linearly independent of α2. So we can choose α2 =     0 0 1 −1     , α1 =     −1 2 1 0     , Consider (3). α3 is a solution of Ax = x + α2. Moreover, α3 should satisfy (5). So we can choose α3 =     1 −2 0 0     Consider (4). α4 is a solution of Ax = x + α3. We can choose α4 =     −1 3 0 0     Finally, we get S =     −1 0 1 −1 2 0 −2 3 1 1 0 0 0 −1 0 0     Notice here the order to determine the vectors is (i) α2: which satisfies the equation (2) and conditions (5). (ii) α1: which satisfies the equation (1) and is linearly independent of α2. (iii) α3: which satisfies the equation (3) and conditions (5). (iv) α4: which satisfies the equation (4). 2. Application 2.1. Computations. Given A ∈ Mn(C), let J be its Jordan form and let S be the nonsingular matrix such that A = SJS−1 . Then for all k ∈ Z A k = SJkS −1 . Define e A = 1 + A + A2 2! + · · · + Ak k! + · · · = X∞ k=0 Ak k! Then e A = SeJ S −1 Example 3. Consider a single Jordan block J = Jm(λ) =        λ 1 λ 1 . . . . . . λ 1 λ       