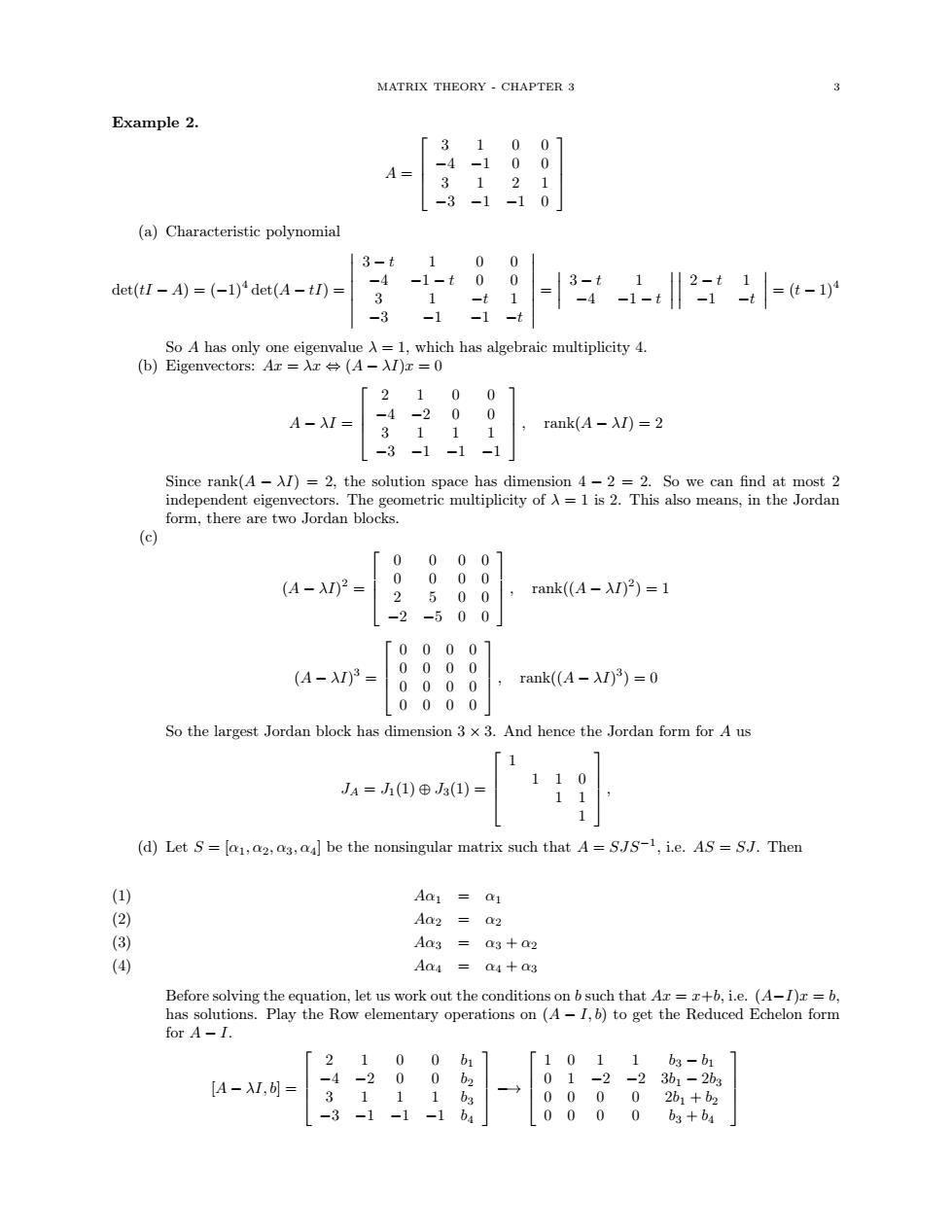
MATRIX THEORY CHAPTER 3 3 Example 2. (a)Characteristic polynomial 3-t det(tI-A)=(-1)det(A-tI)= -1 lw- -1-t0 So A has only one eigenvalue A=1,which has algebraic multiplicity 4. (b)Eigenvectors:Ar=(AAl)r=0 1 A-AI= 311 rank(A-AI)=2 -3-1-1-1 Since rank(A-Al)=2.the solution space has dimension 4-2=2.So we can find at most 2 independent eigenvecorin bleekcometrie multipucity or 2.This also means,in the Jordan form,there are two (e) 88881 「0 (4-λI)2= 500 rank((A-AD)2)=1 2 -2-500 「00001 (4-A)3 888 rank((A-AI))=0 0000 So the largest Jordan block has dimension 3 x 3.And hence the Jordan form for A us 「1 JA=(1)3(1) 110 11 1 (d)Let S=[a1,a2,a3,aa]be the nonsingular matrix such that A=S.JS-1,i.e.AS=SJ.Then Ao1 =01 四 A02=02 Aa3 =03+02 Aa4 04+03 Before solving the equation,let us work out the conditions on bsuch that Ar=r+b,i.e.(A-I)=b, Ply the Row etary peo(togt th Reede 2 0 0 10 [A-AI,6]= 01-2-2361-2h MATRIX THEORY - CHAPTER 3 3 Example 2. A =     3 1 0 0 −4 −1 0 0 3 1 2 1 −3 −1 −1 0     (a) Characteristic polynomial det(tI − A) = (−1)4 det(A − tI) =         3 − t 1 0 0 −4 −1 − t 0 0 3 1 −t 1 −3 −1 −1 −t         =     3 − t 1 −4 −1 − t         2 − t 1 −1 −t     = (t − 1)4 So A has only one eigenvalue λ = 1, which has algebraic multiplicity 4. (b) Eigenvectors: Ax = λx ⇔ (A − λI)x = 0 A − λI =     2 1 0 0 −4 −2 0 0 3 1 1 1 −3 −1 −1 −1     , rank(A − λI) = 2 Since rank(A − λI) = 2, the solution space has dimension 4 − 2 = 2. So we can find at most 2 independent eigenvectors. The geometric multiplicity of λ = 1 is 2. This also means, in the Jordan form, there are two Jordan blocks. (c) (A − λI) 2 =     0 0 0 0 0 0 0 0 2 5 0 0 −2 −5 0 0     , rank((A − λI) 2 ) = 1 (A − λI) 3 =     0 0 0 0 0 0 0 0 0 0 0 0 0 0 0 0     , rank((A − λI) 3 ) = 0 So the largest Jordan block has dimension 3 × 3. And hence the Jordan form for A us JA = J1(1) ⊕ J3(1) =     1 1 1 0 1 1 1     , (d) Let S = [α1, α2, α3, α4] be the nonsingular matrix such that A = SJS−1 , i.e. AS = SJ. Then (1) Aα1 = α1 (2) Aα2 = α2 (3) Aα3 = α3 + α2 (4) Aα4 = α4 + α3 Before solving the equation, let us work out the conditions on b such that Ax = x+b, i.e. (A−I)x = b, has solutions. Play the Row elementary operations on (A − I, b) to get the Reduced Echelon form for A − I. [A − λI, b] =     2 1 0 0 b1 −4 −2 0 0 b2 3 1 1 1 b3 −3 −1 −1 −1 b4     −→     1 0 1 1 b3 − b1 0 1 −2 −2 3b1 − 2b3 0 0 0 0 2b1 + b2 0 0 0 0 b3 + b4    