正在加载图片...
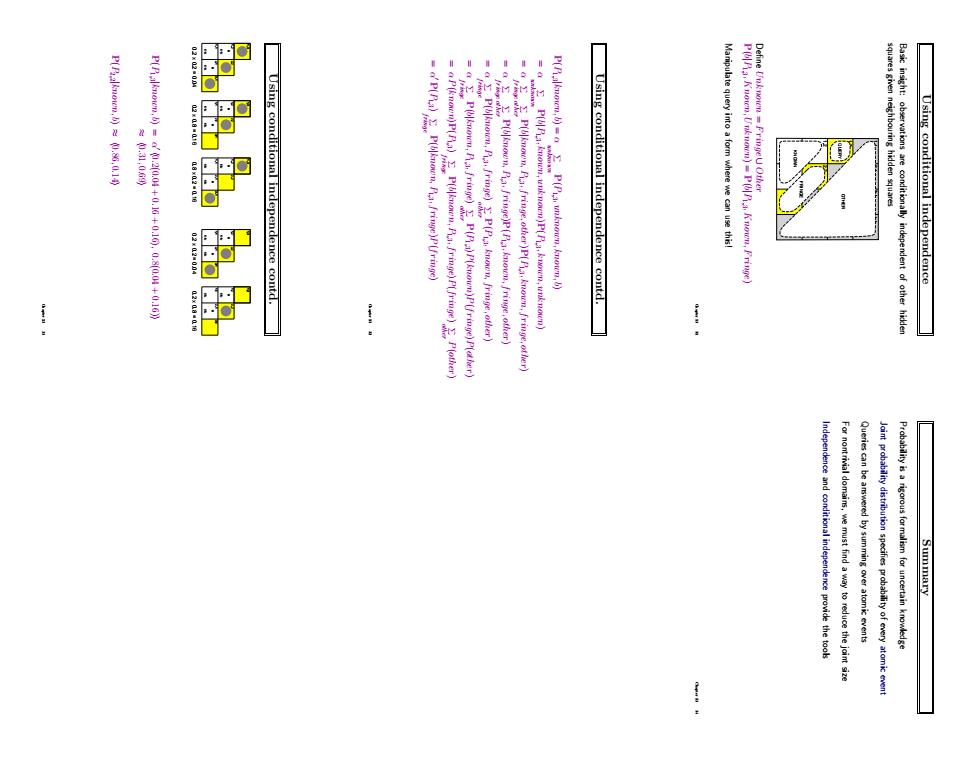
P(,)0.8,0.14 P(Poien,5)o/0.2(0.04+0.16+0.1G),0.8(0.04+0.16) 08 x02-016 Using conditional independence contd. P(Pa)P(e,Pia.fringe)p(fringe) =Pnown,Fia.fringe)P(P(nonen)P(fringe)P( P(nown,Pia.fringeP(P,known,fringe,other) P(blinowen.Pi.fringe,other)P(P.known,fringe.other Using conditional independence contd. Manipulate queryinto a form where we can use this! Using conditional independence Independence and conditional independence provide the tools Fornontrivadomaiswe must find a way toreuce the joint size Queries can be answered by summing over atomic events SummaryUsing conditional independence Basic insight: observations are conditionally independent of other hidden squares given neighbouring hidden squares 1,1 2,1 3,1 4,1 1,2 2,2 3,2 4,2 1,3 2,3 3,3 4,3 1,4 2,4 3,4 4,4 KNOWN FRINGE QUERY OTHER Define Unknown = Fringe ∪ Other P(b|P1,3, Known,Unknown) = P(b|P1,3, Known, Fringe) Manipulate query into a form where we can use this! Chapter 13 31 Using conditional independence contd. P(P1,3|known, b) = α X unknown P(P1,3, unknown, known, b) = α X unknown P(b|P1,3, known, unknown)P(P1,3, known, unknown) = α X fringe X other P(b|known,P1,3, fringe, other)P(P1,3, known, fringe, other) = α X fringe X other P(b|known,P1,3, fringe)P(P1,3, known, fringe, other) = α X fringe P(b|known,P1,3, fringe) X other P(P1,3, known, fringe, other) = α X fringe P(b|known,P1,3, fringe) X other P(P1,3)P(known)P(fringe)P(other) = α P(known)P(P1,3) X fringe P(b|known,P1,3, fringe)P(fringe) X other P(other) = α 0 P(P1,3) X fringe P(b|known,P1,3, fringe)P(fringe) Chapter 13 32 Using conditional independence contd. OK 1,1 2,1 3,1 1,2 2,2 1,3 OK OK B B OK 1,1 2,1 3,1 1,2 2,2 1,3 OK OK B B OK 1,1 2,1 3,1 1,2 2,2 1,3 OK OK B B 0.2 x 0.2 = 0.04 0.2 x 0.8 = 0.16 0.8 x 0.2 = 0.16 OK 1,1 2,1 3,1 1,2 2,2 1,3 OK OK B B OK 1,1 2,1 3,1 1,2 2,2 1,3 OK OK B B 0.2 x 0.2 = 0.04 0.2 x 0.8 = 0.16 P(P1,3|known, b) = α 0 h0.2(0.04 + 0.16 + 0.16), 0.8(0.04 + 0.16)i ≈ h0.31, 0.69i P(P2,2|known, b) ≈ h0.86, 0.14i Chapter 13 33 Summary Probability is a rigorous formalism for uncertain knowledge Joint probability distribution specifies probability of every atomic event Queries can be answered by summing over atomic events For nontrivial domains, we must find a way to reduce the joint size Independence and conditional independence provide the tools Chapter 13 34