正在加载图片...
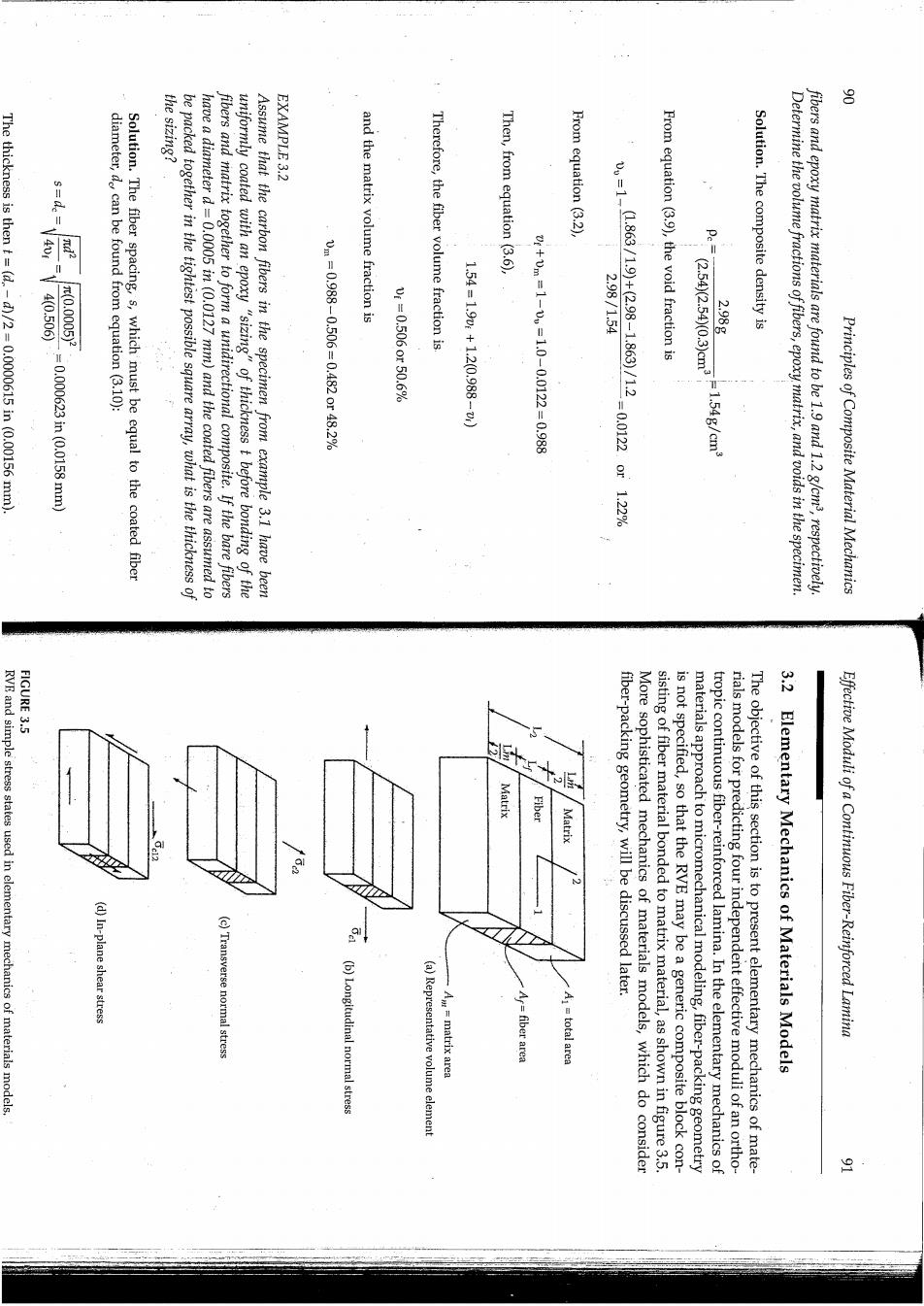
the sizing? EXAMPLE 3.2 The thickness is then t=(d.-d)/2=0.0000615 in(0.00156 mm) V40.506 (.0.00063in (00156 mm) diameter,do can be found from equation (3.10): Solution.The fiber spacing,s,which must be equal to the coated fiber be packed together in the tightest possible square array,what is the thickness of have a diameter d=0.0005 in (0.0127 mm)and the coated fibers are assumed to fibers and matrix together to form a unidirectional composite.If the bare fibers uniformly coated with an epoxy "sizing"of thickness t before bonding of the Assume that the carbon fibers in the specimen from example 3.1 have been and the matrix volume fraction is Therefore,the fiber volume fraction is Then,from equation (3.6), From equation(3.2) 10-0988-0.506-04320748.22% 15-0.5060750699 1.54-190541.20988-2V 5+00-1-00-1.0-00122-0288 u-1-0Ma/1gte%/0.809y12-00m0g1308 2.9871.54 From equation (3.9),the void fraction is P.(2.54)(2.54)0.3)cm Solution.The composite density is 2018 F1.548/cma Determine the volume fractions of fibers,epoxy matrix,and voids in the specimen. fibers and epoxy matrix materials are found to be 1.9 and 1.2 g/cm3,respectively. Principles of Composite Material Mechanics FIGURE 3.5 RVE and simple stress states used in elementary mechanics of materials models. Matrix Fiber Matrix (d)In-plane shear stress fiber-packing geometry,will be discussed later. (c)Transverse normal stress (b)Longitudinal normal stress (a)Representative volume element A=matrix area Ar=fber area A=total area More sophisticated mechanics of materials models,which do consider sisting of fiber material bonded to matrix material,as shown in figure 3.5 is not specified,so that the RVE may be a generic composite block con- materials approach to micromechanical modeling,fiber-packing geometry tropic continuous fiber-reinforced lamina.In the elementary mechanics of rials models for predicting four independent effective moduli of an ortho- The objective of this section is to present elementary mechanics of mate- Elementary Mechanics of Materials Models Effective Moduli of a Continuous Fiber-Reinforced Lamina