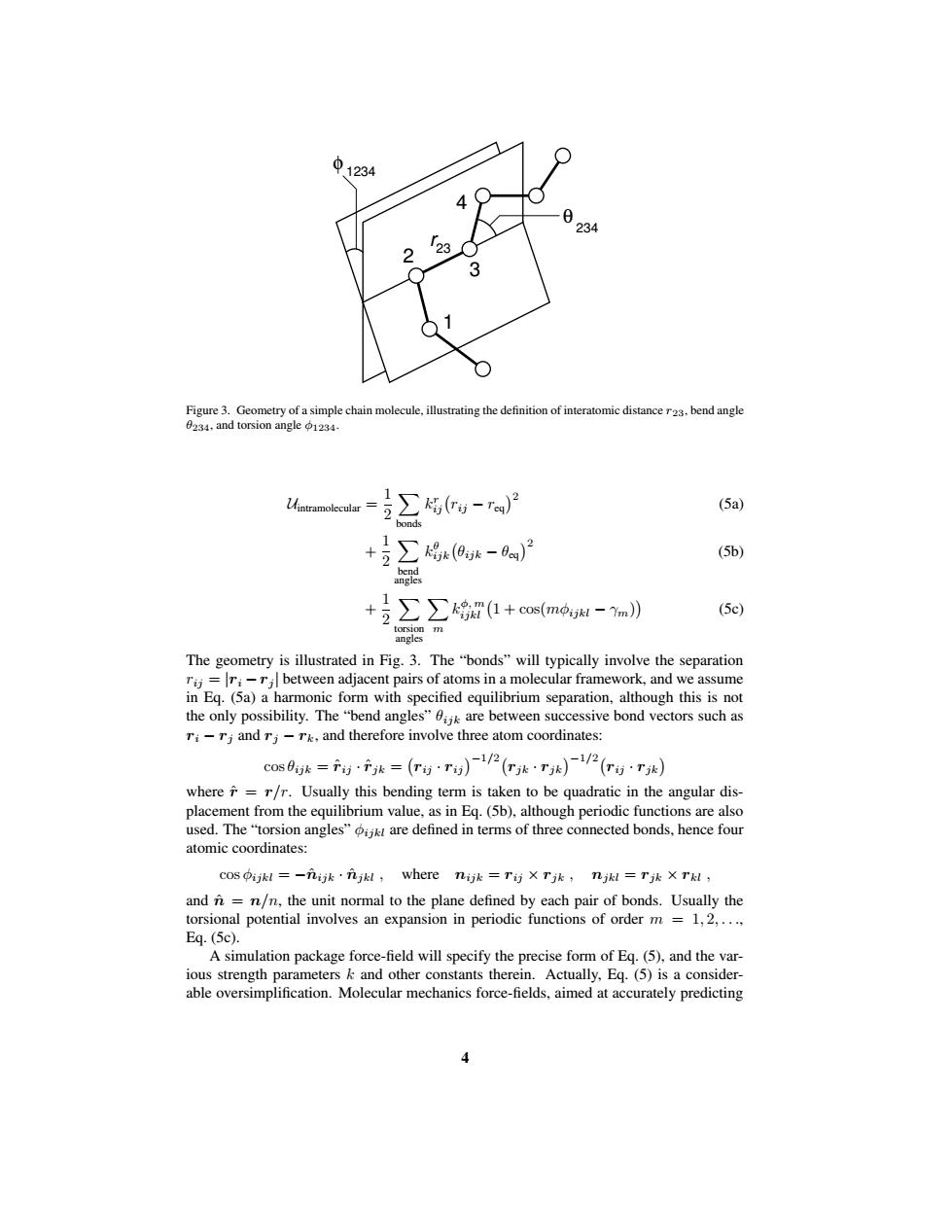
中1234 234 3 Figure 3.Geometry of a simple chain molecule,illustrating the definition of interatomic distance r23.bend angle 0234.and torsion angle 1234. Uintramolecular= ∑5w-r网 (5a) bonds +互∑号9-)2 (5b) bend angles +号∑∑g量1+smo,u-n》 (5c) mem The geometry is illustrated in Fig.3.The "bonds"will typically involve the separation r;=r:-ri between adjacent pairs of atoms in a molecular framework,and we assume in Eq.(5a)a harmonic form with specified equilibrium separation,although this is not the only possibility.The"bend angles"k are between successive bond vectors such as ri-rj and rj-rk,and therefore involve three atom coordinates: cosk==()12()2() where=r/r.Usually this bending term is taken to be quadratic in the angular dis- placement from the equilibrium value,as in Eq.(5b),although periodic functions are also used.The"torsion angles"are defined in terms of three connected bonds,hence four atomic coordinates: cos ijkl=-元k·元jkl,where nijk=T)XTjk,njkl=Tjk X Tkl, and n=n/n,the unit normal to the plane defined by each pair of bonds.Usually the torsional potential involves an expansion in periodic functions of order m =1,2,.... Eq.(5c). A simulation package force-field will specify the precise form of Eq.(5),and the var- ious strength parameters k and other constants therein.Actually,Eq.(5)is a consider- able oversimplification.Molecular mechanics force-fields,aimed at accurately predicting1 3 φ 1234 234 4 2 θ 23 r Figure 3. Geometry of a simple chain molecule, illustrating the definition of interatomic distance r23, bend angle θ234, and torsion angle φ1234. Uintramolecular = 1 2 X bonds k r ij ￾ rij − req
2 (5a) + 1 2 X bend angles k θ ijk ￾ θijk − θeq
2 (5b) + 1 2 X torsion angles X m k φ, m ijkl ￾ 1 + cos(mφijkl − γm)
(5c) The geometry is illustrated in Fig. 3. The “bonds” will typically involve the separation rij = |ri −rj | between adjacent pairs of atoms in a molecular framework, and we assume in Eq. (5a) a harmonic form with specified equilibrium separation, although this is not the only possibility. The “bend angles” θijk are between successive bond vectors such as ri − rj and rj − rk, and therefore involve three atom coordinates: cos θijk = rˆij · rˆjk = ￾ rij · rij
−1/2￾ rjk · rjk
−1/2￾ rij · rjk
where rˆ = r/r. Usually this bending term is taken to be quadratic in the angular dis￾placement from the equilibrium value, as in Eq. (5b), although periodic functions are also used. The “torsion angles” φijkl are defined in terms of three connected bonds, hence four atomic coordinates: cos φijkl = −nˆijk · nˆjkl , where nijk = rij × rjk , njkl = rjk × rkl , and nˆ = n/n, the unit normal to the plane defined by each pair of bonds. Usually the torsional potential involves an expansion in periodic functions of order m = 1, 2, . . ., Eq. (5c). A simulation package force-field will specify the precise form of Eq. (5), and the var￾ious strength parameters k and other constants therein. Actually, Eq. (5) is a consider￾able oversimplification. Molecular mechanics force-fields, aimed at accurately predicting 4