正在加载图片...
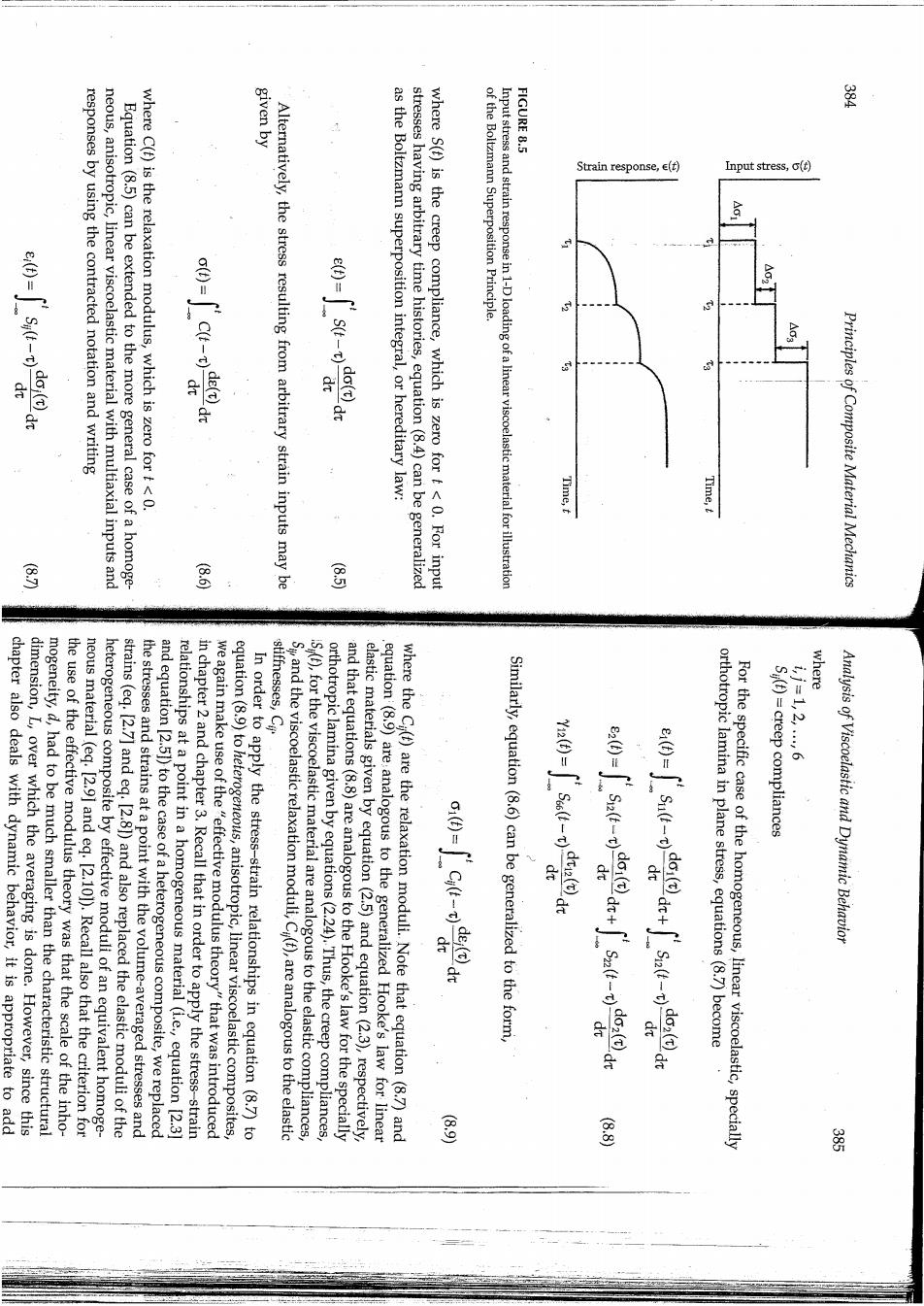
given by FIGURE 8.5 responses by using the contracted notation and writing Strain response,E(t Input stress,o(t) neous,anisotropic,linear viscoelastic material with multiaxial inputs and Equation(8.5)can be extended to the more general case of a homoge- where C(t)is the relaxation modulus,which is zero for t<0. Alternatively,the stress resulting from arbitrary strain inputs may be of the Boltzmann Superposition Principle. do(dr as the Boltzmann superposition integral,or hereditary law: stresses having arbitrary time histories,equation(8.4)can be generalized where S(t)is the creep compliance,which is zero for t<0.For input Input stress and strain response in1-Dloading of a linear viscoelastic material for illustration Time,t Time, Principles of Composite Material Mechanics 丑 stiffnesses,C where chapter also deals with dynamic behavior,it is appropriate to add dimension,L,over which the averaging is done.However,since this mogeneity,d,had to be much smaller than the characteristic structural the use of the effective modulus theory was that the scale of the inho- neous material (eq.[2.9]and eq.[2.101).Recall also that the criterion for heterogeneous composite by effective moduli of an equivalent homoge- the stresses and strains at a point with the volume-averaged stresses and strains (eq.[2.7]and eq.[2.81)and also replaced the elastic moduli of the relationships at a point in a homogeneous material (i.e.,equation [2.3] and equation [2.5])to the case of a heterogeneous composite,we replaced in chapter 2 and chapter 3.Recall that in order to apply the stress-strain equation(8.9)to heterogeneous,anisotropic,linear viscoelastic composites, we again make use of the "effective modulus theory"that was introduced In order to apply the stress-strain relationships in equation(8.7)to Sand the viscoelastic relaxation moduli,C(),are analogous to the elastic S),for the viscoelastic material are analogous to the elastic compliances, and that equations(8.8)are analogous to the Hooke's law for the specially orthotropic lamina given by equations(2.24).Thus,the creep compliances, equation(8.9)are:analogous to the generalized Hooke's law for linear elastic materials given by equation(2.5)and equation(2.3),respectively, where the C(t)are the relaxation moduli.Note that equation(8.7)and Similarly,equation(8.6)can be generalized to the form, orthotropic lamina in plane stress,equations(8.7)become S(t)=creep compliances 17512.r6 For the specific case of the homogeneous,linear viscoelastic,specially Analysis of Viscoelastic and Dynamic Behavior 思 图