正在加载图片...
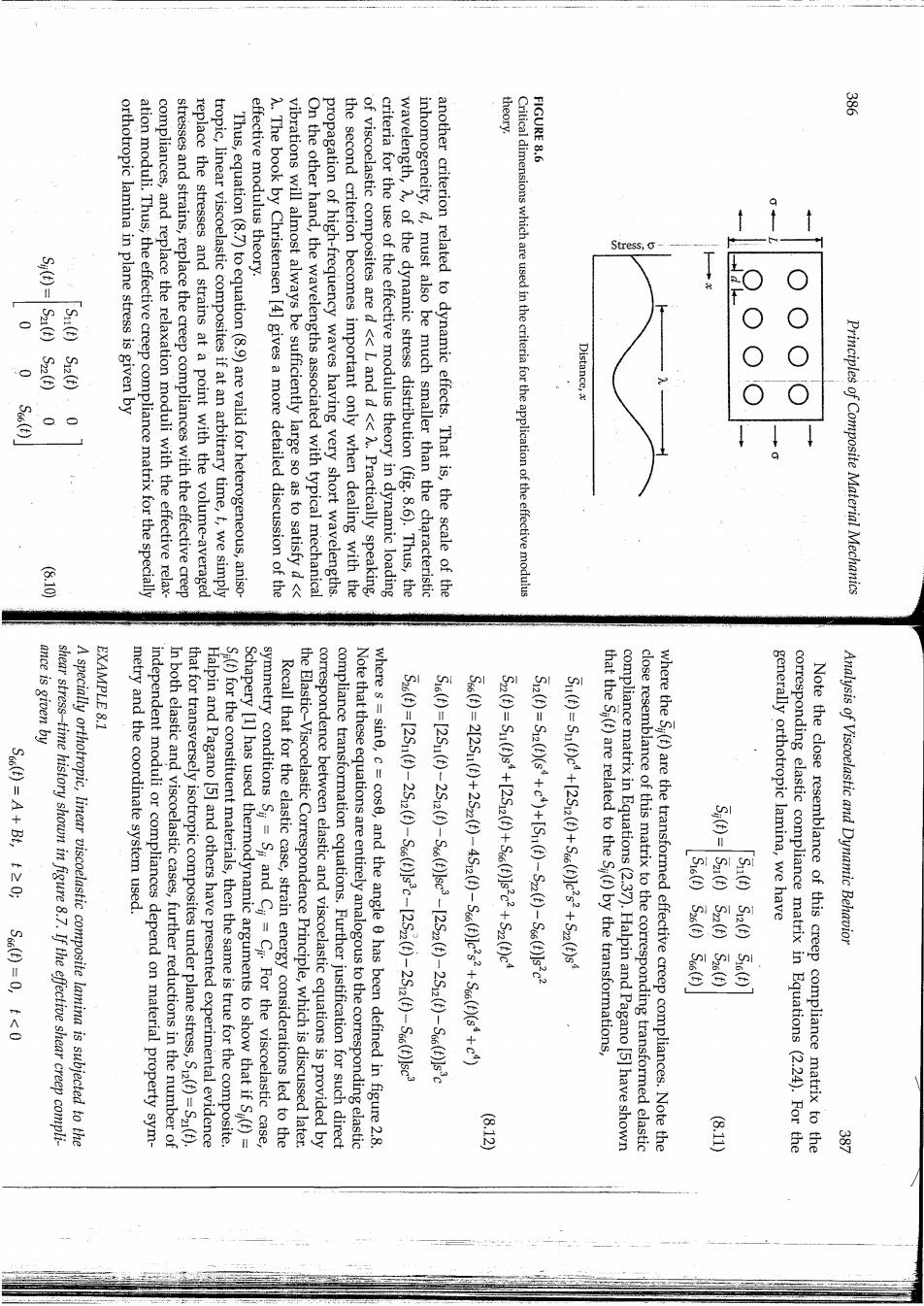
theory. FIGURE 8.6 Su(E)S12(f) orthotropic lamina in plane stress is given by S22(t) ation moduli.Thus,the effective creep compliance matrix for the specially compliances,and replace the relaxation moduli with the effective relax- stresses and strains,replace the creep compliances with the effective creep replace the stresses and strains at a point with the volume-averaged tropic,linear viscoelastic composites if at an arbitrary time,t,we simply Thus,equation(8.7)to equation(8.9)are valid for heterogeneous,aniso- effective modulus theory. A.The book by Christensen [4]gives a more detailed discussion of the vibrations will almost always be sufficiently large so as to satisfy d<< On the other hand,the wavelengths associated with typical mechanical propagation of high-frequency waves having very short wavelengths the second criterion becomes important only when dealing with the of viscoelastic composites are d <L and d<<A.Practically speaking, criteria for the use of the effective modulus theory in dynamic loading wavelength,A,of the dynamic stress distribution (fig.8.6).Thus,the inhomogeneity,d,must also be much smaller than the characteristic another criterion related to dynamic effects.That is,the scale of the Critical dimensions which are used in the criteria for the application of the effective modulus Stress, Distance, Principles of Composite Material Mechanics 610 ance is given by EXAMPLE 8.1 shear stress-time history shown in figure 8.7.If the effective shear creep compli- A specially orthotropic,linear viscoelastic composite lamina is subjected to the metry and the coordinate system used. independent moduli or compliances depend on material property sym- In both elastic and viscoelastic cases,further reductions in the number of that for transversely isotropic composites under plane stress,S2(f)=S2(t) Halpin and Pagano [5]and others have presented experimental evidence S()for the constituent materials,then the same is true for the composite. Schapery [1]has used thermodynamic arguments to show that if Si(f)= symmetry conditions S=Si and Cy=Ci For the viscoelastic case, Recall that for the elastic case,strain energy considerations led to the the Elastic-Viscoelastic Correspondence Principle,which is discussed later. correspondence between elastic and viscoelastic equations is provided by compliance transformation equations.Further justification for such direct Note that these equations are entirely analogous to the corresponding elastic where s=sine,c=cos6,and the angle 0 has been defined in figure 2.8. Sag-251g-255g-56ga-c-25:0-25:0-5%060 S0g-251g-255g-5%geeu-25:电-255g-s6cee l452g-B%c8e:s:+S%dS0-2125104+25:-Ss6 Sz(t)=Su()s4+[2S2()+So()s2c2+Sz()c Sr2()=Siz()(s+c)+[Su()-Sz()-S66(t)]s2c2 Su(t)=Su(t)c4+[2S2()+Sco()s2+Sz()s that the S;(t)are related to the Si(f)by the transformations, close resemblance of this matrix to the corresponding transformed elastic compliance matrix in Equations(2.37).Halpin and Pagano [5]have shown where the S(t)are the transformed effective creep compliances.Note the generally orthotropic lamina,we have S16(t)S26(t)S66(t) S22(t)S26(t) Su(t)S12(t)Sio(t) corresponding elastic compliance matrix in Equations (2.24).For the Note the close resemblance of this creep compliance matrix to the Analysis of Viscoelastic and Dynamic Behavior 8.12 s10