罗 56. 55. 52. London. New York. tures.Composites Science and Technology,56(9),1001-1015. Huybrechts,S.and Tsai,S.W.1996.Analysis and behavior of grid struc- grid structures.Journal of Composite Materials,30(4),503-534. Chen,H.J.and Tsai,S.W.1996.Analysis and optimum design of composite ymposium,CD-ROM. stiffened geodesic composite structures.Proc.44 International SAMPE Rehfield,L.W.1999.A brief history of analysis methodology for grid- Journal of Mechanical Sciences,46,561-583. ing.Part I:analytical models and minimum weight design.International beams with composite faces and a foam core,loaded in three-point bend- Steeves,C.A.and Fleck,N.A.2004.Collapse mechanisms of sandwich Zenkert,D.1995.An Introduction to Sandwich Construction.Chameleon, tional Journal of Mechanical Sciences,46,585-608. ing.Part II:Experimental investigation and numerical modeling.Interna- beams with composite faces and a foam core,loaded in three-point bend- Steeves,C.A.and Fleck,N.A.2004.Collapse mechanisms of sandwich 兵!:M Vinson,J.R.1999.The Behavior of Sandwich Structures of Isotropic and Composite Sun,C.T.1998.Mechanics of Aircraft Structures.John Wiley Sons,Inc. Principles of Composite Material Mechanics such a way that the current strains depend more strongly on the recent Stress-fime history thannth enough. Behavior is often referred to as"fading memory"because they remember the past in strains depend only on the current stresses.Viscoelastic materials have what memory"because they remember only the unstrained state and the current materials is memory.Perfectly elastic solids are said to have only "simple energy storage.Viscoelastic materials,however,are capable of both storage and dissipation of energy under load.Another characteristic of viscoelastic under load,but not energy dissipation,whereas ideal Newtonian fluids under nonhydrostatic stresses are capable of energy dissipation,but not We know that ideal Hookean elastic solids are capable of energy storage glass transition temperature,TAt temperatures below T however,it will deform just as much,and in the same way if the test time is long temperatures.It will deform like a rubber at temperatures just above the ing at processing temperatures,but is a glassy solid at service(ambient) fluids or solids,depending on the time scale or the temperature.For example,polycarbonate,a thermoplastic polymer,is a liquid during mold- that exhibit characteristics of both viscous fluids and elastic solids.Poly- meric materials,which are known to be viscoelastic,may behave like The word "viscoelastic"has evolved as a way of describing materials needed for the analysis of both viscoelastic and dynamic behavior of com- posites and their constituents. having polymeric constituents.This chapter contains the basic information wave propagation.In addition,many composites exhibit time-dependent viscoelastic behavior under load;this is particularly true for composites ents exhibit time-independent linear elastic behavior.However,composite structures are often subjected to dynamic loading caused by vibration or In the analyses of chapter 1 to chapter 7,it has been assumed that the applied loads are static in nature and that the composite and its constitu- Introduction Analysis of Viscoelastic and Dynamic
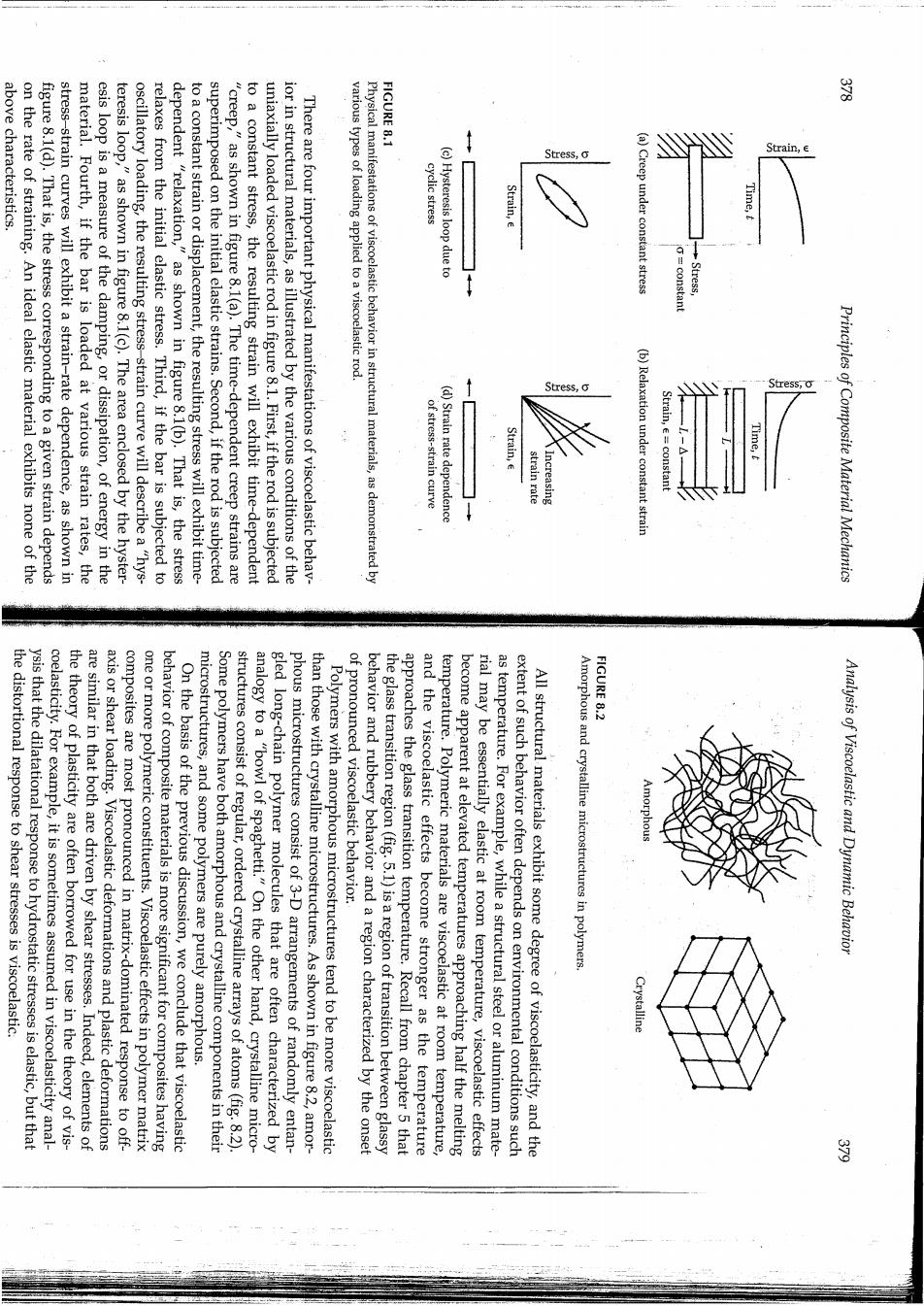
above characteristics ·1 FI Stress,o Strain,e on the rate of straining.An ideal elastic material exhibits none of the figure 8.1(d).That is,the stress corresponding to a given strain depends stress-strain curves will exhibit a strain-rate dependence,as shown in material.Fourth,if the bar is loaded at various strain rates,the esis loop is a measure of the damping,or dissipation,of energy in the teresis loop,"as shown in figure 8.1(c).The area enclosed by the hyster- oscillatory loading,the resulting stress-strain curve will describe a "hys- relaxes from the initial elastic stress.Third,if the bar is subjected to dependent "relaxation, to a constant strain or displacement,the resulting stress will exhibit time- superimposed on the initial elastic strains.Second,if the rod is subjected "creep,"as shown in figure 8.1(a).The time-dependent creep strains are to a constant stress,the resulting strain will exhibit time-dependent uniaxially loaded viscoelastic rod in figure 8.1.First,if the rod is subjected ior in structural materials,as illustrated by the various conditions of the There are four important physical manifestations of viscoelastic behav- various types of loading applied to a viscoelastic rod. Physical manifestations of viscoelastic behavior in structural materials,as demonstrated by cyclic stress (c)Hysteresis loop due to Strain,e (a)Creep under constant stress Time,t constan "as shown in figure 8.1(b).That is,the stress Stress ot stress-strain curve (d)Strain rate dependence Strain,E strain rate Increasing (b)Relaxation under constant strain St女ess Strain,e=constant Time, Principles of Composite Material Mechanics FIGURE 8.2 the distortional response to shear stresses is viscoelastic. ysis that the dilatational response to hydrostatic stresses is elastic,but that coelasticity.For example,it is sometimes assumed in viscoelasticity anal- the theory of plasticity are often borrowed for use in the theory of vis- are similar in that both are driven by shear stresses.Indeed,elements of axis or shear loading.Viscoelastic deformations and plastic deformations composites are most pronounced in matrix-dominated response to off- one or more polymeric constituents.Viscoelastic effects in polymer matrix behavior of composite materials is more significant for composites having On the basis of the previous discussion,we conclude that viscoelastic microstructures,and some polymers are purely amorphous. Some polymers have both amorphous and crystalline components in their structures consist of regular,ordered crystalline arrays of atoms(fig.8.2) gled long-chain polymer molecules that are often characterized by analogy to a"bowl of spaghetti."On the other hand,crystalline micro- phous microstructures consist of 3-D arrangements of randomly entan- than those with crystalline microstructures.As shown in figure 8.2,amor- Polymers with amorphous microstructures tend to be more viscoelastic behavior and rubbery behavior and a region characterized by the onset of pronounced viscoelastic behavior. the glass transition region(fig.5.1)is a region of transition between glassy approaches the glass transition temperature.Recall from chapter 5 that and the viscoelastic effects become stronger as the temperature temperature.Polymeric materials are viscoelastic at room temperature, become apparent at elevated temperatures approaching half the melting rial may be essentially elastic at room temperature,viscoelastic effects as temperature.For example,while a structural steel or aluminum mate- extent of such behavior often depends on environmental conditions such All structural materials exhibit some degree of viscoelasticity,and the Amorphous and crystalline microstructures Analysis of Viscoelastic and Dynamic Behavior in polymers Crystalline

多 neous composites. of homogeneous materials to the corresponding analyses of heteroge- be used to extend various viscoelastic analyses and dynamic analyses tions,the concept of an effective modulus or effective compliance will coelastic and dynamic analyses of composites.Under certain restric- introduced in chapter 2 and chapter 3,is indispensable in both vis- Finally,it will be shown that the effective modulus theory,which was damping behavior of composites. ularly useful in the development of analytical models for predicting the coelastic Correspondence Principle from viscoelasticity theory are partic- It will be shown that the complex modulus notation and the Elastic-Vis- als,especially if the composite has one or more polymeric constituents erally have better damping than conventional metallic structural materi- is obviously important for noise and vibration control.Composites gen- Damping,which is one of the manifestations of viscoelastic behavior or laminates without coupling will be considered. for vibrations and wave propagation in specially orthotropic composites or some other complex mode.In this chapter,however,only the analyses symmetric laminate may respond in a coupled bending-twisting mode ing moment will respond in pure flexural modes of vibration,but a non- vibration.For example,an isotropic beam subjected to an oscillatory bend- Anisotropic coupling effects often lead to complex waves or modes of in an anisotropic composite depends on the direction of propagation isotropic material is independent of orientation,whereas the wave speed anisotropic behavior.For example,the speed of a propagating wave in an The dynamic response of composites may also be complicated by their interfaces,complicating matters further. plex reflection and/or refraction effects at fiber/matrix interfaces or ply from the boundaries.Wave propagation in composites may involve com- waves (i.e.,vibrations)will be set up as the waves begin to reflect back shorter than the characteristic structural dimension,otherwise standing Wave propagation will continue if the response wavelength is much sion.Both types of exeitation usually cause wave propagation initially. the wavelength of the response and the characteristic structural dimen- vibration,depending on the elapsed time and the relative magnitudes of oscillatory.Dynamic response consists of either a propagating wave or a Dynamic loading is usually categorized as being either impulsive or so-called Elastic-Viscoelastic Correspondence Principle. analogous to Hookean elastic stress-strain relationships,leading to the gral transforms,the viscoelastic stress-strain relationships turn out to be relaxation,and sinusoidal oscillation.Following the use of certain inte- posites.These stress-strain relationships take on special forms for creep stress-strain relationships for linear viscoelastic materials and their com- In this chapter we will be concerned with the development of Principles of Composite Material Mechanics stress o is 8.2 typically E(t)decreases with time. section. The creep compliance for a constant stress-strain curve is the time-dependent Young's modulus,E(f),and figure 8.3(a),we can plot the so-called isochronous stress-strain curve at time t =ti,as shown in figure 8.3(b).The slope of the isochronous plot stress-strain curves at different times.For example,by taking the ratio of stress to strain at each stress level corresponding to time fi in curves.If a number of creep curves are generated at different stress levels as shown schematically in figure 8.3(a),these creep curves can be used to time-dependent strain.The strain versus time curves are known as creep constant tensile stress to a specimen and measurement of the resulting creep test described in figure 8.1(a),which involves the application of a approach to verification of linear viscoelastic behavior.Probably the most widely used method of characterizing viscoelastic behavior is the tensile ior,however,it is instructive to briefly discuss a phenomenological Before getting into the analytical modeling of linear viscoelastic behav- the Boltzmann superposition integral,which is developed in the next These criteria form the basis of the stress-strain relationship known as Any response not satisfying these criteria would be a nonlinear response. Superposition:i.e.,R(+=R(Ia+R(),where I and I may be the same or different time histories 1.Proportionality:i.e.,R(cl)=cR(n),where c is a constant following conditions: For linear viscoelastic behavior,the response R(n must satisfy both the stated as follows: that the current value of R is a function of the time history of the input I. Let the response R to an input Ibe written as R=R(I),where R(I)denotes any system.Following the notation of Schapery [1],the criteria can be ship depends on the time history of the input.The mathematical criteria for linear viscoelastic behavior are similar to those for linear behavior of also exhibits a linearity between stress and strain,but the linear relation- this linear relationship is independent of time.A linear viscoelastic solid A linear elastic solid exhibits a linearity between stress and strain,and Linear Viscoelastic Behavior of Composites Analysis of Viscoelastic and Dynamic Behavior 空 岁

curves. FIGURE 8.3 学 学 学 e(tj)4 3 Strain,e(t) linear viscoelastic behavior.For example,moving So to the left-hand side scale becomes a straight line,and this provides another way to check for scales to plot creep compliance data.A power law plotted on a log-log erally conducted over several decades,it is often convenient to use log-log posite and the polymer matrix material.Since creep experiments are gen- only on the polymer matrix,and indeed that n is the same for the com- [2]that,for polymer matrix composites,the creep exponent n depends determined parameters.It has been shown experimentally by Beckwith where So is the initial elastic compliance and Si and n are empirically power law expression of the form as those shown in figure 8.3(a)can be described mathematically using a Typically,the creep compliance for linear viscoelastic creep curves such rials being discussed are linear viscoelastic. viscoelastic.In this book,it is always assumed that the viscoelastic mate- become nonlinear,and this means that the material becomes nonlinear stress level becomes high enough,the isochronous stress-strain curve will continue to behave in a linear viscoelastic manner.For example,if the limits on the ranges of stress and time within which a material will which its isochronous stress-strain curves are linear.There are always material is linear viscoelastic within the range of stresses and times for and obviously S(t)increases with time.Phenomenologically speaking,a Illustration of creep curves at constant stress and corresponding isochronous stress-strain (a)Creep curves at constant stress Time,t S(t)=So+Sut" 9 at time t=t1 (b)Isochronous stress-strain curve Strain at time Principles of Composite Material Mechanics 621re8.4. yields FIGURE 8.4 e8-A6181-7)4268.51-727426884-7g history in figure 8.5,the total strain response at any time t>ta is given by time since the application of the input stress.Thus,for the stress-time to the input stress,but the proportionality factor is a function of the elapsed mann Superposition Principle,the strain response is linearly proportional and ta respectively,as shown in figure 8.5.According to the Boltz- neous linear viscoelastic material by the stresses Ao,Aoz,and Aos at times Consider the 1-D isothermal loading of a nonaging,isotropic,homoge- temperature and aging effects will be considered in section 8.2.6. material,which is different from viscoelastic creep or relaxation.Both the elapsed time (t-t)only.Aging is a time-dependent change in the at any time t due to an input at time f=t is a function of the input and material is at a constant temperature and is "nonaging,"then the response developed by using the Boltzmann Superposition Principle [3].If the The stress-strain relationships for a linear viscoelastic maternal can be 8.2.1 Boltzmann Superposition Integrals for Creep and Relaxation versus logf with slope n and vertical axis intercept logS,as shown in which is the equation for a straight line on a log-log plot of logIS(t)-Sol of equation(8.2)and taking the log of both sides of the resulting equation Illustration of log creep compliance vs log time plot Analysis of Viscoelastic and Dynamic Behavior 思 器
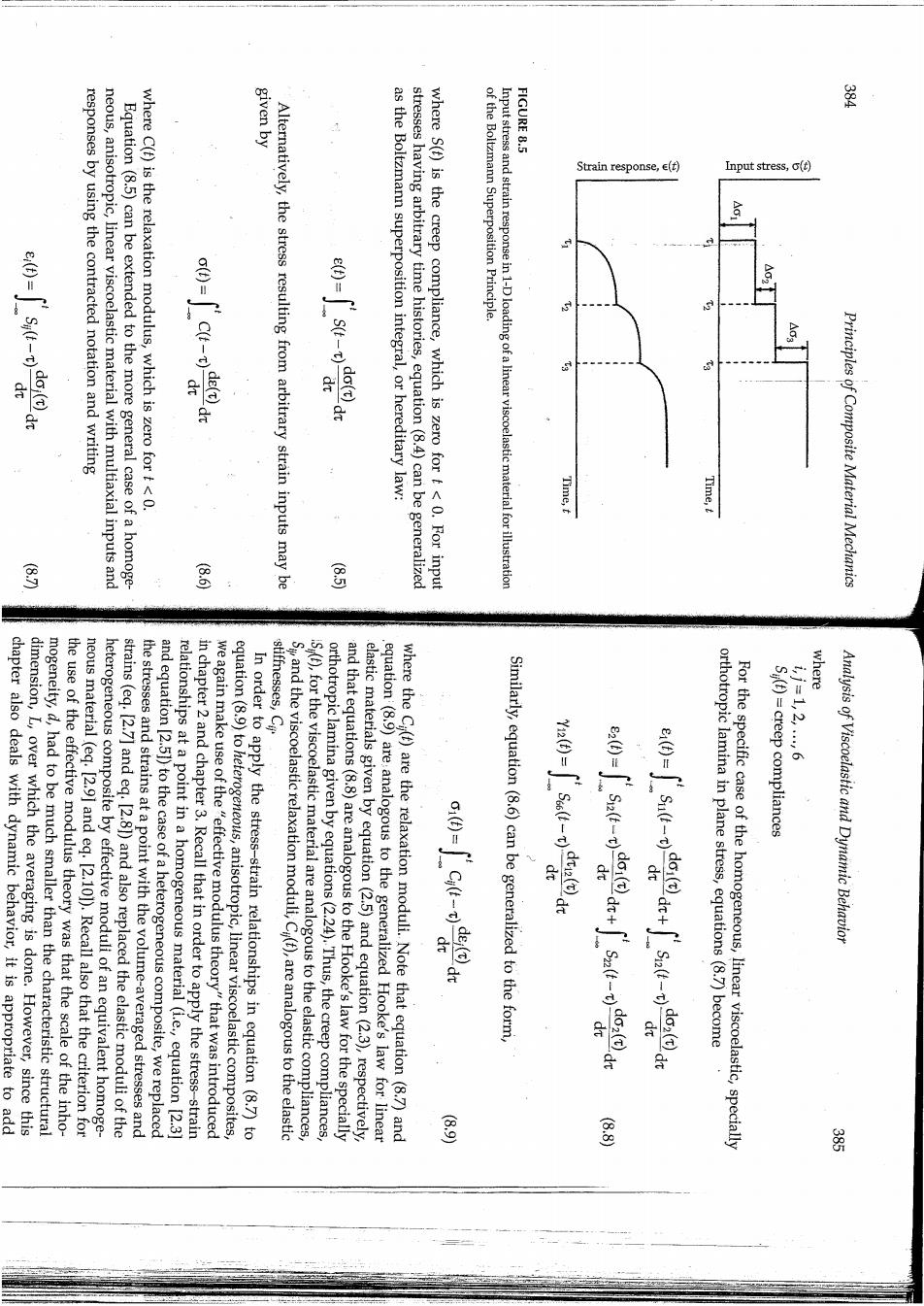
given by FIGURE 8.5 responses by using the contracted notation and writing Strain response,E(t Input stress,o(t) neous,anisotropic,linear viscoelastic material with multiaxial inputs and Equation(8.5)can be extended to the more general case of a homoge- where C(t)is the relaxation modulus,which is zero for t<0. Alternatively,the stress resulting from arbitrary strain inputs may be of the Boltzmann Superposition Principle. do(dr as the Boltzmann superposition integral,or hereditary law: stresses having arbitrary time histories,equation(8.4)can be generalized where S(t)is the creep compliance,which is zero for t<0.For input Input stress and strain response in1-Dloading of a linear viscoelastic material for illustration Time,t Time, Principles of Composite Material Mechanics 丑 stiffnesses,C where chapter also deals with dynamic behavior,it is appropriate to add dimension,L,over which the averaging is done.However,since this mogeneity,d,had to be much smaller than the characteristic structural the use of the effective modulus theory was that the scale of the inho- neous material (eq.[2.9]and eq.[2.101).Recall also that the criterion for heterogeneous composite by effective moduli of an equivalent homoge- the stresses and strains at a point with the volume-averaged stresses and strains (eq.[2.7]and eq.[2.81)and also replaced the elastic moduli of the relationships at a point in a homogeneous material (i.e.,equation [2.3] and equation [2.5])to the case of a heterogeneous composite,we replaced in chapter 2 and chapter 3.Recall that in order to apply the stress-strain equation(8.9)to heterogeneous,anisotropic,linear viscoelastic composites, we again make use of the "effective modulus theory"that was introduced In order to apply the stress-strain relationships in equation(8.7)to Sand the viscoelastic relaxation moduli,C(),are analogous to the elastic S),for the viscoelastic material are analogous to the elastic compliances, and that equations(8.8)are analogous to the Hooke's law for the specially orthotropic lamina given by equations(2.24).Thus,the creep compliances, equation(8.9)are:analogous to the generalized Hooke's law for linear elastic materials given by equation(2.5)and equation(2.3),respectively, where the C(t)are the relaxation moduli.Note that equation(8.7)and Similarly,equation(8.6)can be generalized to the form, orthotropic lamina in plane stress,equations(8.7)become S(t)=creep compliances 17512.r6 For the specific case of the homogeneous,linear viscoelastic,specially Analysis of Viscoelastic and Dynamic Behavior 思 图
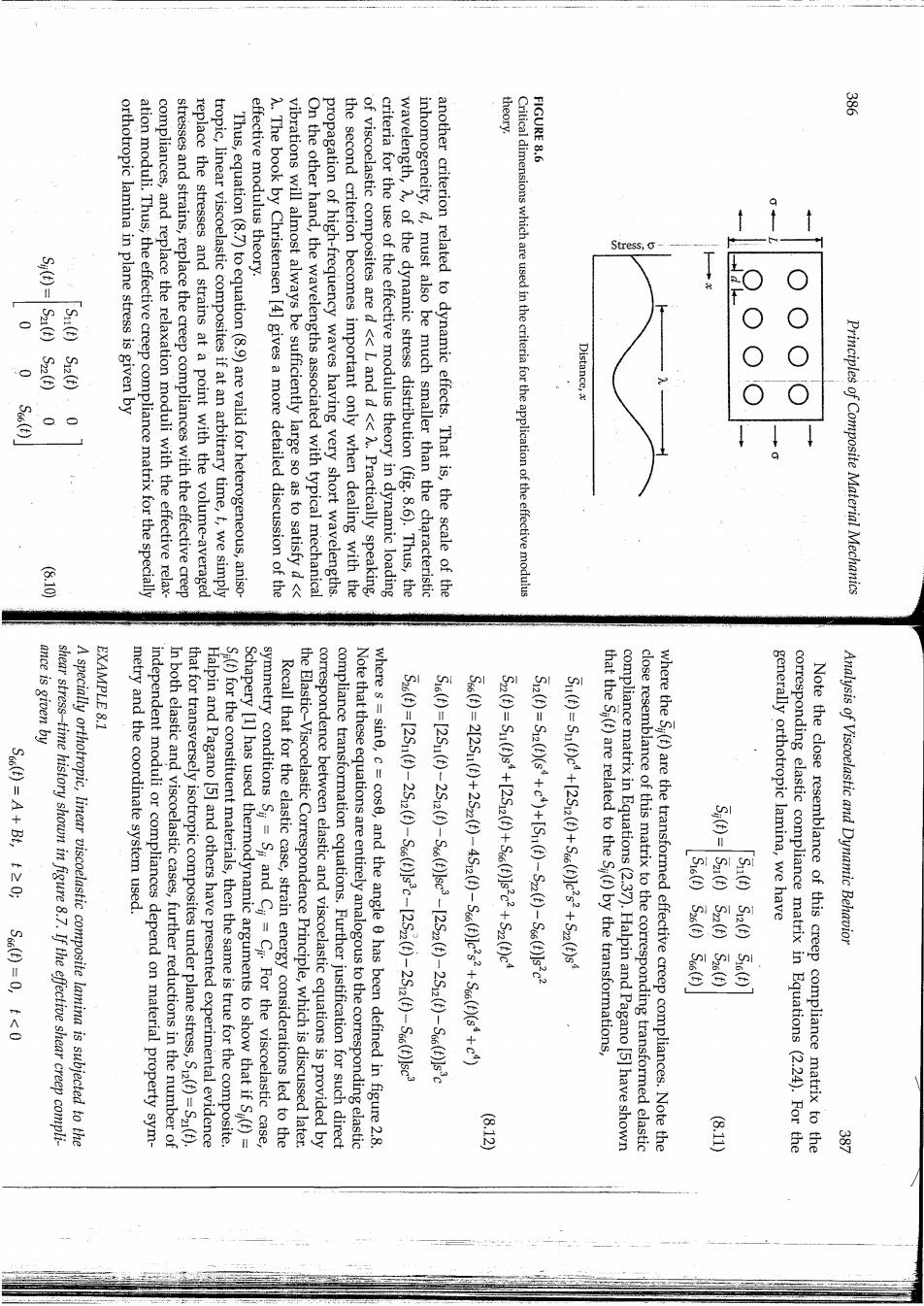
theory. FIGURE 8.6 Su(E)S12(f) orthotropic lamina in plane stress is given by S22(t) ation moduli.Thus,the effective creep compliance matrix for the specially compliances,and replace the relaxation moduli with the effective relax- stresses and strains,replace the creep compliances with the effective creep replace the stresses and strains at a point with the volume-averaged tropic,linear viscoelastic composites if at an arbitrary time,t,we simply Thus,equation(8.7)to equation(8.9)are valid for heterogeneous,aniso- effective modulus theory. A.The book by Christensen [4]gives a more detailed discussion of the vibrations will almost always be sufficiently large so as to satisfy d<< On the other hand,the wavelengths associated with typical mechanical propagation of high-frequency waves having very short wavelengths the second criterion becomes important only when dealing with the of viscoelastic composites are d <L and d<<A.Practically speaking, criteria for the use of the effective modulus theory in dynamic loading wavelength,A,of the dynamic stress distribution (fig.8.6).Thus,the inhomogeneity,d,must also be much smaller than the characteristic another criterion related to dynamic effects.That is,the scale of the Critical dimensions which are used in the criteria for the application of the effective modulus Stress, Distance, Principles of Composite Material Mechanics 610 ance is given by EXAMPLE 8.1 shear stress-time history shown in figure 8.7.If the effective shear creep compli- A specially orthotropic,linear viscoelastic composite lamina is subjected to the metry and the coordinate system used. independent moduli or compliances depend on material property sym- In both elastic and viscoelastic cases,further reductions in the number of that for transversely isotropic composites under plane stress,S2(f)=S2(t) Halpin and Pagano [5]and others have presented experimental evidence S()for the constituent materials,then the same is true for the composite. Schapery [1]has used thermodynamic arguments to show that if Si(f)= symmetry conditions S=Si and Cy=Ci For the viscoelastic case, Recall that for the elastic case,strain energy considerations led to the the Elastic-Viscoelastic Correspondence Principle,which is discussed later. correspondence between elastic and viscoelastic equations is provided by compliance transformation equations.Further justification for such direct Note that these equations are entirely analogous to the corresponding elastic where s=sine,c=cos6,and the angle 0 has been defined in figure 2.8. Sag-251g-255g-56ga-c-25:0-25:0-5%060 S0g-251g-255g-5%geeu-25:电-255g-s6cee l452g-B%c8e:s:+S%dS0-2125104+25:-Ss6 Sz(t)=Su()s4+[2S2()+So()s2c2+Sz()c Sr2()=Siz()(s+c)+[Su()-Sz()-S66(t)]s2c2 Su(t)=Su(t)c4+[2S2()+Sco()s2+Sz()s that the S;(t)are related to the Si(f)by the transformations, close resemblance of this matrix to the corresponding transformed elastic compliance matrix in Equations(2.37).Halpin and Pagano [5]have shown where the S(t)are the transformed effective creep compliances.Note the generally orthotropic lamina,we have S16(t)S26(t)S66(t) S22(t)S26(t) Su(t)S12(t)Sio(t) corresponding elastic compliance matrix in Equations (2.24).For the Note the close resemblance of this creep compliance matrix to the Analysis of Viscoelastic and Dynamic Behavior 8.12 s10

FIGURE 8.7 第 The Laplace transform,f(t)]or f(s),of a function f(t)is defined by preted by using differential equations. and strain.Physical models for viscoelastic behavior can be easily inter to an ordinary differential equation involving time derivatives of stress transforms will be used to convert the Boltzmann superposition integral us to understand viscoelastic behavior better.In this section,Laplace rial,it does not lend itself easily to the use of physical models that help expression of the stress-strain relationship for a linear viscoelastic mate- Although the Boltzmann superposition integral is a valid mathematical 8.2.2 Differential Equations and Spring-Dashpot Models For t>To,we have which,for the case of To where A and B are material constants and t is time.Find the expressions for the Shear stress time history for example 8.1. Shear stress,() Time,t Principles of Composite Material Mechanics (8.17)becomes If we perform similar operations on equation(8.5),we find that where e(0)is the initial strain.If we neglect the initial conditions,equation But from the properties of Laplace transforms of derivatives [61, Thus,equation (8.14)can be written as Taking the inverse Laplace transform of equation(8.15),we find that convolution integral [61,we can also write tion is given by' Noting that the right-hand side of equation(8.14)is in the form of a given by equation(8.6).The Laplace transform of both sides of the equa- take the Laplace transform of the 1-D Boltzmann superposition integral where s is the Laplace parameter.For purposes of illustration,we now Analysis of Viscoelastic and Dynamic Behavior 8.20 (810 (6·18) 81M (816) (615 骂

tionship is two polynomials in the Laplace parameter s as follows: must be related by The coefficient term in equation(8.20)can also be written as a ratio of when t>0 and for long times when to,the mathematically exact rela- Value Theorem of Laplace transforms(see Problem 8.2)that for short times and it can be shown by using the Initial Value Theorem and the Final However,a usually good approximation is ically related by a simple inverse relationship.That is,in general, However,the corresponding time domain properties are not mathemat- 5(6C() creep compliance and the Laplace transform of the relaxation modulus ing to equation(8.19)and equation(8.20),the Laplace transform of the spondence Principle,which will be discussed later.Note also that accord- materials and is another building block in the Elastic-Viscoelastic Corre- the correspondence between the equations for elastic and viscoelastic Laplace transform of the relaxation modulus.This is another example of ality constants are the Laplace transform of the creep compliance and the forms of the stresses and strains are linearly related,and the proportion- as Hooke's law for linear elastic materials,except that the Laplace trans- Note that equation(8.19)and equation(8.20)are now of the same form Principles of Composite Material Mechanics (8.2) 图 (622) ment is of the form g=o/k,whereas the corresponding equation for the viscous dashpot is de/dt=o/u. where viscosity #Thus,the stress-strain relationship for the elastic spring ele- law and the dashpot is assumed to be filled with a Newtonian fluid of cous dashpot,where the spring of modulus k is assumed to follow Hooke's constructed from simple elements such as the elastic spring and the vis- As shown in figure 8.8 to figure 8.10,useful physical models can be derivatives of stress and strain. physical models of linear viscoelastic behavior that include various time behavior is the dependence of stress on strain rate;such strain rate effects can be modeled with equation(8.28).We now consider several simple (ie.,aoe=boo).Recall that one of the physical manifestations of viscoelastic case of equation(8.28)when all time derivatives of stress and strain vanish Note that the linear elastic material described by Hooke's law is a special differential equation as well as by the Boltzmann superposition integral. Thus,linear viscoelastic behavior may also be described by an ordinary 但w+3P of equation(8.26),we find that 被0个 d2o Making use of equation(8.27)and taking the inverse Laplace transform derivative of a function f()is But if we neglect the initial conditions,the Laplace transform of the nth Thus,we can write P(s)=ao+as+azs2+...+ans" Analysis of Viscoelastic and Dynamic Behavior (828 图 826
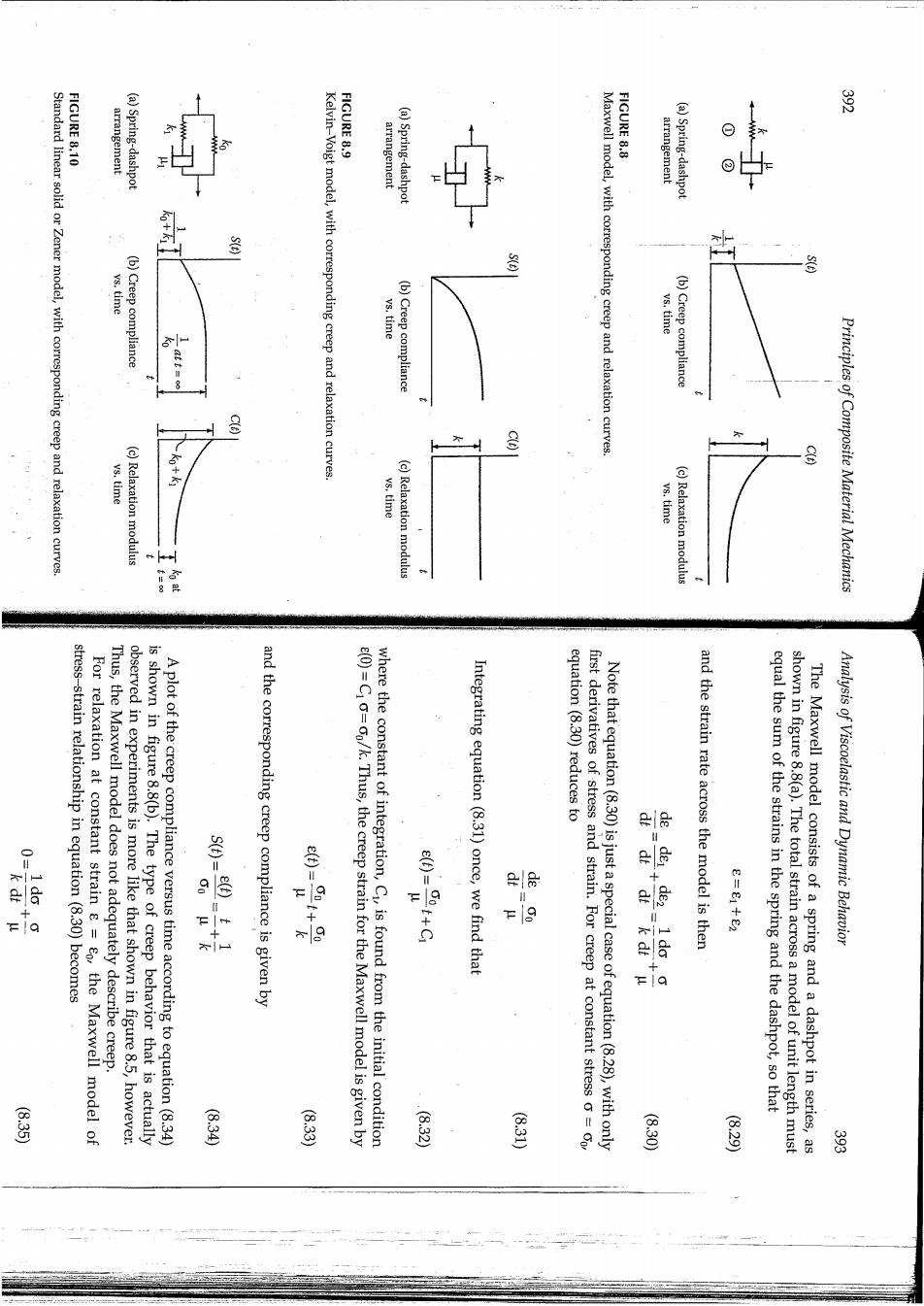
FIGURE 8.10 常 arrangement (a)Spring-dashpot FIGURE 8.9 Kelvin-Voigt model,with corresponding creep and relaxation curves arrangement (a)Spring-dashpot FIGURE 8.8 Standard linear solid or Zener model,with corresponding creep and relaxation curves. vs.time (b)Creep compliance ys.time (b)Creep compliance Maxwell model,with corresponding creep and relaxation curves. arrangement (a)Spring-dashpot vs.time (b)Creep compliance ys.time (c)Relaxation modulus + 8 ys,time (c)Relaxation modulus vs.time (c)Relaxation modulus Principles of Composite Material Mechanics 入 stress-strain relationship in equation(8.30)becomes For relaxation at constant strain g =8o,the Maxwell model of Thus,the Maxwell model does not adequately describe creep. observed in experiments is more like that shown in figure 8.5,however. is shown in figure 8.8(b).The type of creep behavior that is actually A plot of the creep compliance versus time according to equation(8.34) and the corresponding creep compliance is given by e(0)=Cio=0o/k.Thus,the creep strain for the Maxwell model is given by where the constant of integration,C1,is found from the initial condition O-8+O Integrating equation(8.31)once,we find that first derivatives of stress and strain.For creep at constant stress o=oo equation(8.30)reduces to Note that equation(8.30)is just a special case of equation(8.28),with only and the strain rate across the model is then 8-e+e2 Analysis of Viscoelastic and Dynamic Behavior 18 equal the sum of the strains in the spring and the dashpot,so that shown in figure 8.8(a).The total strain across a model of unit length must The Maxwell model consists of a spring and a dashpot in series,as 835 9.33 (8.32 (8.30 (639 常

relaxation modulus is given by where p =u/k is now referred to as the retardation time.Similarly,the pliance for the Kelvin-Voigt model is given by only first derivatives of strain.It can also be shown that the creep com- Equation(8.39)is seen to be another special case of equation(8.28),with the Kelvin-Voigt model is given by it can be shown that the differential equation describing the behavior of arrangement and following a procedure similar to the one just outlined, and a dashpot in parallel.Using the appropriate equations for a parallel Figure 8.9(a)shows the Kelvin-Voigt model,which consists of a spring the relaxation phenomenon,but not the creep response. experimentally.Thus,the Maxwell model appears to describe adequately (8.38),which is in general agreement with the type of relaxation observed Figure 8.8(c)shows the relaxation modulus versus time from equation relaxation modulus is C(t)= measure of the internal time scale of the material.The corresponding relax to 1/e,or 37%of its initial value.The relaxation time is therefore a where A=u/k is the relaxation time,or the time required for the stress to (t)=Goe-k/a =Goet/ o(0)=0o.The resulting stress relaxation function is where the constant of integration,C2,is found from the initial condition Integrating equation (8.35)once,we find Principles of Composite Material Mechanics 墨 640) 8.39 3.37 (8.36 Practically speaking,complete relaxation for the Zener model occurs in less the relaxation modulus versus the logarithm of time,as shown in figure 8.11 pletely adequate.This remaining inadequacy is best described by plotting and relaxation behavior in linear viscoelastic materials,it still is not com- correctly describes all expected features of experimentally observed creep mentally observed. Although the Zener model is the simplest spring-dashpot model that the general shape of the curve appears to be similar to what is experi- the predicted relaxation modulus curve from equation(8.44),and,again time for the Maxwell model consisting of and k.Figure 8.10(c)shows where =/k is the relaxation time.Note that is just the relaxation observations.The relaxation modulus for the Zener model is given by from equation(8.43)matches the expected shape based on experimental As shown in figure 8.10(b),the shape of the creep compliance curve where p=(/kok)(ko+k)is the retardation time. compliance for the Zener model is given by figure 8.10(a)is just a Maxwell model in parallel with a spring.The creep (8.28).It is also interesting to note that the Zener model shown in (8.42)is obviously another special case of the general differential equation where the parameters koki,and are defined in figure 8.10(a).Equation differential equation for the Zener model is given by to as the standard linear solid or Zener model.It can be shown that the elements.One such improved model,shown in figure 8.10(a),is referred One obvious way to improve the spring-dashpot model is to add more mentally observed creep and relaxation. Kelvin-Voigt model does not adequately describe all features of experi- constant,as shown in figure 8.9(c).Thus,like the Maxwell model,the the other hand,the relaxation modulus has not been observed to be imental observation,except that the initial elastic response is missing.On figure 8.9(c),respectively.The creep compliance curve agrees with exper- Equation (8.40)and equation (8.41)are plotted in figure 8.9(b)and Analysis of Viscoelastic and Dynamic Behavior 墨 .3) 3) 芳