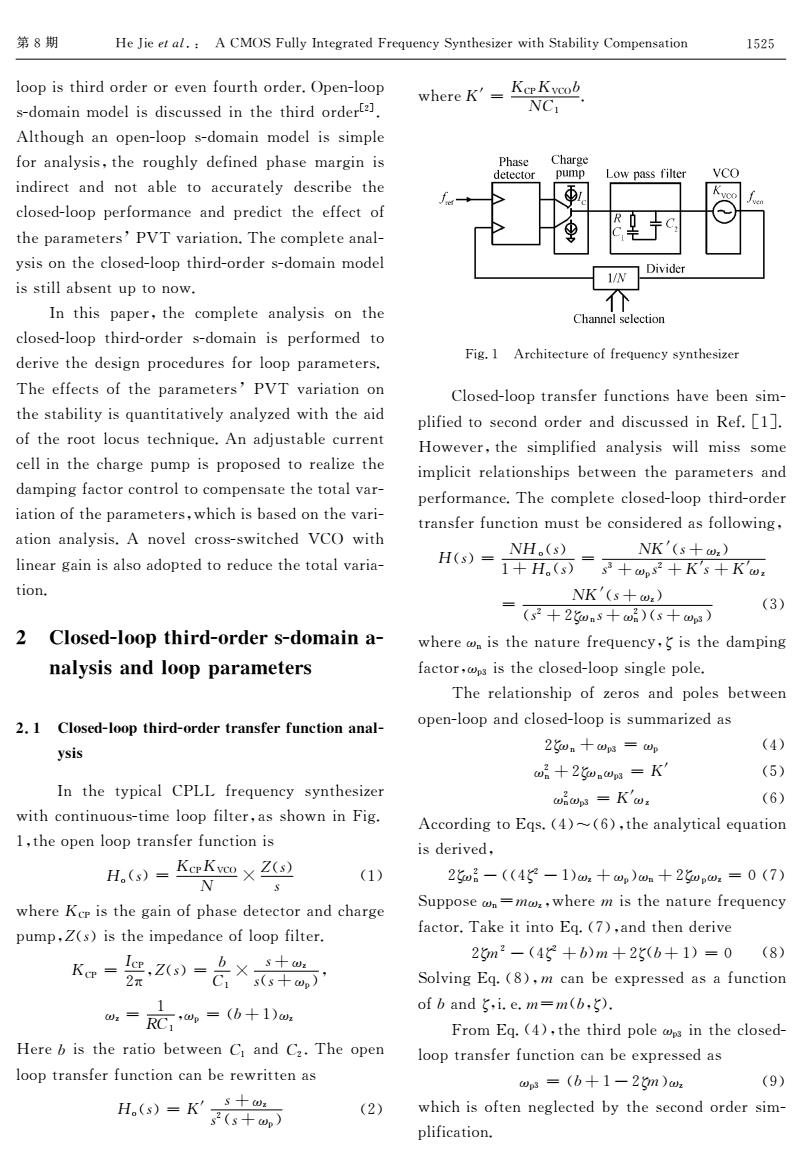
第8期 He Jie et al.A CMOS Fully Integrated Frequency Synthesizer with Stability Compensation 1525 loop is third order or even fourth order.Open-loop where K'=Kcr Kvcob s-domain model is discussed in the third orderta. NC Although an open-loop s-domain model is simple for analysis,the roughly defined phase margin is Phase Charge detector pump Low pass filter VCO indirect and not able to accurately describe the closed-loop performance and predict the effect of the parameters'PVT variation.The complete anal- ysis on the closed-loop third-order s-domain model Divider 1/N is still absent up to now. In this paper,the complete analysis on the Channel selection closed-loop third-order s-domain is performed to derive the design procedures for loop parameters. Fig.I Architecture of frequency synthesizer The effects of the parameters'PVT variation on Closed-loop transfer functions have been sim- the stability is quantitatively analyzed with the aid plified to second order and discussed in Ref.[1]. of the root locus technique.An adjustable current However,the simplified analysis will miss some cell in the charge pump is proposed to realize the implicit relationships between the parameters and damping factor control to compensate the total var- performance.The complete closed-loop third-order iation of the parameters,which is based on the vari- transfer function must be considered as following, ation analysis.A novel cross-switched VCO with NH(s) NK'(s+@z) linear gain is also adopted to reduce the total varia- H()=1+H.=+,F+K+K@, tion. NK'(s+@z) =(+20as+2)(s+mm） (3) Closed-loop third-order s-domain a- where is the nature frequency,is the damping nalysis and loop parameters factor,@ps is the closed-loop single pole. The relationship of zeros and poles between 2.1 Closed-loop third-order transfer function anal- open-loop and closed-loop is summarized as ysis 2g0n十ws=wp (4) 0+28n@ms =K' (5) In the typical CPLL frequency synthesizer wnwps =K'wz (6) with continuous-time loop filter,as shown in Fig. According to Eqs.(4)(6),the analytical equation 1,the open loop transfer function is is derived, H.(s)-KerKvea x Z(s) N (1) 2知-(42-1)w2+wp)wa+2 pWz=0(7) where Kcr is the gain of phase detector and charge Suppose @n=mwz,where m is the nature frequency pump,Z(s)is the impedance of loop filter. factor.Take it into Eq.(7),and then derive K。=g20=总×0 29m2-(4+b)m+25(b+1)=0(8) Solving Eq.(8),m can be expressed as a function 1 0.=RC:-(b+1)o of b and ,i.e.m=m(b,). From Eq.(4),the third pole @ps in the closed- Here b is the ratio between C and C2.The open loop transfer function can be expressed as loop transfer function can be rewritten as Wps (b+1-2gm)wz (9) H.(s)=K's+w: (2) which is often neglected by the second order sim- s2(s+@p) plification.第&期 I=.8=9=+>S( *)AW,UN99L(?F=JD7F=RUD=^N=?:L,L?FG=<8]=D@8FG,F7K898FL)E6M=?<7F8E? 9EEM8<FG8DREDR=DED=X=?HENDFGEDR=D>WM=?09EEM <0RE678?6ER=98<R8<:N<<=R8?FG=FG8DREDR=D-!. > *9FGENJG7?EM=?09EEM<0RE678? 6ER=98<<86M9= HED7?79L<8<$FG=DENJG9LR=H8?=RMG7<= 67DJ8?8< 8?R8D=:F7?R ?EF7K9=FE7::ND7F=9L R=<:D8K=FG= :9E<=R09EEMM=DHED67?:=7?RMD=R8:FFG==HH=:FEH FG=M7D76=F=D<*-V/X7D87F8E?>/G=:E6M9=F=7?790 L<8<E?FG=:9E<=R09EEMFG8DR0EDR=D<0RE678?6ER=9 8<<F8997K<=?FNMFE?E@> (?FG8<M7M=D$FG=:E6M9=F=7?79L<8<E?FG= :9E<=R09EEMFG8DR0EDR=D<0RE678?8<M=DHED6=RFE R=D8X=FG=R=<8J?MDE:=RND=<HED9EEMM7D76=F=D<> /G==HH=:F<EHFG=M7D76=F=D<*-V/X7D87F8E?E? FG=<F7K898FL8<^N7?F8F7F8X=9L7?79L]=R@8FGFG=78R EHFG=DEEF9E:N<F=:G?8^N=>*?7R;N<F7K9=:NDD=?F :=998?FG=:G7DJ=MN6M8<MDEME<=RFED=798]=FG= R76M8?JH7:FED:E?FDE9FE:E6M=?<7F=FG=FEF79X7D0 87F8E?EHFG=M7D76=F=D<$@G8:G8<K7<=RE?FG=X7D80 7F8E?7?79L<8<>* ?EX=9:DE<<0<@8F:G=R V)W @8FG 98?=7DJ78?8<79<E7REMF=RFED=RN:=FG=FEF79X7D870 F8E?> + 0:9>@LW:99D?HJALW9AL@A>WL9RCJGCW GC:I>J>CGL:99DDCACR@?@A> +YE 0:9>@LW:99D?HJALW9AL@A?ACG>M@AM<GN?J9GCGC:W I>J> (?FG=FLM8:79)-PP HD=^N=?:L<L?FG=<8]=D @8FG:E?F8?NEN<0F86=9EEMH89F=D$7<<GE@?8?U8J> $$FG=EM=?9EEMFD7?<H=DHN?:F8E?8< 7E%;&V N)-NV)W G [5%;& ; %$& @G=D=N)-8<FG=J78?EHMG7<=R=F=:FED7?R:G7DJ= MN6M$5%;&8<FG=86M=R7?:=EH9EEMH89F=D> N)- VT)- !($5%;&V H A$ [ ;Z*] ;%;Z*M& $ *] V $ BA$ $*M V %HZ$&*] I=D=H8<FG=D7F8EK=F@==?A$ 7?RA!>/G=EM=? 9EEMFD7?<H=DHN?:F8E?:7?K=D=@D8FF=?7< 7E%;&V N\ ;Z*] ;!%;Z*M& %!& @G=D=N\ V N)-NV)WH GA$ S U8J>$ *D:G8F=:FND=EHHD=^N=?:L<L?FG=<8]=D )9E<=R09EEMFD7?<H=DHN?:F8E?<G7X=K==?<860 M98H8=RFE<=:E?REDR=D7?RR8<:N<<=R8?Z=H>-$.> IE@=X=D$FG=<86M98H8=R7?79L<8< @899 68<<<E6= 86M98:8FD=97F8E?<G8M<K=F@==?FG=M7D76=F=D<7?R M=DHED67?:=>/G=:E6M9=F=:9E<=R09EEMFG8DR0EDR=D FD7?<H=DHN?:F8E?6N<FK=:E?<8R=D=R7<HE99E@8?J$ 7%;&V G7E%;& $Z7E%;&V GN\%;Z*]& ;4 Z*M;! ZN\;ZN\*] V GN\%;Z*]& %;! Z!+*?;Z*! ?&%;Z*M4& %4& @G=D=*?8<FG=?7FND=HD=^N=?:L$+8<FG=R76M8?J H7:FED$*M48<FG=:9E<=R09EEM<8?J9=ME9=> /G=D=97F8E?<G8MEH]=DE<7?RME9=<K=F@==? EM=?09EEM7?R:9E<=R09EEM8<<N667D8]=R7< !+*?Z*M4 V*M %2& *! ?Z!+*?*M4 V N\ %5& *! ?*M4 V N\*] %#& *::EDR8?JFE’^<>%2&#%#&$FG=7?79LF8:79=^N7F8E? 8<R=D8X=R$ !+*! ?Y %%2+ ! Y$&*]Z*M&*?Z!+*M*] V"%3& ,NMME<=*?g8*]$@G=D=88<FG=?7FND=HD=^N=?:L H7:FED>/7a=8F8?FE’^>%3&$7?RFG=?R=D8X= !+8! Y %2+ ! ZH&8Z!+%HZ$&V" %&& ,E9X8?J’^>%&&$8 :7?K==\MD=<<=R7<7HN?:F8E? EHH7?R+$8>=>8g8%H$+&S UDE6’^>%2&$FG=FG8DRME9=*M48?FG=:9E<=R0 9EEMFD7?<H=DHN?:F8E?:7?K==\MD=<<=R7< *M4 V %HZ$Y!+8&*] %%& @G8:G8<EHF=??=J9=:F=RKLFG=<=:E?REDR=D<860 M98H8:7F8E?> $5!5