正在加载图片...
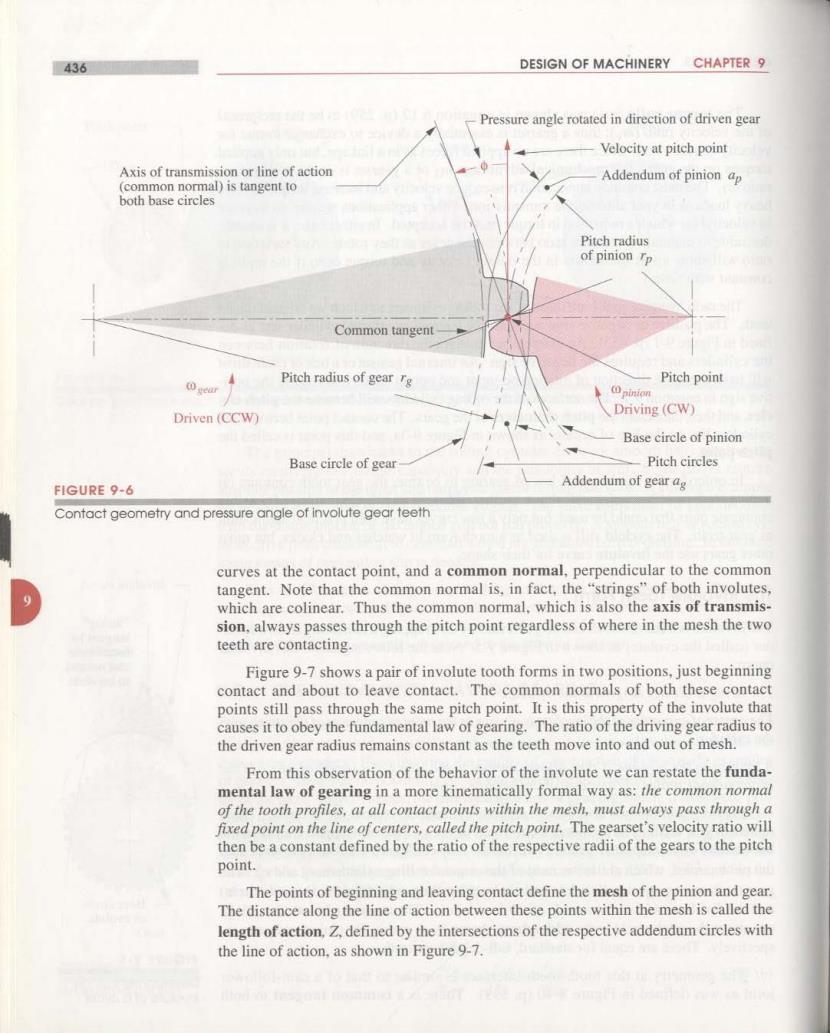
436 DESIGN OF MACHINERY CHAPTER 9 Pressure angle rotated in direction of driven gear Velocity at pitch point Axis of transmission or line of action Addendum of pinion ap (common normal)is tangent to both base circles Pitch radius of pinion rp Common tangent geur Pitch radius of gear re Pitch point pinion Driven(CCW) Driving(CW) Base circle of pinion Base circle of gear Pitch circles FIGURE 9-6 Addendum of gear ag Contact geometry and pressure angle of involute gear teeth curves at the contact point,and a common normal,perpendicular to the common tangent.Note that the common normal is,in fact,the "strings"of both involutes, which are colinear.Thus the common normal,which is also the axis of transmis- sion,always passes through the pitch point regardless of where in the mesh the two teeth are contacting. Figure 9-7 shows a pair of involute tooth forms in two positions,just beginning contact and about to leave contact.The common normals of both these contact points still pass through the same pitch point.It is this property of the involute that causes it to obey the fundamental law of gearing.The ratio of the driving gear radius to the driven gear radius remains constant as the teeth move into and out of mesh. From this observation of the behavior of the involute we can restate the funda- mental law of gearing in a more kinematically formal way as:the common normal of the tooth profiles,at all contact points within the mesh,must always pass through a fixed point on the line of centers,called the pitch point.The gearset's velocity ratio will then be a constant defined by the ratio of the respective radii of the gears to the pitch point. The points of beginning and leaving contact define the mesh of the pinion and gear. The distance along the line of action between these points within the mesh is called the length of action,Z,defined by the intersections of the respective addendum circles with the line of action,as shown in Figure 9-7