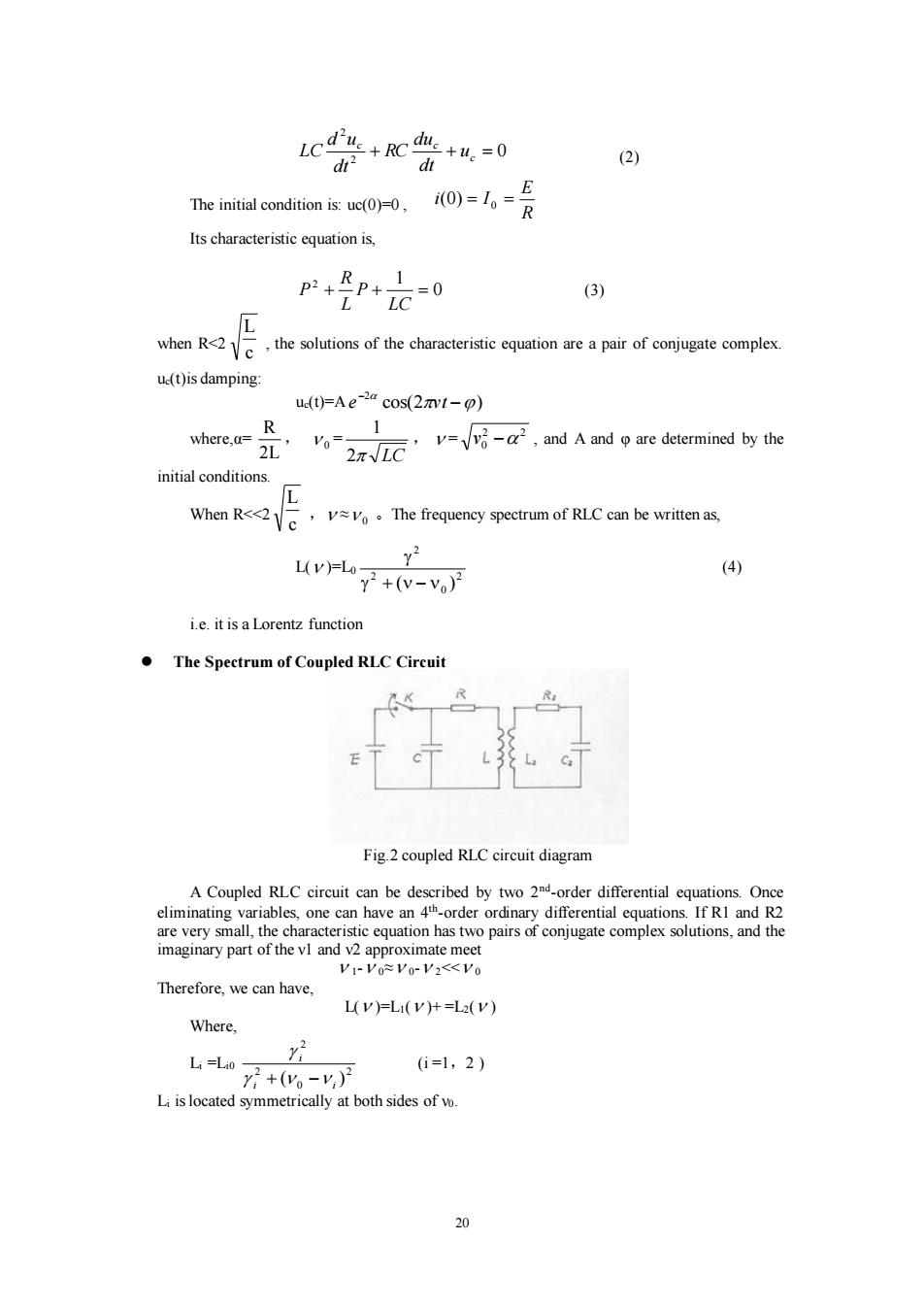
Lcd+血+M.=0 dt2 dt (2) The initial condition is:uc()=0, 0)=1。= R Its characteristic equation is, p2+ RP* 1 =0 L LC (3) L when R<2c the solutions of the characteristic equation are a pair of conjugate complex. ue(t)is damping: u()=Ae-2a cos(2n1-) R 1 where,a= 2L Vo= =,=v-a2,and A and are determined by the 2πVL initial conditions. L When R<<21 ，v≈vo。The frequency spectrum of RLC can be written as, L(V)=L0- (4) 2+(v-vo)2 i.e.it is a Lorentz function The Spectrum of Coupled RLC Circuit Fig.2 coupled RLC circuit diagram A Coupled RLC circuit can be described by two 2nd-order differential equations.Once eliminating variables,one can have an 4th-order ordinary differential equations.If RI and R2 are very small,the characteristic equation has two pairs of conjugate complex solutions,and the imaginary part of the vl and v2 approximate meet V1-Vo≈V0-V2<<Vo Therefore,we can have, L(V）=L(V)+=L2(V) Where, Li=Lio- (i=1,2) +(yo-y,)2 Li is located symmetrically at both sides of vo. 2020 0 2 2 + + c = c c u dt du RC dt d u LC (2) The initial condition is: uc(0)=0 , R E i(0) = I 0 = Its characteristic equation is, 0 2 1 + + = LC P L R P (3) when R<2 c L , the solutions of the characteristic equation are a pair of conjugate complex. uc(t)is damping: uc(t)=A cos(2 ) 2    − − e vt where,α= 2L R ，  0 = 2 LC 1 ，  = 2 2 v0 − , and A and φ are determined by the initial conditions. When R<<2 c L ， ≈ 0 。The frequency spectrum of RLC can be written as, L(  )=L0 2 0 2 2  + ( −  )  (4) i.e. it is a Lorentz function ⚫ The Spectrum of Coupled RLC Circuit Fig.2 coupled RLC circuit diagram A Coupled RLC circuit can be described by two 2nd -order differential equations. Once eliminating variables, one can have an 4 th -order ordinary differential equations. If R1 and R2 are very small, the characteristic equation has two pairs of conjugate complex solutions, and the imaginary part of the ν1 and ν2 approximate meet  1- 0≈ 0- 2<<  0 Therefore, we can have, L(  )=L1(  )+ =L2(  ) Where, Li =Li0 2 0 2 2 ( ) i i i     + − (i =1，2 ) Li is located symmetrically at both sides of ν0