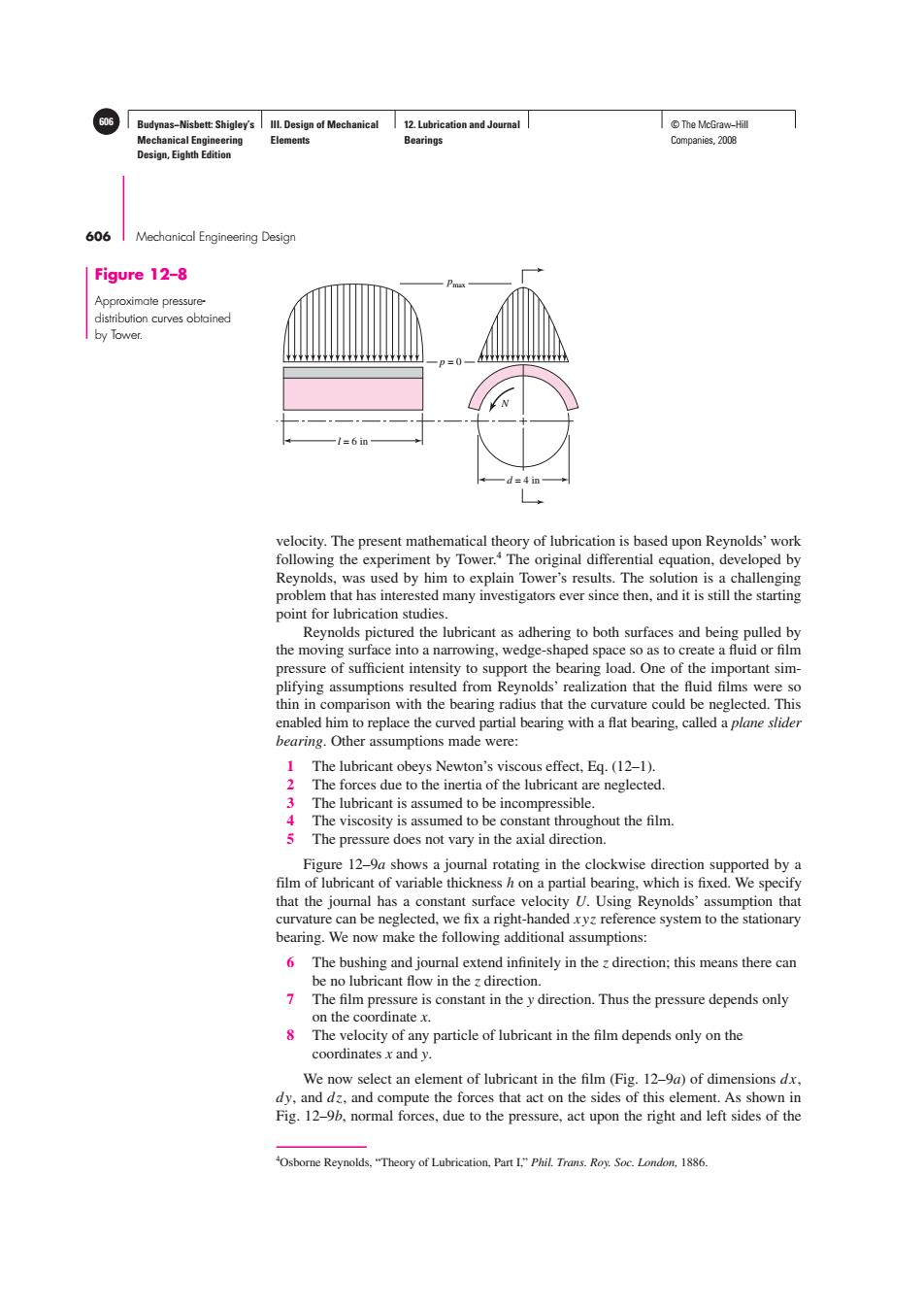
606 Budynas-Nisbett:Shigley's Ill.Design of Mechanical 12.Lubrication and Journal T©The McGraw-Hil Mechanical Engineering Elements Bearings Companies,2008 Design,Eighth Edition 606 Mechanical Engineering Design Figure 12-8 Approximate pressure- distribution curves obtained by Tower. =6in =4n velocity.The present mathematical theory of lubrication is based upon Reynolds'work following the experiment by Tower.The original differential equation,developed by Reynolds,was used by him to explain Tower's results.The solution is a challenging problem that has interested many investigators ever since then,and it is still the starting point for lubrication studies Reynolds pictured the lubricant as adhering to both surfaces and being pulled by the moving surface into a narrowing,wedge-shaped space so as to create a fluid or film pressure of sufficient intensity to support the bearing load.One of the important sim- plifying assumptions resulted from Reynolds'realization that the fluid films were so thin in comparison with the bearing radius that the curvature could be neglected.This enabled him to replace the curved partial bearing with a flat bearing,called a plane slider bearing.Other assumptions made were: 1 The lubricant obeys Newton's viscous effect,Eg.(12-1). 2 The forces due to the inertia of the lubricant are neglected. 3 The lubricant is assumed to be incompressible. 4 The viscosity is assumed to be constant throughout the film. 5 The pressure does not vary in the axial direction. Figure 12-9a shows a journal rotating in the clockwise direction supported by a film of lubricant of variable thickness h on a partial bearing.which is fixed.We specify that the journal has a constant surface velocity U.Using Reynolds'assumption that curvature can be neglected,we fix a right-handed xyz reference system to the stationary bearing.We now make the following additional assumptions: 6 The bushing and journal extend infinitely in the zdirection:this means there can be no lubricant flow in the z direction. 7 The film pressure is constant in the y direction.Thus the pressure depends only on the coordinate x. 8 The velocity of any particle of lubricant in the film depends only on the coordinates x and y. We now select an element of lubricant in the film (Fig.12-9a)of dimensions dx. dy,and dz,and compute the forces that act on the sides of this element.As shown in Fig.12-9b,normal forces,due to the pressure,act upon the right and left sides of the Osborne Reynolds,"Theory of Lubrication,Part I,"Phil.Trans.Roy.Soc.London,1886Budynas−Nisbett: Shigley’s Mechanical Engineering Design, Eighth Edition III. Design of Mechanical Elements 12. Lubrication and Journal Bearings 606 © The McGraw−Hill Companies, 2008 606 Mechanical Engineering Design Figure 12–8 Approximate pressure￾distribution curves obtained by Tower. pmax p = 0 N l = 6 in d = 4 in velocity. The present mathematical theory of lubrication is based upon Reynolds’ work following the experiment by Tower.4 The original differential equation, developed by Reynolds, was used by him to explain Tower’s results. The solution is a challenging problem that has interested many investigators ever since then, and it is still the starting point for lubrication studies. Reynolds pictured the lubricant as adhering to both surfaces and being pulled by the moving surface into a narrowing, wedge-shaped space so as to create a fluid or film pressure of sufficient intensity to support the bearing load. One of the important sim￾plifying assumptions resulted from Reynolds’ realization that the fluid films were so thin in comparison with the bearing radius that the curvature could be neglected. This enabled him to replace the curved partial bearing with a flat bearing, called a plane slider bearing. Other assumptions made were: 1 The lubricant obeys Newton’s viscous effect, Eq. (12–1). 2 The forces due to the inertia of the lubricant are neglected. 3 The lubricant is assumed to be incompressible. 4 The viscosity is assumed to be constant throughout the film. 5 The pressure does not vary in the axial direction. Figure 12–9a shows a journal rotating in the clockwise direction supported by a film of lubricant of variable thickness h on a partial bearing, which is fixed. We specify that the journal has a constant surface velocity U. Using Reynolds’ assumption that curvature can be neglected, we fix a right-handed xyz reference system to the stationary bearing. We now make the following additional assumptions: 6 The bushing and journal extend infinitely in the z direction; this means there can be no lubricant flow in the z direction. 7 The film pressure is constant in the y direction. Thus the pressure depends only on the coordinate x. 8 The velocity of any particle of lubricant in the film depends only on the coordinates x and y. We now select an element of lubricant in the film (Fig. 12–9a) of dimensions dx, dy, and dz, and compute the forces that act on the sides of this element. As shown in Fig. 12–9b, normal forces, due to the pressure, act upon the right and left sides of the 4 Osborne Reynolds, “Theory of Lubrication, Part I,” Phil. Trans. Roy. Soc. London, 1886