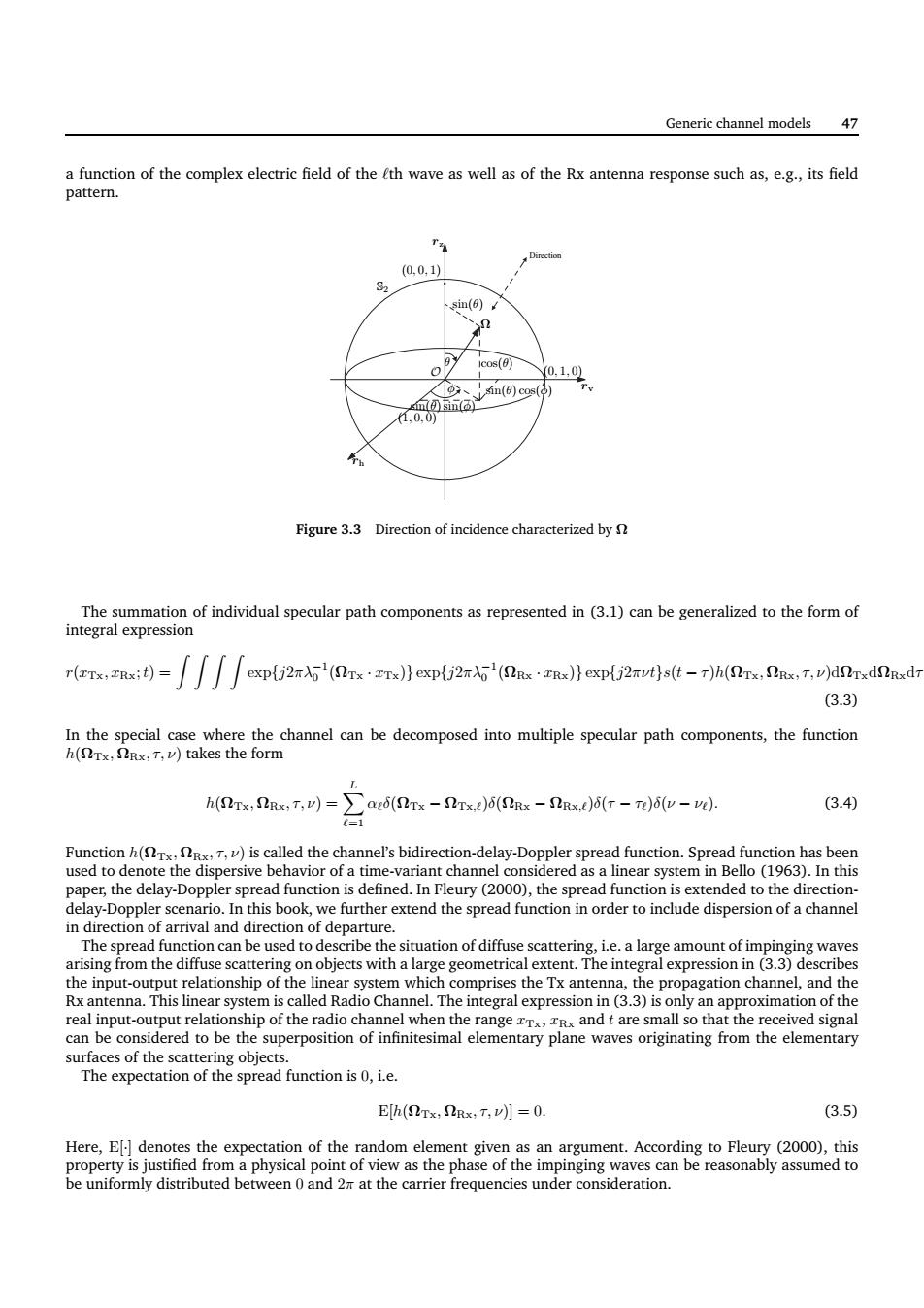
Generic channel models 47 ion of the complex clectrie field of the th wave as well as of the R antenna response such s,g,its fiel n(0)cos( Figure .3 Direction of incidence characterized by The summation of individual specular path components as represented in (3.1)can be generalized to the form of integral expression r(Tx,rx:t)=exp{j (Sx))exp(j ()]exp(j2rut)s(t-T)h(,Sax,T,v) (3.3) In the special case where the channel can be decomposed into multiple specular path components.the function h(Tx,SRs,Tv)takes the form h(s,m)=(s-)6(us-Su)6(r-)6(v-) (3.4 the chanel's bidirection-delay-Doppler spread function.Spread funcion has been paper,the delay-Dopplersp ead function is defined.Fleury(2000),the spread function is extended to the direction delay-Doppler scenario.Ir this book,we further extend the spread function inorder toinclude dispersion of a channel he preaboderbe the io ofiernm e部op0rcc se sca on objects with a large geometrical extent.The integ scribes can ementary faces of the scatt The expectation of the spread function is0,i.e. E[h(Tx,Rx,T,v】=0. (3.5) of the to fley 2000).this er considerationGeneric channel models 47 a function of the complex electric field of the ℓth wave as well as of the Rx antenna response such as, e.g., its field pattern. Direction S2 rh rv rz (0, 0, 1) (0, 1, 0) (1, 0, 0) sin(θ) cos(θ) sin(θ) sin(φ) sin(θ) cos(φ) θ φ O Ω Figure 3.3 Direction of incidence characterized by Ω The summation of individual specular path components as represented in (3.1) can be generalized to the form of integral expression r(xTx, xRx;t) = Z Z Z Z exp{j2πλ−1 0 (ΩTx · xTx)} exp{j2πλ−1 0 (ΩRx · xRx)} exp{j2πνt}s(t − τ)h(ΩTx, ΩRx, τ, ν)dΩTxdΩRxdτd (3.3) In the special case where the channel can be decomposed into multiple specular path components, the function h(ΩTx, ΩRx, τ, ν) takes the form h(ΩTx, ΩRx, τ, ν) = X L ℓ=1 αℓδ(ΩTx − ΩTx,ℓ)δ(ΩRx − ΩRx,ℓ)δ(τ − τℓ)δ(ν − νℓ). (3.4) Function h(ΩTx, ΩRx, τ, ν) is called the channel’s bidirection-delay-Doppler spread function. Spread function has been used to denote the dispersive behavior of a time-variant channel considered as a linear system in Bello (1963). In this paper, the delay-Doppler spread function is defined. In Fleury (2000), the spread function is extended to the direction￾delay-Doppler scenario. In this book, we further extend the spread function in order to include dispersion of a channel in direction of arrival and direction of departure. The spread function can be used to describe the situation of diffuse scattering, i.e. a large amount of impinging waves arising from the diffuse scattering on objects with a large geometrical extent. The integral expression in (3.3) describes the input-output relationship of the linear system which comprises the Tx antenna, the propagation channel, and the Rx antenna. This linear system is called Radio Channel. The integral expression in (3.3) is only an approximation of the real input-output relationship of the radio channel when the range xTx, xRx and t are small so that the received signal can be considered to be the superposition of infinitesimal elementary plane waves originating from the elementary surfaces of the scattering objects. The expectation of the spread function is 0, i.e. E[h(ΩTx, ΩRx, τ, ν)] = 0. (3.5) Here, E[·] denotes the expectation of the random element given as an argument. According to Fleury (2000), this property is justified from a physical point of view as the phase of the impinging waves can be reasonably assumed to be uniformly distributed between 0 and 2π at the carrier frequencies under consideration