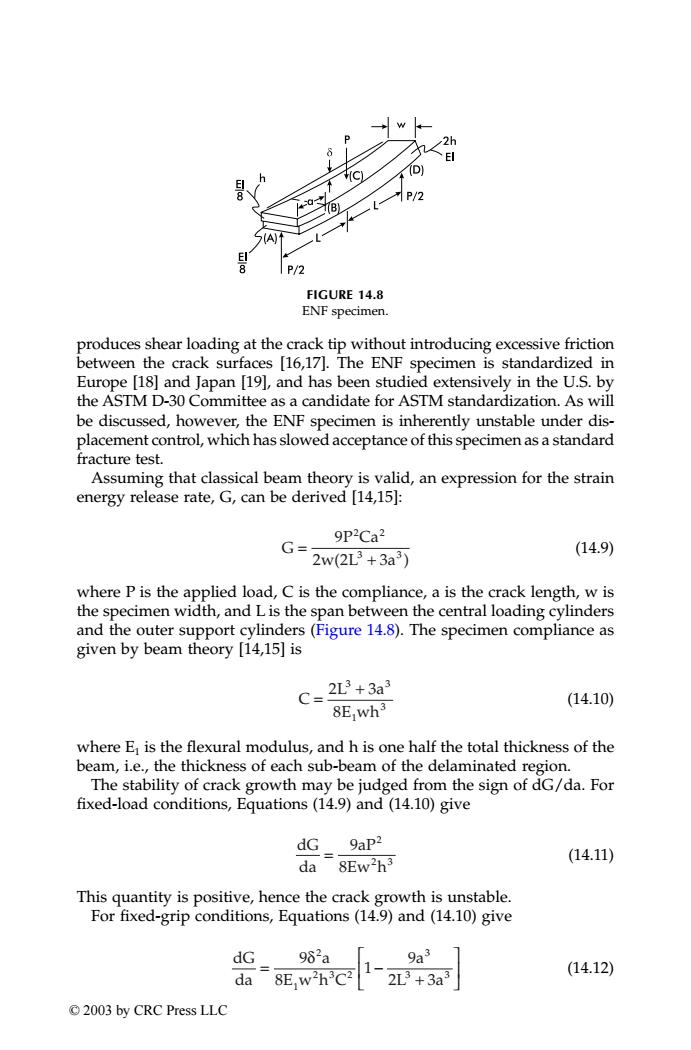
P/2 (B) P/2 FIGURE 14.8 ENF specimen. produces shear loading at the crack tip without introducing excessive friction between the crack surfaces [16,17].The ENF specimen is standardized in Europe [18]and Japan [19],and has been studied extensively in the U.S.by the ASTM D-30 Committee as a candidate for ASTM standardization.As will be discussed,however,the ENF specimen is inherently unstable under dis- placement control,which has slowed acceptance of this specimen as a standard fracture test. Assuming that classical beam theory is valid,an expression for the strain energy release rate,G,can be derived [14,15]: 9P2Ca2 G= (14.9) 2w(2L3+3a3) where P is the applied load,C is the compliance,a is the crack length,w is the specimen width,and L is the span between the central loading cylinders and the outer support cylinders(Figure 14.8).The specimen compliance as given by beam theory [14,15]is c= L+3a3 (14.10) 8E wh3 where E is the flexural modulus,and h is one half the total thickness of the beam,i.e.,the thickness of each sub-beam of the delaminated region. The stability of crack growth may be judged from the sign of dG/da.For fixed-load conditions,Equations (14.9)and (14.10)give dG 9ap2 da 8Ew2h3 (14.11) This quantity is positive,hence the crack growth is unstable. For fixed-grip conditions,Equations(14.9)and(14.10)give dG 982a 9a3 da 8E,w2hC2 2L3+3a3 (14.12) 2003 by CRC Press LLCproduces shear loading at the crack tip without introducing excessive friction between the crack surfaces [16,17]. The ENF specimen is standardized in Europe [18] and Japan [19], and has been studied extensively in the U.S. by the ASTM D-30 Committee as a candidate for ASTM standardization. As will be discussed, however, the ENF specimen is inherently unstable under dis￾placement control, which has slowed acceptance of this specimen as a standard fracture test. Assuming that classical beam theory is valid, an expression for the strain energy release rate, G, can be derived [14,15]: (14.9) where P is the applied load, C is the compliance, a is the crack length, w is the specimen width, and L is the span between the central loading cylinders and the outer support cylinders (Figure 14.8). The specimen compliance as given by beam theory [14,15] is (14.10) where E1 is the flexural modulus, and h is one half the total thickness of the beam, i.e., the thickness of each sub-beam of the delaminated region. The stability of crack growth may be judged from the sign of dG/da. For fixed-load conditions, Equations (14.9) and (14.10) give (14.11) This quantity is positive, hence the crack growth is unstable. For fixed-grip conditions, Equations (14.9) and (14.10) give (14.12) FIGURE 14.8 ENF specimen. G P Ca wL a = + 9 22 3 2 2 3 3 ( ) C L a E wh = 2 3 + 8 3 3 1 3 dG da aP Ew h = 9 8 2 2 3 dG da a EwhC a L a = − +       9 8 1 9 2 3 2 1 23 2 3 3 3 δ TX001_ch14_Frame Page 192 Saturday, September 21, 2002 5:09 AM © 2003 by CRC Press LLC