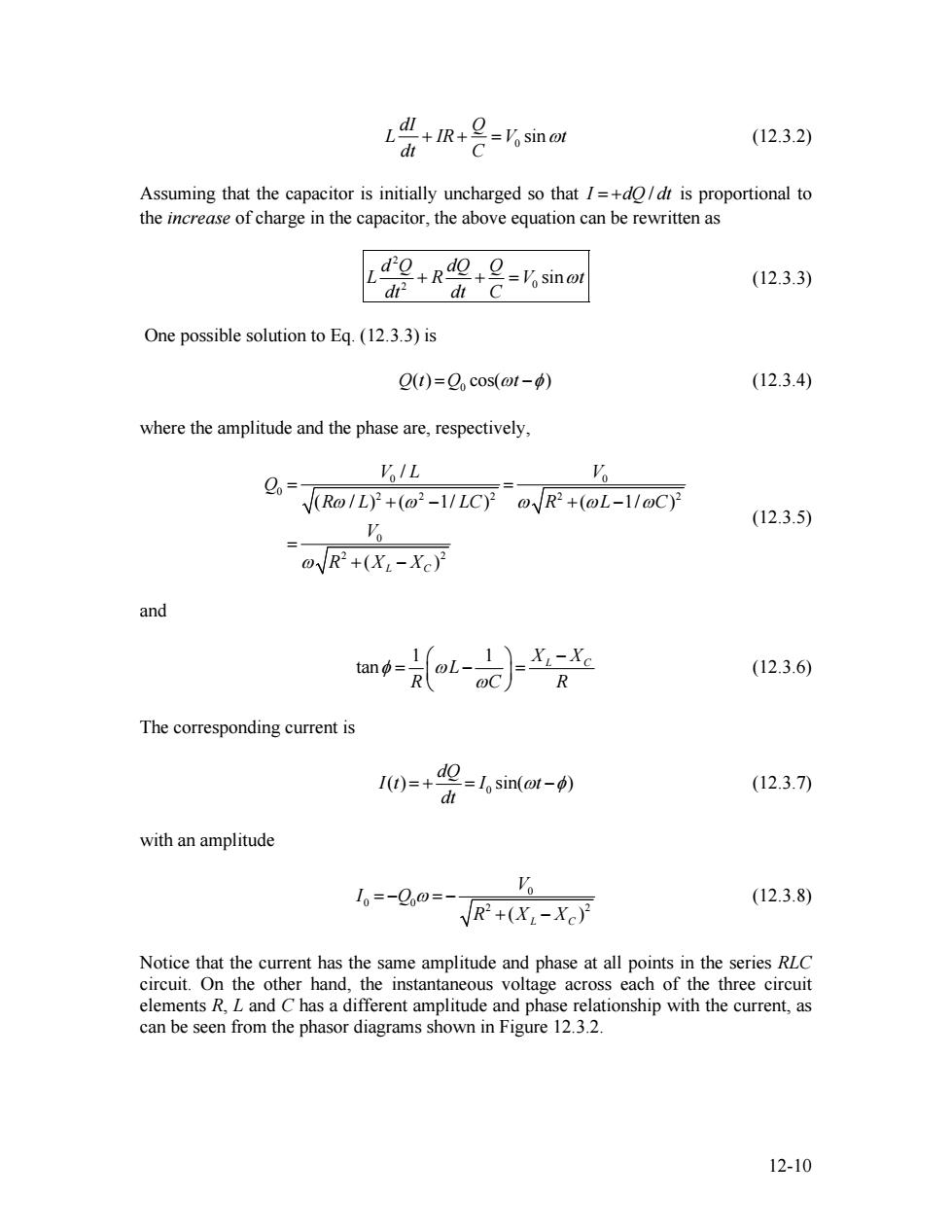
+I-Vosinot (12.3.2) d Assuming that the capacitor is initially uncharged so that I=+do/dt is proportional to the increase of charge in the capacitor,the above equation can be rewritten as do +Ra№+9=osinot C (12.3.3) d d One possible solution to Eq.(12.3.3)is Q(t)=Qcos(ot-φp) (12.3.4) where the amplitude and the phase are,respectively, '%/L Vo R-VLCy @R2+(@L-1/@C) (12.3.5) oVR2+(X-Xc)月 and tand= oi-nc (12.3.6) R @C The corresponding current is I()= d-l,sin(@t--p） (12.3.7) dt with an amplitude 10=-Qo0=- a (12.3.8) VR+(X-Xc)2 Notice that the current has the same amplitude and phase at all points in the series RLC circuit.On the other hand,the instantaneous voltage across each of the three circuit elements R,L and C has a different amplitude and phase relationship with the current,as can be seen from the phasor diagrams shown in Figure 12.3.2. 12-100 sin dI Q L IR V dt C + + = ωt (12.3.2) Assuming that the capacitor is initially uncharged so that I = +dQ / dt is proportional to the increase of charge in the capacitor, the above equation can be rewritten as 2 2 0 sin d Q dQ Q L R V dt dt C + + = ωt (12.3.3) One possible solution to Eq. (12.3.3) is 0 Q t( ) =Q cos(ωt −φ) (12.3.4) where the amplitude and the phase are, respectively, 0 0 0 2 2 2 2 0 2 2 / ( / ) ( 1/ ) ( 1/ ) ( ) L C V L V Q 2 R L LC R L V R X X ω ω ω ω ω ω = = + − + − = + − C (12.3.5) and 1 1 tan XL XC L R C R φ ω ω ⎛ ⎞ − = − ⎜ ⎟ = ⎝ ⎠ (12.3.6) The corresponding current is 0 ( ) sin( ) dQ I t I t dt = + = ω −φ (12.3.7) with an amplitude 0 0 0 2 ( ) L C V I Q R X X = − ω = − + − 2 (12.3.8) Notice that the current has the same amplitude and phase at all points in the series RLC circuit. On the other hand, the instantaneous voltage across each of the three circuit elements R, L and C has a different amplitude and phase relationship with the current, as can be seen from the phasor diagrams shown in Figure 12.3.2. 12-10