CHAPTER 11 STRAIN ENERGY Summary The energy stored within a material when work has been done on it is termed the strain energy or resilience, i.e. strain energy work done In general there are four types of loading which can be applied to a material: 1.Direct load (tension or compression) CP2ds P2L Strain energy U= 2AE or 2AE o2AL a2 2E=2E :×volume of bar 2.Shear load fo2ds 02L Strain energy U= 2AG or 2AG 2G×AL= 2G x volume of bar 3.Bending M2ds M2L Strain energy U= or 2EI 2EI if M is constant 4.Torsion "T2ds T2L Strain energy U= or 2GJ 2GJ if T is constant From 1 above,the strain energy or resilience when the tensile stress reaches the proof stress p,i.e.the proof resilience,is x volume of bar 2E and the modulus of resilience is 2E The strain energy per unit volume of a three-dimensional principal stress system is 1 U=2E[oi+吃+号-2w(o102+a203+031】 254
CHAPTER 11 STRAIN ENERGY Summary The energy stored within a material when work has been done on it is termed the strain energy or resilience, i.e. strain energy = work done In general there are four types of loading which can be applied to a material: 1. Direct load (tension or compression) 1% P2 L or - 2 AE Strain energy U = O~AL a2 =-- -- x volume of bar 2E 2E 2. Shear load j‘g or - QZL 2 AG Strain energy U = 72 T2 2G 2G Strain energy U = 1:; - or - if M is constant = - x AL = - x volume of bar 3. Bending M2L 2 EZ 4. Torsion if T is constant 1; T2L or - 2GJ Strain energy U = - From 1 above, the strain energy or resilience when the tensile stress reaches the proof stress ap, i.e. the proof resilience, is - 4 x volume of bar 2E and the modulus of resilience is - 0; 2E The strain energy per unit volume of a three-dimensional principal stress system is 1 2E U =--a:+a 254

Strain Energy 255 The volumetric or "dilatational"strain energy per unit volume is then -2[o,+2+P门 6E and the shear,or"distortional",strain energy per unit volume is 12G[o1-2P+(o2-032+(a3-1}] 1 The maximum instantaneous stress in a uniform bar caused by a weight Wfalling through a distance h on to the bar is given by (+] The instantaneous extension is then given by 6-% If this is small compared to the height h,then 2WEh AL For any shock-loaded system the instantaneous deflection is given by -±(+】 where 6,is the deflection under an equal static load. Castigliano's first theorem for deflection states that: If the total strain energy expressed in terms of the external loads is partially differentiated with respect to one of the loads the result is the deflection of the point of application of that load and in the direction of that load (see Examples 11.5 and 11.6): aU i.e. Deflection in direction of In applications where bending provides practically all of the strain energy, CM2ds [MOM =aw]2EI ds EI aW This is sometimes written in the form 6= Mm E where m OM aw the bending moment resulting from a unit load only in the place of W.This method of solution is then termed the unit load method
Strain Energy 255 The volumetric or “dilatational” strain energy per unit volume is then and the shear, or “distortional”, strain energy per unit volume is 1 - [(ai- 02)2 + (02 - 4 + (03 - (71)21 12G The maximum instantaneous stress in a uniform bar caused by a weight W falling through a distance h on to the bar is given by 2 WEh A- The instantaneous extension is then given by dL 6=- E If this is small compared to the height h, then //2 WEh\ For any shock-loaded system the instantaneous deflection is given by 6 = 6, [ 1 * J( 1 +;)I where 6, is the deflection under an equal static load. Castigliano’sfirst theorem for tiefiction states that: If the total strain energy expressed in terms of the external loads is partially diyerentiated with respect to one of the loads the result is the defection of the point of application of that load and in the direction of that load (see Examples 11.5 and 11.6): au aw i.e. Deflection in direction of W = - = 6 In applications where bending provides practically all of the strain energy, This is sometimes written in the form 8M where m = ~ = the bending moment resulting from a unit load only in the place of W. This method of solution is then termed the unit load method. aw
256 Mechanics of Materials Castigliano's theorem also applies to angular movements: If the total strain energy expressed in terms of the external moments be partially differentiated with respect to one of the moments,the result is the angular deflection in radians of the point of application of that moment and in its direction 0-EI OM where M;is the actual or imaginary moment at the point where 0 is required. Defections due to shear Beam loading Shear deflection Rectangular-section beam I-section beam 6WL WL Cantilever-concentrated end load W' 5AG AG 3wL2 wL2 WL Cantilever-u.d.I. 5AG 2AG2AG Simply supported 3WL WL beam-central concentrated load W 10AG 4AG Simply supported beam-concentrated 6Wab load dividing span SAGL into lengths a and b 3wL2 wL2 WL Simply supported 8AG8AG beam-u.d.l. 20AG Introduction Energy is normally defined as the capacity to do work and it may exist in any of many forms, e.g.mechanical(potential or kinetic),thermal,nuclear,chemical,etc.The potential energy ofa body is the form of energy which is stored by virtue of the work which has previously been done on that body,e.g.in lifting it to some height above a datum.Strain energy is a particular form of potential energy which is stored within materials which have been subjected to strain, i.e.to some change in dimension.The material is then capable of doing work,equivalent to the amount of strain energy stored,when it returns to its original unstrained dimension. Strain energy is therefore defined as the energy which is stored within a material when work has been done on the material.Here it is assumed that the material remains elastic whilst work is done on it so that all the energy is recoverable and no permanent deformation occurs due to yielding of the material, i.e. strain energy U work done Thus for a gradually applied load the work done in straining the material will be given by the shaded area under the load-extension graph of Fig.11.1. U=Pδ
256 Mechanics of Materials Beam loading Castigliano’s theorem also applies to angular movements: If the total strain energy expressed in terms of the external moments be partially diferentiated with respect to one of the moments, the result is the angular deflection in radians of the point of application of that moment and in its direction M 8M O= --d~ 1.1 aMi where Mi is the actual or imaginary moment at the point where 0 is required, Deflections due to shear Shear deflection Rectangular-section beam I-section beam 6 WL WL Cantileverxoncentrated end load W’ Cantilever-u.d.1. 3WLZ 5AG __ Simply supported 3WL beam -central concentrated load W Simply supported beam - concentrated load dividing span into lengths a and b Simply supported beam-u.d.1. 6 Wab 5AGL ~ wL2 WL 2AG ZAG Idlo WL 3wL2 20AG __ wL2 WL 8AG 8AG Introduction Energy is normally defined as the capacity to do work and it may exist in any of many forms, e.g. mechanical (potential or kinetic), thermal, nuclear, chemical, etc. The potential energy of a body is the form of energy which is stored by virtue of the work which has previously been done on that body, e.g. in lifting it to some height above a datum. Strain energy is a particular form of potential energy which is stored within materials which have been subjected to strain, i.e. to some change in dimension. The material is then capable of doing work, equivalent to the amount of strain energy stored, when it returns to its original unstrained dimension. Strain energy is therefore deJined as the energy which is stored within a material when work has been done on the material. Here it is assumed that the material remains elastic whilst work is done on it so that all the energy is recoverable and no permanent deformation occurs due to yielding of the material, i.e. strain energy U = work done the shaded area under the load-extension graph of Fig. 11.1. Thus for a gradually applied load the work done in straining the material will be given by U=iPG
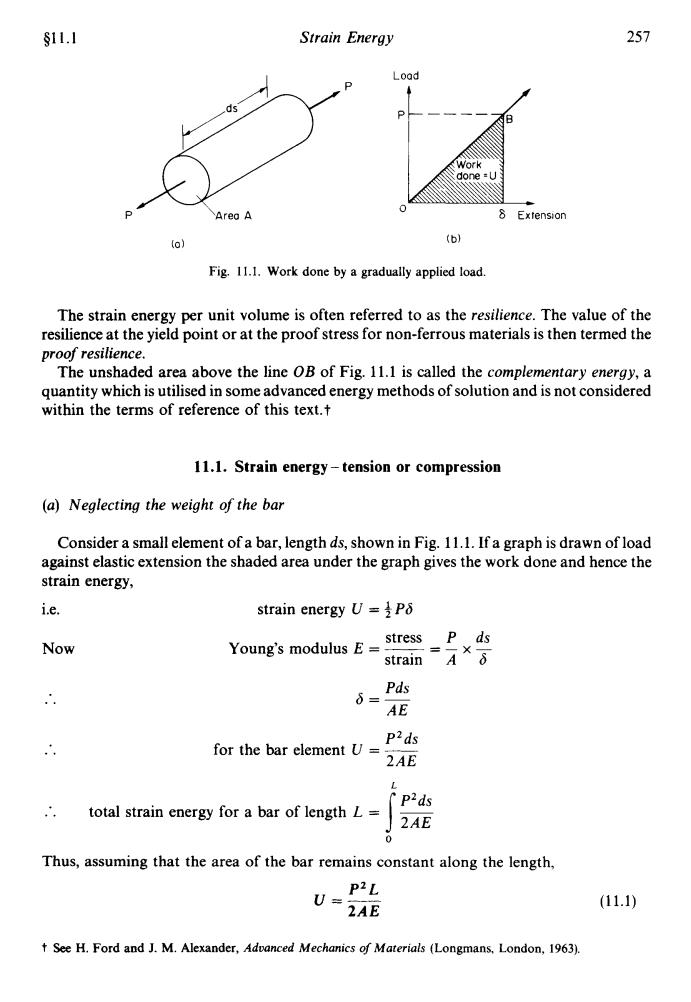
S11.1 Strain Energy 257 Load Work done±U Areo A 8 Extension (o) (b】 Fig.11.1.Work done by a gradually applied load. The strain energy per unit volume is often referred to as the resilience.The value of the resilience at the yield point or at the proof stress for non-ferrous materials is then termed the proof resilience. The unshaded area above the line OB of Fig.11.1 is called the complementary energy,a quantity which is utilised in some advanced energy methods of solution and is not considered within the terms of reference of this text.t 11.1.Strain energy-tension or compression (a)Neglecting the weight of the bar Consider a small element of a bar,length ds,shown in Fig.11.1.If a graph is drawn of load against elastic extension the shaded area under the graph gives the work done and hence the strain energy, i.e. strain energy U=Pδ stress P ds Now Young's modulus E= strain=A×8 Pds 6= AE P2ds for the bar element U= 2AE f p2ds total strain energy for a bar of length L= 2AE 0 Thus,assuming that the area of the bar remains constant along the length, P2L U= 2AE (11.1) t See H.Ford and J.M.Alexander,Advanced Mechanics of Materials (Longmans.London,1963)
$11.1 Strain Energy 257 Load P P 6 Extension Fig. 11.1. Work done by a gradually applied load. The strain energy per unit volume is often referred to as the resilience. The value of the resilience at the yield point or at the proof stress for non-ferrous materials is then termed the proof resilience. The unshaded area above the line OB of Fig. 11.1 is called the complementary energy, a quantity which is utilised in some advanced energy methods of solution and is not considered within the terms of reference of this text. t 11.1. Strain energy - tension or compression (a) Neglecting the weight of the bar Consider a small element of a bar, length ds, shown in Fig. 11.1. If a graph is drawn of load against elastic extension the shaded area under the graph gives the work done and hence the strain energy, i.e. strain energy U = fP6 Now .. stress P ds strain - A s Young’s modulus E = - - - Pds a=- AE P2ds for the bar element U = __ 2 AE .. L 1E :. total strain energy for a bar of length L = 0 Thus, assuming that the area of the bar remains constant along the length, P2 L u=-- 2AE (11.1) t See H. Ford and J. M. Alexander, Advanced Mechanics of Materials (Longmans, London, 1963)
258 Mechanics of Materials §11.1 or,in terms of the stress a(=P/A), U=gAL。g2 2E=2E×volume of bar (11.2) i.e.strain energy,or resilience,per unit volume of a bar subjected to direct load,tensile or compressive 02 (11.3) 2E or,alternatively, 10 =50X5=0Xe i.e. resilience =stress x strain (b)Including the weight of the bar Consider now a bar of length L mounted vertically,as shown in Fig.11.2.At any section AB the total load on the section will be the external load P together with the weight of the bar material below AB. LEEC666660066602206666 ds Fig.11.2.Direct load-tension or compression. Assuming a uniform cross-section of area A with density p, load on section AB P+pgAs the positive sign being used when P is tensile and the negative sign when P is compressive. Thus,for a tensile force P the extension of the element ds is given by the definition of Young's modulus E to be 6=ads E _P+g4的d5 AE
258 Mechanics of Materials $11.1 or, in terms of the stress o (= P/A), 02AL s2 2E 2E u=- = - x volume of bar (11.2) i.e. strain energy, or resilience, per unit volume of a bar subjected to direct load, tensile or compressive a' 2E =- (11.3) or, alternatively, i.e. resilience = istress x strain (b) Including the weight of the bar Consider now a bar of length L mounted vertically, as shown in Fig. 11.2. At any section AB the total load on the section will be the external load P together with the weight of the bar material below AB. Fig. 11.2. Direct load - tension or compression. Assuming a uniform cross-section of area A with density p, load on section AB = P pgAs the positive sign being used when P is tensile and the negative sign when P is compressive. Thus, for a tensile force P the extension of the element ds is given by the definition of Young's modulus E to be ods 6=- E - (' + 'g As) ds - AE
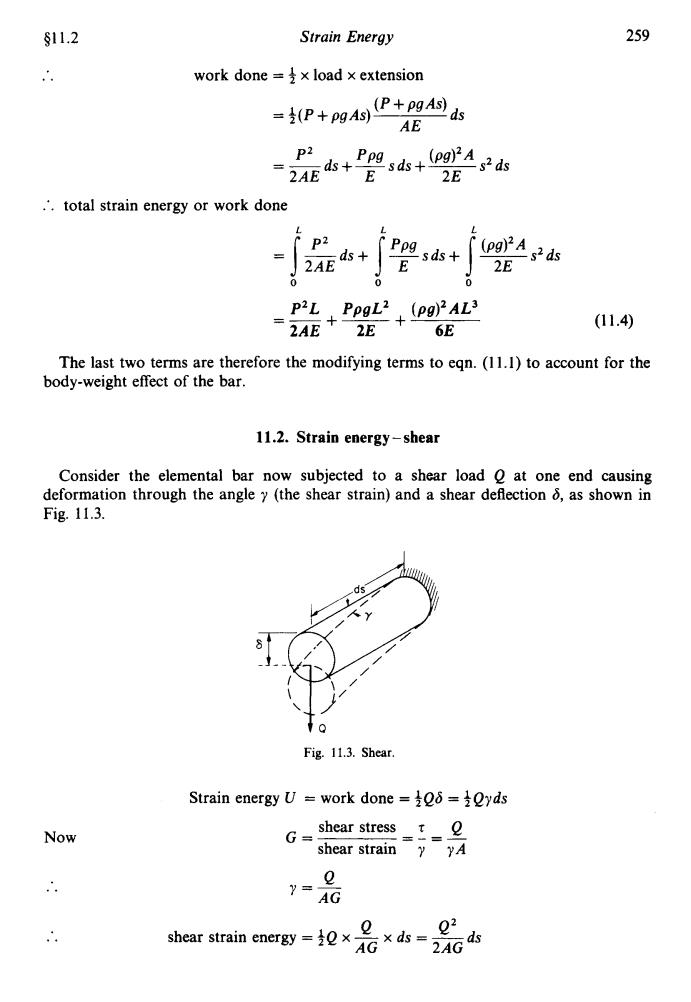
S11.2 Strain Energy 259 work done =x load x extension =5P+pgA的P+pgAd AE P2 2AEdsE = 2E .∴,total strain energy or work done P2 ds+ P sds+ 2AE E 片(p9PAg2as 2E 0 0 P2L PpgL2 (pg)2AL 2AE 2E+ 6E (11.4) The last two terms are therefore the modifying terms to eqn.(11.1)to account for the body-weight effect of the bar. 11.2.Strain energy-shear Consider the elemental bar now subjected to a shear load o at one end causing deformation through the angle y(the shear strain)and a shear deflection as shown in Fg.11.3. ds Fig.11.3.Shear. Strain energy U work done =108=Qyds Now G- shear stress t shear strain y yA y= AG Q2 shear strain energy =x Gx ds =ds
$1 1.2 Strain Energy 259 .. work done = 3 x load x extension :. total strain energy or work done L L L -- p2 ds+ jFsds+ {ws2ds 2AE 2E 0 0 0 (1 1.4) PzL PpgL’ + (pg)’AL3 2AE 2E 6E +--- - -- The last two terms are therefore the modifying terms to eqn. (1 1.1) to account for the body-weight effect of the bar. 11.2. Strain energy-shear Consider the elemental bar now subjected to a shear load Q at one end causing deformation through the angle y (the shear strain) and a shear deflection 6, as shown in Fig. 11.3. Now .. tQ Fig. 11.3. Shear. Strain energy U = work done = 3QS = 3Qyds shear stress t Q shear strain y yA G= =-=- Q y=- AG Q shear strain energy = 3Q x - x ds = ds AG 2AG

260 Mechanics of Materials §11.3 .'total strain energy resulting from shear o'ds Q2L 2AG 2AG (11.5) or,in terms of the shear stresst =(O/A), 2AL t2 U= 2G=2G×volume of bar (11.6) 11.3.Strain energy-bending Let the element now be subjected to a constant bending moment M causing it to bend into an arc of radius R and subtending an angle de at the centre(Fig.11.4).The beam will also have moved through an angle de. .1d8 M R Fig.11.4.Bending. Strain energy work done =x moment x angle turned through (in radians) =是Md0 ME But ds=Rde and T-R do dsM ds R=E团 M2ds strain energy=支Mx M ds= EI 2EI Total strain energy resulting from bending, L M2ds U= (11.7) 2EI
260 Mechanics of Materials 01 1.3 :. total strain energy resulting from shear L or, in terms of the shear stress 5 = (Q/A), T2~~ z2 ty=-- -- x volume of bar 2G 2G (11.5) (11.6) 11.3. Strain energy -bending Let the element now be subjected to a constant bending moment M causing it to bend into an arc of radius R and subtending an angle de at the centre (Fig. 11.4). The beam will also have moved through an angle de. M \ I But Fig. 11.4. Bending. Strain energy = work done = x moment x angle turned through (in radians) = $ Md0 ME ds = Rd0 and -=- IR M M’ds strain energy = 3M x -ds = ~ EI 2EI ... Total strain energy resulting from bending, (11.7)
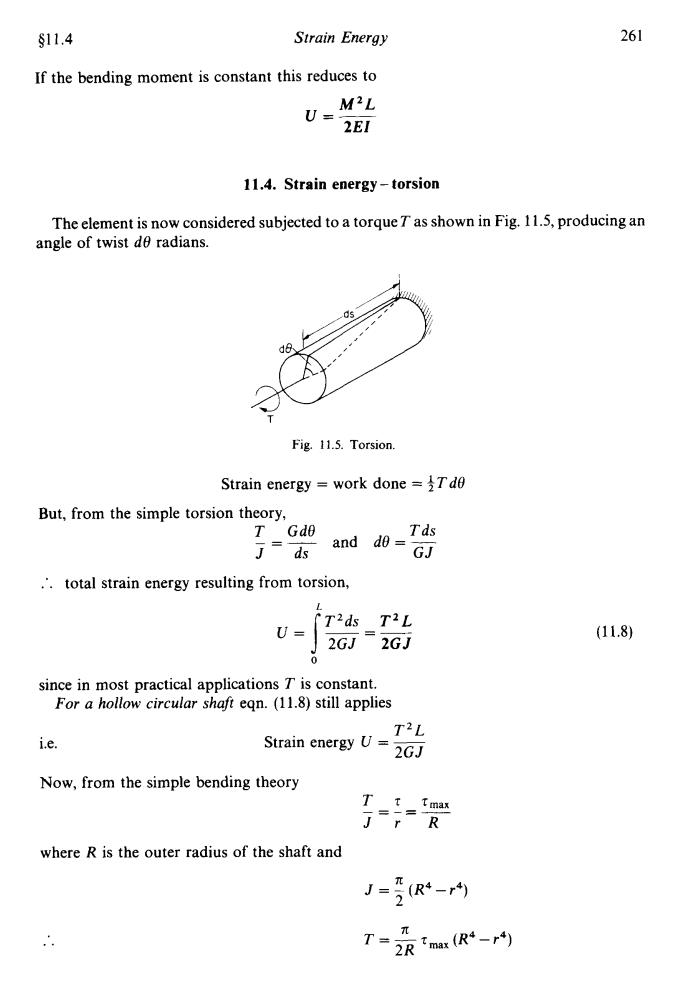
§11.4 Strain Energy 261 If the bending moment is constant this reduces to M2L U= 2EI 11.4.Strain energy-torsion The element is now considered subjected to a torque T as shown in Fig.11.5,producing an angle of twist de radians. ds de., Fig.11.5.Torsion. Strain energy work done =iTde But,from the simple torsion theory, T Gde Tds Jds and do= GJ .'total strain energy resulting from torsion, CT2ds T2L U= (11.8) 2GJ 2GJ since in most practical applications T is constant. For a hollow circular shaft eqn.(11.8)still applies T2L i.e. Strain energy U=2GJ Now,from the simple bending theory Tt Tmax 了,=R where R is the outer radius of the shaft and J= 5(R4-r4) π T-2Rtma (R)
91 1.4 Strain Energy 26 1 If the bending moment is constant this reduces to 11.4. Strain energy - torsion The element is now considered subjected to a torque T as shown in Fig. 11.5, producing an angle of twist dO radians. Fig. 11.5. Torsion. Strain energy = work done = 3TdO But, from the simple torsion theory, Tds and dO =- T GdO J ds GJ - .'. total strain energy resulting from torsion, T2ds T2L 2GJ 2GJ 0 since in most practical applications T is constant. For a hollow circular shaji eqn. (1 1.8) still applies T~L Strain energy U = - 2GJ i.e. Now, from the simple bending theory T 7 Tmax - - -- -- JrR where R is the outer radius of the shaft and 7t J=-(R4-r4) 2 (11.8)

262 Mechanics of Materials $11.5 Substituting in the strain energy equation(11.8)we have: (R)L 2R U 20a*-r内 txπ(R4-r)L = 4G R2 []x volume of shaft 4G R2 Strain energy/unit volume= tx [R2+r2] or 4G R2 (11.8a) It should be noted that in the four types of loading case considered above the strain energy expressions are all identical in form, (applied“load")2×L i.e. strain energy Uxproduct of two related constants the constants being related to the type of loading considered.In bending,for example,the relevant constants which appear in the bending theory are Eand I,whilst for torsion Gand J are more applicable.Thus the above standard equations for strain energy should easily be remembered. 11.5.Strain energy of a three-dimensional principal stress system The reader is referred to $14.17 for the derivation of the following expression for the strain energy of a system of three principal stresses: 1 V=2E[i+ai+号-2w(a1o+a+o3i】per unit volume It is then shown in $14.17 that this total strain energy can be conveniently considered as made up of two parts: (a)the volumetric or dilatational strain energy; (b)the shear or distortional strain energy. 11.6.Volumetric or dilatational strain energy This is the strain energy associated with a mean or hydrostatic stress of (+2+3)=actingequally in all three mutually perpendicular directions giving rise to no distortion,merely a change in volume. Then from eqn.(14.22), volumetric strain energy-[(1++03)2]per unit volume
262 Mechanics of Materials $1 1.5 Substituting in the strain energy equation (1 1.8) we have: [%(R4-r4)] 2 L U= x 2G-(R4-r4) zLx z(R4 - r4)L 4G R2 2 -- tLx [Rz +r2] 4G R2 -- x volume of shaft or zLax [R2 +r2] 4G R2 Strain energy/unit volume = - (11.8a) It should be noted that in the four types of loading case considered above the strain energy expressions are all identical in form, i.e. (applied “load”)’ x L 2 x product of two related constants strain energy U = the constants being related to the type of loading considered. In bending, for example, the relevant constants which appear in the bending theory are E and I, whilst for torsion G and J are more applicable. Thus the above standard equations for strain energy should easily be remembered. 11.5. Strain energy of a three-dimensional principal stress system The reader is referred to $14.17 for the derivation of the following expression for the strain energy of a system of three principal stresses: v=-[ a: + ai + 63 - 2v(ala2 + a263 + a3a1)] per unit volume 1 2E It is then shown in $14.17 that this total strain energycan beconvenientlyconsidered as made up of two parts: (a) the volumetric or dilatational strain energy; (b) the shear or distortional strain energy. 11.6. Volumetric or dilatational strain energy This is the strain energy associated with a mean or hydrostatic stress of $(a, + o2 + os) = 0 acting equally in all three mutually perpendicular directions giving rise to no distortion, merely a change in volume. Then from eqn. (14.22), (1 - 2v) volumetric strain energy = ___ [(al + a2 + a3)’] per unit volume 6E
S11.7 Strain Energy 263 11.7.Shear or distortional strain energy In order to consider the general principal stress case it has been shown necessary,in $14.6, to add to the mean stress in the three perpendicular directions,certain so-called deviatoric stress values to return the stress system to values of o,o2 and o3.These deviatoric stresses are then associated directly with change of shape,i.e.distortion,without change in volume and the strain energy associated with this mechanism is shown to be given by shear strain energy-2[(()]per unit volume 6G[oi+吃+of-(a12+a2o3+a3o,】per unit volume This equation is used as the basis of the Maxwell-von Mises theory of elastic failure which is discussed fully in Chapter 15. 11.8.Suddenly applied loads If a load Pis applied gradually to a bar to produce an extension the load-extension graph will be as shown in Fig.11.1 and repeated in Fig.11.6,the work done being given by U=P6. Lood Work done gradually applied load) .P8 Work done suddenly applied load) Extension P‘8 Fig.11.6.Work done by a suddenly applied load. If now a load P'is suddenly applied(i.e.applied with an instantaneous value,not gradually increasing from zero to P')to produce the same extension o,the graph will now appear as a horizontal straight line with a work done or strain energy =P'8. The bar will be strained by an equal amount o in both cases and the energy stored must therefore be equal, i.e. P'δ=支P8 or P=P Thus the suddenly applied load which is required to produce a certain value of instantaneous strain is half the equivalent value of static load required to perform the same function.It is then clear that vice versa a load P which is suddenly applied will produce twice the effect of the same load statically applied.Great care must be exercised,therefore,in the design
$11.7 Strain Energy 263 11.7. Shear or distortional strain energy In order to consider the general principal stress case it has been shown necessary, in 5 14.6, to add to the mean stress 5 in the three perpendicular directions, certain so-called deviatoric stress values to return the stress system to values of al, a’ and a3. These deuiatoric stresses are then associated directly with change of shape, i.e. distortion, without change in volume and the strain energy associated with this mechanism is shown to be given by 1 12G 1 6G shear strain energy = __ [(a, - a’)’ + (a2 - a3)’ + (a3 - ol)’] = - [u: + u: + t~: - (al u2 + u2 uj + uj ul )] per unit volume This equation is used as the basis of the Maxwell-von Mises theory of elastic failure which is discussed fully in Chapter 15. per unit volume 11.8. Suddenly applied loads If a load Pis applied gradually to a bar to produce an extension 6 the load-extension graph will be as shown in Fig. 11.1 and repeated in Fig. 11.6, the work done being given by u = iP6. Fig. 11.6. Work done by a suddenly applied load. If now a load P’ is suddenly applied (i.e. applied with an instantaneous value, not gradually increasing from zero to P’) to produce the same extension 6, the graph will now appear as a horizontal straight line with a work done or strain energy = P‘6. The bar will be strained by an equal amount 6 in both cases and the energy stored must therefore be equal, i.e. P’6 = 3P6 or p’ = &p Thus the suddenly applied load which is required to produce a certain value of instantaneous strain is half the equivalent value of static load required to perform the same function. It is then clear that vice versa a load P which is suddenly applied will produce twice the effect of the same load statically applied. Great care must be exercised, therefore, in the design