
2011 Semifinal Exam AAPT UNITEDSTATES PHYSICS TEAM AIP 2011 Semifinal Exam DO NOT DISTRIBUTE THIS PAGE Important Instructions for the Exam Supervisor This examination consists of two parts. Part A has four questions and is allowed 90 minutes. Part B has two questions and is allowed 90 minutes. The first page that follows is a cover sheet.Examinees may keep the cover sheet for both parts of the exam. The parts are then identified by the center header on each page.Examinees are only allowed to do one part at a time,and may not work on other parts,even if they have time remaining. .Allow 90 minutes to complete Part A.Do not let students look at Part B.Collect the answers to Part A before allowing the examinee to begin Part B.Examinees are allowed a 10 to 15 minutes break between parts A and B. Allow 90 minutes to complete Part B.Do not let students go back to Part A. Ideally the test supervisor will divide the question paper into 3 parts:the cover sheet(page 2),Part A(pages 3-4),and Part B(pages 6-7).Examinees should be provided parts A and B individually,although they may keep the cover sheet. The supervisor must collect all examination questions,including the cover sheet,at the end of the exam,as well as any scratch paper used by the examinees.Examinees may not take the exam questions.The examination questions may be returned to the students after March 31,2011. Examinees are allowed calculators,but they may not use symbolic math,programming,or graphic features of these calculators.Calculators may not be shared and their memory must be cleared of data and programs.Cell phones,PDA's or cameras may not be used during the exam or while the exam papers are present.Examinees may not use any tables,books, or collections of formulas. Please provide the examinees with graph paper for Part A. Copyright C2011 American Association of Physics Teachers

2011 Semifinal Exam Cover Sheet 2 AAPT UNITED STATES PHYSICS TEAM AIP 2011 Semifinal Exam INSTRUCTIONS DO NOT OPEN THIS TEST UNTIL YOU ARE TOLD TO BEGIN Work Part A first.You have 90 minutes to complete all four problems.Each question is worth 25 points.Do not look at Part B during this time. After you have completed Part A you may take a break. Then work Part B.You have 90 minutes to complete both problems.Each question is worth 50 points.Do not look at Part A during this time. Show all your work.Partial credit will be given.Do not write on the back of any page.Do not write anything that you wish graded on the question sheets. Start each question on a new sheet of paper.Put your AAPT ID number,your name,the question number and the page number/total pages for this problem,in the upper right hand corner of each page.For example, AAPT ID# Doe,Jamie A1-1/3 A hand-held calculator may be used.Its memory must be cleared of data and programs.You may use only the basic functions found on a simple scientific calculator.Calculators may not be shared.Cell phones,PDA's or cameras may not be used during the exam or while the exam papers are present.You may not use any tables,books,or collections of formulas. Questions with the same point value are not necessarily of the same difficulty. In order to maintain exam security,do not communicate any information about the questions(or their answers/solutions)on this contest until after April 1,2011. Possibly Useful Information.You may use this sheet for both parts of the exam. g=9.8N/kg G=6.67×10-11N·m2/kg2 k=1/4re0=8.99×109N.m2/C2 km=4o/4r=10-7T.m/A c=3.00×108m/s B=1.38×1023J/K NA=6.02×1023(mol)-1 R=NAkB =8.31 J/(mol.K) σ=5.67×10-8J/(s·m2.K4) e=1.602×10-19C 1eV=1.602×10-19J h=6.63×10-34J·s=4.14×10-15eV.s me=9.109×10-31kg=0.511MeV/c2 (1+x)n≈1+nx for x<1 sin0≈0-303forl0<1 cos9≈1-号82forl9l<1 Copyright C2011 American Association of Physics Teachers

2011 Semifinal Exam Part A 3 Part A Question A1 Single bubble sonoluminescence occurs when sound waves cause a bubble suspended in a fluid to collapse so that the gas trapped inside increases in temperature enough to emit light.The bubble actually undergoes a series of expansions and collapses caused by the sound wave pressure variations. We now consider a simplified model of a bubble undergoing sonoluminescence.Assume the bub- ble is originally at atmospheric pressure Po=101 kPa.When the pressure in the fluid surrounding the bubble is decreased,the bubble expands isothermally to a radius of 36.0 im.When the pressure increases again,the bubble collapses to a radius of 4.50 um so quickly that no heat can escape. Between the collapse and subsequent expansion,the bubble undergoes isochoric (constant volume) cooling back to its original pressure and temperature.For a bubble containing a monatomic gas, suspended in water of T=293 K,find a.the number of moles of gas in the bubble, b.the pressure after the expansion, c.the pressure after collapse, d.the temperature after the collapse,and e.the total work done on the bubble during the whole process. You may find the following useful:the specific heat capacity at constant volume is Cy=3R/2 and the ratio of specific heat at constant pressure to constant volume is y=5/3 for a monatomic gas. Question A2 A thin,uniform rod of length L and mass M =0.258 kg is suspended from a point a distance R away from its center of mass.When the end of the rod is displaced slightly and released it executes simple harmonic oscillation.The period,T,of the oscillation is timed using an electronic timer. The following data is recorded for the period as a function of R.What is the local value of g?Do not assume it is the canonical value of 9.8 m/s2.What is the length,L,of the rod?No estimation of error in either value is required.The moment of inertia of a rod about its center of mass is (1/12)ML2 R T R T (m) (s) (m) (s) 0.050 3.842 0.211 2.074 0.075 3.164 0.302 1.905 0.102 2.747 0.387 1.855 0.156 2.301 0.451 1.853 0.198 2.115 0.588 1.900 You must show your work to obtain full credit.If you use graphical techniques then you must plot the graph;if you use linear regression techniques then you must show all of the formulae and associated workings used to obtain your result. Copyright C2011 American Association of Physics Teachers

2011 Semifinal Exam Part A 4 Question A3 A light bulb has a solid cylindrical filament of length L and radius a,and consumes power P. You are to design a new light bulb,using a cylindrical filament of the same material,operating at the same voltage,and emitting the same spectrum of light,which will consume power nP.What are the length and radius of the new filament?Assume that the temperature of the filament is approximately uniform across its cross-section;the filament doesn't emit light from the cnds:and energy loss due to convection is minimal. Question A4 In this problem we consider a simplified model of the electromagnetic radiation inside a cubical box of side length L.In this model,the electric field has spatial dependence E(,y,2)=Eosin(kz)sin(kuy)sin(kz2) where one corner of the box lies at the origin and the box is aligned with the a,y,and z axes.Let h be Planck's constant,kB be Boltzmann's constant,and c be the speed of light. a.The electric field must be zero everywhere at the sides of the box.What condition does this impose on k,ky,and k2?(Assume that any of these may be negative,and include cases where one or more of the ki is zero,even though this causes E to be zero.) b.In the model,each permitted value of the triple (k,ky,k)corresponds to a quantum state. These states can be visualized in a state space,which is a notional three-dimensional space with axes corresponding to kz,ky,and kz.How many states occupy a volume s of state space, if s is large enough that the discreteness of the states can be ignored? c.Each quantum state,in turn,may be occupied by photons with frequencyw-ck, f where kl=Vka2+ky2+kz2 In the model,if the temperature inside the box is T,no photon may have energy greater than kBT.What is the shape of the region in state space corresponding to occupied states? d.As a final approximation,assume that each occupied state contains exactly one photon.What is the total energy of the photons in the box,in terms of h,kB,c,T,and the volume of the box V?Again,assume that the temperature is high enough that there are a very large number of occupied states.(Hint:divide state space into thin regions corresponding to photons of the same energy. Note that while many details of this model are extremely inaccurate,the final result is correct except for a numerical factor. Copyright C2011 American Association of Physics Teachers

2011 Semifinal Exam Part A STOP:Do Not Continue to Part B If there is still time remaining for Part A,you should review your work for Part A,but do not continue to Part B until instructed by your exam supervisor. Copyright C2011 American Association of Physics Teachers

2011 Semifinal Exam Part B Part B Question B1 An AC power line cable transmits electrical power using a sinusoidal waveform with frequency 60 Hz.The load receives an RMS voltage of 500 kV and requires 1000 MW of average power. For this problem,consider only the cable carrying current in one of the two directions,and ignore effects due to capacitance or inductance between the cable and with the ground. a.Suppose that the load on the power line cable is a residential area that behaves like a pure resistor. i.What is the RMS current carried in the cable? ii.The cable has diameter 3 cm,is 500 km long,and is made of aluminum with resistivity 2.8 x 10-8 n.m.How much power is lost in the wire? b.A local rancher thinks he might be able to extract electrical power from the cable using electromagnetic induction.The rancher constructs a rectangular loop of length a and width b<a,consisting of N turns of wire.One edge of the loop is to be placed on the ground;the wire is straight and runs parallel to the ground at a height h much less than the length of the wire.Write the current in the wire as I =Io sinwt,and assume the return wire is far away. i.Determine an expression for the magnitude of the magnetic field at a distance r from the power line cable in terms of I,r,and fundamental constants. ii.Where should the loop be placed,and how should it be oriented,to maximize the induced emf in the loop? iii.Assuming the loop is placed in this way,determine an expression for the emf induced in the loop (as a function of time)in terms of any or all of lo,h,a,b,N,w,t,and fundamental constants. iv.Suppose that a =5 m,b=2 m,and h=100 m.How many turns of wire N does the rancher need to generate an RMS emf of 120 V? c.The load at the end of the power line cable changes to include a manufacturing plant with a large number of electric motors.While the average power consumed remains the same,it now behaves like a resistor in parallel with a 0.25 H inductor. i.Does the power lost in the power line cable increase,decrease,or stay the same?(You need not calculate the new value explicitly,but you should show some work to defend your answer.) ii.The power company wishes to make the load behave as it originally did by installing a capacitor in parallel with the load.What should be its capacitance? Copyright C2011 American Association of Physics Teachers
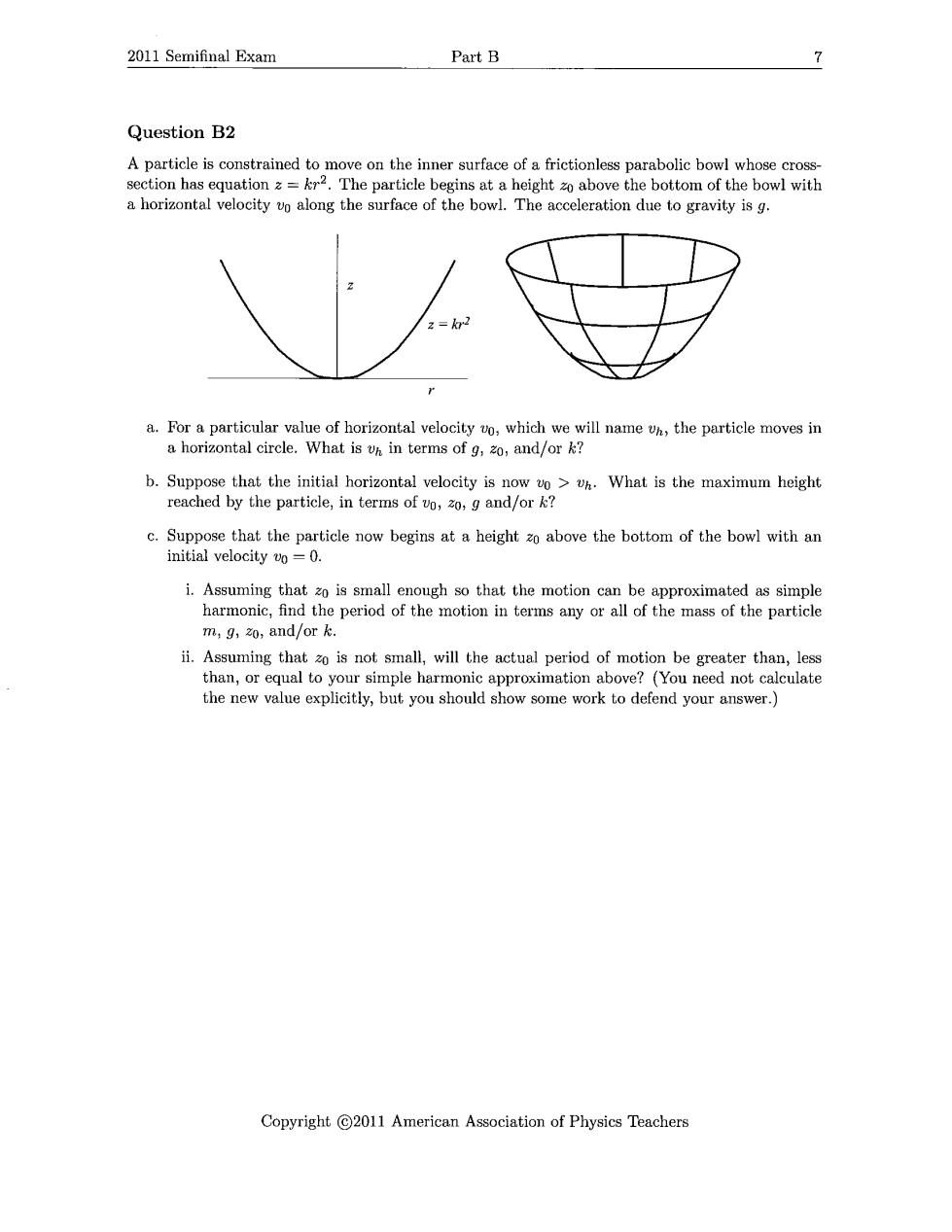
2011 Semifinal Exam Part B 7 Question B2 A particle is constrained to move on the inner surface of a frictionless parabolic bowl whose cross- section has equation z=kr2.The particle begins at a height zo above the bottom of the bowl with a horizontal velocity vo along the surface of the bowl.The acceleration due to gravity is g. a.For a particular value of horizontal velocity vo,which we will name uh,the particle moves in a horizontal circle.What is vn in terms of g,zo,and/or k? b.Suppose that the initial horizontal velocity is now vo >Uh.What is the maximum height reached by the particle,in terms of vo,z0,g and/or k? c.Suppose that the particle now begins at a height zo above the bottom of the bowl with an initial velocity vo =0. i.Assuming that zo is small enough so that the motion can be approximated as simple harmonic,find the period of the motion in terms any or all of the mass of the particle m,g,20,and/or k. ii.Assuming that zo is not small,will the actual period of motion be greater than,less than,or equal to your simple harmonic approximation above?(You need not calculate the new value explicitly,but you should show some work to defend your answer.) Copyright C2011 American Association of Physics Teachers