Principles of Quantum Computation
Principles of Quantum Computation

Quantum Computation From Wikipedia Quantum computing is the use of quantum-mechanical phenomena such as superposition and entanglement to perform computation.A quantum computer is used to perform such computation,which can be implemented theoretically or physically
Quantum Computation From Wikipedia Quantum computing is the use of quantum-mechanical phenomena such as superposition and entanglement to perform computation. A quantum computer is used to perform such computation, which can be implemented theoretically or physically
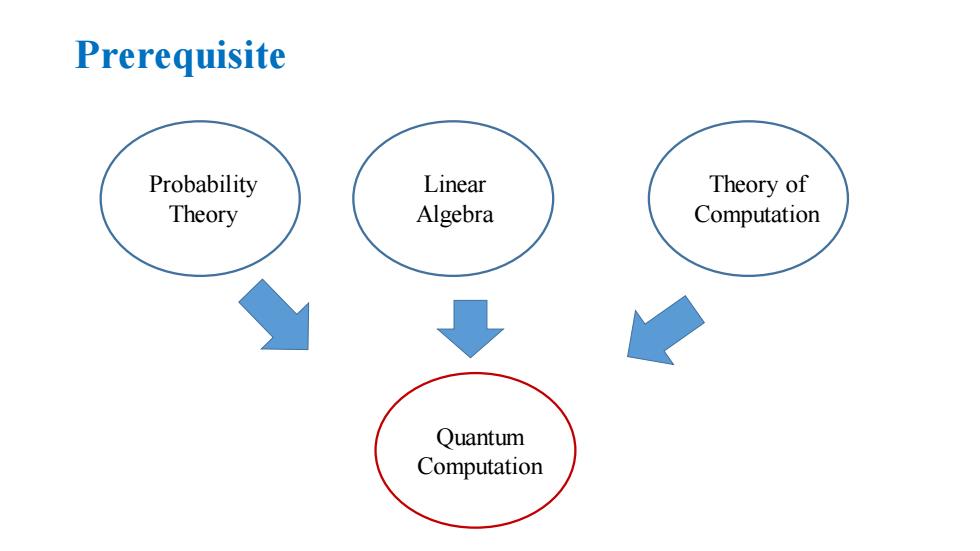
Prerequisite Probability Linear Theory of Theory Algebra Computation Quantum Computation
Prerequisite Theory of Computation Quantum Computation Linear Algebra Probability Theory

Prerequisite Probability Linear Theory of Theory Algebra Computation Quantum Computation
Prerequisite Theory of Computation Quantum Computation Linear Algebra Probability Theory
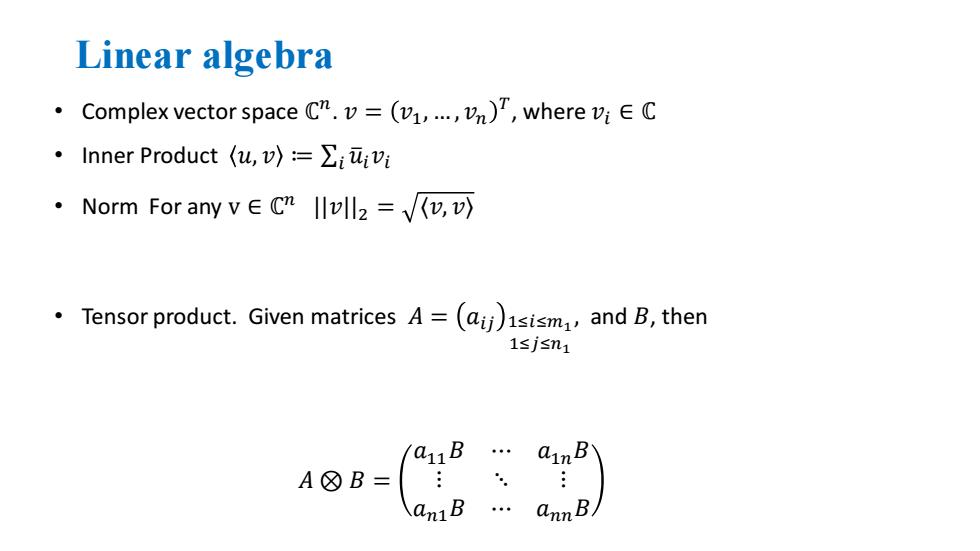
Linear algebra ·Complex vector space CT.v=(,n)T,where vi∈C ·Inner Product(u,)=iuvi ·Norm For any v E CTlv2=√(,) Tensor product.Given matrices A=(aij)1sism,and B,then 1≤j≤n1 /a11B 4◆ ainB A☒B= aniB … annB/
Linear algebra • Complex vector space ℂ 𝑛 . 𝑣 = 𝑣1 , … , 𝑣𝑛 𝑇 , where 𝑣𝑖 ∈ ℂ • Inner Product 𝑢, 𝑣 ≔ σ𝑖 𝑢ത𝑖𝑣𝑖 • Norm For any v ∈ ℂ 𝑛 ||𝑣||2 = ⟨𝑣, 𝑣⟩ • Tensor product. Given matrices 𝐴 = 𝑎𝑖𝑗 1≤𝑖≤𝑚1 1≤𝑗≤𝑛1 , and 𝐵, then 𝐴 ⊗ 𝐵 = 𝑎11𝐵 ⋯ 𝑎1𝑛𝐵 ⋮ ⋱ ⋮ 𝑎𝑛1𝐵 ⋯ 𝑎𝑛𝑛𝐵
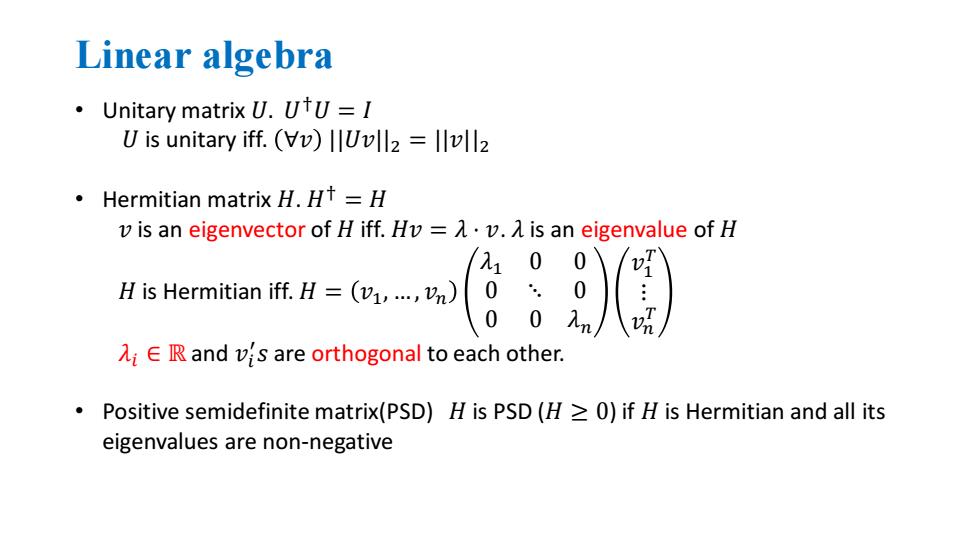
Linear algebra Unitary matrix U.UTU =I U is unitary iff.(V)2=2 Hermitian matrix H.H =H v is an eigenvector of H iff.Hv=1.v.A is an eigenvalue of H 九1 0 0 Hermiian-8置2 Ai E Rand vs are orthogonal to each other. Positive semidefinite matrix(PSD)H is PSD (H>0)if H is Hermitian and all its eigenvalues are non-negative
Linear algebra • Unitary matrix 𝑈. 𝑈 †𝑈 = 𝐼 𝑈 is unitary iff. ∀𝑣 ||𝑈𝑣||2 = ||𝑣||2 • Hermitian matrix 𝐻. 𝐻 † = 𝐻 𝑣 is an eigenvector of 𝐻 iff. 𝐻𝑣 = 𝜆 ⋅ 𝑣. 𝜆 is an eigenvalue of 𝐻 𝐻 is Hermitian iff. 𝐻 = 𝑣1 , … , 𝑣𝑛 𝜆1 0 0 0 ⋱ 0 0 0 𝜆𝑛 𝑣1 𝑇 ⋮ 𝑣𝑛 𝑇 𝜆𝑖 ∈ ℝ and 𝑣𝑖 ′ 𝑠 are orthogonal to each other. • Positive semidefinite matrix(PSD) 𝐻 is PSD (𝐻 ≥ 0) if 𝐻 is Hermitian and all its eigenvalues are non-negative
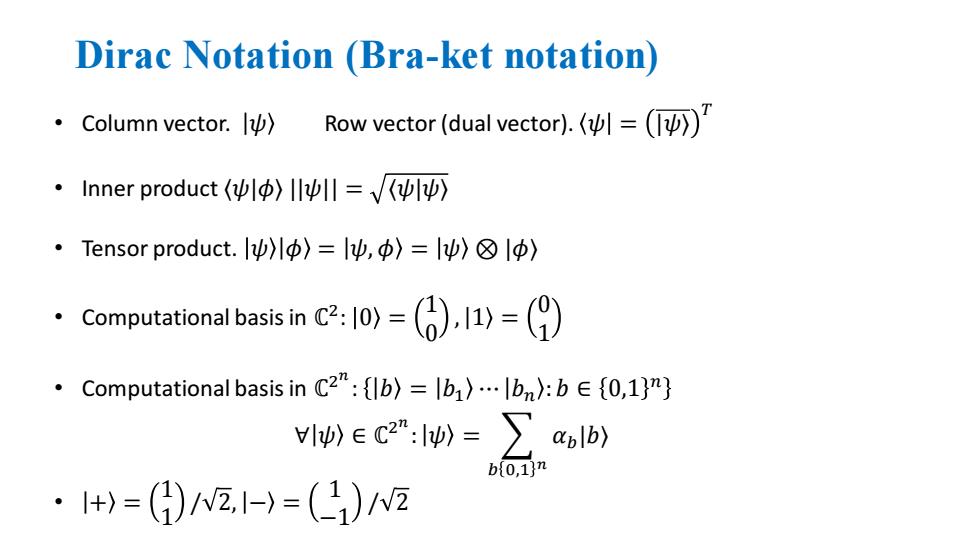
Dirac Notation (Bra-ket notation) ·Column vector..ly) Row vector(dual vector).(= ·Inner product(lp)lll=√lp) ·Tensor product.l)川p)=l中,φ〉=lψ〉☒lφ) .Computational basis in 2:=(=0 Computational basis in C2":lb〉=lb1)…lbn:b∈{0,1}ry lp)∈c2”:I〉=>ab) b0,1]n ·+)=()W2,-)=(1)N2
Dirac Notation (Bra-ket notation) • Column vector. 𝜓 Row vector (dual vector). 𝜓 = |𝜓⟩ 𝑇 • Inner product ⟨𝜓|𝜙⟩ ||𝜓|| = ⟨𝜓|𝜓⟩ • Tensor product. 𝜓 𝜙 = 𝜓,𝜙 = 𝜓 ⊗ |𝜙⟩ • Computational basis in ℂ 2 : 0 = 1 0 , 1 = 0 1 • Computational basis in ℂ 2 𝑛 : 𝑏 = 𝑏1 ⋯ 𝑏𝑛 : 𝑏 ∈ 0,1 𝑛 ∀ 𝜓 ∈ ℂ 2 𝑛 : 𝜓 = 𝑏 0,1 𝑛 𝛼𝑏 |𝑏⟩ • + = 1 1 / 2, − = 1 −1 / 2
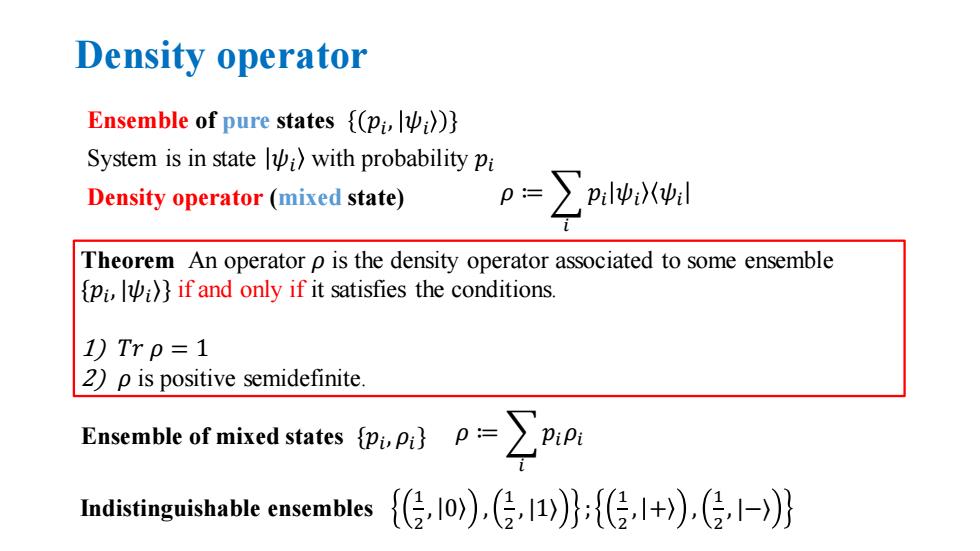
Density operator Ensemble of pure states {(pi,)} System is in state)with probability pi Density operator (mixed state) iv:wil Theorem An operator p is the density operator associated to some ensemble pi,i)}if and only if it satisfies the conditions. 1)Trp=1 2)p is positive semidefinite. Easemble of mixed states Indistinguishable ensembles {1o),(任11}:(经,+).(经1-}
Density operator Ensemble of pure states 𝑝𝑖 , 𝜓𝑖 } Density operator (mixed state) Theorem An operator 𝜌 is the density operator associated to some ensemble {𝑝𝑖 , |𝜓𝑖 ⟩} if and only if it satisfies the conditions. 1) 𝑇𝑟 𝜌 = 1 2) 𝜌 is positive semidefinite. Ensemble of mixed states {𝑝𝑖 , 𝜌𝑖 } 𝜌 ≔ 𝑖 𝑝𝑖𝜌𝑖 𝜌 ≔ 𝑖 𝑝𝑖 𝜓𝑖 𝜓𝑖 System is in state 𝜓𝑖 with probability 𝑝𝑖 Indistinguishable ensembles 1 2 , 0 , 1 2 , |1⟩ ; 1 2 , + , 1 2 , |−⟩
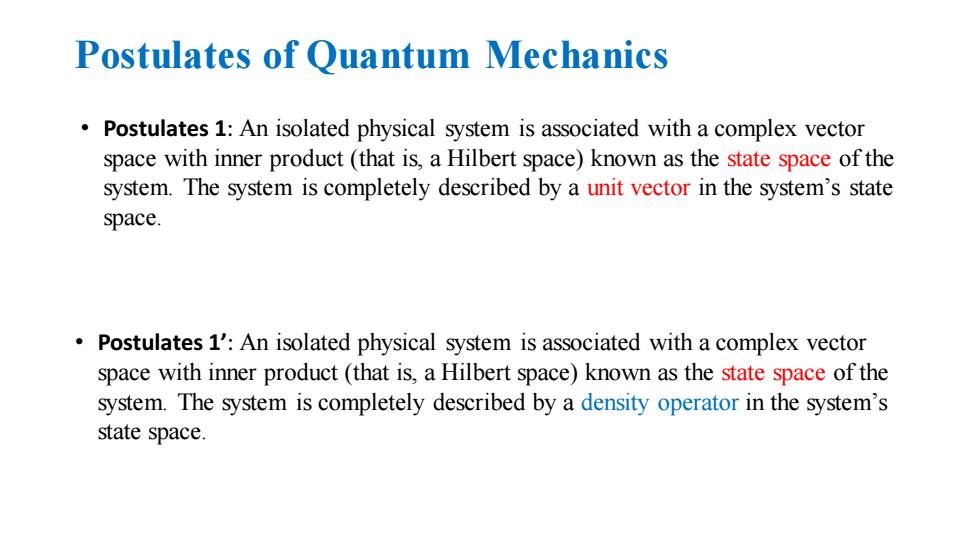
Postulates of Quantum Mechanics Postulates 1:An isolated physical system is associated with a complex vector space with inner product(that is,a Hilbert space)known as the state space of the system.The system is completely described by a unit vector in the system's state space. Postulates 1':An isolated physical system is associated with a complex vector space with inner product(that is,a Hilbert space)known as the state space of the system.The system is completely described by a density operator in the system's state space
Postulates of Quantum Mechanics • Postulates 1: An isolated physical system is associated with a complex vector space with inner product (that is, a Hilbert space) known as the state space of the system. The system is completely described by a unit vector in the system’s state space. • Postulates 1’: An isolated physical system is associated with a complex vector space with inner product (that is, a Hilbert space) known as the state space of the system. The system is completely described by a density operator in the system’s state space
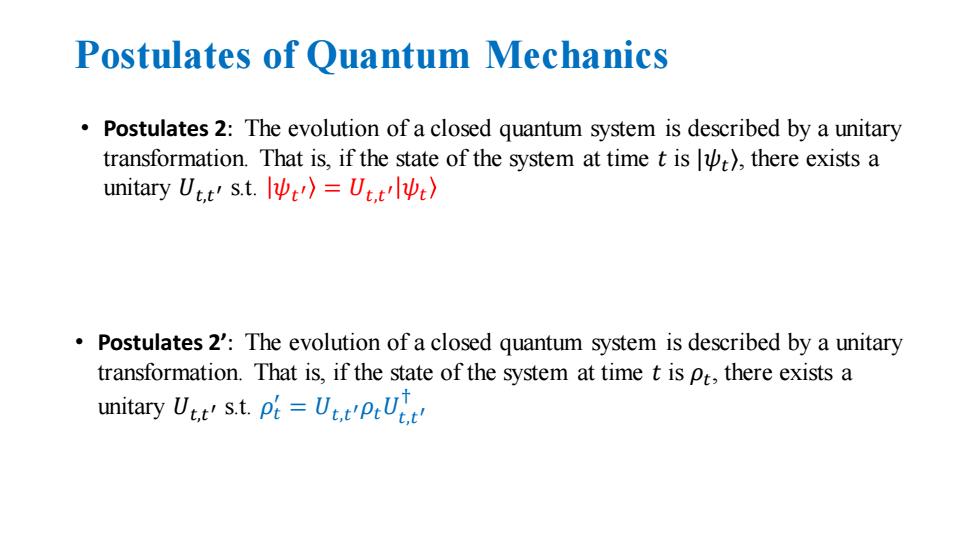
Postulates of Quantum Mechanics Postulates 2:The evolution of a closed quantum system is described by a unitary transformation.That is,if the state of the system at time t ist),there exists a unitary Utt's.t.=U) Postulates 2':The evolution of a closed quantum system is described by a unitary transformation.That is,if the state of the system at time t is pt,there exists a uitary Us.t.P=UPU
Postulates of Quantum Mechanics • Postulates 2: The evolution of a closed quantum system is described by a unitary transformation. That is, if the state of the system at time 𝑡 is |𝜓𝑡 ⟩, there exists a unitary 𝑈𝑡,𝑡 ′ s.t. 𝜓𝑡 ′ = 𝑈𝑡,𝑡 ′ 𝜓𝑡 • Postulates 2’: The evolution of a closed quantum system is described by a unitary transformation. That is, if the state of the system at time 𝑡 is 𝜌𝑡 , there exists a unitary 𝑈𝑡,𝑡 ′ s.t. 𝜌𝑡 ′ = 𝑈𝑡,𝑡 ′𝜌𝑡𝑈𝑡,𝑡 ′ †