上海交通大学：《热力学 Thermodynamics（I）》课程教学资源（课件讲义）Lecture 15_Polytropic process

上游充通大￥ SHANGHAI JIAO TONG UNIVERSITY Engineering Thermodynamics I Lectures 15 Spring,2017 Prof.,Dr.Yonghua HUANG R几L E http://cc.sjtu.edu.cn/G2S/site/thermo.html 1日G

Engineering Thermodynamics I Lectures 15 Spring, 2017 Prof., Dr. Yonghua HUANG http://cc.sjtu.edu.cn/G2S/site/thermo.html

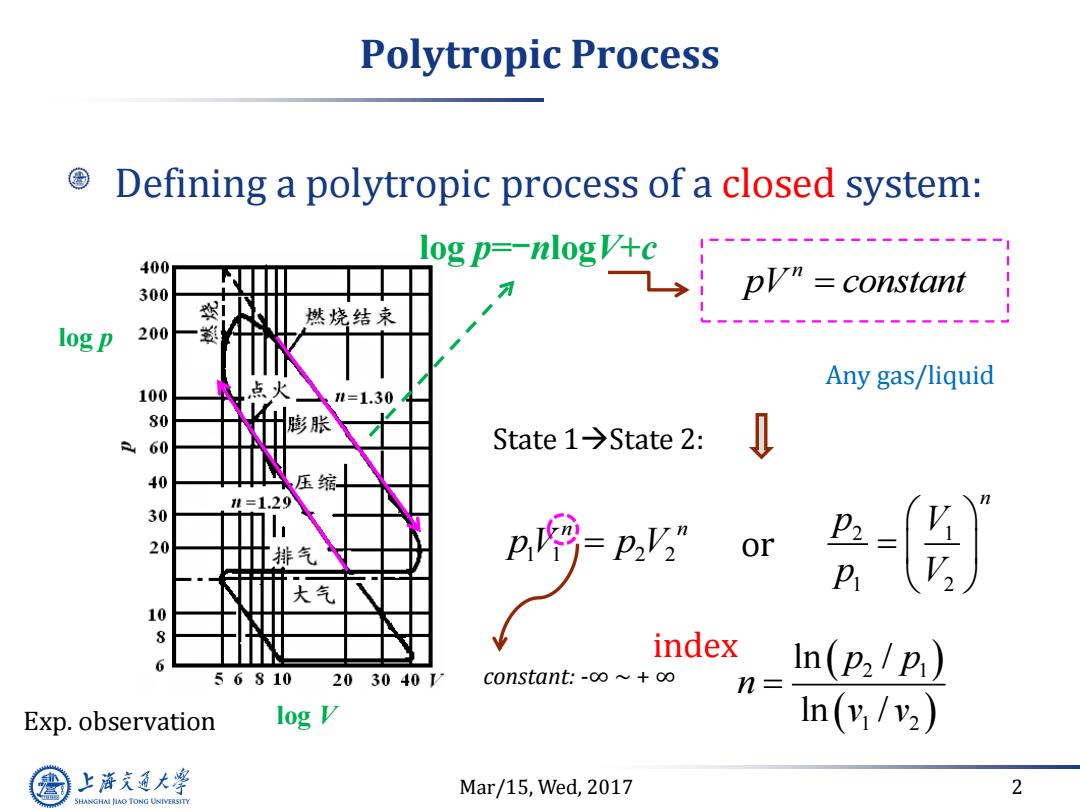
Polytropic Process Defining a polytropic process of a closed system: 400 300 燃烧结束 sgpr一nogvse ph=cnsn log p 200 点火 Any gas/liquid 100 l=1.30 膨胀 State1→State2: 40 压缩 =1.29 30 20 排气 or 大气 10 8 index 6 In(p2/p) 56810 203040V constant.-o∞~+o∞ n= Exp.observation log V In(v/v2) 上游充通大 Mar/15,Wed,2017 2 SHANGHAI JIAO TONG UNIVERSITY

Mar/15, Wed, 2017 2 Polytropic Process Defining a polytropic process of a closed system: n pV constant  constant: -∞ ~ + ∞ State 1State 2: 1 1 2 2 n n pV p V  or 2 1 1 2 n p V p V        log p Exp. observation log V     2 1 1 2 ln / ln / p p n v v  Any gas/liquid log p=-nlogV+c index

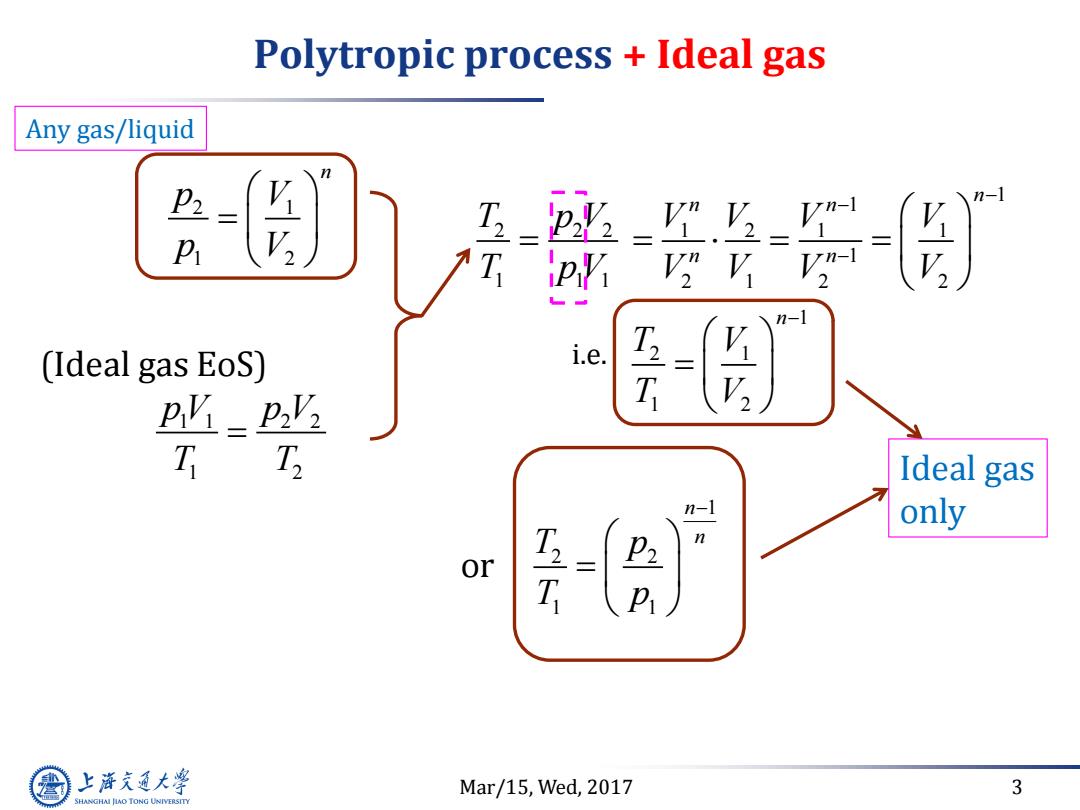
Polytropic process Ideal gas Any gas/liquid P (Ideal gas EoS) i.e. py pvz T Ideal gas n-1 only 27 n or P 上游究大学 Mar/15,Wed,2017 3 SHANGHAI JLAO TONG UNIVERSITY

Mar/15, Wed, 2017 3 Polytropic process + Ideal gas 1 1 2 2 1 2 p V p V T T  (Ideal gas EoS) 2 2 2 1 1 1 T p V T p V  1 2 2 1 1 n n T p T p         2 1 1 2 n p V p V        or Ideal gas only 1 1 1 2 1 1 1 2 1 2 2 n n n n n V V V V V V V V              Any gas/liquid 1 2 1 1 2 n T V T V         i.e

Four particular cases n=0 p =const. (isobaric process;constant pressure process) n=1 pv =const. (isothermal process;constant temperature process) p"=p2V2” n=K pv*=const. (isentropic process;reversible adiabatic process) For ideal gas:adiabatic index K =k(specific heat ratio) n=士o∞ V=const. (isometric/isochoric process;constant volume process) 上游充通大学 Mar/15,Wed,2017 4 SHANGHAI JLAO TONG UNIVERSITY

Mar/15, Wed, 2017 4 Four particular cases 1 1 2 2 n n pV p V  n p   0 const. n pv   1 const. n pv const.     n v   const. (isobaric process; constant pressure process) (isometric/isochoric process; constant volume process) (isentropic process; reversible adiabatic process) (isothermal process; constant temperature process) For ideal gas: adiabatic index = k (specific heat ratio) 

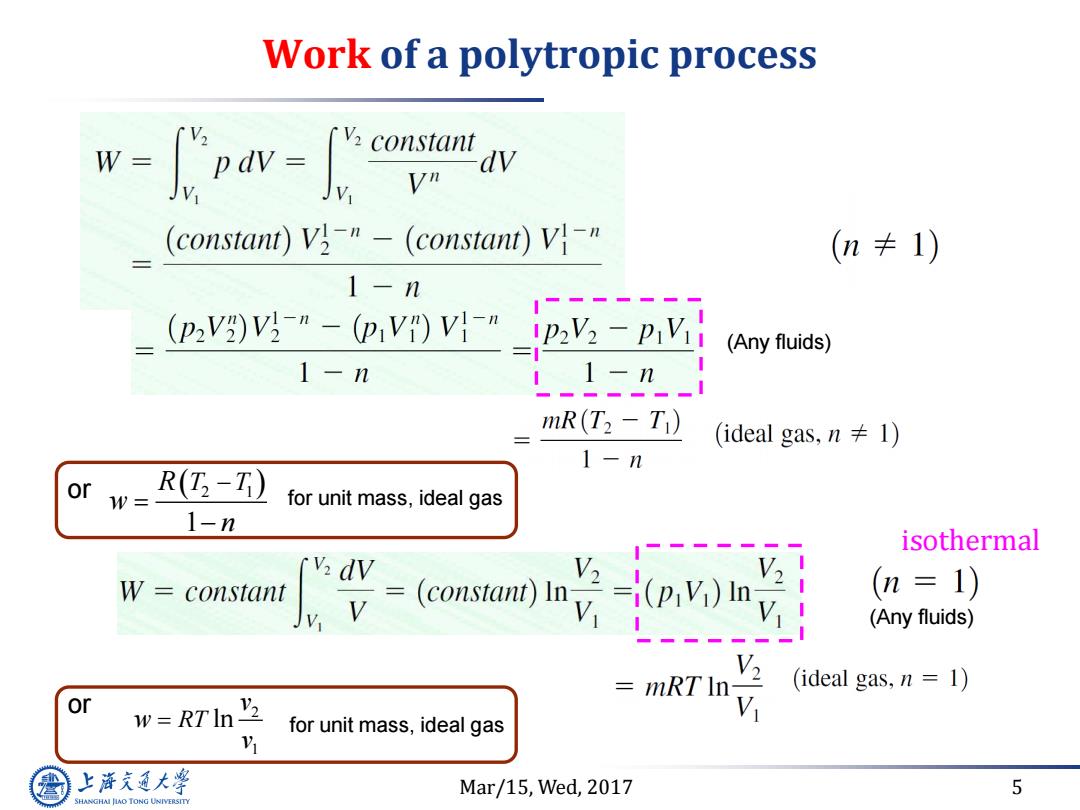
Work of a polytropic process m- w= Vn (constant）V3-"-(constant)）V}-" (n卡1) 1-n (pV)Vg-"-(p,V）V-nt (Any fluids) 1-n 11-n mR(T2-Ti) (ideal gas,n≠I) 1-n or w= (T-T) for unit mass,ideal gas 1-n isothermal [v dv W constant v= V (n=1) (Any fluids) (ideal gas,n 1) or mRT In- w=RTIn for unit mass,ideal gas 上泽通大学 Mar/15,Wed,2017 5 SHANGHAI JIAO TONG UNIVERSITY

Mar/15, Wed, 2017 5 Work of a polytropic process isothermal (Any fluids) (Any fluids)   2 1 1 R T T w n    or for unit mass, ideal gas for unit mass, ideal gas 2 1 RT ln v w v  or

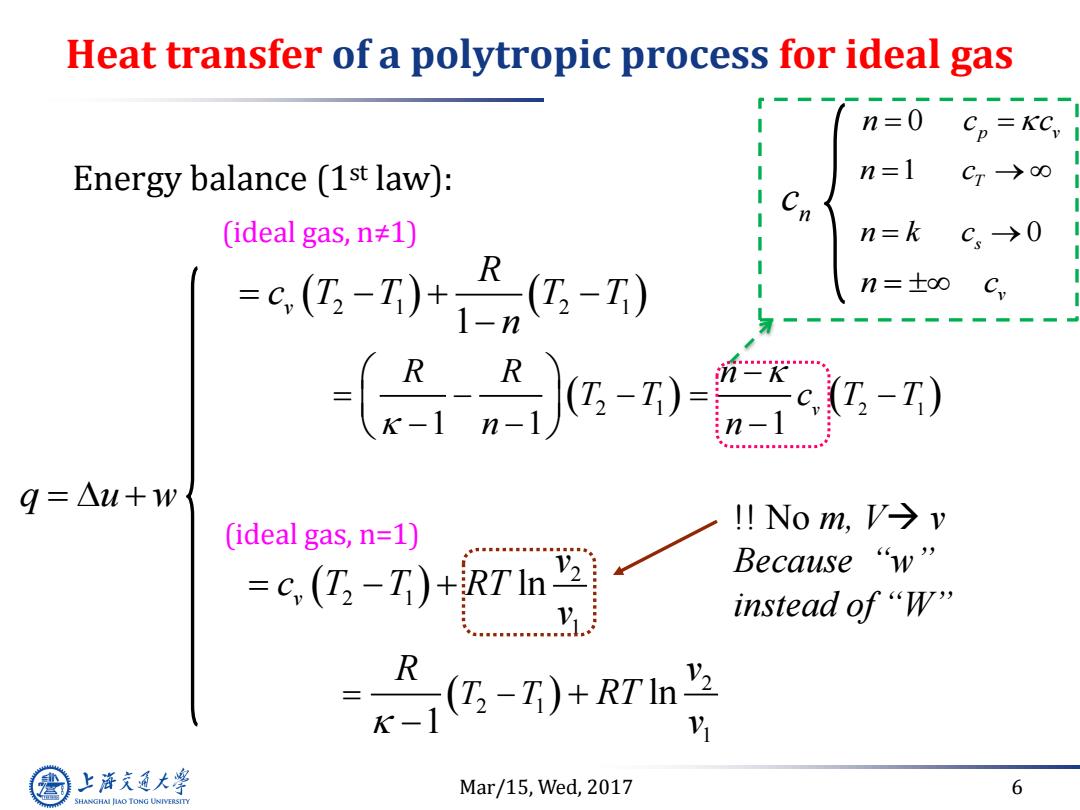
Heat transfer of a polytropic process for ideal gas n=0 Energy balance (1st law): n=1 Cr→0 (ideal gas,n≠1) n=k C→0 -区-0+-W n=±oo C -T) n-l q=△u+w !No m,V→v (ideal gas,n=1) =c (T-T)+RTIn Because“w" V instead of“W" k-g-T)+R7n兰 R 1 上游充通大 Mar/15,Wed,2017 6 SHANGHAI JLAO TONG UNIVERSITY

Mar/15, Wed, 2017 6 Heat transfer of a polytropic process for ideal gas         2 1 2 1 2 1 2 1 1 1 1 1 v v R R n T T c T T n n R c T T T T n                       Energy balance (1st law): (ideal gas, n≠1) q u w    n c 0 p v n c c    n c    1 T n k c  s 0 n c   v (ideal gas, n=1)     2 2 1 1 2 2 1 1 ln ln 1 v T T v c T T RT v R v RT  v        !! No m, V v Because “w” instead of “W

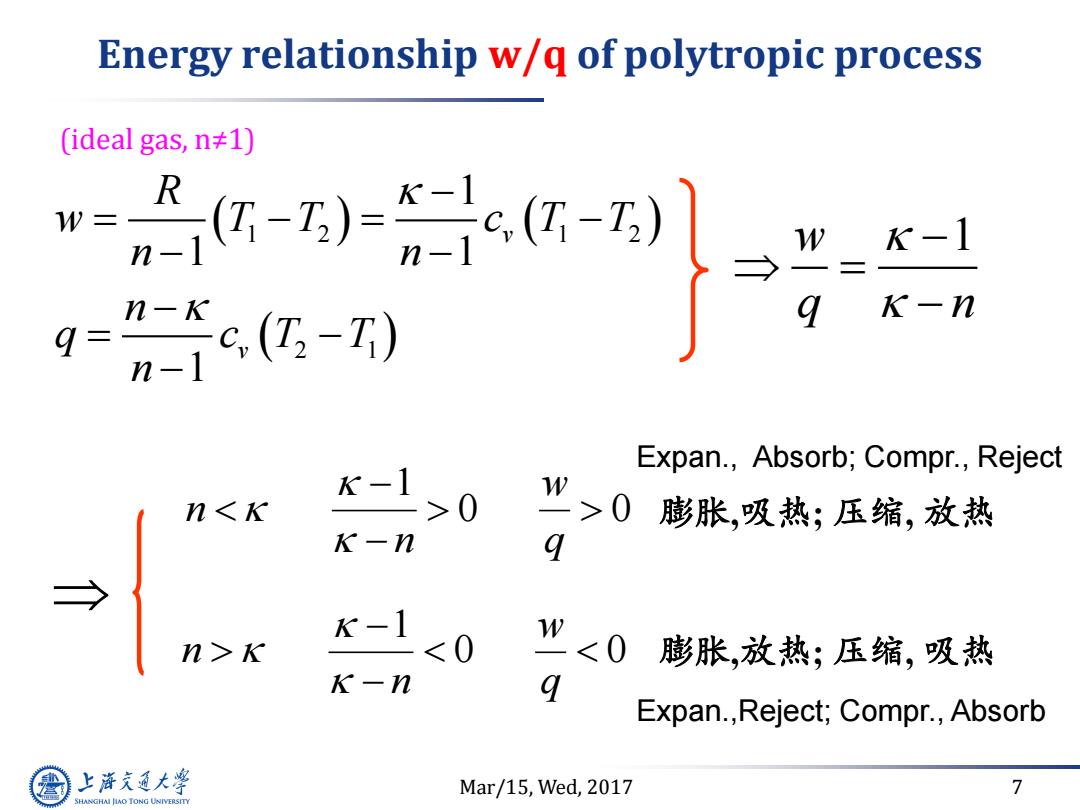
Energy relationship w/q of polytropic process (ideal gas,n≠1) R W K-1 -) K-n q=- Expan.,Absorb;Compr.,Reject K-1 W n0 >0膨胀，吸热；压缩，放热 K-n 9 K-1 n>K <0 w<0 膨胀，放热；压缩，吸热 K一n 9 Expan.,Reject;Compr.,Absorb 上游通大学 Mar/15,Wed,2017 7 SHANGHAI JLAO TONG UNIVERSITY

Mar/15, Wed, 2017 7 Energy relationship w/q of polytropic process       1 2 1 2 2 1 1 1 1 1 v v R w T T c T T n n n q c T T n               0 0 1      q w n n    w 1 q n       0 0 1      q w n n    (ideal gas, n≠1) 膨胀,吸热; 压缩, 放热 Expan., Absorb; Compr., Reject 膨胀,放热; 压缩, 吸热 Expan.,Reject; Compr., Absorb

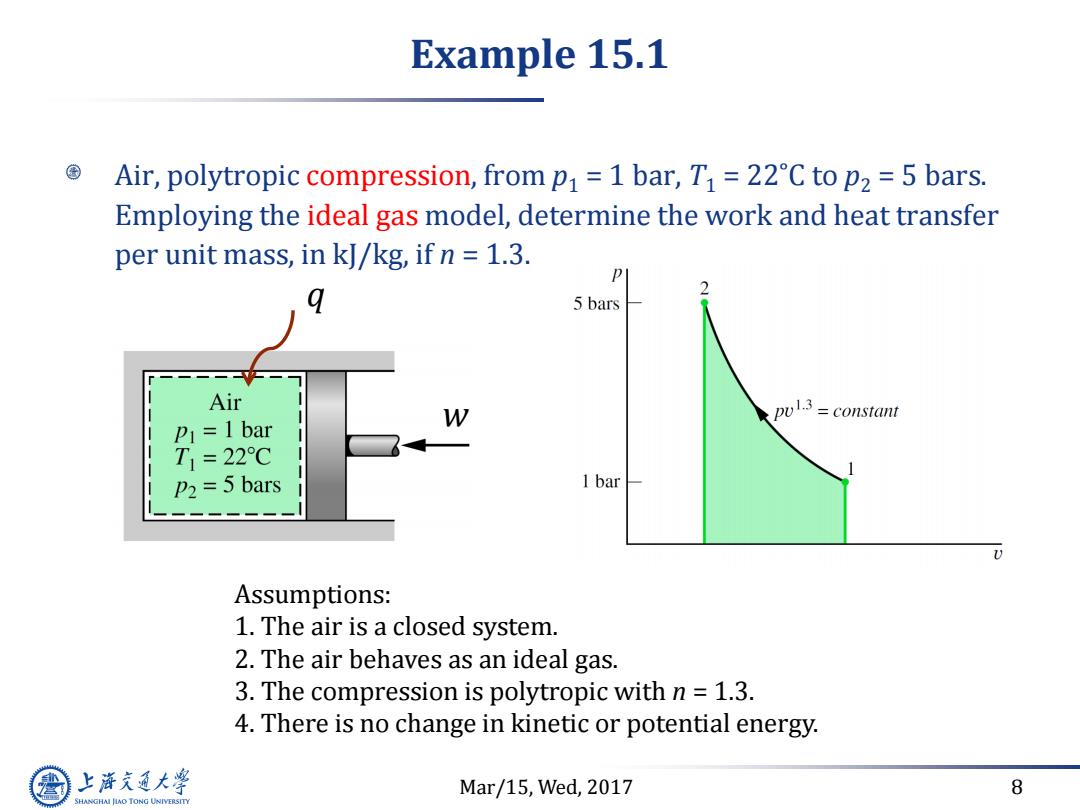
Example 15.1 Air,polytropic compression,from p=1 bar,T1=22C to p2 =5 bars. Employing the ideal gas model,determine the work and heat transfer per unit mass,in kJ/kg,if n 1.3. 5 bars Air W pul3=constant P1=1 bar T1=22℃ P2=5 bars 1 bar Assumptions: 1.The air is a closed system. 2.The air behaves as an ideal gas. 3.The compression is polytropic with n 1.3. 4.There is no change in kinetic or potential energy. 上游充通大 Mar/15,Wed,2017 8 SHANGHAI JLAO TONG UNIVERSITY

Mar/15, Wed, 2017 8 Example 15.1 Air, polytropic compression, from p1 = 1 bar, T1 = 22˚C to p2 = 5 bars. Employing the ideal gas model, determine the work and heat transfer per unit mass, in kJ/kg, if n = 1.3. q w Assumptions: 1. The air is a closed system. 2. The air behaves as an ideal gas. 3. The compression is polytropic with n = 1.3. 4. There is no change in kinetic or potential energy

Example 15.1 Solution P s bars 22C pul3 =constant w-fow W R(T2-F 1bar m 1-31.3 T2 from the process equation (n-1)/m =295K (1.3-1)/1.3 =428K D W= R(T2-T) 8.314kJ 428K-295K =-127.2kJ/kg 1-n 1-1.3 Table A-17 Energy balance △u=9-1w→9=w+(4-4)=-127.2+(306.53-210.49)=-31.16kkg 上游充通大 Mar/15,Wed,2017 9 SHANGHAI JIAO TONG UNIVERSITY

Mar/15, Wed, 2017 9 Example 15.1 Solution 22˚C 1.3 ? T2 from the process equation Energy balance    u q w w  w  2 1 q w u u     ( ) Table A-17

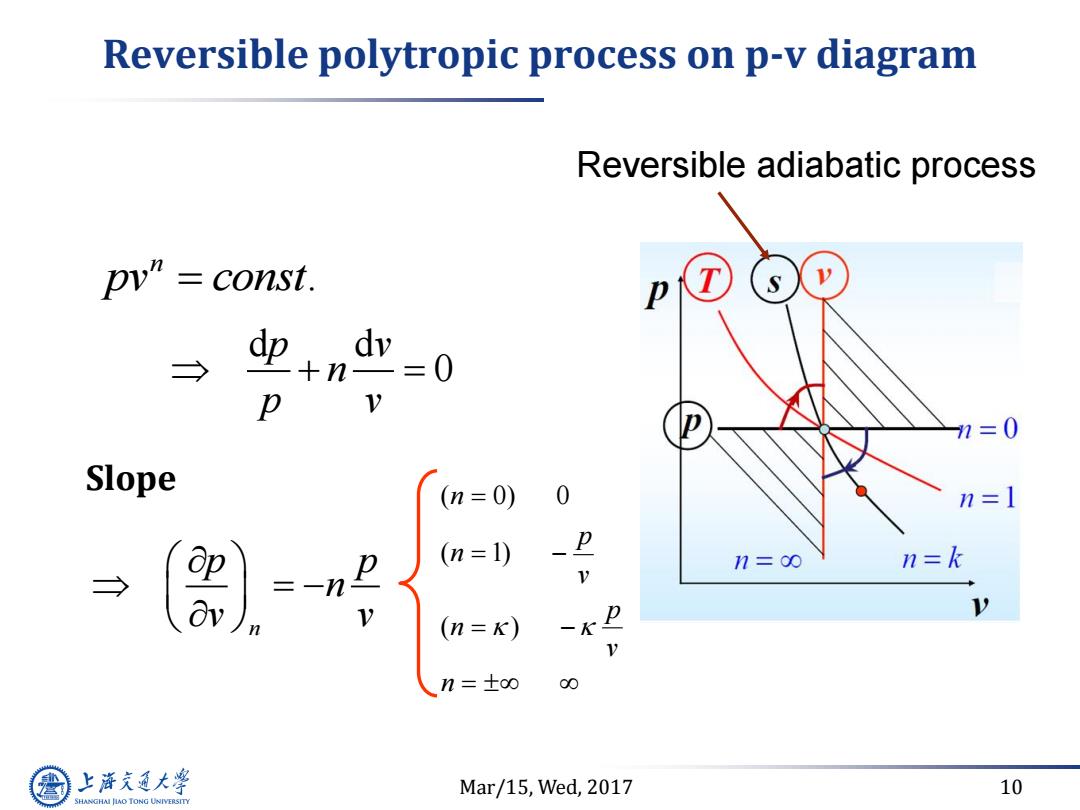
Reversible polytropic process on p-v diagram Reversible adiabatic process pv'”=const. T → dp+n dv =0 p V n=0 Slope (n=0) 0 n=1 → =-n P (n=1) n=0 n=k V p (n=K) 一K D n=±oo 00 上游究通大学 Mar/15,Wed,2017 10 SHANGHAI JLAO TONG UNIVERSITY

Mar/15, Wed, 2017 10 Reversible polytropic process on p-v diagram . n pv const  d d 0 p v n p v    n p p n v v            Slope         n v p n v p n n (  )  ( 1) ( 0) 0 Reversible adiabatic process