上游充通大¥ SHANGHAI JIAO TONG UNIVERSITY Engineering Thermodynamics I Lectures 27-28 Chapter 6 The Second law of thermodynamics (Section 6.7-6.11) Spring,2017 强 Prof.,Dr.Yonghua HUANG 月是a http://cc.sjtu.edu.cn/G2S/site/thermo.html 1日G
Engineering Thermodynamics I Lectures 27-28 Spring, 2017 Prof., Dr. Yonghua HUANG Chapter 6 The Second law of thermodynamics (Section 6.7 -6.11) http://cc.sjtu.edu.cn/G2S/site/thermo.html

Questions about Thermodynamic Cycles How much of the heat put in at high temperature can be converted to work? What is the maximum work? Can two engines working between the same two heat reservoirs drive one another? Nicolas Leonard Sadi Carnot 上游究通大学 Apr/5 Wed,2017 2 SHANGHAI JLAO TONG UNIVERSITY
Apr/5 Wed, 2017 2 Questions about Thermodynamic Cycles How much of the heat put in at high temperature can be converted to work? What is the maximum work? Can two engines working between the same two heat reservoirs drive one another? Nicolas Léonard Sadi Carnot
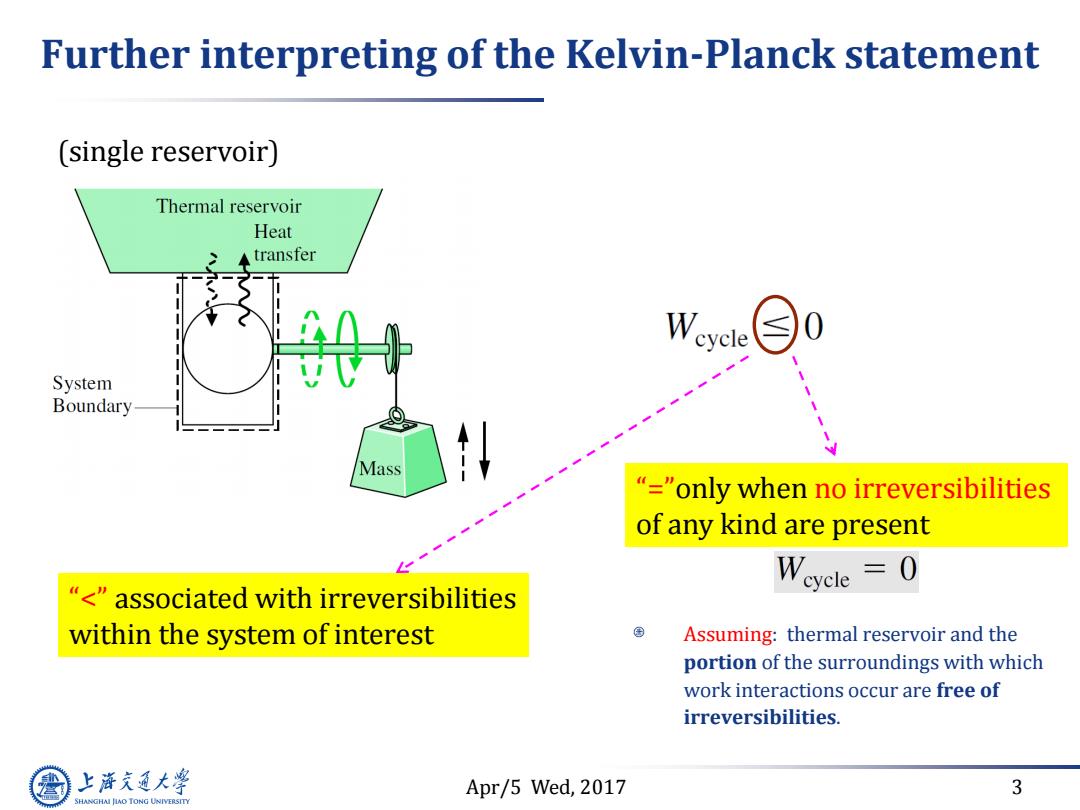
Further interpreting of the Kelvin-Planck statement (single reservoir) Thermal reservoir Heat Atransfer W ycle System Boundary Mass "="only when no irreversibilities of any kind are present "<"associated with irreversibilities within the system of interest Assuming:thermal reservoir and the portion of the surroundings with which work interactions occur are free of irreversibilities. 上游充通大 Apr/5 Wed,2017 3 SHANGHAI JIAO TONG UNIVERSITY
Apr/5 Wed, 2017 3 Further interpreting of the Kelvin-Planck statement Assuming: thermal reservoir and the portion of the surroundings with which work interactions occur are free of irreversibilities. “<” associated with irreversibilities within the system of interest “=”only when no irreversibilities of any kind are present (single reservoir)
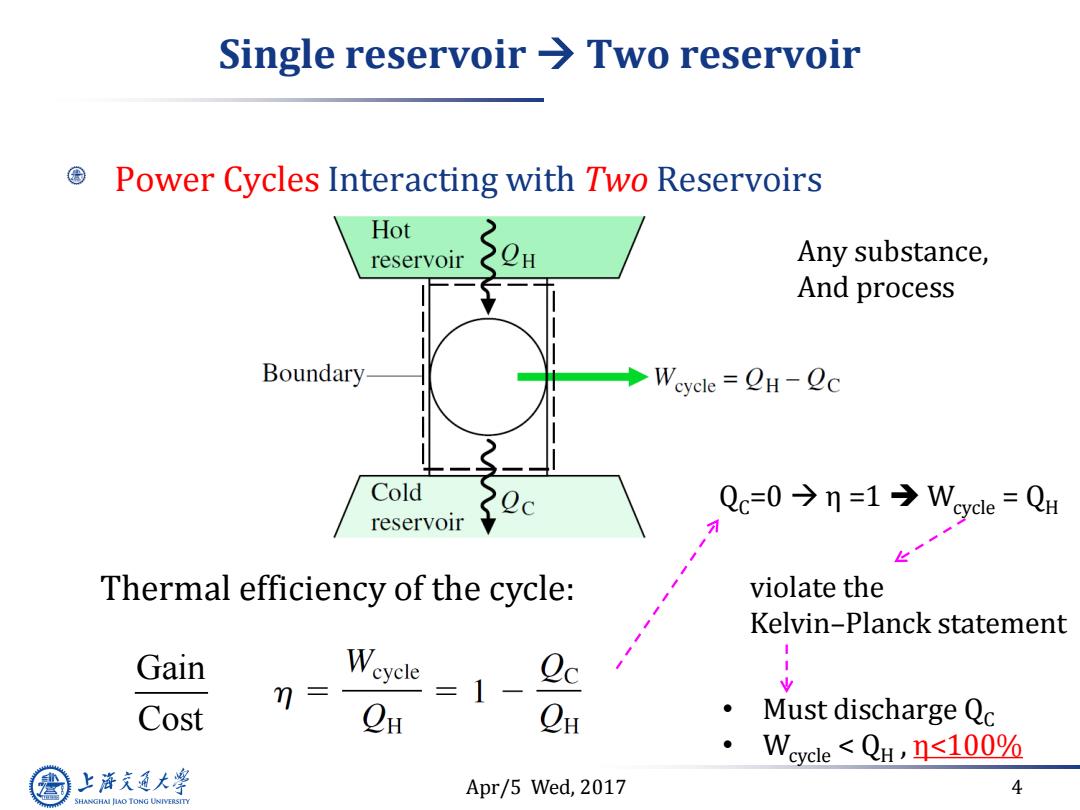
Single reservoir Two reservoir Power Cycles Interacting with Two Reservoirs Hot reservoir Any substance, And process Boundary ◆Weycle=QH-Qc Cold reservoir Qc=0今n=1→Wycle=QH Thermal efficiency of the cycle: violate the Kelvin-Planck statement Gain n= =1- Cost C CH Must discharge Qc Weycle<QH,n≤100% 上游充通大 Apr/5 Wed,2017 4 SHANGHAI JLAO TONG UNIVERSITY
Apr/5 Wed, 2017 4 Single reservoir Two reservoir Power Cycles Interacting with Two Reservoirs Thermal efficiency of the cycle: QC=0 η =1 Wcycle = QH violate the Kelvin–Planck statement • Must discharge QC • Wcycle < QH , η<100% Any substance, And process Gain Cost
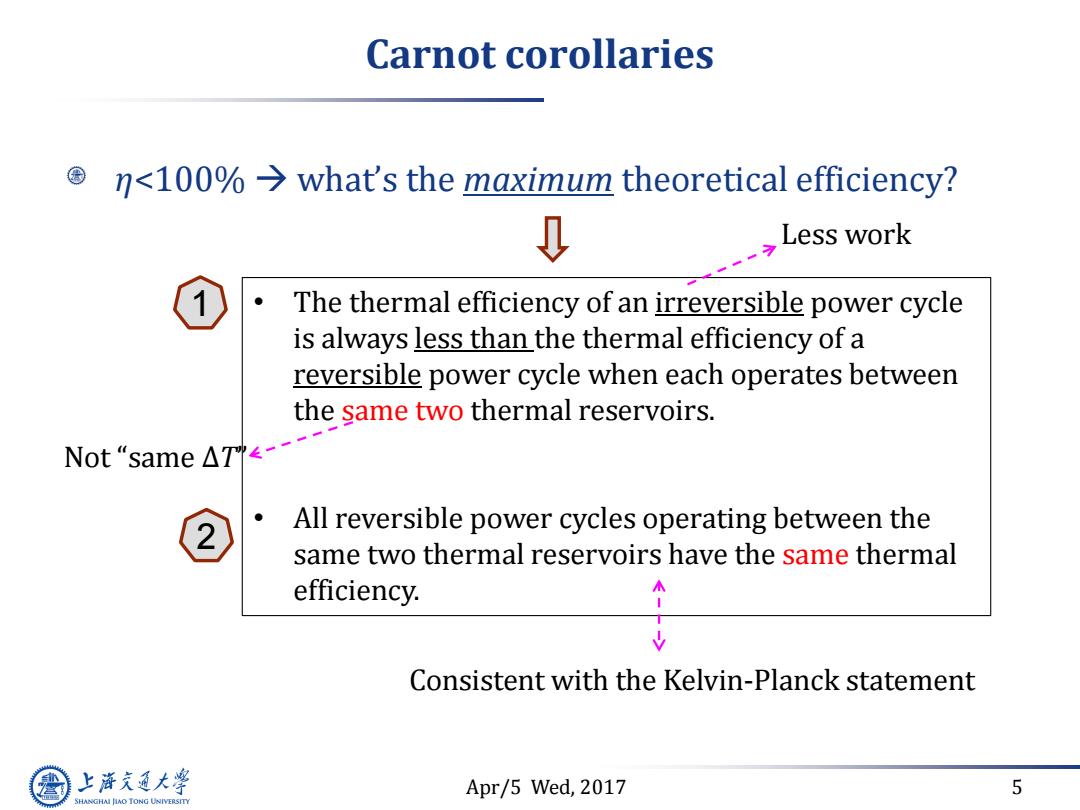
Carnot corollaries nwhat's the maximum theoretical efficiency? Less work The thermal efficiency of an irreversible power cycle is always less than the thermal efficiency of a reversible power cycle when each operates between the same two thermal reservoirs. Not“same△T<-- All reversible power cycles operating between the same two thermal reservoirs have the same thermal efficiency. Consistent with the Kelvin-Planck statement 上游充通大学 Apr/5 Wed,2017 5 SHANGHAI JIAO TONG UNIVERSITY
Apr/5 Wed, 2017 5 Carnot corollaries η<100% what’s the maximum theoretical efficiency? • The thermal efficiency of an irreversible power cycle is always less than the thermal efficiency of a reversible power cycle when each operates between the same two thermal reservoirs. • All reversible power cycles operating between the same two thermal reservoirs have the same thermal efficiency. Not “same ΔT” Less work Consistent with the Kelvin-Planck statement 1 2
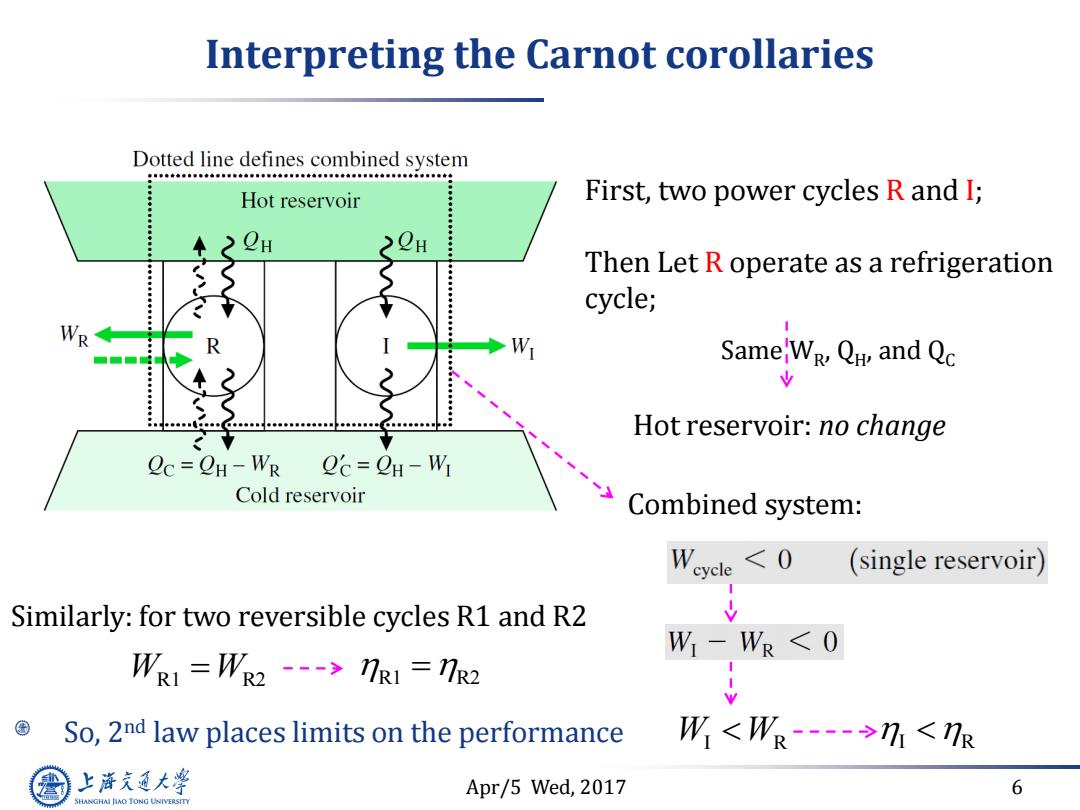
Interpreting the Carnot corollaries Dotted line defines combined system Hot reservoir First,two power cycles R and I; Then Let R operate as a refrigeration cycle; WR W SameW Q and Qc Hot reservoir:no change Oc=CH-WR Oc=CH-WI Cold reservoir Combined system: W cle 7R1=R2 So,2nd law places limits on the performance W<W-→1<R 上游充通大 Apr/5 Wed,2017 6 SHANGHAI JIAO TONG UNIVERSITY
Apr/5 Wed, 2017 6 Interpreting the Carnot corollaries First, two power cycles R and I; Then Let R operate as a refrigeration cycle; Same WR , QH , and QC Hot reservoir: no change Combined system: W W I R I R W W R1 R2 R1 R2 Similarly: for two reversible cycles R1 and R2 So, 2nd law places limits on the performance
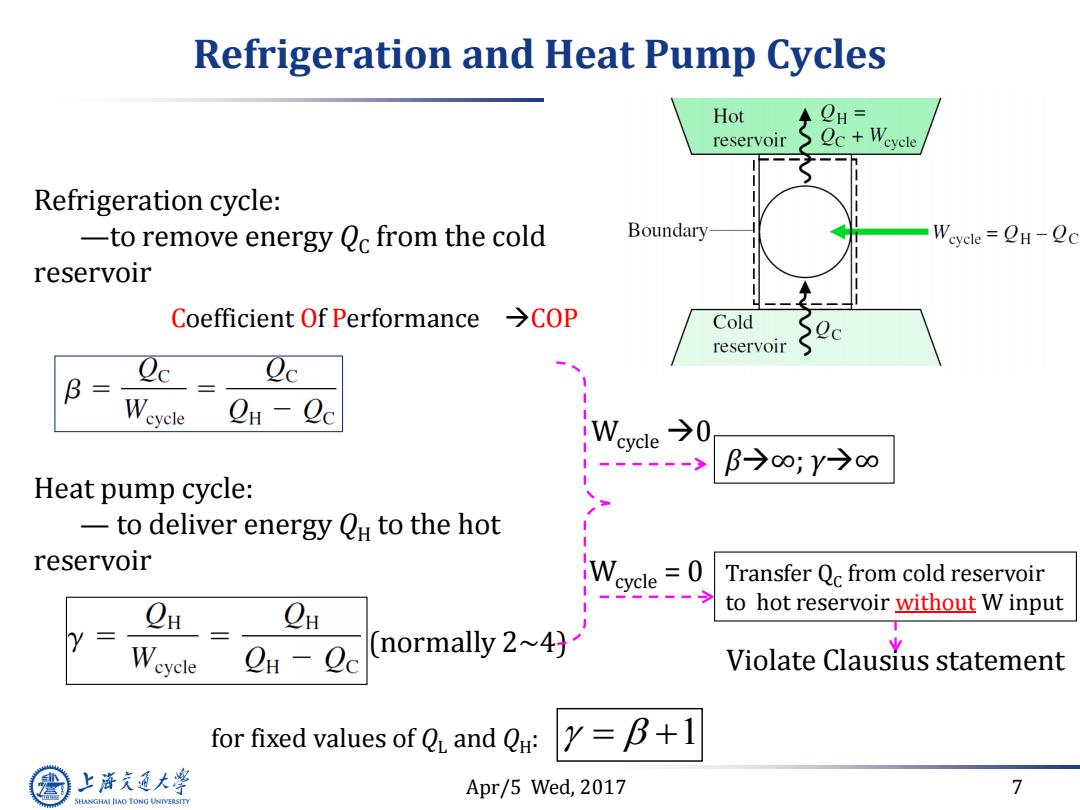
Refrigeration and Heat Pump Cycles Hot CH= reservoir c+Weyele Refrigeration cycle: -to remove energy Qc from the cold Boundary Weyele OH-Oc reservoir Coefficient Of Performance >COP Cold reservoir Qe B Weycle CH-Qc B→∞;Y→∞ Heat pump cycle: -to deliver energy OH to the hot reservoir cycle =0 Transfer Qc from cold reservoir CH to hot reservoir without W input Wcycle Cn-Qc (normally 2~4) Violate Clausius statement for fixed values of Q and Q: Y=B+1 上泽通大学 Apr/5 Wed,2017 7 SHANGHAI JIAO TONG UNIVERSITY
Apr/5 Wed, 2017 7 Refrigeration and Heat Pump Cycles Refrigeration cycle: —to remove energy QC from the cold reservoir Heat pump cycle: — to deliver energy QH to the hot reservoir Coefficient Of Performance COP for fixed values of QL and QH : 1 (normally 2~4) Wcycle 0 β∞; γ∞ Wcycle = 0 Transfer QC from cold reservoir to hot reservoir without W input Violate Clausius statement
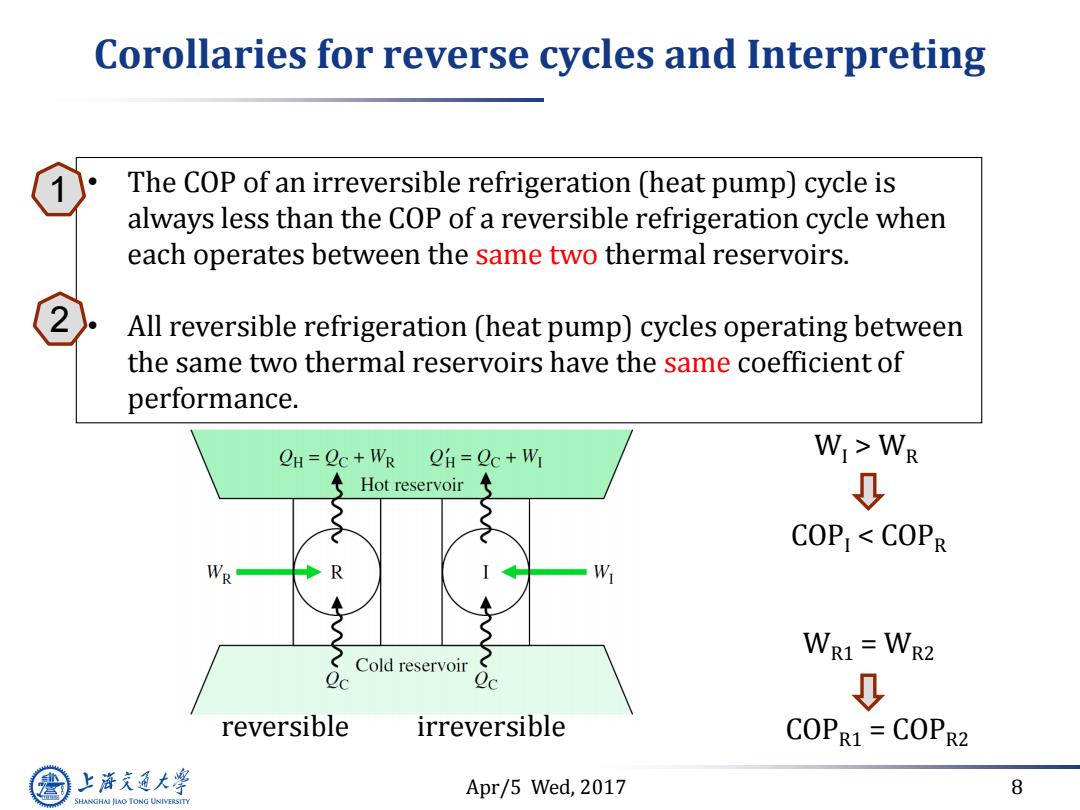
Corollaries for reverse cycles and Interpreting The COP of an irreversible refrigeration (heat pump)cycle is always less than the COP of a reversible refrigeration cycle when each operates between the same two thermal reservoirs. All reversible refrigeration (heat pump)cycles operating between the same two thermal reservoirs have the same coefficient of performance. CH=Oc+WR OH=Qc+WI WI>WR Hot reservoir 0 COPI<COPR WR R W WR1=WR2 2c Cold reservoir 2c ↓ reversible irreversible COPR1=COPR2 上游文通大学 Apr/5 Wed,2017 8 SHANGHAI JIAO TONG UNIVERSITY
Apr/5 Wed, 2017 8 Corollaries for reverse cycles and Interpreting reversible irreversible WI > WR COPI < COPR WR1 = WR2 COPR1 = COPR2 • The COP of an irreversible refrigeration (heat pump) cycle is always less than the COP of a reversible refrigeration cycle when each operates between the same two thermal reservoirs. • All reversible refrigeration (heat pump) cycles operating between the same two thermal reservoirs have the same coefficient of performance. 1 2
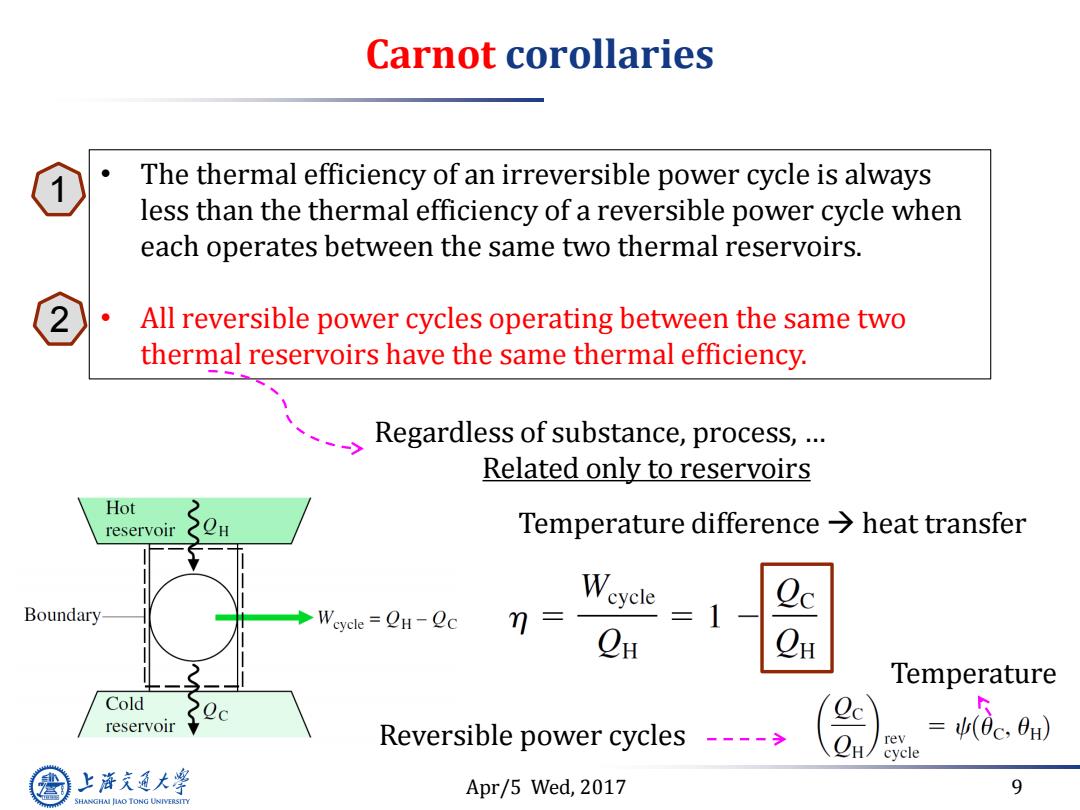
Carnot corollaries ● The thermal efficiency of an irreversible power cycle is always less than the thermal efficiency of a reversible power cycle when each operates between the same two thermal reservoirs. All reversible power cycles operating between the same two thermal reservoirs have the same thermal efficiency. Regardless of substance,process,... Related only to reservoirs Hot reservoir Temperature difference>heat transfer Boundary Weyele=CH-Oc m= CH CH Temperature Cold reservoir Reversible power cycles ---- rev cycle 上降文通大学 Apr/5 Wed,2017 9 SHANGHAI JIAO TONG UNIVERSITY
Apr/5 Wed, 2017 9 Carnot corollaries • The thermal efficiency of an irreversible power cycle is always less than the thermal efficiency of a reversible power cycle when each operates between the same two thermal reservoirs. • All reversible power cycles operating between the same two thermal reservoirs have the same thermal efficiency. Regardless of substance, process, … Related only to reservoirs Temperature difference heat transfer Reversible power cycles Temperature 1 2
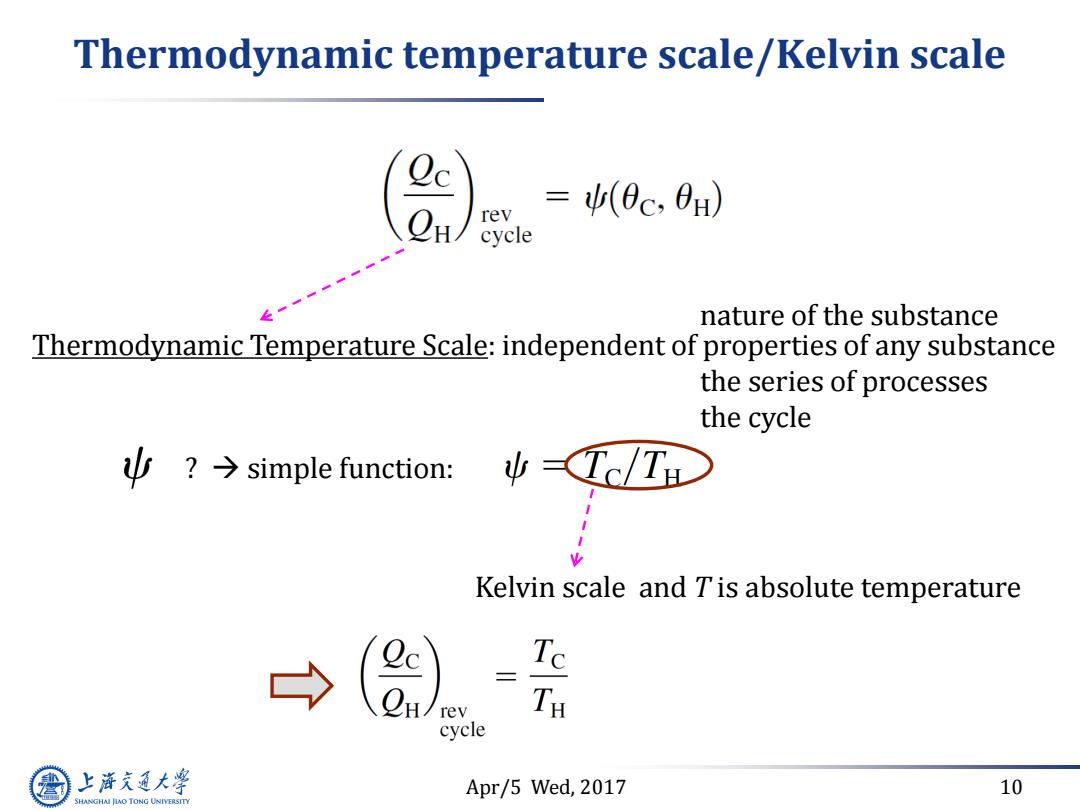
Thermodynamic temperature scale/Kelvin scale =(0c,0H) cycle nature of the substance Thermodynamic Temperature Scale:independent of properties of any substance the series of processes the cycle y?→simple function: ψ(Tc/TH Kelvin scale and Tis absolute temperature Te rey TH cycle 上游气通大粤 Apr/5 Wed,2017 10 SHANGHAI JLAO TONG UNIVERSITY
Apr/5 Wed, 2017 10 Thermodynamic temperature scale/Kelvin scale Thermodynamic Temperature Scale: independent of properties of any substance ? simple function: Kelvin scale and T is absolute temperature nature of the substance the series of processes the cycle