同济大学课程考核试卷(A卷) 2011一2012学年第1学期 Course Name:Finite Element Method(5 CPs)-Theory part Name _Student No. Major _Score_ (100 points,120 minutes,closed) A (20 points) (10 points) (15 points) (15 points)(30 points)(10 points) 120op ing True(T)/False(F)Questions.Please mark No Questions T/F 7 In a finite element,the sum of shape functions is equal to 1.0 at the nodes only. 2 T nodes triangular elements,the strain can be discontinuous across element boundaries. The numbering of the nodes of 3-noded triangle finite elements should always be clockwise 4 In a beam element,both end-point displacement and slop T (rotation)should be continuous. p-refinement means increasing the number elements(reducing individual element size) 6 The shape of a quadrilateral isoparametric element can be convex or concave. For welded truss connections,beam elements should be used 8 Linear elements (field variable itself is linear)are linear in strain and stress. Higher order shape functions can increase the accuracy of 9 an approximate solution,but there is a price to pay:the 人 required computational effort increases. 10 order of the ele ent correlates with the order of displacement,strain and stress that is modeled
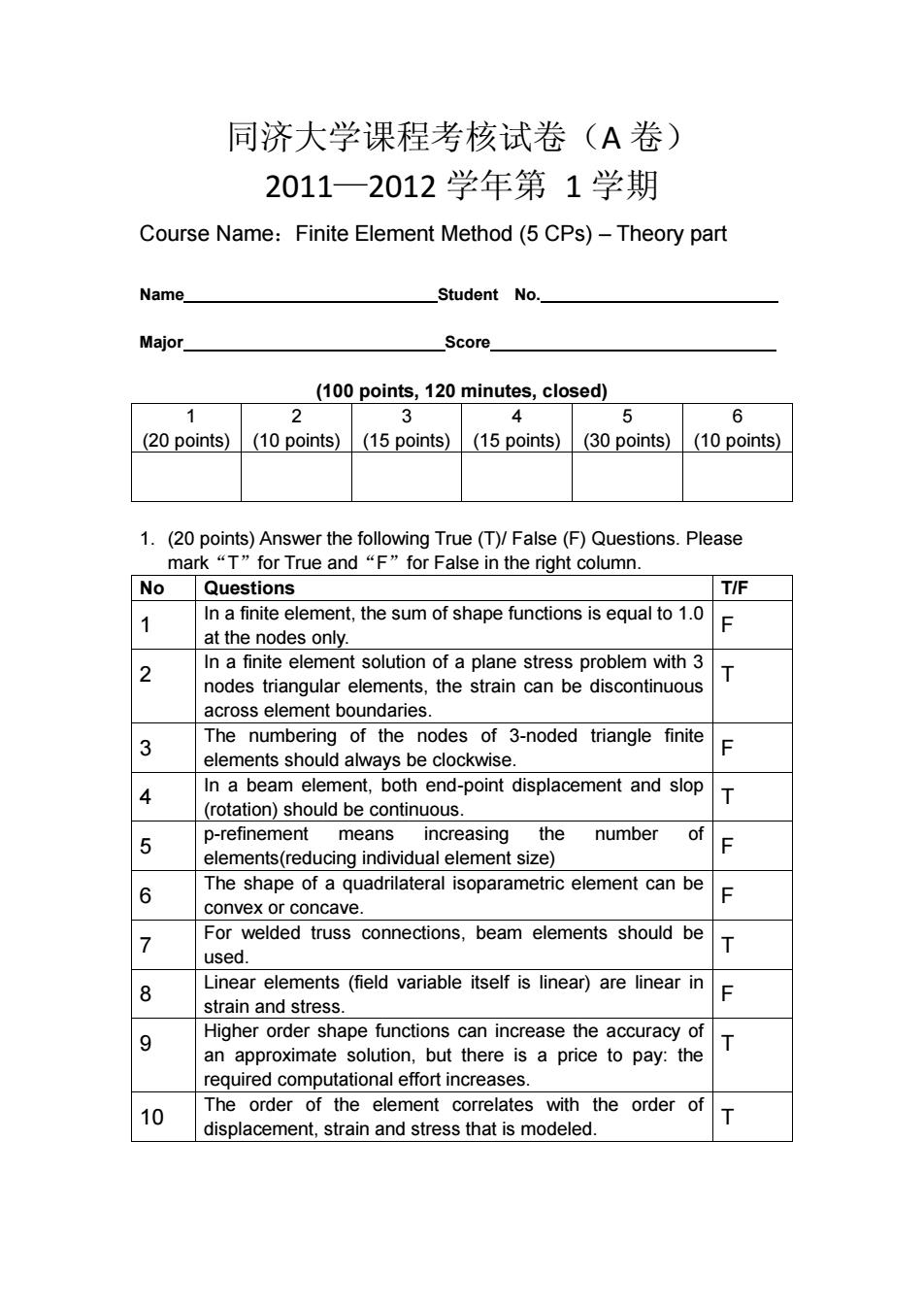
同济大学课程考核试卷(A 卷) 2011—2012 学年第 1 学期 Course Name:Finite Element Method (5 CPs) – Theory part Name Student No. Major Score (100 points, 120 minutes, closed) 1 (20 points) 2 (10 points) 3 (15 points) 4 (15 points) 5 (30 points) 6 (10 points) 1. (20 points) Answer the following True (T)/ False (F) Questions. Please mark“T”for True and“F”for False in the right column. No Questions T/F 1 In a finite element, the sum of shape functions is equal to 1.0 at the nodes only. F 2 In a finite element solution of a plane stress problem with 3 nodes triangular elements, the strain can be discontinuous across element boundaries. T 3 The numbering of the nodes of 3-noded triangle finite elements should always be clockwise. F 4 In a beam element, both end-point displacement and slop (rotation) should be continuous. T 5 p-refinement means increasing the number of elements(reducing individual element size) F 6 The shape of a quadrilateral isoparametric element can be convex or concave. F 7 For welded truss connections, beam elements should be used. T 8 Linear elements (field variable itself is linear) are linear in strain and stress. F 9 Higher order shape functions can increase the accuracy of an approximate solution, but there is a price to pay: the required computational effort increases. T 10 The order of the element correlates with the order of displacement, strain and stress that is modeled. T

2.(10 points)What are requirements for shape function?The quadratic mP(x.yayis incomplete but symmetric.st fo representing the fied variable a four-node rectangular element having 1 degree of freedom per node?Explain. Solution:Shape functions must meet:(1)Completeness:(2)Compatibility. It's wrong,because it cannot represent rigid motion and constant strain status compatibility,that is be like this:P(x,y)=a+bx+cy+dxy
2. (10 points) What are requirements for shape function? The quadratic polynomial P(x,y)=a0+a1x 2 +a2xy+a3y 2 is incomplete but symmetric. Is this function suitable for representing the field variable in a four-node rectangular element having 1 degree of freedom per node? Explain. Solution: Shape functions must meet: (1) Completeness; (2) Compatibility. It’s wrong, because it cannot represent rigid motion and constant strain status (for structural analysis), and cannot guarantee the compatibility, that is continuity of field variables along the common boundary. The right one should be like this: P(x,y)=a+bx+cy+dxy

3.(15 points)Examine if the following isoparametric mapping is invertible? What conclusion can you draw from the results? 3 1.1) 5 T-1 (0.0 Note:The shape functions in natural coordinates are N(5,)=20-50-.N,(5,)=20+50-列 Ne0=20+50+N,(G,)=0-50+0 and the determinant of Jacobian is J=detJ= Ox oy ax Oy ogon onog Solution: 嘉8Gm-s0GG0-0n y-总G-a5+G0-0+9 Note thatthe map is not invertable. Conclusion:Clockwise numbering of nodes is prohibited
3. (15 points) Examine if the following isoparametric mapping is invertible? What conclusion can you draw from the results? Note: The shape functions in natural coordinates are and the determinant of Jacobian is Solution: Conclusion: Clockwise numbering of nodes is prohibited

4.(15 points)Develop the shape functions Ns and N for the 5-node transition rectangular element shown below.(Node 5 is located at midpoint of side 1-2.you can use the shape function formula in Problem 3) 3 (1.1) Solution: £-7=0 (1.1) N5=B*(5-7)(5+7) N,(0,-1)=B0-(-1)0+(-1)=-B=1 B=-1 N=0-50+=2-5,x@-0=月 N,=40-500-0)-2N
4. (15 points) Develop the shape functions N5 and N1 for the 5-node transition rectangular element shown below. (Node 5 is located at midpoint of side 1-2. you can use the shape function formula in Problem 3) Solution: N5= *( )( ) 1 5 1 2 2 5 5 2 1 (1 )(1 ) 4 1 2 1 ( )( ) , (0, 1) 1 (0, 1) (0 ( 1))(0 ( 1)) 1 N N N N N 5

5.(30 points)Figure below shows a pin-jointed plane truss discretized with 2 elements and 3 nodes.node 3 is fixed whereas 1 and 2 move over rollers as shown.Thec ero applied load acts upward on node 1.Solve this problem by the Start from the element stiffness equations given below.These are listed and already incorporate the E AL factor(stiffness factor for bar element)in the stiffness matrices. P=51 10,0 月302,0 →x (r v)node coordinate written in parenthesis E-200N2,.=3m 62 for both (1)and (2) after node numbers 26.-0 The element stiffness equations in global coordinates are as follows: 50-50-50 507 5050-50-507 -505050-50 5050-50-50 -50-505050 L-50-505050J (1)Assemble the master stiffness equations (2)Apply the given force and displacement BCs to get a reduced system of 2 equations and show it. (3)Solve the reduced stiffness system for the unknown displacements and show the complete node displacen nent vector. (4)Recover the axial force F(2)in element(2)using the displacements you got in (3),noting sign. Note:The displacement transformation is 0 0 -5c00 0 0 0 0
5. (30 points) Figure below shows a pin-jointed plane truss discretized with 2 elements and 3 nodes. Node 3 is fixed whereas 1 and 2 move over rollers as shown. The only nonzero applied load acts upward on node 1. Solve this problem by the Direct Stiffness Method. Start from the element stiffness equations given below. These are listed and already incorporate the E e A e /L e factor (stiffness factor for bar element) in the stiffness matrices. The element stiffness equations in global coordinates are as follows: (1) Assemble the master stiffness equations. (2) Apply the given force and displacement BCs to get a reduced system of 2 equations and show it. (3) Solve the reduced stiffness system for the unknown displacements and show the complete node displacement vector. (4) Recover the axial force F(2) in element (2) using the displacements you got in (3), noting sign. Note: The displacement transformation is

(For Problem 5) Solution: (a)Assembled master stiffessequations 900 0 (Q13) 0-50 -50 [8-[ Q1.40 (c)Solving: 4x2= -1/10=-0.10.41=1/5=0.2 Q1.5 Complete displacement solution: m=00.20-0.10000] Q1.6) Points are deducted for not listing this vector. ber (2)which rom the 81f "2=0 0 Q1.7 6*105=9=+0m 1 (Q1.8)
(For Problem 5) Solution:

6.(10 points)Identify three problems in the mesh shown below.Mark at the corresponding lines and explain in text. 08 CST Q4 LST 08 Solution: 3 21 Q8 CST Q4 LST Q8 (1)Incompatibility between linear displacement and quadratic displacement along the common edge. (2)Incompatibility between quadratic triangle and bilinear quadrilateral (rectangular)along the common edge. (3)Incompatibility between bilinear and quadratic quadrilateral (rectangular) elements along the overlapped edge
6. (10 points) Identify three problems in the mesh shown below. Mark at the corresponding lines and explain in text. Solution: (1) Incompatibility between linear displacement and quadratic displacement along the common edge. (2) Incompatibility between quadratic triangle and bilinear quadrilateral (rectangular) along the common edge. (3) Incompatibility between bilinear and quadratic quadrilateral (rectangular) elements along the overlapped edge