
2014/5/15 The Direct Stiffness Method(DSM) Importance:DSM is used by all major commercial FEM codes DSM-Steps The Example Truss (bar elements in 2-D space)-FEM Model -Member(Element)Formation ·Assembly&Solution -Globalization Merge S8nonoBcs Nodes,Eements and DOFs Applled Loads and Supp -Recovery of Derived Quantities Global(Master)Stiffness Equations Element(Member)Stiffness Equations T-Ka 自作图 1
2014/5/15 1 FEM – Chapter 3 Direct Stiffness Method (DSM) The Direct Stiffness Method (DSM) • Importance: DSM is used by all major commercial FEM codes DSM - Steps • Breakdown - Disconnection - Localization - Member (Element) Formation • Assembly & Solution - Globalization - Merge - Application of BCs - Solution - Recovery of Derived Quantities The Example Truss (bar elements in 2-D space) - FEM Model: Nodes, Elements and DOFs Applied Loads and Supports Global(Master) Stiffness Equations Element (Member) Stiffness Equations

2014/5/15 omcum The 2-Node Bar(Truss)Element ,ai, These steps are conceptua(not actally programmed) hanic5ownt5Sto网 Globalization:Displacement Transformation 图[间三安 [:询空 Displacement Transformation(cont'd) Globalization:Force Transformation [图E目 o Tw 阁陶 r=tyt
2014/5/15 2 First Two Breakdown Steps: Disconnection and Localization These steps are conceptual(not actually programmed) The 2-Node Bar (Truss) Element Bar (Truss) Element Formulation by Mechanics of Materials (MoM) Globalization: Displacement Transformation Displacement Transformation (cont'd) Globalization: Force Transformation

2014/5/15 The Example Truss-FEM Model R。- -Tr P=(TYP K-(T)RT Rules That Govern Assembly 图图 mof lmembers 圓-调 and.it is 信图 Expanded Element Stiffness Equations n m。 围 =k 3
2014/5/15 3 Globalization: Transformation of Element Stiffness Matrices The Example Truss - FEM Model Obtain the Globalized Element Stiffness Equations of the Example Truss Rules That Govern Assembly 1. Compatibility: The joint displacements of all members meeting at a joint must be the same 2. Equilibrium: The sum of forces exerted by all members that meet at a joint must balance the external force applied to that joint. To apply these rules in assembly by hand, it is convenient to expand or augment the element stiffness equations as shown for the example truss on the next slide. Expanded Element Stiffness Equations Reconnecting Members by Enforcing Compatibility Rule

2014/5/15 Apply Equilibrium Rule 【=r,rP,-kKK=Ku B t-m.p. Where Do Boundary Conditions Go? 非修 [-[ [:-[ R幻三 4
2014/5/15 4 Apply Equilibrium Rule Applying this to all joints: Forming the Master Stiffness Equations through Equilibrium Rule Applying Support and Loading Boundary Conditions Where Do Boundary Conditions Go? Reduced Master Stiffness Equations for Hand Computation Strike out rows and columns pertaining to known displacements: Solve for Unknown Node Displacements and Complete the Displacement Vector
2014/5/15 Recovery of Node Forces Including Reactions w-Tr Example Solution: For the plane truss shown belw mhrdoerpeF P-1000 EN.L-La B:1- =,=】 and the BC.we have -=0 -[竖-wa 5
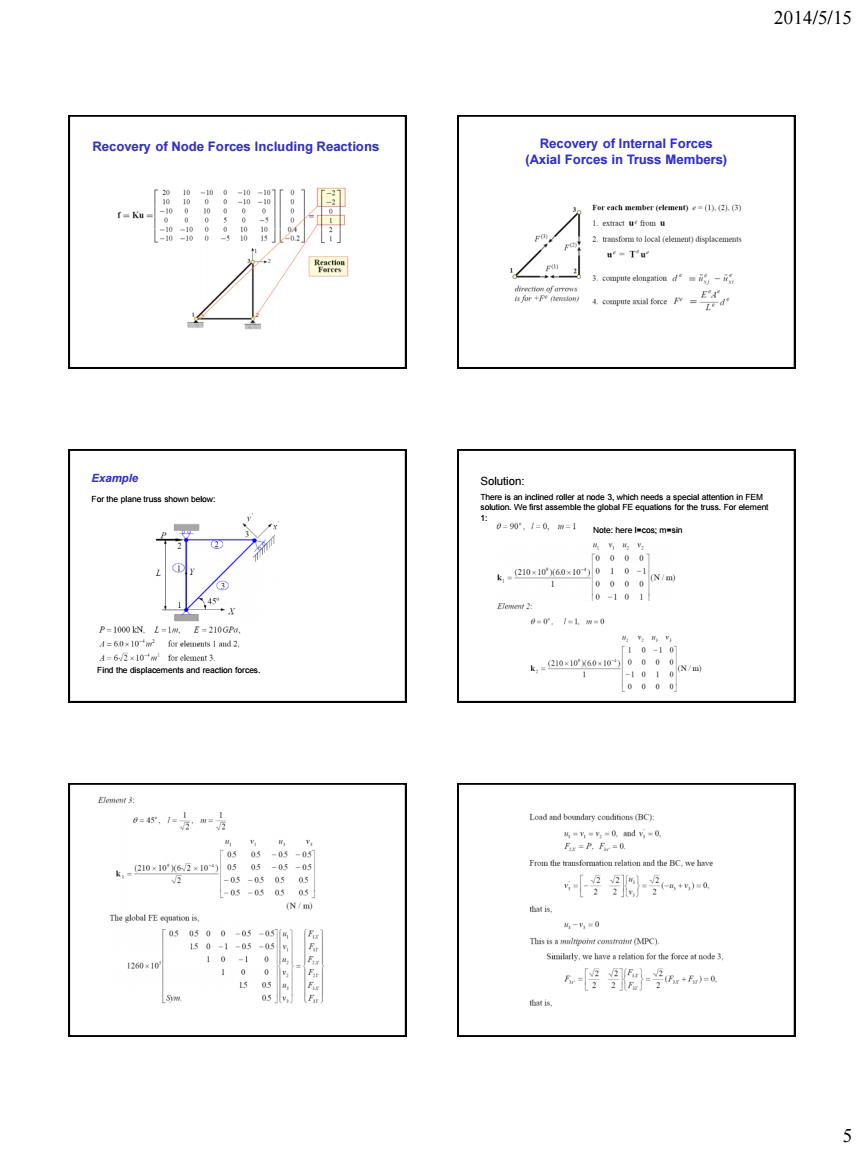
2014/5/15 5 Recovery of Node Forces Including Reactions Recovery of Internal Forces (Axial Forces in Truss Members) Example For the plane truss shown below: Find the displacements and reaction forces. Solution: There is an inclined roller at node 3, which needs a special attention in FEM solution. We first assemble the global FE equations for the truss. For element 1: Note: here l=cos; m=sin

2014/5/15 +=0 ,3Ee m月 1200.0 aao-aa 一电卧图 e p m园 ) 6
2014/5/15 6