
ioing biparite classical distributions and quantum states Rahul Jain,Yaoyun Shi,Zhaohui Wei,Shengyu Zhang
Rahul Jain, Yaoyun Shi, Zhaohui Wei, Shengyu Zhang
randomness/entanglement Shared randomness/entanglement is an important resource in distributive settings. Question:How hard to generate a bipartite classical distribution or quantum state?
randomness/entanglement • Shared randomness/entanglement is an important resource in distributive settings. • Question: How hard to generate a bipartite classical distribution or quantum state?
3 scenarios Distribution generation Distribution approximation Quantum state approximation
3 scenarios • Distribution generation • Distribution approximation • Quantum state approximation
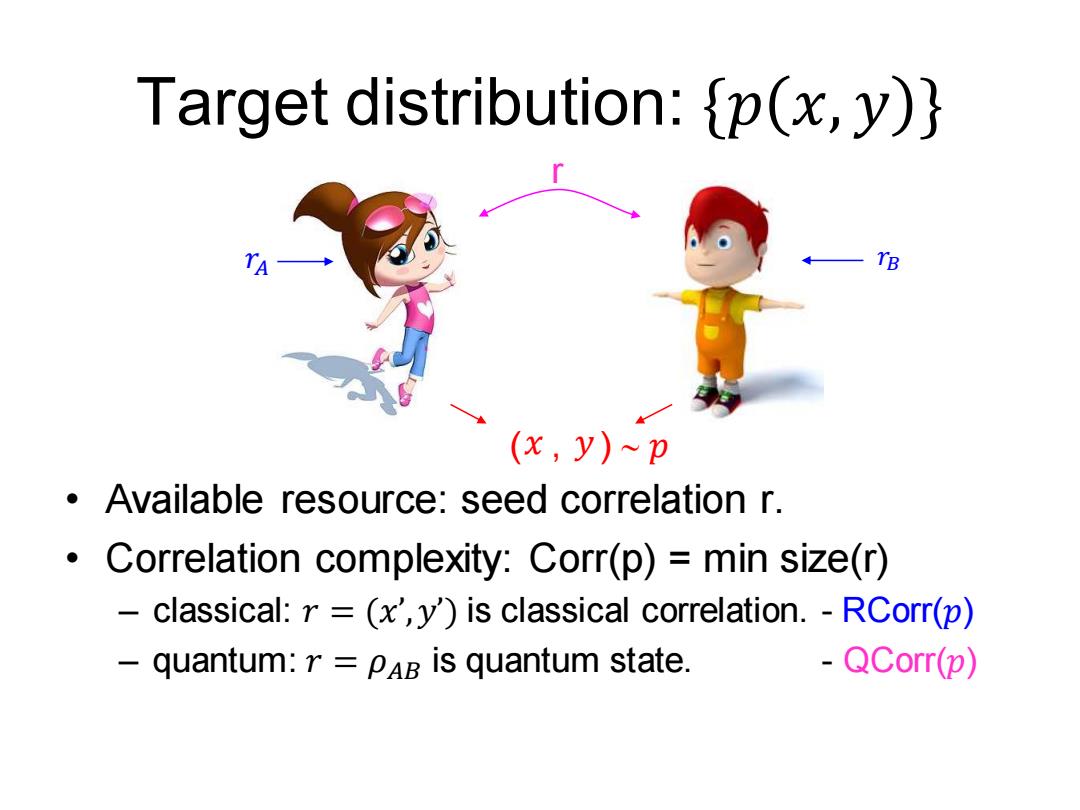
Target distribution:p(x,y)} TB (x,y)~p Available resource:seed correlation r. Correlation complexity:Corr(p)=min size(r) classical:r =(x',y')is classical correlation.-RCorr(p) 一 quantum:r =PAB is quantum state. QCorr(p)
Target distribution: {𝑝 𝑥, 𝑦 } • Available resource: seed correlation r. • Correlation complexity: Corr(p) = min size(r) – classical: 𝑟 = (𝑥’,𝑦’) is classical correlation. - RCorr(𝑝) – quantum: 𝑟 = 𝜌𝐴𝐵 is quantum state. - QCorr(𝑝) 𝑟𝐵 𝑥 𝑦 r ( , ) 𝑝 𝑟𝐴
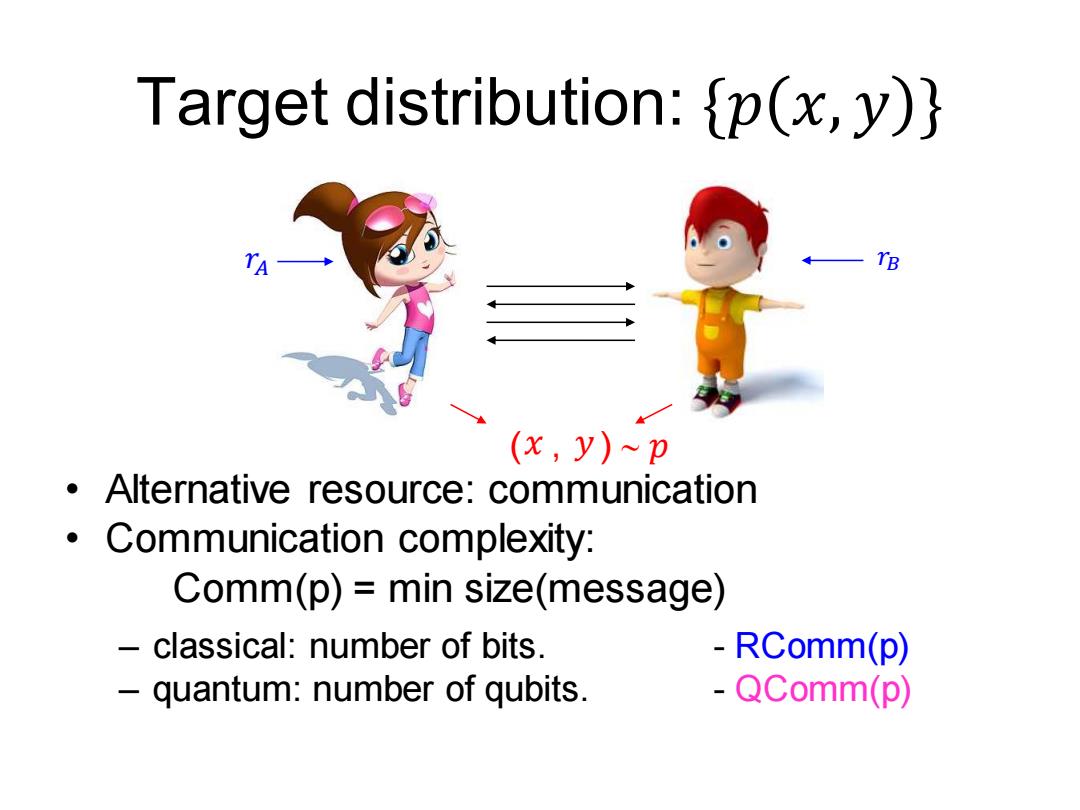
Target distribution:{p(x,y)} TB (x,y)~卫 Alternative resource:communication Communication complexity: Comm(p)=min size(message) classical:number of bits. RComm(p) quantum:number of qubits. QComm(p) -
Target distribution: {𝑝 𝑥, 𝑦 } • Alternative resource: communication • Communication complexity: Comm(p) = min size(message) – classical: number of bits. - RComm(p) – quantum: number of qubits. - QComm(p) 𝑟𝐵 (𝑥 , 𝑦 ) 𝑝 𝑟𝐴
Target distribution:p(x,y)} (x,y)~卫 Question:What are these measures? ·[Z12] 88m8=&8m8.-89 ·[Z12] R(p)=flogrank+(P)] -P=[p(x,y)]xy
Target distribution: {𝑝 𝑥, 𝑦 } • Question: What are these measures? • [Z’12] RCorr(𝑝) = RComm(𝑝). −𝑅(𝑝) QCorr(𝑝) = QComm(𝑝), −𝑄(𝑝) • [Z’12] 𝑅(𝑝) = ⌈log 𝑟𝑎𝑛𝑘+(𝑃)⌉ – 𝑃 = 𝑝 𝑥, 𝑦 𝑥,𝑦 (𝑥, 𝑦) 𝑝
R(p)=logrank+(P) For (entry-wise)nonnegative matrices,we can define more variants. ·Nonnegative rank rank(M)=minfr:M=>=1Mi,rank(Mi)=13 rank+(M)=min{r:M =k=1Mk,rank(Mk)=1,Mk =0 Extensively-studied in linear algebra and engineering.Many connections to (T)CS
𝑅(𝑝) = ⌈log 𝑟𝑎𝑛𝑘+(𝑃)⌉ • For (entry-wise) nonnegative matrices, we can define more variants. • Nonnegative rank 𝑟𝑎𝑛𝑘 𝑀 = min 𝑟: 𝑀 = σ𝑖=1 𝑟 𝑀𝑖 , 𝑟𝑎𝑛𝑘 𝑀𝑖 = 1 𝑟𝑎𝑛𝑘+ 𝑀 = min 𝑟:𝑀 = σ𝑘=1 𝑟 𝑀𝑘 , 𝑟𝑎𝑛𝑘 𝑀𝑘 = 1,𝑀𝑘 ≥ 0 • Extensively-studied in linear algebra and engineering. Many connections to (T)CS
Quantum complexity (x,y)~p ·[Z12]4 log rank(P)≤Q(p)≤min{log rank(M):MoM=P} [Z'12]3p:Q(p)=1,R(p)=logn. [LKZ'11,FMPTW'12]3p:Q(p)=0(logn),R(p)=2(n). 。[This paper] Q(p)=logrankpsa(P) Improve previous lower bound to 12 log rank(P)
Quantum complexity • [Z’12] ¼ log 𝑟𝑎𝑛𝑘(𝑃) ≤ 𝑄 𝑝 ≤ min{⌈log 𝑟𝑎𝑛𝑘(𝑀)⌉: 𝑀 ∘ 𝑀ഥ = 𝑃} • [Z’12] ∃𝑝: 𝑄 𝑝 = 1, 𝑅 𝑝 ≥ log 𝑛. [LKZ’11,FMPTW’12] ∃𝑝: 𝑄 𝑝 = 𝑂 log 𝑛 , 𝑅 𝑝 = Ω(𝑛). • [This paper] 𝑄(𝑝) = log 𝑟𝑎𝑛𝑘𝑝𝑠𝑑 𝑃 – Improve previous lower bound to ½ log 𝑟𝑎𝑛𝑘(𝑃). (𝑥, 𝑦) 𝑝

n psd-rank m ax rank(M)=minfr:Mxy (ax,by),ax,by E R"} rankpsd(M)=minfr:Mxy =(Ax,By),Ax,By E PSDrxr} o Defined in [FMPTW'12],and showed to characterize the communication complexity of the following task: Px POVM {E} Requirement: E[]=p(x,y) quantum comm flogrankpsa(P)]
psd-rank 𝑟𝑎𝑛𝑘 𝑀 = min 𝑟: 𝑀𝑥𝑦 = 𝑎𝑥 , 𝑏𝑦 , 𝑎𝑥 , 𝑏𝑦 ∈ 𝐑 𝑟 𝑟𝑎𝑛𝑘𝑝𝑠𝑑 𝑀 = min 𝑟:𝑀𝑥𝑦 = 𝐴𝑥,𝐵𝑦 , 𝐴𝑥 ,𝐵𝑦 ∈ 𝐏𝐒𝐃𝑟×𝑟 • Defined in [FMPTW’12], and showed to characterize the communication complexity of the following task: quantum comm ≈ ⌈log 𝑟𝑎𝑛𝑘𝑝𝑠𝑑(𝑃)⌉ 𝑥 𝜌𝑥 𝑃𝑂𝑉𝑀 { 𝐸𝜃 𝑦 } Requirement: 𝐄 𝜃 = 𝑝(𝑥, 𝑦) m r r n 𝑎𝑥 𝑏𝑦

Proof sketch ·[Fact灯Q(p)= min{s-rank()):purifies p} -S-rank:Schmidt-rank. Now given Py =(Cx,Dy),let A and B share |〉=∑=1lx)A1vxi)A2☒ly)B1Wy,i)B2 -)ith-row ofCxwyi):ith-column ofDy. Measuring registers (A1,B1): Pr[x,y]=∑,j=1 VxiVxj)(Wy,iwy,)=(Cx,Dy〉 o Similar for the other direction
Proof sketch • [Fact] 𝑄 𝜌 = min{S−rank(|𝜓〉): |𝜓〉 purifies 𝜌} – S-rank: Schmidt-rank. • Now given 𝑃𝑥𝑦 = 〈𝐶𝑥,𝐷𝑦〉, let A and B share 𝜓 = σ𝑖=1 𝑟 𝑥 𝐴1 𝑣𝑥,𝑖 𝐴2 ⊗ 𝑦 𝐵1 𝑤𝑦,𝑖 𝐵2 – 𝑣𝑥,𝑖 : 𝑖 𝑡ℎ -row of 𝐶𝑥, 𝑤𝑦,𝑖 : 𝑖 𝑡ℎ -column of 𝐷𝑦. • Measuring registers (𝐴1,𝐵1): Pr 𝑥, 𝑦 = σ𝑖,𝑗=1 𝑟 〈𝑣𝑥,𝑖 𝑣𝑥,𝑗 〈𝑤𝑦,𝑖 𝑤𝑦,𝑗 = 𝐶𝑥,𝐷𝑦 • Similar for the other direction