CHAPTER 13 GAMETHEORYAND COMPETITIVESTRATEGY QUESTIONS FOR REVIEW 1.What is the difference between a cooperative and a noncooperative game? Give an example ofeach. In a noncooperative game the players do not formally communicate in an effort to coordinate their actions.They are aware of one another's existence,but act independently The primary noncooperative game is that a binding contract,ie.,an agreement between the parties to which both parties must adhere,is possible in the former,but not in the latter.An example ofa cooperative game would be a formal cartel agreement,such as OPEC.or a joint venture.An example of a noncooperative game would be a race in research and development to obtain a patent. 2.What is a dominant strategy?Why is an equilibrium stable in dominant strategies? A dominant strategy is one that is best no matter what action is taken by the other party to the game.When both players have dominant strategies the outcome is stable because neither party has an incentive to change. 3.Explain the meaning of a Nash equilibrium.How does it differ from an equilibrium in dominant strategies? A Nash equilibrium isan outcome where both play believe that they are doingthe can,given the action of th game is in equilibriun if neither player has an incentive to change his or her choice,unless there is a change by the other player.The key feature that distinguishes a Nash equilibrium from an equilibrium in dominant strategies is the dependence on the opponent's behavior.An equilibrium in dominant strategies results if each player has a best choice regardless of the other player's choice.Every dominant strategy but the revere does not hold. 4.How does a Nash equilibrium differ from a game's maximin solution?In what situations is a maximin solution a more likely outcome than a Nash equilibrium? A maximin strategy is one in which each player determines the worst outcome for each of the opponent's actions and chooses the option that maximizes the minimum n that be arnd.Unlke the Nash them not require players to react to an opporent's choice. If no dominant strategy exi (in which case outcomes depend on the opponent's behavior),players can reduce the uncertainty inherent in relying on the opponent's rationality by conservatively following a maximin strategy.The maximin solution is more likely than the Nash
CHAPTER 13 GAME THEORY AND COMPETITIVE STRATEGY 一、QUESTIONS FOR REVIEW 1. What is the difference between a cooperative and a noncooperative game? Give an example of each. In a noncooperative game the players do not formally communicate in an effort to coordinate their actions. They are aware of one another’s existence, but act independently. The primary difference between a cooperative and a noncooperative game is that a binding contract, i.e., an agreement between the parties to which both parties must adhere, is possible in the former, but not in the latter. An example of a cooperative game would be a formal cartel agreement, such as OPEC, or a joint venture. An example of a noncooperative game would be a race in research and development to obtain a patent. 2. What is a dominant strategy? Why is an equilibrium stable in dominant strategies? A dominant strategy is one that is best no matter what action is taken by the other party to the game. When both players have dominant strategies, the outcome is stable because neither party has an incentive to change. 3. Explain the meaning of a Nash equilibrium. How does it differ from an equilibrium in dominant strategies? A Nash equilibrium is an outcome where both players correctly believe that they are doing the best they can, given the action of the other player. A game is in equilibrium if neither player has an incentive to change his or her choice, unless there is a change by the other player. The key feature that distinguishes a Nash equilibrium from an equilibrium in dominant strategies is the dependence on the opponent’s behavior. An equilibrium in dominant strategies results if each player has a best choice, regardless of the other player’s choice. Every dominant strategy equilibrium is a Nash equilibrium but the reverse does not hold. 4. How does a Nash equilibrium differ from a game’s maximin solution? In what situations is a maximin solution a more likely outcome than a Nash equilibrium? A maximin strategy is one in which each player determines the worst outcome for each of the opponent’s actions and chooses the option that maximizes the minimum gain that can be earned. Unlike the Nash equilibrium, the maximin solution does not require players to react to an opponent’s choice. If no dominant strategy exists (in which case outcomes depend on the opponent’s behavior), players can reduce the uncertainty inherent in relying on the opponent’s rationality by conservatively following a maximin strategy. The maximin solution is more likely than the Nash

solution in cases where there is a higher probability of irrational (non-optimizing) behavior. 5.What is a"tit-for-tat"strategy?Why is it a rational strategy for the infinitely repeated prisoners'dilemma? A player ollowing a "tit-for-tat"strategy will cooperate as bng as his or be opponent is cooperating and will switch to a noncooperative strategy if their opponent switches strategies.When the competitors assume that they will be repeating their interaction in every future period.the long-term gains from erating will outweigh anysort-tem gains from not operating Because the -tat strategy encourages cooperation in infinitely repeated games,it is rational 6.Consider a game in which the prisoners'dilemma is repeated 10 times and both players are rational and fully informed.Is a tit-for-tat strategy optimal in this case?Under what conditions would such a strategy be optimal? Since cooperation will unravel from the last period back to the first period,the "tit-for-tatstrategy is notoptimal when there is a finite number of periods and both players anticip ate the competitor's in every period G iven tha at there is response possible in the eleventh period for action in the tenth (and last)period cooperation breaks down in the last period.Then,knowing that there is no cooperation in the last period,players should maximize their self-interest by not However,if there is some doubt about whether the opponent has fully anticipated the consequences of the "tit-for-tat"strategy in the final period,the game will not unravel and the"tit-for-tat"strategy can be optimal. 7.Suppose you and your competitor are playing the pricing game shown in Table 13.8.Both of you must announce your prices at the same time.Can you rove by promising you competitor that you If the game is to be played only aw times,there is little to gain.If you are Firm and promise to announce a high price.Firm 2 will undercut you and you will end up with a payoff of-50.However,next period you will undercut too,and both fims will earn 10.If the game is played many times,there is a better chance that Fim 2 will realize that if it high p e.the long-term payoff of 50 each period is better than 10at first and 10 thereaft 8.What is meant by "first-mover advantage"?Give an example of a gaming situation with a first-mover advantage. A "first-mover"advantage can occur in a game where the first player to act receives the highest payoff.The first-mover signals his or her choice to the opponent,and the opponent must choose a response.given this signal.The first-mover goes on the offensive and the second-mover respo onds defensively.In many recreational
solution in cases where there is a higher probability of irrational (non-optimizing) behavior. 5. What is a “tit-for-tat” strategy? Why is it a rational strategy for the infinitely repeated prisoners’ dilemma? A player following a “tit-for-tat” strategy will cooperate as long as his or her opponent is cooperating and will switch to a noncooperative strategy if their opponent switches strategies. When the competitors assume that they will be repeating their interaction in every future period, the long-term gains from cooperating will outweigh any short-term gains from not cooperating. Because the “tit-for-tat” strategy encourages cooperation in infinitely repeated games, it is rational. 6. Consider a game in which the prisoners’ dilemma is repeated 10 times and both players are rational and fully informed. Is a tit -for-tat strategy optimal in this case? Under what conditions would such a strategy be optimal? Since cooperation will unravel from the last period back to the first period, the “tit-for-tat” strategy is not optimal when there is a finite number of periods and both players anticipate the competitor’s response in every period. Given that there is no response possible in the eleventh period for action in the tenth (and last) period, cooperation breaks down in the last period. Then, knowing that there is no cooperation in the last period, players should maximize their self-interest by not cooperating in the second-to-last period. This unraveling occurs because both players assume that the other player has considered all consequences in all periods. However, if there is some doubt about whether the opponent has fully anticipated the consequences of the “tit-for-tat” strategy in the final period, the game will not unravel and the “tit-for-tat” strategy can be optimal. 7. Suppose you and your competitor are playing the pricing game shown in Table 13.8. Both of you must announce your prices at the same time. Can you improve your outcome by promising your competitor that you will announce a high price? If the game is to be played only a few times, there is little to gain. If you are Firm 1 and promise to announce a high price, Firm 2 will undercut you and you will end up with a payoff of -50. However, next period you will undercut too, and both firms will earn 10. If the game is played many times, there is a better chance that Firm 2 will realize that if it matches your high price, the long-term payoff of 50 each period is better than 100 at first and 10 thereafter. 8. What is meant by “first-mover advantage”? Give an example of a gaming situation with a first-mover advantage. A “first-mover” advantage can occur in a game where the first player to act receives the highest payoff. The first-mover signals his or her choice to the opponent, and the opponent must choose a response, given this signal. The first-mover goes on the offensive and the second-mover responds defensively. In many recreational

games.from chess to football the first-mover has an advantage.In many markets the firstfirm to product canet the standard In some cases,the standard-setting power of the first mover becomes so pervasive in the market that the brand name of the product becomes synonymous with the product,e.g,"Kleenex"the name of Kleenex-brand facial tissue.is used by many consumers to refer to facial tissue ofany brand. 9.What is a "strategic move"?How can the development of a certain kind of reputation be a strategic move? A strategic move involves a commitment to reduce one's options The strateg move might not seem rational outside the context of the game in which it is played but it is rational given the anticipated response of the other player.Random responses to an opponent's action may not appear to be rational,but developing a reputation of being unpredictable oould lead to higher payoffs in the long run. Another exampl would be making a promise to give a unt to all p consumers if you give a discount toone.Such a move makes the firm vulnerabk but the goal of such a strategic move is to signal to rivals that you won't be discounting price and hope that your rivals follow suit. 10.Can the threat of a price war deter entry by potential competitors?What actions might a firm take to make this threat credible? Both the incumbent and the potential entrant know that a price war will leave their firms worse off Normally uch a threat is not crdibe.Thus the incumbent must make his or her threat ofa price war belevable by signalingto the potenti entrant that a price war will result if entry occurs.One strategic move is to increase capacity,signaling a lower future price,and another is to engage in apparently irrational behavior.Both types of strategic behavior might deter entry. but for different reasons.While an inc sein capacity reduces expected profits by reducing prices rrational behavio expected profits by increasin uncertainty,hence increasing the rate at which future profits must be discounted into the present. 11.A strategic move limits one's flexibility and yet gives one an advantage.Why? How might a strategic move give one an advantage in bargaining? A strategic move influences conditional behavior by the opponent.If the game is well understood and the reaction can be pre icted.a strategi e es the play transac a bargain, mphcit or explicit.In every bargain,we assume that both parties attempt to maximize their self-interest.Strategic moves by one plaver provide signals to which another player reacts.If a bargaining game is played only once (so no reputations are involved).the plavers might act strategically to maximize their payoffs.If bargaining is repeated,players might act strategically to establish reputations for expected negotiations 12.Why is the winner's curse potentially a problem for a bidder in a common value auction but not in a private value auction?
games, from chess to football, the first-mover has an advantage. In many markets, the first firm to introduce a product can set the standard for competitors to follow. In some cases, the standard-setting power of the first mover becomes so pervasive in the market that the brand name of the product becomes synonymous with the product, e.g., “Kleenex,” the name of Kleenex-brand facial tissue, is used by many consumers to refer to facial tissue of any brand. 9. What is a “strategic move”? How can the development of a certain kind of reputation be a strategic move? A strategic move involves a commitment to reduce one’s options. The strategic move might not seem rational outside the context of the game in which it is played, but it is rational given the anticipated response of the other player. Random responses to an opponent’s action may not appear to be rational, but developing a reputation of being unpredictable could lead to higher payoffs in the long run. Another example would be making a promise to give a discount to all previous consumers if you give a discount to one. Such a move makes the firm vulnerable, but the goal of such a strategic move is to signal to rivals that you won’t be discounting price and hope that your rivals follow suit. 10. Can the threat of a price war deter entry by potential competitors? What actions might a firm take to make this threat credible? Both the incumbent and the potential entrant know that a price war will leave their firms worse off. Normally, such a threat is not credible. Thus, the incumbent must make his or her threat of a price war believable by signaling to the potential entrant that a price war will result if entry occurs. One strategic move is to increase capacity, signaling a lower future price, and another is to engage in apparently irrational behavior. Both types of strategic behavior might deter entry, but for different reasons. While an increase in capacity reduces expected profits by reducing prices, irrational behavior reduces expected profits by increasing uncertainty, hence increasing the rate at which future profits must be discounted into the present. 11. A strategic move limits one’s flexibility and yet gives one an advantage. Why? How might a strategic move give one an advantage in bargaining? A strategic move influences conditional behavior by the opponent. If the game is well understood and the opponent’s reaction can be predicted, a strategic move leaves the player better off. Economic transactions involve a bargain, whether implicit or explicit. In every bargain, we assume that both parties attempt to maximize their self-interest. Strategic moves by one player provide signals to which another player reacts. If a bargaining game is played only once (so no reputations are involved), the players might act strategically to maximize their payoffs. If bargaining is repeated, players might act strategically to establish reputations for expected negotiations. 12. Why is the winner’s curse potentially a problem for a bidder in a common value auction but not in a private value auction?

The winner's curse states that"The winner of a common value auction is likely to be madeworseof(than notwinning)because the winner was overly optimistic and,as a consequen,bid more for the item than it was actually worth In a private value auction,you are aware of your own reservation price,and will bid accordingly Once the price has escalated above your reservation price.you will no bnger bid.If you win,it is because the winning bid was below your reservation price.In a common value auction,you do not know the exact value of the good you are bidding 0n. The winne end to be valu the good,assuming that some bidders overestimate and some underestimate.If al bids are below the actual value,then there is no winner's curse. 二、EXERCISES 1.In many oligopolistic industries,the same firms compete over a long period of time,setting prices and observing each other's behavior repeatedly.Given that the number of repetitions is large,why don't collusive outcomes typically result? If gamesar repeated indefinitely and all players know all payoffs rational behavior will lead to apparently coll ve outcomes,1.e. the same outcomes tha would result if firms were actively colluding.All payoffs.bowever.might not be known by all players.Sometimes the payoffs of other firms can only be known by engaging in extensive (and costly)information exchanges or by making a move and observing rivals'responses.Also.successful collusion encourages entry.Perhaps the greates poblem in maintaining is that change in marke conditions change the collusive price and quantity The firms then have to repeatedly change their agreement on price and quantity,which is costly.and this increases the ability of one firm to cheat without being discovered. 2.Many industries are often plagued by overcapacity-firms simultaneously make maior investments in capacity exp ansion,so total cap acity far exceeds demand.This hap t only in industries s in which de nd is highly volatile and unpredictable, but also in industries in whie h demand i fairly sta Wha factors lead to overcapacity?Explain each briefly. In Chapter 12.we found that excess capacity may arise in industries with easy entry and differentiated products.In the monopolistic competition model, downward-sloping demand curvesfor eachfirm lead to output with average cost above minim The between the res sulting outpu output at minimum long-run average cost is defined as excess capacity. n this chapter,we saw that overcapacity oould be used to deter new entry;that is, investments in capacity expansion could convince potential competitors that entry would be unprofitable.(Note that although threats of capacity expansion may deter entry.these threats must be credible. 3.Two computer firms,A and B.are planning to market network systems for office information management. Each can dev lop either a fast high-quality system (H),or a slower,low-quality system (L).Market research
The winner’s curse states that “The winner of a common value auction is likely to be made worse off (than not winning) because the winner was overly optimistic and, as a consequence, bid more for the item than it was actually worth.” In a private value auction, you are aware of your own reservation price, and will bid accordingly. Once the price has escalated above your reservation price, you will no longer bid. If you win, it is because the winning bid was below your reservation price. In a common value auction, you do not know the exact value of the good you are bidding on. The winner will tend to be the person who has most overestimated the value of the good, assuming that some bidders overestimate and some underestimate. If all bids are below the actual value, then there is no winner’s curse. 二、EXERCISES 1. In many oligopolistic industries, the same firms compete over a long period of time, setting prices and observing each other’s behavior repeatedly. Given that the number of repetitions is large, why don’t collusive outcomes typically result? If games are repeated indefinitely and all players know all payoffs, rational behavior will lead to apparently collusive outcomes, i.e., the same outcomes that would result if firms were actively colluding. All payoffs, however, might not be known by all players. Sometimes the payoffs of other firms can only be known by engaging in extensive (and costly) information exchanges or by making a move and observing rivals’ responses. Also, successful collusion encourages entry. Perhaps the greatest problem in maintaining a collusive outcome is that changes in market conditions change the collusive price and quantity. The firms then have to repeatedly change their agreement on price and quantity, which is costly, and this increases the ability of one firm to cheat without being discovered. 2. Many industries are often plagued by overcapacity-firms simultaneously make major investments in capacity expansion, so total capacity far exceeds demand. This happens not only in industries in which demand is highly volatile and unpredictable, but also in industries in which demand is fairly stable. What factors lead to overcapacity? Explain each briefly. In Chapter 12, we found that excess capacity may arise in industries with easy entry and differentiated products. In the monopolistic competition model, downward-sloping demand curves for each firm lead to output with average cost above minimum average cost. The difference between the resulting output and the output at minimum long-run average cost is defined as excess capacity. In this chapter, we saw that overcapacity could be used to deter new entry; that is, investments in capacity expansion could convince potential competitors that entry would be unprofitable. (Note that although threats of capacity expansion may deter entry, these threats must be credible.) 3. Two computer firms, A and B, are planning to market network systems for office information management. Each firm can develop either a fast, high-quality system (H), or a slower, low-quality system (L). Market research

indicates that the resulting profits to each firm for the alternative strategies are given by the following payoff matrix Firm B H H 50,40 60,45 FirmA L 55,55 15,20 a If both firms make their decisions at the same time and follow maximin (low-risk)strategies,what willthe outcome be? With a maximin strategy,a firm determines the worst outcome for each option,ther chooses the option that maximizes the payoff among the worst outcomes.If Firm A chooses H,the worst payoff would occur if Firm Bchooses H:A's payoff would be 50. If Firm A chooses L.the worst pavoff would occur if Firm B chooses L:A's pavoff would be 15.With a maximin strategy.A therefore chooses H.If Firm B cho payoff would occur ifFirm AchoosesL:the payoff would be 20.If Firr B chooses H the worst payoff,40.would oecur if Firm A chooses H.With a maximin strategy.B therefore chooses H.So under maximin,both A and B produce a high-quality system. Suppose both firms try to maximize profits,but Firm A has a head start in planning,and can commit first.Now what will the outcome be?What will the e outcome be if Firm B has a head start in planning and I can commit first? If Firm A can commit first,it will choose H because it knows that Firm B will rationally choose L.since L gives a higher payoff to B(45 vs.40).This gives Firm A a payoff of 60.If Firm A instead committed to L B would choose H (55 vs.20) giving A 55 instead of 60.If Firm B can commit first,it will choose H because it s that Firm A will rationally chooseL since L gives a higher payoff to A(55 vs 50 This gives Firm Ba payoffof 55 Getting a head start costs money (you have to gear up a large engineering team).Now consider the two-stage game in which first,each firm decides how much money to spend to speed up its planning.and second,it announces which product (H or L)it will produce.Which firm will spend mor speed planning?How will it nd?Should the other firm spend anything to speed up its planning?Explain In this game.there is an apparent advantage to being the first mover.If A move first,its profit is 60.If it moves second,its profit is 55,a difference of 5.Thus,it would be willing to spend up to 5 for the option of announcing first.On the other
indicates that the resulting profits to each firm for the alternative strategies are given by the following payoff matrix: Firm B H L H 50, 40 60, 45 Firm A L 55, 55 15, 20 a. If both firms make their decisions at the same time and follow maximin (low-risk) strategies, what will the outcome be? With a maximin strategy, a firm determines the worst outcome for each option, then chooses the option that maximizes the payoff among the worst outcomes. If Firm A chooses H, the worst payoff would occur if Firm B chooses H: A’s payoff would be 50. If Firm A chooses L, the worst payoff would occur if Firm B chooses L: A’s payoff would be 15. With a maximin strategy, A therefore chooses H. If Firm B chooses L, the worst payoff would occur if Firm A chooses L: the payoff would be 20. If Firm B chooses H, the worst payoff, 40, would occur if Firm A chooses H. With a maximin strategy, B therefore chooses H. So under maximin, both A and B produce a high-quality system. b. Suppose both firms try to maximize profits, but Firm A has a head start in planning, and can commit first. Now what will the outcome be? What will the outcome be if Firm B has a head start in planning and can commit first? If Firm A can commit first, it will choose H, because it knows that Firm B will rationally choose L, since L gives a higher payoff to B (45 vs. 40). This gives Firm A a payoff of 60. If Firm A instead committed to L, B would choose H (55 vs. 20), giving A 55 instead of 60. If Firm B can commit first, it will choose H, because it knows that Firm A will rationally choose L, since L gives a higher payoff to A (55 vs. 50). This gives Firm B a payoff of 55. c. Getting a head start costs money (you have to gear up a large engineering team). Now consider the two-stage game in which first, each firm decides how much money to spend to speed up its planning, and second, it announces which product (H or L) it will produce. Which firm will spend more to speed up its planning? How much will it spend? Should the other firm spend anything to speed up its planning? Explain. In this game, there is an apparent advantage to being the first mover. If A moves first, its profit is 60. If it moves second, its profit is 55, a difference of 5. Thus, it would be willing to spend up to 5 for the option of announcing first. On the other

hand,if B moves first,its profit is 55.If it moves second,its profit is 45,a differene of 10,and it thus would be willing topend upto10 for the option of announcing first. Once Firm A realizes that Firm B is willing to spend something on the option of announcing first,then the value of the option decreases for Firm A.because if both firms were to invest both firms would choose to produce the high-quality system. which gives them both a lower pavoff.Firm A should not spend money to speed up the introduction of its product in any case if b goes first and chooses high then A instead of the50it ent high also This is best for B also because if B goes high and A low,Bends up with 55 instead of 40.so even ifit spends the 10 it is still ahead by 5.A should let B go first.Finally. note that even though B is better off if A goes first and chooses high(45 vs.40).B is still best off if it es first.Overall it is worthwhile for B to spend the money and announce but it is not worthwhile for A tospend any money 4.Two firms are in the chocolate market.Each can choose togo for the high end of the market (high quality)or the low end (low quality). Resulting profits are given by the following payoff matrix: Firm2 High Low 20,30 900,600 Firm 1 High 100,800 50,50 What outcomes,if any,are Nash equilibria? A Nash equilibrium exists when neither party has an inentive to alter its strategy,taking the other's strategy as given.If Firm 2 chooses Low and Firm I chooses High,neither will have an incentive to change (100>20 for Firm 1 and 800>50 for Firm 2).If Firm 2 chooses High and Firm 1 chooses low.neither will have an incentive to change (900>50 for Firm 1 and 600>30 for Firm 2).Both outoomes are Nash equilibria.Both firms choosing low is nota Nash equilibrium because,for example,if Firm chooses low then firm 2 is better off by switching to high since 600 is greater than -30. If the manager of each firm is conservative and each follows a maximin strategy,what will be the outcome? If Firm 1 chooses Low.its worst pavoff-20.would occur if Firm 2 chooses Low.If Firm 1 chooses High its worst payoff 50,would occur if Firm chooses High. .Therefore,with a conservative maximin strategy,Firm l
hand, if B moves first, its profit is 55. If it moves second, its profit is 45, a difference of 10, and it thus would be willing to spend up to 10 for the option of announcing first. Once Firm A realizes that Firm B is willing to spend something on the option of announcing first, then the value of the option decreases for Firm A, because if both firms were to invest both firms would choose to produce the high-quality system, which gives them both a lower payoff. Firm A should not spend money to speed up the introduction of its product in any case. If B goes first and chooses high then A can choose low and end up with 55 instead of the 50 it would get if it went high also. This is best for B also because if B goes high and A low, B ends up with 55 instead of 40, so even if it spends the 10 it is still ahead by 5. A should let B go first. Finally, note that even though B is better off if A goes first and chooses high (45 vs. 40), B is still best off if it goes first. Overall, it is worthwhile for B to spend the money and announce but it is not worthwhile for A to spend any money. 4. Two firms are in the chocolate market. Each can choose to go for the high end of the market (high quality) or the low end (low quality). Resulting profits are given by the following payoff matrix: Firm 2 Low High Low -20, -30 900, 600 Firm 1 High 100, 800 50, 50 a. What outcomes, if any, are Nash equilibria? A Nash equilibrium exists when neither party has an incentive to alter its strategy, taking the other’s strategy as given. If Firm 2 chooses Low and Firm 1 chooses High, neither will have an incentive to change (100 > -20 for Firm 1 and 800 > 50 for Firm 2). If Firm 2 chooses High and Firm 1 chooses Low, neither will have an incentive to change (900 > 50 for Firm 1 and 600 > -30 for Firm 2). Both outcomes are Nash equilibria. Both firms choosing low is not a Nash equilibrium because, for example, if Firm 1 chooses low then firm 2 is better off by switching to high since 600 is greater than -30. b. If the manager of each firm is conservative and each follows a maximin strategy, what will be the outcome? If Firm 1 chooses Low, its worst payoff, -20, would occur if Firm 2 chooses Low. If Firm 1 chooses High, its worst payoff, 50, would occur if Firm 2 chooses High. Therefore, with a conservative maximin strategy, Firm 1
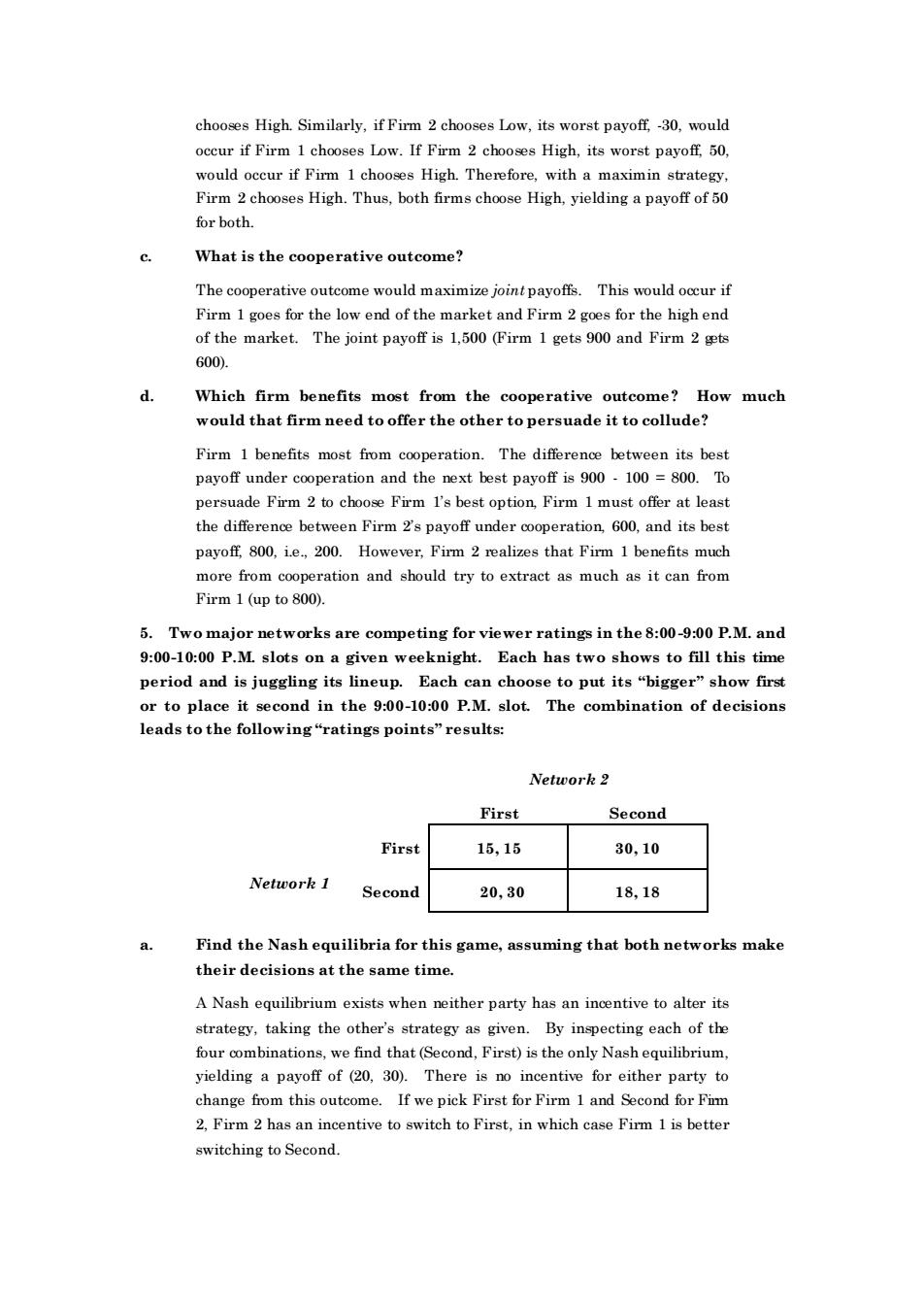
chooses High.Similarly,if Firm 2 chooses Low,its worst payoff,-30,would occur if Firm 1 chooses Low.If Firm 2 chooses High,its worst payoff50. would oceur if Firm I chooses High.Therefore, with a maximin strategy Firm 2 chooses High.Thus,both firms choose High,vielding a payoff of 50 for both. What is the cooperative outcome? The cooperative outcome would maximize joint payoffs.This would occur if Firm 1goes for the low end of the market and Firm 2goes for the highend The payoff is 1.500 (Firm 1gets 90 and Firm 2 gts G00 Which firm benefits most from the cooperative outcome? How much would that firm need to offer the other to persuade it to collude? Firm 1 benefits most from cooperation.The difference between its best payoff under cooperation and the next best payoff is 900.100 =800.To persuade Firm 2 to cboose firm l's best option.Firm 1 must offer at least the differen between Firm2s payof under its best payoff 800.However,Firm 2 realizes that Firm 1 benefits much more from cooperation and should try to extract as much as it can from Firm 1 (up to 800). 5.Two major networks are competing for viewer ratings in the8:00-9:00 P.M.and 9:00-10:00 P.M.slots on a given weeknight.Each has two shows to fill this time period and is juggling its lin Each can choose to put its"bigger"show first or to plac it second in the:0-10:00P.M. slot. The combination of decisions leads to the following"ratings points"results Netuork 2 First Second First 15,15 30,10 Second 20,30 18,18 Find the Nash equilibria for this game,assuming that both networks make their decisions at the same time A Nash equilibrium exists when neither party has an incentive to alter its strategy,taking the other's strategy as given.By inspecting each of the four combinations,we find that(Second,First)is the only Nash equilibrium. yielding a payoff of (20,30).There is no incentive for either party to change from this outcome.If we pick First for Firm 1 and Second for Fim 2.Firm 2 has an incentive to switch to First.in which case Firm 1is better switching to Second
chooses High. Similarly, if Firm 2 chooses Low, its worst payoff, -30, would occur if Firm 1 chooses Low. If Firm 2 chooses High, its worst payoff, 50, would occur if Firm 1 chooses High. Therefore, with a maximin strategy, Firm 2 chooses High. Thus, both firms choose High, yielding a payoff of 50 for both. c. What is the cooperative outcome? The cooperative outcome would maximize joint payoffs. This would occur if Firm 1 goes for the low end of the market and Firm 2 goes for the high end of the market. The joint payoff is 1,500 (Firm 1 gets 900 and Firm 2 gets 600). d. Which firm benefits most from the cooperative outcome? How much would that firm need to offer the other to persuade it to collude? Firm 1 benefits most from cooperation. The difference between its best payoff under cooperation and the next best payoff is 900 - 100 = 800. To persuade Firm 2 to choose Firm 1’s best option, Firm 1 must offer at least the difference between Firm 2’s payoff under cooperation, 600, and its best payoff, 800, i.e., 200. However, Firm 2 realizes that Firm 1 benefits much more from cooperation and should try to extract as much as it can from Firm 1 (up to 800). 5. Two major networks are competing for viewer ratings in the 8:00-9:00 P.M. and 9:00-10:00 P.M. slots on a given weeknight. Each has two shows to fill this time period and is juggling its lineup. Each can choose to put its “bigger” show first or to place it second in the 9:00-10:00 P.M. slot. The combination of decisions leads to the following “ratings points” results: Network 2 First Second First 15, 15 30, 10 Network 1 Second 20, 30 18, 18 a. Find the Nash equilibria for this game, assuming that both networks make their decisions at the same time. A Nash equilibrium exists when neither party has an incentive to alter its strategy, taking the other’s strategy as given. By inspecting each of the four combinations, we find that (Second, First) is the only Nash equilibrium, yielding a payoff of (20, 30). There is no incentive for either party to change from this outcome. If we pick First for Firm 1 and Second for Firm 2, Firm 2 has an incentive to switch to First, in which case Firm 1 is better switching to Second

If each network is risk averse and uses a maximin strategy,what will be the resultingequilibrium? This strategy of minimizing the maximum loss focuseso good outcomes. If Network 1 plays First,the worst payoff is 15.If Network 1 plavs Second.the worst pavoff is 18.Under maximin Network 1 plavs Second.If Network 2 plays First,the worst payoff is 15.If Network 2 plays Second,the worst payoff is 10.Under maximin,Network 2 plays First (Thisa dom The aximin equilibr un is (Se First)with a payoff of(20.30). What will be the equilibrium if Network I can makes its selection first?If Network 2 goes first? If Network 1plays First,Network 2 will play First,yielding 15 for Network 1.If Network 1 plays Second,Network 2 will play First.yielding 20 for Network 1.Therefore,if it has the first move,Network 1will play nd the ing equilibrium will be Second,Fir If N First,Network 1 will play Second,yielding 30 for Network 2.If Network plays Second,Network 1 will play First,yielding 10 for Network 2.If it has the first move,Network 2 will play First,and the equilibrium will again be (Second.First). Suppose the network managers meet to coordinate schedules and Network mises to schedule its big show first.Is this promise credible?What would be the likely outcome? A move is credible if,once declared,there is no incentive to change.If Network I goes first.then Network 2 will also want to go first which gives them both 15.In this case,once Network 1 knows that Network 2 also wants to go first.Network 1 will wants to change its strategy to Second.In this case,the promise to schedule the bigg show first is not redibhk Network 2 schedule its bigger show Firs strategy and the coordinated outcome is likely to be(Second,First)
b. If each network is risk averse and uses a maximin strategy, what will be the resulting equilibrium? This conservative strategy of minimizing the maximum loss focuses on limiting the extent of the worst possible outcome, to the exclusion of possible good outcomes. If Network 1 plays First, the worst payoff is 15. If Network 1 plays Second, the worst payoff is 18. Under maximin, Network 1 plays Second. If Network 2 plays First, the worst payoff is 15. If Network 2 plays Second, the worst payoff is 10. Under maximin, Network 2 plays First (This is a dominant strategy). The maximin equilibrium is (Second, First) with a payoff of (20,30). c. What will be the equilibrium if Network 1 can makes its selection first? If Network 2 goes first? If Network 1 plays First, Network 2 will play First, yielding 15 for Network 1. If Network 1 plays Second, Network 2 will play First, yielding 20 for Network 1. Therefore, if it has the first move, Network 1 will play Second, and the resulting equilibrium will be (Second, First). If Network 2 plays First, Network 1 will play Second, yielding 30 for Network 2. If Network 2 plays Second, Network 1 will play First, yielding 10 for Network 2. If it has the first move, Network 2 will play First, and the equilibrium will again be (Second, First). d. Suppose the network managers meet to coordinate schedules and Network 1 promises to schedule its big show first. Is this promise credible? What would be the likely outcome? A move is credible if, once declared, there is no incentive to change. If Network 1 goes first, then Network 2 will also want to go first which gives them both 15. In this case, once Network 1 knows that Network 2 also wants to go first, Network 1 will wants to change its strategy to Second. In this case, the promise to schedule the bigger show first is not credible. Network 2 will schedule its bigger show First since this is a dominant strategy and the coordinated outcome is likely to be (Second,First)
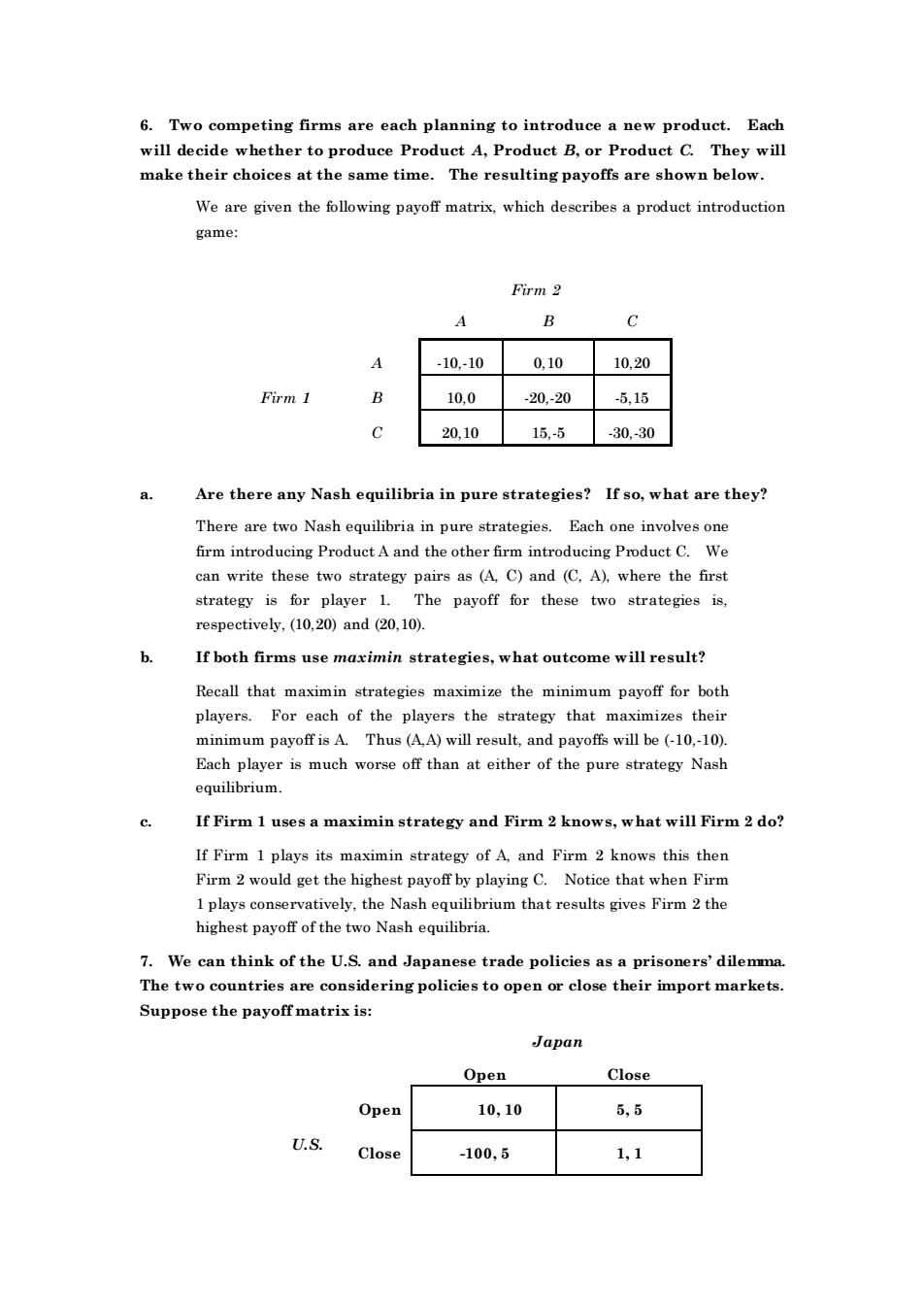
6.Two competing firms are each planning to introduce a new product.Each will decide whether to produce Produet A,Product B.or Product C.They will make their choices at the same time.The resulting payoffs are shown below. We are given the following payoff matrix.which describes a product introduction game: Firm? 4 B -10-10 0,1010,20 Firm 1 10.0 20,-205,15 20.10 15.5 .30.-30 Are there any Nash equilibria in pure strategies?If so,what are they? There are Nash in pure strategies.Each one invalve one firm introducing Product A and the other firm introdueing ProductC. we can write these two strategy pairs as (A.C)and (C.A).where the first strategy is for player 1.The payoff for these two strategies is, respectively.(10.20)and (20.10). If both firms use maximin strategies,what outcome will result? Recall that maximin strategies maximize the minimum payoff for both players each of the ers strategy that izes heir minimum payoff is A.Thus (A.A)will result,and payoffs will be (-10.-10) Each player is much worse off than at either of the pure strategy Nash equilibrium. c. If Firm 1 uses a maximin strategy and Firm 2 knows,what will Firm 2 do? If Firm 1 plays its maximin strategy of A.and Firm 2 knows this then Firm 2 would get the highest payoff by plaving c.Notice that when firm tively,the Nash equilibrium that results givesFirm 2 the highest payo of the two 7.We can think of the U.S.and Japanese trade policies as a prisoners'dilemma The two countries are considering policies to open or close their import markets. Suppose the payoffmatrix is: JJapan Open Close Open 10.10 5,5 U.S. Close -100.5 1,1
6. Two competing firms are each planning to introduce a new product. Each will decide whether to produce Product A, Product B, or Product C. They will make their choices at the same time. The resulting payoffs are shown below. We are given the following payoff matrix, which describes a product introduction game: Firm 2 A B C A -10,-10 0,10 10,20 Firm 1 B 10,0 -20,-20 -5,15 C 20,10 15,-5 -30,-30 a. Are there any Nash equilibria in pure strategies? If so, what are they? There are two Nash equilibria in pure strategies. Each one involves one firm introducing Product A and the other firm introducing Product C. We can write these two strategy pairs as (A, C) and (C, A), where the first strategy is for player 1. The payoff for these two strategies is, respectively, (10,20) and (20,10). b. If both firms use maximin strategies, what outcome will result? Recall that maximin strategies maximize the minimum payoff for both players. For each of the players the strategy that maximizes their minimum payoff is A. Thus (A,A) will result, and payoffs will be (-10,-10). Each player is much worse off than at either of the pure strategy Nash equilibrium. c. If Firm 1 uses a maximin strategy and Firm 2 knows, what will Firm 2 do? If Firm 1 plays its maximin strategy of A, and Firm 2 knows this then Firm 2 would get the highest payoff by playing C. Notice that when Firm 1 plays conservatively, the Nash equilibrium that results gives Firm 2 the highest payoff of the two Nash equilibria. 7. We can think of the U.S. and Japanese trade policies as a prisoners’ dilemma. The two countries are considering policies to open or close their import markets. Suppose the payoff matrix is: Japan Open Close Open 10, 10 5, 5 U.S. Close -100, 5 1, 1
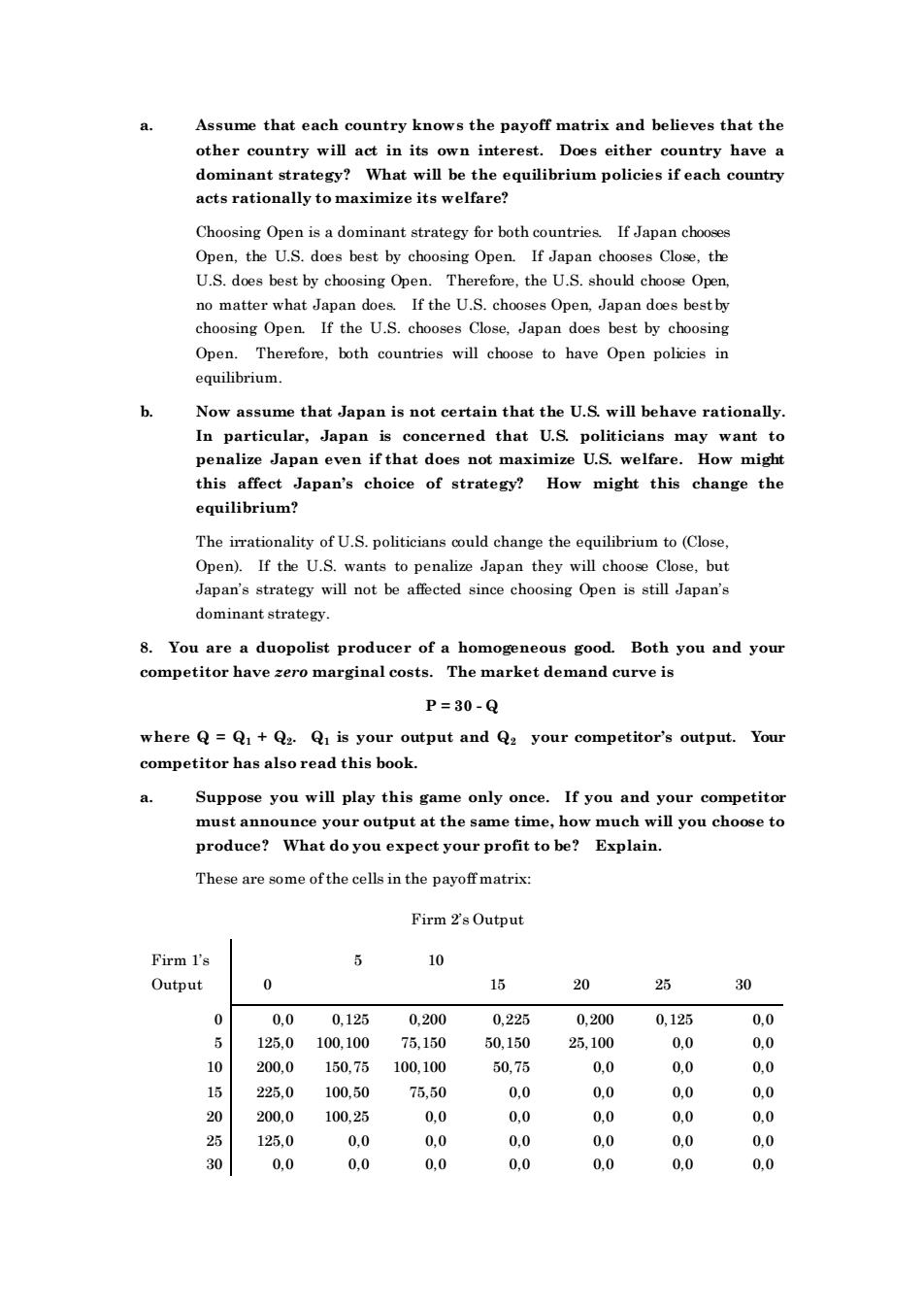
Assume that each country knows the payoff matrix and believes that the other country will act in its own interest.Does either country havea dominant strategy? What will be the equilibrium policies if each country acts rationally to maximize its welfare? Choosing Open is a dominant strategy for both countries.If Japan chooses Open,the U.S.does best by choosing Open.If Japan chooses Close,the U.S.does best by choosing Open.Therefore.the U.S.should choose Open no matter what Japan does If the U.S.chooses Open,Japan does bes best by choo Open.Therefore,both countries will choose to have Open policies in equilibrium. Now assume that Japan is not certain that the U.S.will behave rationally. In particular.Japan is concerned that U.S politicians may want to penalie Japan even if that does not maximize U.S welfare.Howm this affect Japan's choice of strategy?How might this change the equilibrium? The irrationality of U.S.politicians could change the equilibrium to (Close Open).If the U.S.wants to penalize Japan they will choose Close,but Japan's strategy will not be affected since choosing Open is still Japan's dominant strategy. You are a duopolist producer of a hor eous go ood.Both you and vour competitor ha ve zero marginal costs.The market demand curve is P=30-Q where Q=Q+Qz.Qi is y output and Q your competitor's output.Your competitor has also read this book Suppose you will play this game only once.If you and your competitor must announce your output at the same time,how much will you choose to produce?What do you expect your profit to be?Explain. These are some of the cells in the payoff matrix: Firm 2's Output Firm I's 5 10 Output 0 15 20 0 0 0125 0,200 0,225 0,200 0,125 0,0 125.0 100,100 75.150 50.150 25,100 0.0 10 200.0 150,.75 100,100 50.75 0.0 0.0 0.0 76,50 0 00 125,0 0,0 00 0,0 00 0,0 0,0 0.0 0,0 0,0 0.0 00
a. Assume that each country knows the payoff matrix and believes that the other country will act in its own interest. Does either country have a dominant strategy? What will be the equilibrium policies if each country acts rationally to maximize its welfare? Choosing Open is a dominant strategy for both countries. If Japan chooses Open, the U.S. does best by choosing Open. If Japan chooses Close, the U.S. does best by choosing Open. Therefore, the U.S. should choose Open, no matter what Japan does. If the U.S. chooses Open, Japan does best by choosing Open. If the U.S. chooses Close, Japan does best by choosing Open. Therefore, both countries will choose to have Open policies in equilibrium. b. Now assume that Japan is not certain that the U.S. will behave rationally. In particular, Japan is concerned that U.S. politicians may want to penalize Japan even if that does not maximize U.S. welfare. How might this affect Japan’s choice of strategy? How might this change the equilibrium? The irrationality of U.S. politicians could change the equilibrium to (Close, Open). If the U.S. wants to penalize Japan they will choose Close, but Japan’s strategy will not be affected since choosing Open is still Japan’s dominant strategy. 8. You are a duopolist producer of a homogeneous good. Both you and your competitor have zero marginal costs. The market demand curve is P = 30 - Q where Q = Q1 + Q2 . Q1 is your output and Q2 your competitor’s output. Your competitor has also read this book. a. Suppose you will play this game only once. If you and your competitor must announce your output at the same time, how much will you choose to produce? What do you expect your profit to be? Explain. These are some of the cells in the payoff matrix: Firm 2’s Output Firm 1’s Output 0 5 10 15 20 25 30 0 0,0 0,125 0,200 0,225 0,200 0,125 0,0 5 125,0 100,100 75,150 50,150 25,100 0,0 0,0 10 200,0 150,75 100,100 50,75 0,0 0,0 0,0 15 225,0 100,50 75,50 0,0 0,0 0,0 0,0 20 200,0 100,25 0,0 0,0 0,0 0,0 0,0 25 125,0 0,0 0,0 0,0 0,0 0,0 0,0 30 0,0 0,0 0,0 0,0 0,0 0,0 0,0