CHAPTER 23 PROSPECT THEORY
CHAPTER 23 PROSPECT THEORY
Modeling decisions under uncertainty Pervasiveness of uncertainty Cards and gambling:raise?or fold? Career decisions:which major?which career? Insurance choices:buy insurance or go uninsured? How does eating this donut affect the likelihood of heart attack? Which disease treatment to pursue:the cheaper but higher risk one?Or the certain but more expensive option? Bhattacharya,Hyde and Tu-HealthEconomics
Bhattacharya, Hyde and Tu – Health Economics Modeling decisions under uncertainty Pervasiveness of uncertainty Cards and gambling: raise? or fold? Career decisions: which major? which career? Insurance choices: buy insurance or go uninsured? How does eating this donut affect the likelihood of heart attack? Which disease treatment to pursue: the cheaper but higher risk one? Or the certain but more expensive option?
Modeling decisions under uncertainty Consider a"lottery"with uncertain prospects: Option Probability P Option x2 Probability P2 Option X3 Probability P3 … Option XN Probability Pn How should such a lottery be valued? Valuation Function: V(A)=V(x1,Pi x2,p2i...;XN,Pn) Preferred lotteries yield higher values Bhattacharya,Hyde and Tu-HealthEconomics
Bhattacharya, Hyde and Tu – Health Economics Modeling decisions under uncertainty Consider a “lottery” with uncertain prospects: Option x1 Probability p1 Option x2 Probability p2 Option x3 Probability p3 … … Option xN Probability pn How should such a lottery be valued? Valuation Function: V(A) = V(x1 , p1 ; x2 , p2 ; … ; xN , pn ) Preferred lotteries yield higher values
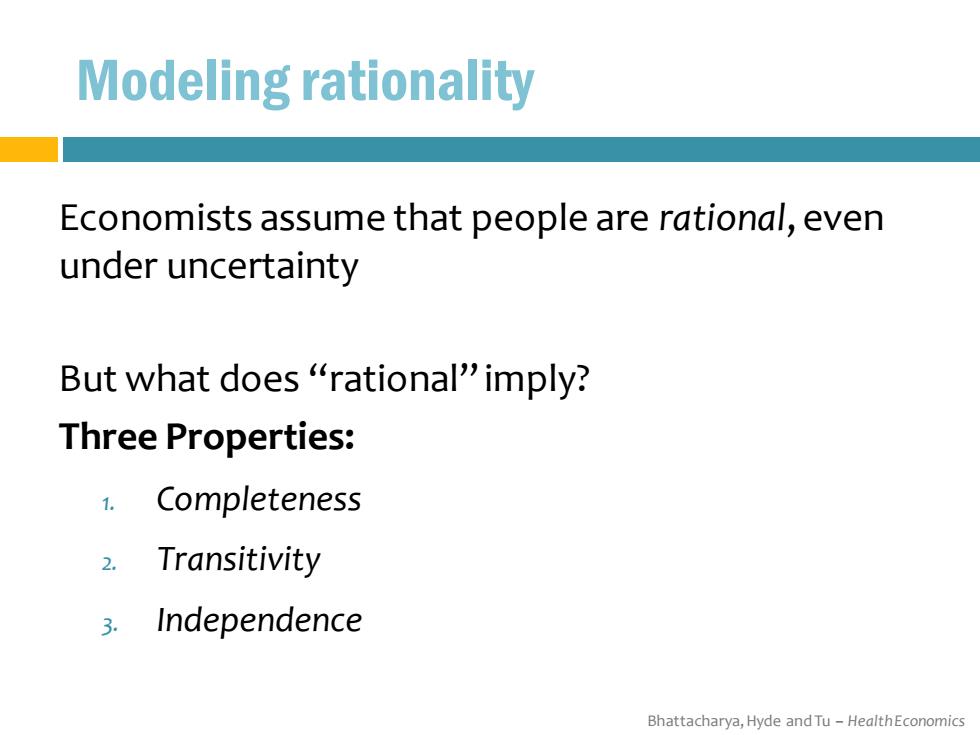
Modeling rationality Economists assume that people are rational,even under uncertainty But what does "rational"imply? Three Properties: 1.Completeness 2.Transitivity 3.Independence Bhattacharya,Hyde and Tu-HealthEconomics
Bhattacharya, Hyde and Tu – Health Economics Modeling rationality Economists assume that people are rational, even under uncertainty But what does “rational” imply? Three Properties: 1. Completeness 2. Transitivity 3. Independence

Modeling rationality:three properties 1.Completeness Between any two options,a decision can always be made. i.e.Either V(A)>V(B) V(A)<V(B) V(A)=V(B) Completeness rules out indecision(but not indifference) 2.Transitivity 3.Independence Bhattacharya,Hyde and Tu-HealthEconomics
Bhattacharya, Hyde and Tu – Health Economics Modeling rationality: three properties 1. Completeness Between any two options, a decision can always be made. i.e. Either V(A) > V(B) V(A) < V(B) V(A) = V(B) Completeness rules out indecision (but not indifference) 2. Transitivity 3. Independence
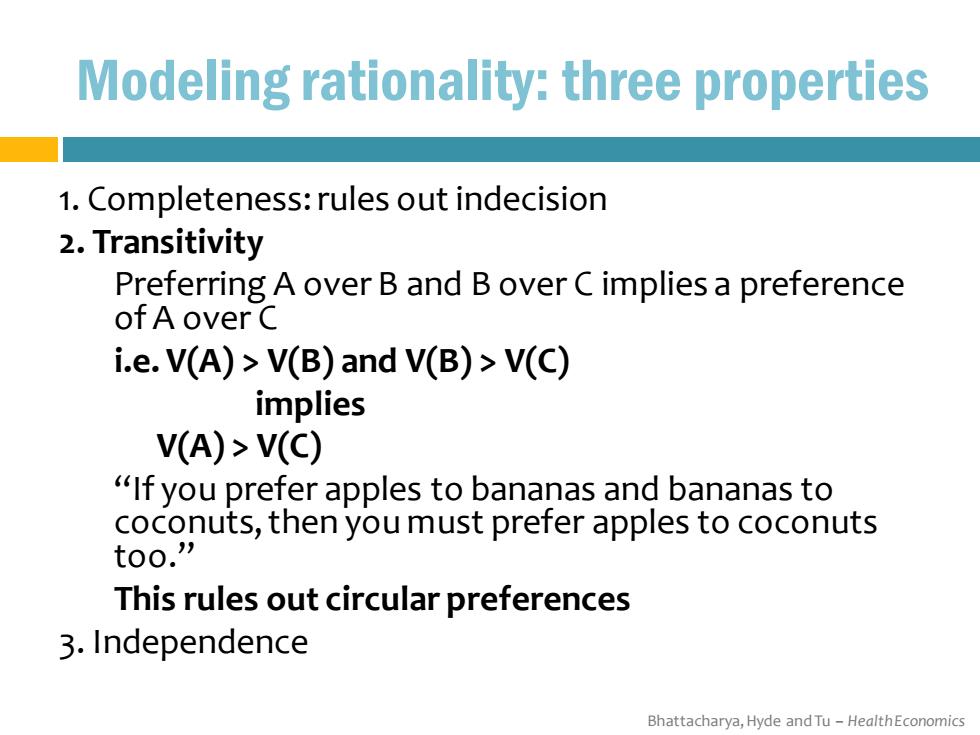
Modeling rationality:three properties 1.Completeness:rules out indecision 2.Transitivity Preferring A over B and B over C implies a preference of A over C i.e.V(A)>V(B)and V(B)>V(C) implies V(A)>V(C) "If you prefer apples to bananas and bananas to coconuts,then you must prefer apples to coconuts t00.” This rules out circular preferences 3.Independence Bhattacharya,Hyde and Tu-HealthEconomics
Bhattacharya, Hyde and Tu – Health Economics Modeling rationality: three properties 1. Completeness: rules out indecision 2. Transitivity Preferring A over B and B over C implies a preference of A over C i.e. V(A) > V(B) and V(B) > V(C) implies V(A) > V(C) “If you prefer apples to bananas and bananas to coconuts, then you must prefer apples to coconuts too.” This rules out circular preferences 3. Independence
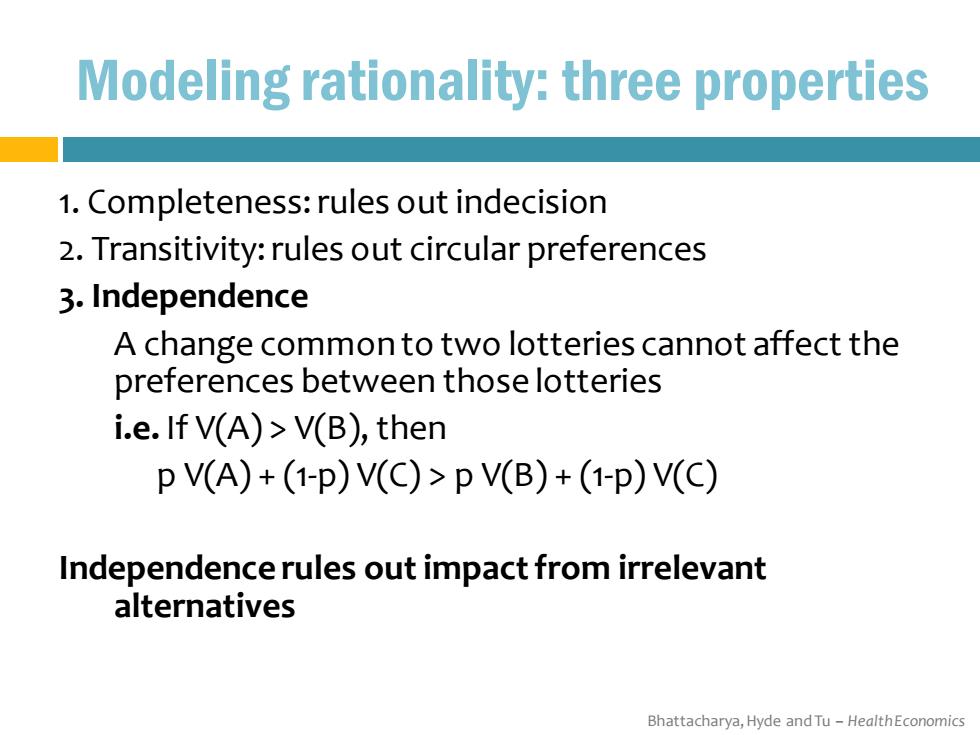
Modeling rationality:three properties 1.Completeness:rules out indecision 2.Transitivity:rules out circular preferences 3.Independence A change common to two lotteries cannot affect the preferences between those lotteries i.e.If V(A)>V(B),then P V(A)+(1-p)V(C)>p V(B)+(1-p)V(C) Independence rules out impact from irrelevant alternatives Bhattacharya,Hyde and Tu-HealthEconomics
Bhattacharya, Hyde and Tu – Health Economics Modeling rationality: three properties 1. Completeness: rules out indecision 2. Transitivity: rules out circular preferences 3. Independence A change common to two lotteries cannot affect the preferences between those lotteries i.e. If V(A) > V(B), then p V(A) + (1-p) V(C) > p V(B) + (1-p) V(C) Independence rules out impact from irrelevant alternatives

Expected utility theory The only Valuation Function satisfying completeness,transitivity,independence is the expected utility function V(A)=V(X1,Pi X2,P2j...;XN,PN) PU(X)+P2U(X2)+...+PN U(XN) =[U(A)] This is what we assumed when we studied health insurance models! Bhattacharya,Hyde and Tu-HealthEconomics
Bhattacharya, Hyde and Tu – Health Economics Expected utility theory The only Valuation Function satisfying completeness, transitivity, & independence is the expected utility function V(A) = V(x1 , p1 ; x2 , p2 ; … ; xN , pN ) = p1U(x1 ) + p2U(x2 ) + … + pN U(xN ) = E[ U(A) ] This is what we assumed when we studied health insurance models!

Bounded rationality But cognitive powers may be bounded Our decisions not always consistent with the three properties of rationality Two psychologists,Daniel Kahneman and Amos Tversky,posed questions to students in a lab and found inconsistencies Kahneman was awarded the 2002 Nobel Prize in Economics(Tversky passed away before 2002) Bhattacharya,Hyde and Tu-HealthEconomics
Bhattacharya, Hyde and Tu – Health Economics Bounded rationality But cognitive powers may be bounded Our decisions not always consistent with the three properties of rationality Two psychologists, Daniel Kahneman and Amos Tversky, posed questions to students in a lab and found inconsistencies Kahneman was awarded the 2002 Nobel Prize in Economics (Tversky passed away before 2002)

Kahneman and Tverky's approach Kahneman and Tversky(1979)surveyed Israeli university students about decisions under uncertainty. These questions offered respondents different hypothetical lotteries to win Israeli money The inconsistencies they find with expected utility theory motivated their alternative theory of decisions under uncertainty:Prospect Theory Bhattacharya,Hyde and Tu-HealthEconomics
Bhattacharya, Hyde and Tu – Health Economics Kahneman and Tverky’s approach Kahneman and Tversky (1979) surveyed Israeli university students about decisions under uncertainty. These questions offered respondents different hypothetical lotteries to win Israeli money The inconsistencies they find with expected utility theory motivated their alternative theory of decisions under uncertainty: Prospect Theory