
计算机问题求解一论题2-15 -红黑树 2020年09月08日
计算机问题求解 – 论题2-15 - 红黑树 2020年09月08日

Red-Black Property 我们为什么需要红黑树这么一种特别的数据结构? 1.Every node is either red or black. 2.The root is black. 3.Every leaf (NIL)is black. 4.If a node is red,then both its children are black. 5.For each node,all simple paths from the node to descendant leaves contain the same number of black nodes
Red-Black Property 我们为什么需要红黑树这么一种特别的数据结构?
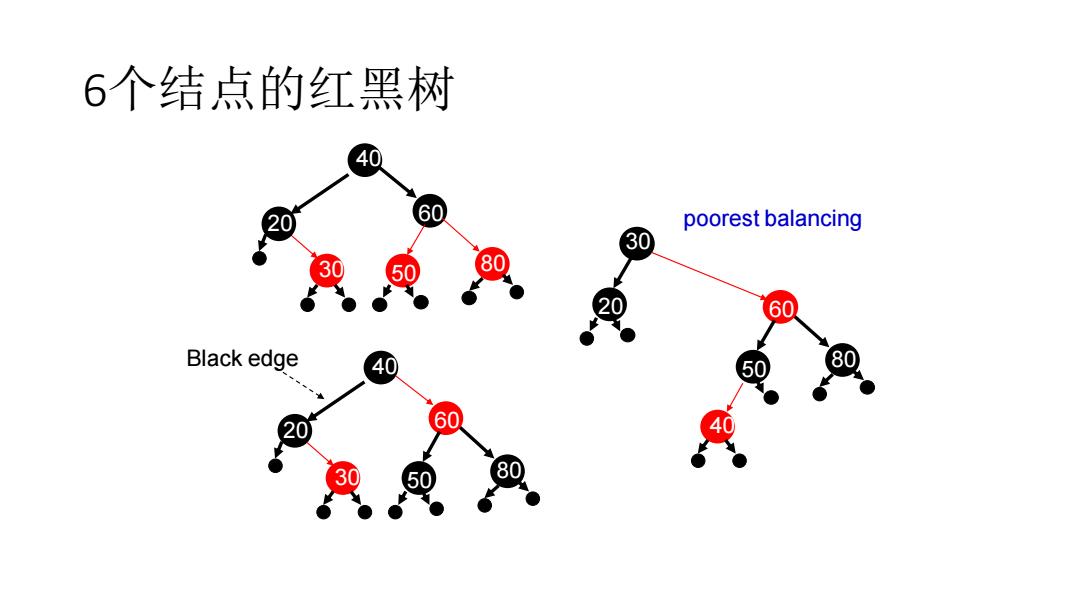
6个结点的红黑树 20 60 poorest balancing 30 60 Black edge
6个结点的红黑树 40 60 80 30 50 20 40 30 80 60 50 20 30 20 80 60 40 50 poorest balancing Black edge

范例 3 26 41 14 2 21 30 47 10 16 19 23 28 38 NIL NIL NIL NIL 20 NIL NIL NIL NIL 39 NIL NIL NIL NIL NIL NIL NIL NIL NIL NIL NIL N NIL (a) 什么是黑高?黑高的定义是well define的吗?
范例
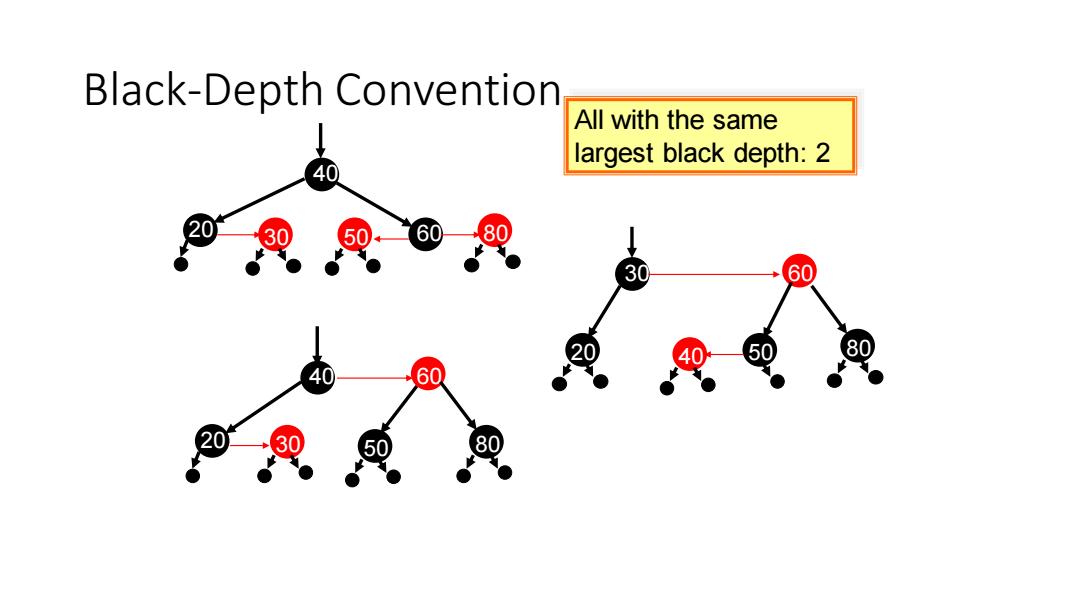
Black-Depth Convention All with the same largest black depth:2 40 0 60 80 30 60 40 60
Black-Depth Convention 40 30 50 80 20 20 40 50 30 60 40 20 30 50 60 80 60 80 All with the same largest black depth: 2
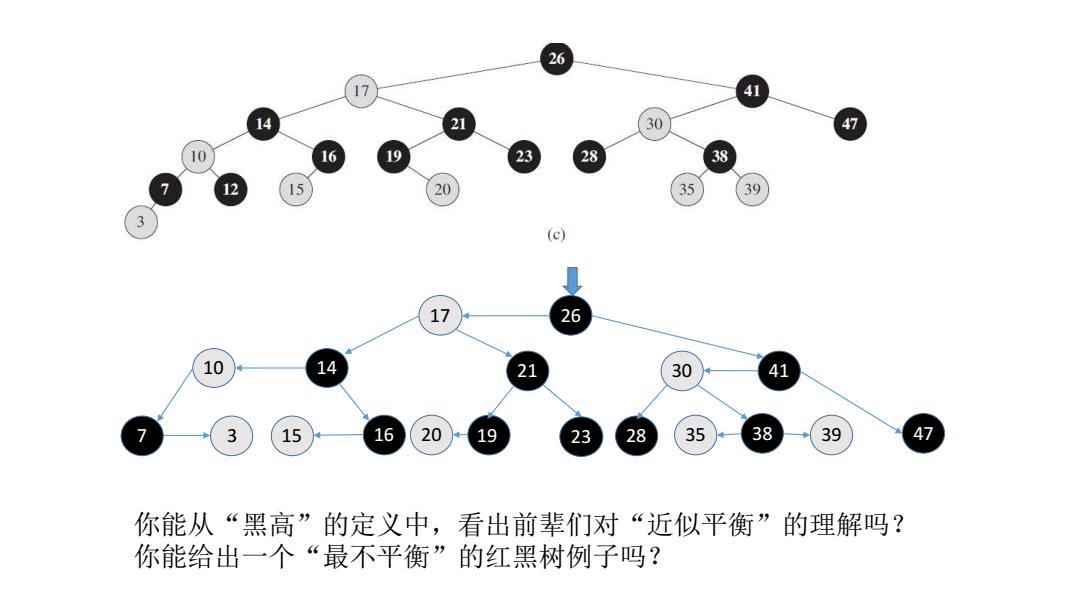
26 41 21 30 47 19 23 38 20 35 39 (c) 17 26 10 14 21 30 41 3 15 16 20 28 35 38 39 47 你能从“黑高”的定义中,看出前辈们对“近似平衡”的理解吗? 你能给出一个“最不平衡”的红黑树例子吗?
26 41 17 14 7 16 38 47 21 19 23 28 30 15 10 3 20 35 39 你能从“黑高”的定义中,看出前辈们对“近似平衡”的理解吗? 你能给出一个“最不平衡”的红黑树例子吗?
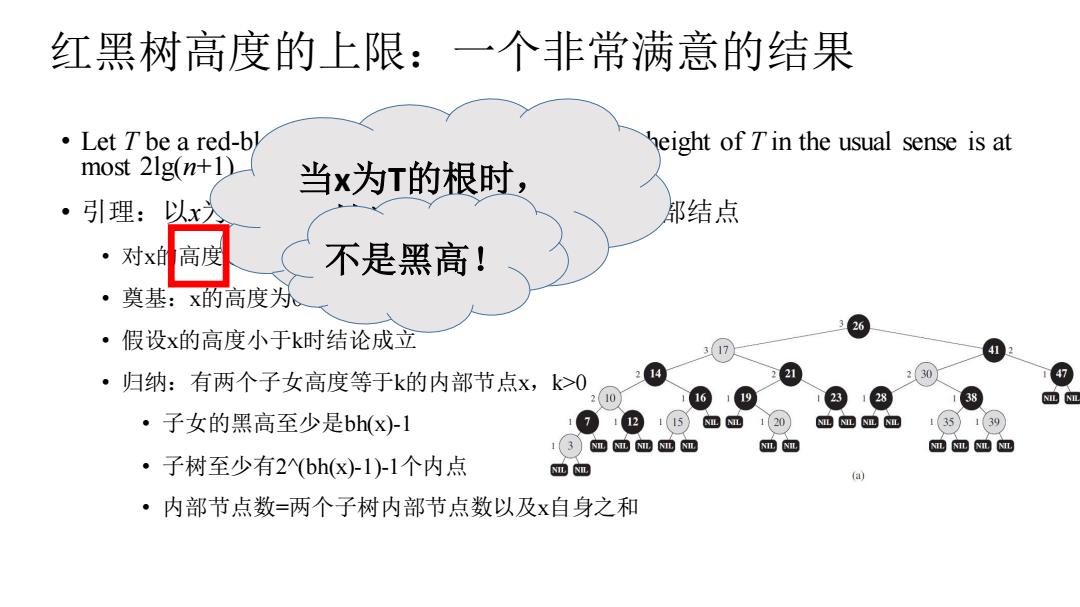
红黑树高度的上限:一个非常满意的结果 ·Let Tbe a red-bh height of Tin the usual sense is at most 21g(n+1) 当x为T的根时, ·引理:以x 邰结点 ·对x日 高度 不是黑高! ·奠基:x的高度为 ·假设x的高度小于k时结论成立 17 ·归纳:有两个子女高度等于k的内部节点x,k>0 230 47 2(10 119 128 NIL NIL ·子女的黑高至少是bh(x)-1 1(15m120 NIL NILNIL NID 1(35)1(39 m AI N10 NIL NR ·子树至少有2(bh(x)-1)1个内点 NINI (a) ·内部节点数=两个子树内部节点数以及x自身之和
红黑树高度的上限:一个非常满意的结果 • Let T be a red-black tree with n internal nodes,the height of T in the usual sense is at most 2lg(n+1). • 引理:以x为根的红黑树子树至少包含2 bh(x) -1个内部结点 • 对x的高度进行归纳 • 奠基:x的高度为0时:x是Nil,正确 • 假设x的高度小于k时结论成立 • 归纳:有两个子女高度等于k的内部节点x,k>0 • 子女的黑高至少是bh(x)-1 • 子树至少有2^(bh(x)-1)-1个内点 • 内部节点数=两个子树内部节点数以及x自身之和 当x为T的根时, 情况如何? 不是黑高!

当x就是树的根T时: ·树的内部节点数n>=2bh(T)-1 。与此同时: bh(T)和T的高h是什么关系? ·h=2bhT)-1>=2h/2-1 问题: h<=2Ig(n+1):这个结论有 什么意义?
当x就是树的根T时: • 树的内部节点数 n>=2bh(T) -1 • 与此同时: • h= 2bh(T) -1>= 2h/2 -1 bh(T)和T的高h是什么关系?

问题: 一棵二叉搜索树,如果平衡性不够 好,我们是否能够改善这个平衡性? 有什么办法?为什么可以这样做?
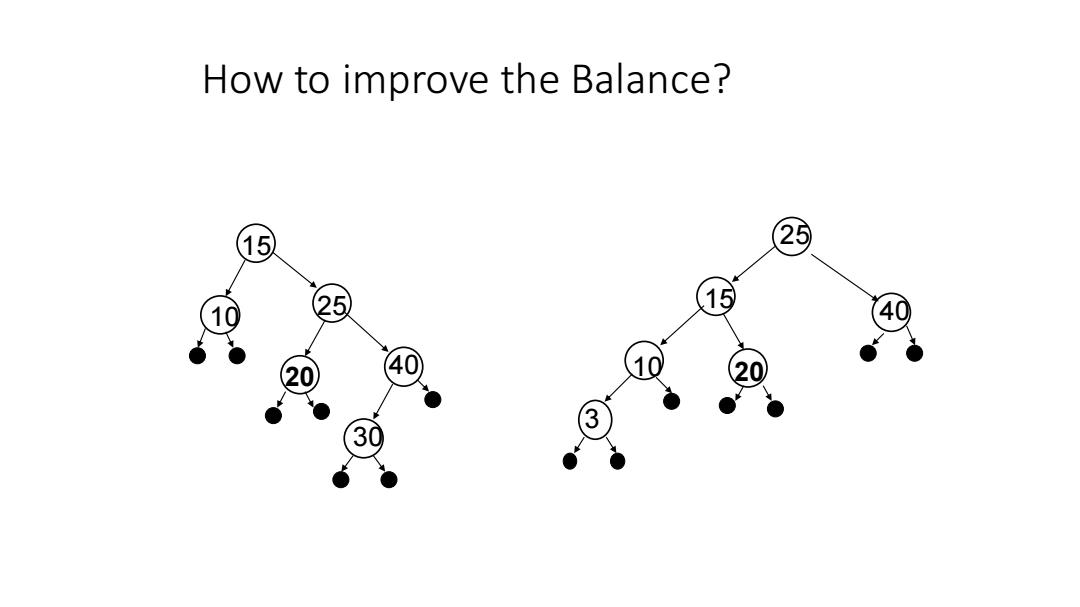
How to improve the Balance? 15 25 25 15 40 3
How to improve the Balance? 15 10 25 20 30 40 25 40 15 10 20 3