
/936 Chapter 9 Nonparametric detection UESTC 1
1 UESTC Chapter 9 Nonparametric detection
Previous detection theories are based upon the underlying statistical models of the measurements. This chapter focuses on nonparametric detection. UESTC 2
2 Previous detection theories are based upon the underlying statistical models of the measurements. This chapter focuses on nonparametric detection. 2 UESTC
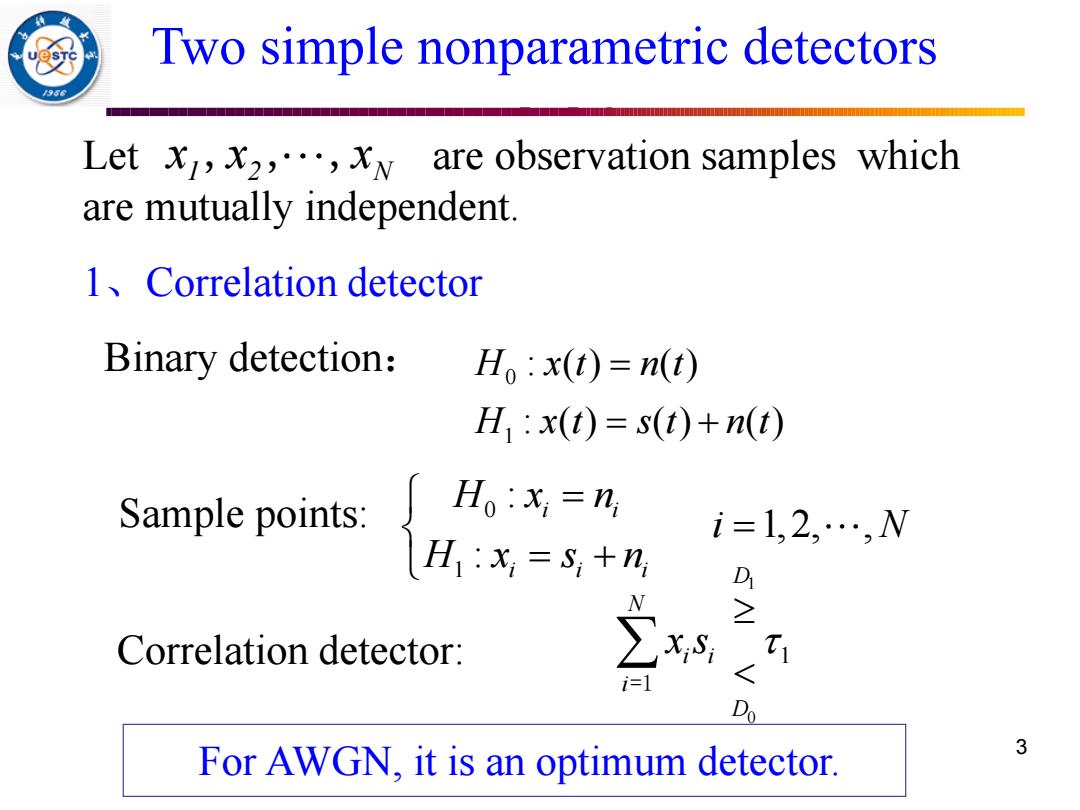
Two simple nonparametric detectors 5 Let x,X2,,xy are observation samples which are mutually independent. 1 Correlation detector Binary detection: Ho:x(t)=n(t) H:x(t)=s(t)+n(t) Sample points: Ho x=n i=1,2,…,W H1:x,=S,+n D Correlation detector: ∑x ≥ t D For AWGN,it is an optimum detector. 3
3 Let are observation samples which are mutually independent. 1、Correlation detector Binary detection: 1 2 N x x x , , , 0 1 : ( ) ( ) : ( ) ( ) ( ) H x t n t H x t s t n t = = + 0 1 : 1,2, , : i i i i i H x n i N H x s n = = = + Sample points: Correlation detector: 1 0 1 D N i i i D x s =1 Two simple nonparametric detectors For AWGN, it is an optimum detector
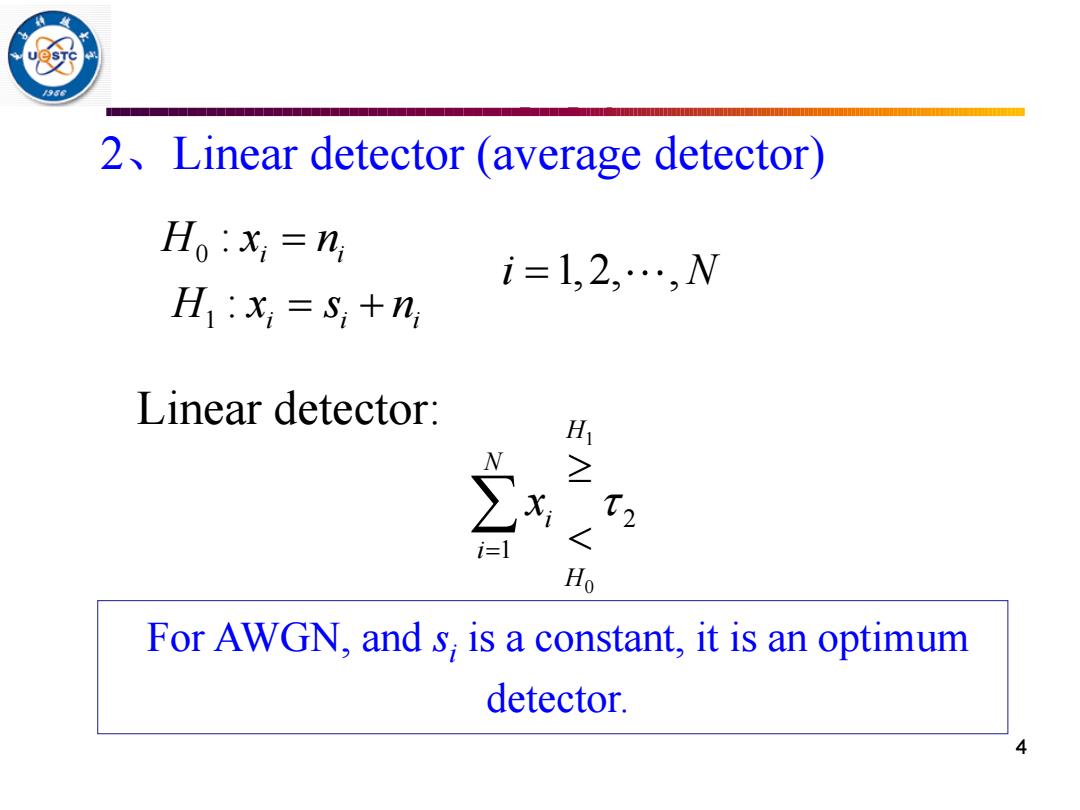
2.Linear detector (average detector) Ho:x;=n i=1,2,…,N H1:X,=S,+n Linear detector: H ≥ X 2 i=1 Ho For AWGN,and s;is a constant,it is an optimum detector
4 2、Linear detector (average detector) 0 1 : 1,2, , : i i i i i H x n i N H x s n = = = + Linear detector: 1 0 2 1 H N i i H x = For AWGN, and si is a constant, it is an optimum detector
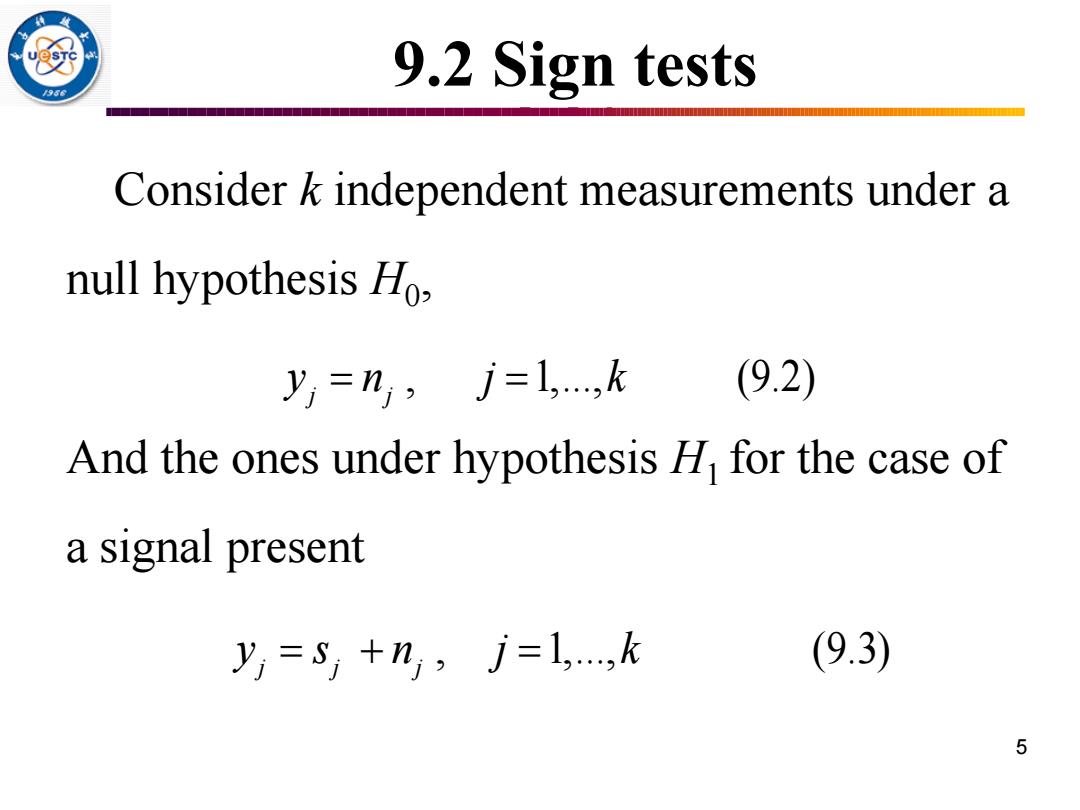
9.2 Sign tests Consider k independent measurements under a null hypothesis Ho yi=n,j=1,...k (9.2) And the ones under hypothesis H for the case of a signal present yi=s,+n,j=1,...k (9.3) 5
5 9.2 Sign tests Consider k independent measurements under a null hypothesis H0 , And the ones under hypothesis H1 for the case of a signal present , 1,..., (9.2) j j y n j k = = , 1,..., (9.3) j j j y s n j k = + =

For the sign test,we need to impose only the following conditions on this model. 。S,≥0 for all j=l,…,k s,>0 for at least onej=1,...k ·The median(中位数)of each“noise”sample is zero.i.e. ro-∫nuh2 (9.4) 6
6 For the sign test, we need to impose only the following conditions on this model. • for all • for at least one • The median(中位数) of each “noise” sample is zero. i.e. 0 j s j k =1, , 0 j k =1, , j s 0 0 1 (0) ( ) (9.4) 2 j F p n dn n j j − = =

/936 e.g. P(x) 0.5 Median-0 0.25 Mean=1/4 2 X -1 axh=小rxh-支mad=0 med 4=jw=05可+025可=4 7
7 0 2 1 0 1 ( ) 0.5 0.25 4 xp x dx xdx xdx − − = = + = x p(x) -1 2 0.25 0.5 Median=0 Mean=1/4 e.g. 1 ( ) ( ) , 0 2 med med p x dx p x dx med − = = =
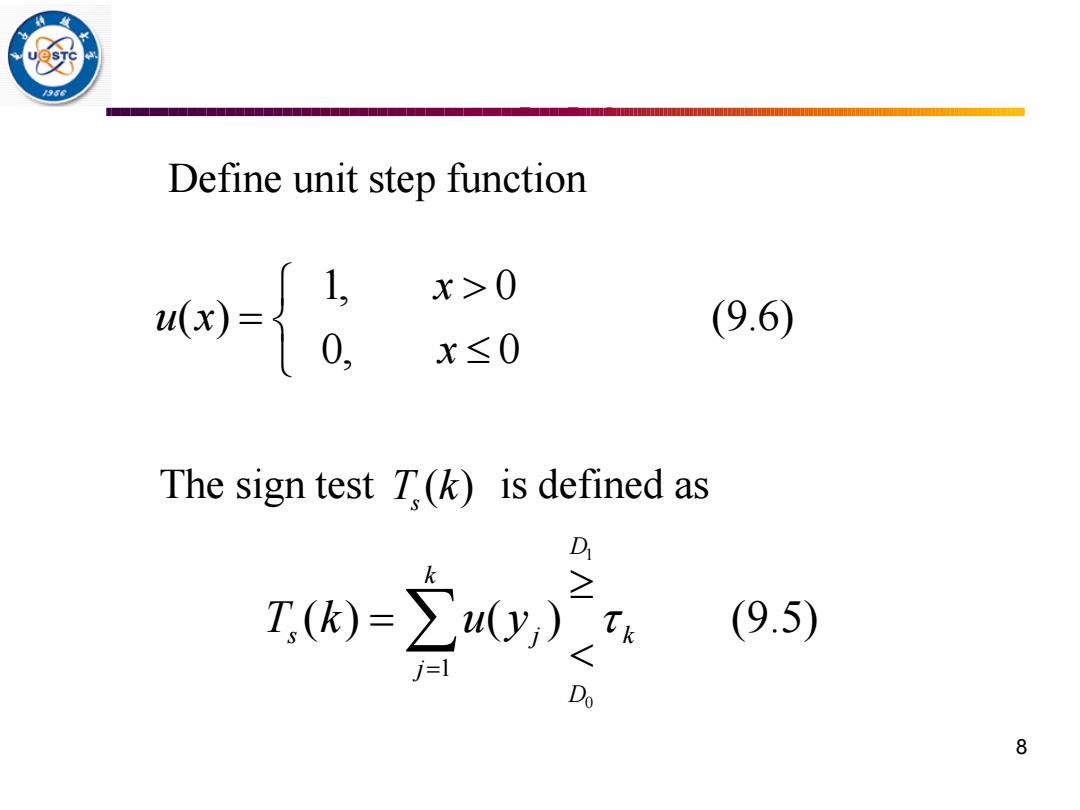
Define unit step function x-i8 x>0 (9.6) x≤0 The sign test T.(k)is defined as 1肉=空 D (9.5) i=1 D 8
8 1 0 1 ( ) ( ) (9.5) D k s j k j D T k u y = = ( ) T k s 1, 0 ( ) (9.6) 0, 0 x u x x = The sign test is defined as Define unit step function

Under Ho hypothesis,yis noise,the probability P0)=IH-)-04E The probability that T(k)is equal to is (coin-tossing experiment z=aj (9.7) 9
9 Under H0 hypothesis, yj is noise, the probability The probability that is equal to l is (coin-tossing experiment ) 0 0 1 { ( ) 1 } { ( ) 0 } 2 P u y H P u y H j j = = = = ( ) T k s 0 1 ( ) (9.7) 2 k S k P T k l H l = =
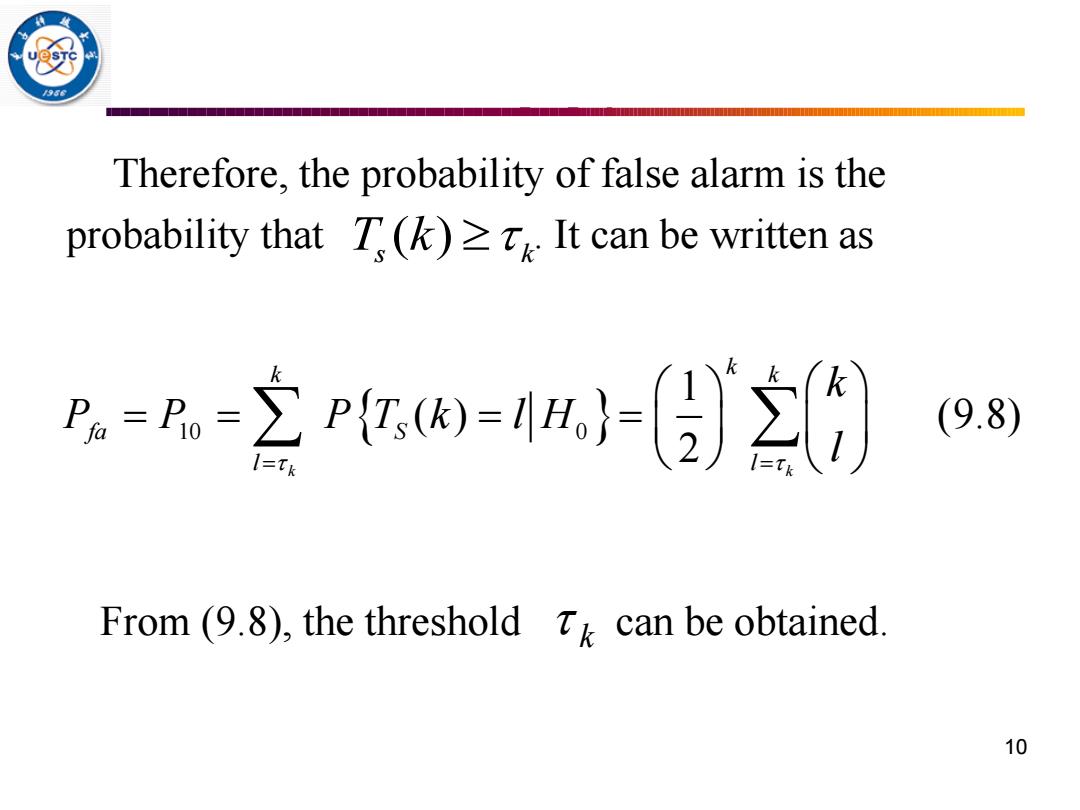
Therefore,the probability of false alarm is the probability that T(k)It can be written as R空侧- (9.8) From(9.8),the threshold tk can be obtained 10
10 Therefore, the probability of false alarm is the probability that . It can be written as ( ) T k s k 10 0 1 ( ) (9.8) 2 k k k k k fa S l l k P P P T k l H l = = = = = = From (9.8), the threshold can be obtained. k