
4.Asymptotic Analysis 1
4. Asymptotic Analysis 1

Overview 1.Continuing Mapping Theorem 2.Silverstein Theorem 2
Overview 2 1. Continuing Mapping Theorem 2. Silverstein Theorem
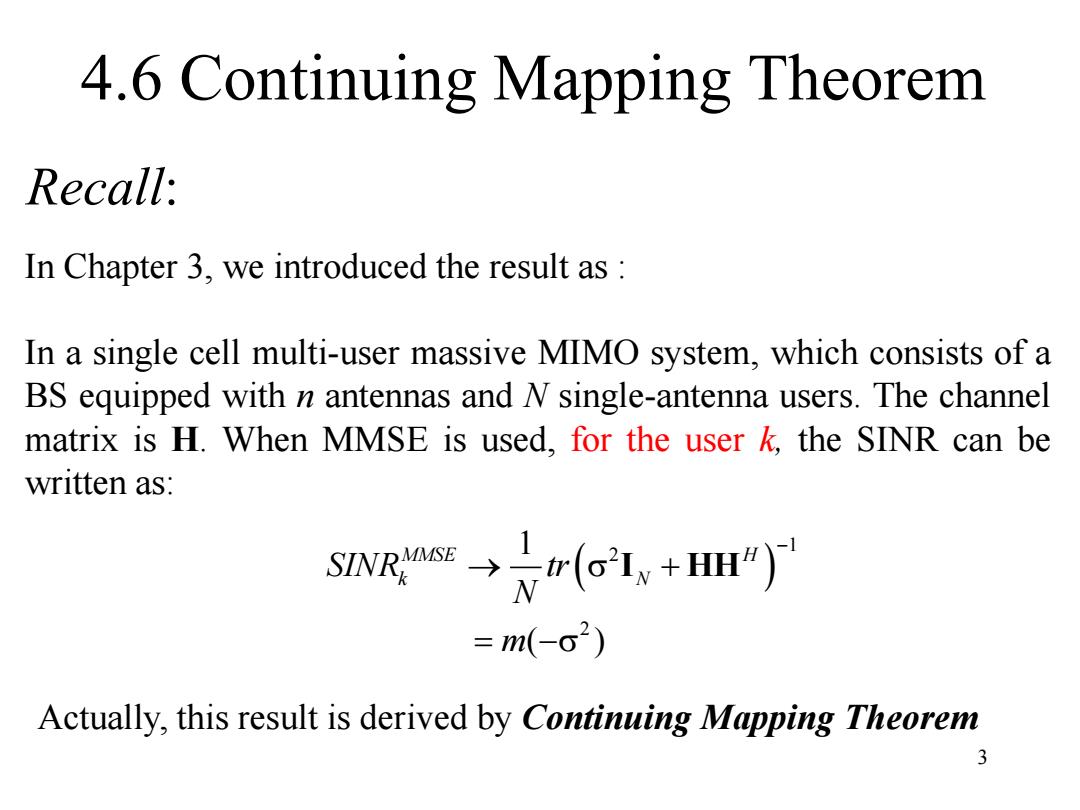
4.6 Continuing Mapping Theorem Recall: In Chapter 3,we introduced the result as In a single cell multi-user massive MIMO system,which consists of a BS equipped with n antennas and N single-antenna users.The channel matrix is H.When MMSE is used,for the user k,the SINR can be written as: SINRINGE-→r(oLy+H)' =m(-2) Actually,this result is derived by Continuing Mapping Theorem
3 Recall: In Chapter 3, we introduced the result as : In a single cell multi-user massive MIMO system, which consists of a BS equipped with n antennas and N single-antenna users. The channel matrix is H. When MMSE is used, for the user k, the SINR can be written as: 4.6 Continuing Mapping Theorem 1 2 2 1 ( ) MMSE H k N SINR tr N m I HH Actually, this result is derived by Continuing Mapping Theorem
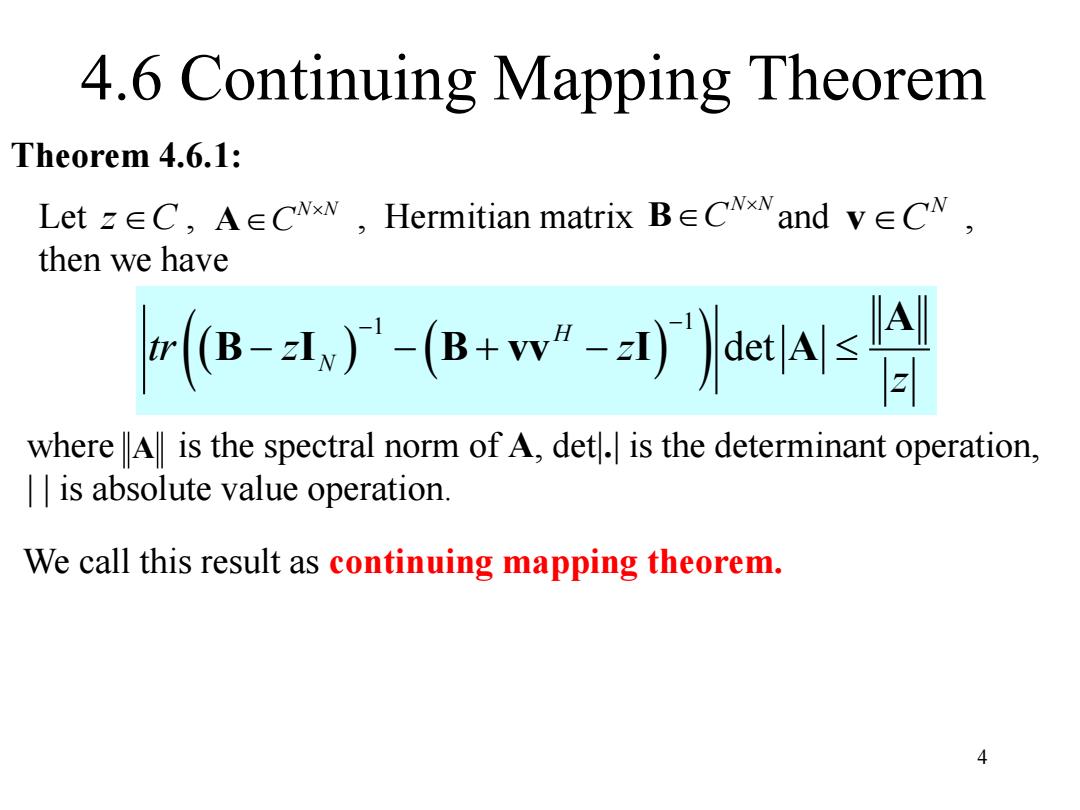
4.6 Continuing Mapping Theorem Theorem 4.6.1: Letz∈C,A∈Cxw,Hermitian matriⅸB∈CNxNand v∈Cw, then we have e-1广-ew-aNe where A is the spectral norm of A,det.is the determinant operation, is absolute value operation. We call this result as continuing mapping theorem. 4
4.6 Continuing Mapping Theorem 4 Let , , Hermitian matrix and , then we have N N C z C A N N C B N vC 1 1 det H N tr z z z A B I B vv I A where is the spectral norm of A A, det|.| is the determinant operation, | | is absolute value operation. Theorem 4.6.1: We call this result as continuing mapping theorem

4.6 Continuing Mapping Theorem We set A=Iv,an then we have ps-)/wyr]ae1uc →lrB-l)-rsw-目 →-厂B*w阳 As N tends to infinity =(B-1,)'-n(B+w-刘0 .S 5
5 4.6 Continuing Mapping Theorem We set , an then we have A I N 1 1 1 1 1 1 det 1 1 1 H N n N H n H n tr z z z tr z tr z z tr z tr z N N z I B I B vv I I B I B vv I B I B vv I . . 1 1 1 1 0 a s H n tr z tr z N N B I B vv I As N tends to infinity
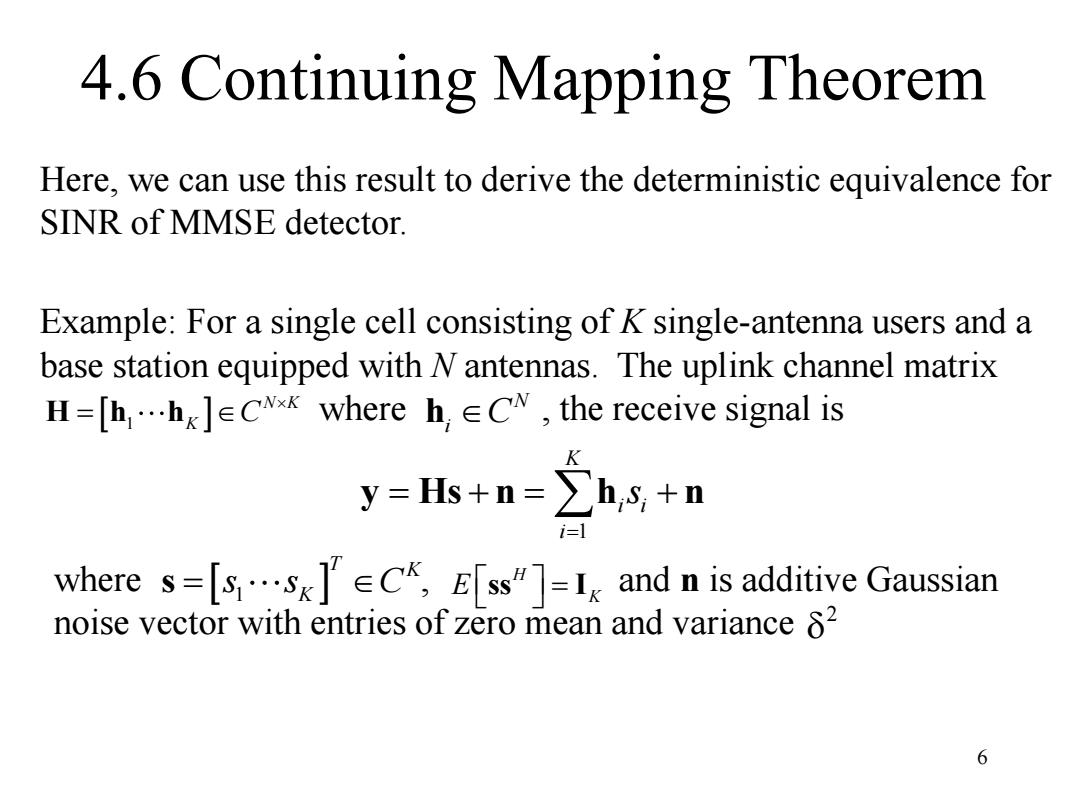
4.6 Continuing Mapping Theorem Here,we can use this result to derive the deterministic equivalence for SINR of MMSE detector. Example:For a single cell consisting of K single-antenna users and a base station equipped with N antennas.The uplink channel matrix H=[hh]eC where h,ECN,the receive signal is y=Hs+n=∑h,+n i=l where s=[sKC Ess=Ig and n is additive Gaussian noise vector with entries of zero mean and variance 82 6
6 4.6 Continuing Mapping Theorem Here, we can use this result to derive the deterministic equivalence for SINR of MMSE detector. Example: For a single cell consisting of K single-antenna users and a base station equipped with N antennas. The uplink channel matrix where , the receive signal is 1 N K K C H h h N hi C 1 K i i i s y Hs n h n where , and n is additive Gaussian noise vector with entries of zero mean and variance 1 T K K s s s C H E K ss I 2

4.6 Continuing Mapping Theorem For user k,the signal by multiplying MMSE decoder matrix is yMe=h(HH”+8Lw)厂y -空a+j2n The sienal part:h. The inerfarnce part[2hA+丁交g The nise prt(2②Au+,” 7
7 4.6 Continuing Mapping Theorem For user k, the signal by multiplying MMSE decoder matrix is 1 2 1 2 1 1 MMSE H H k k N K K H H k i i N i i i i y s h HH I y h h h I h n The signal part: 1 2 1 K H H k i i N k k i s h h h I h The interference part: 1 2 1 1 K K H H k i i N i i i i i k s h h h I h 1 2 1 K H H k i i N i The noise part: h h h I n
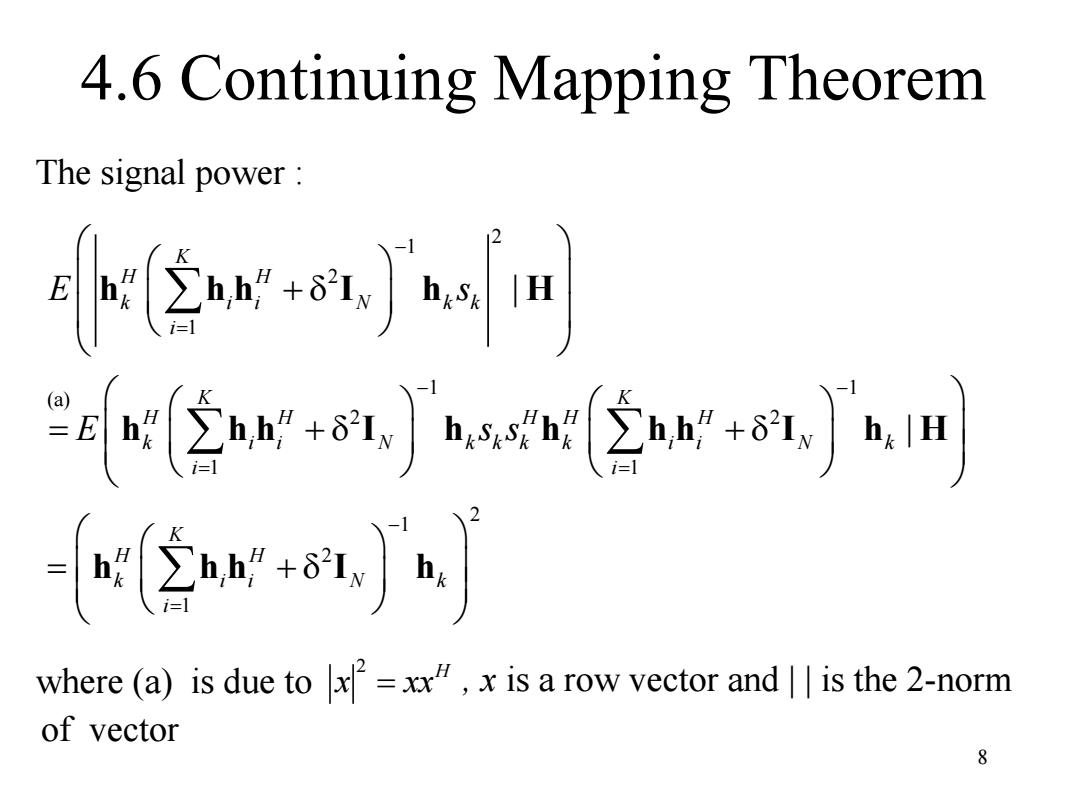
4.6 Continuing Mapping Theorem The signal power wenj小s川 “[+,62A1小'H [2A+n八j】 where (a)is due toxx,x is a row vector and is the 2-norm of vector 8
8 4.6 Continuing Mapping Theorem The signal power : 2 1 2 1 1 1 (a) 2 2 1 1 2 1 2 1 | | K H H k i i N k k i K K H H H H H k i i N k k k k i i N k i i K H H k i i N k i E s E s s h h h I h H h h h I h h h h I h H h h h I h where (a) is due to , x is a row vector and | | is the 2-norm 2 H x xx of vector

4.6 Continuing Mapping Theorem Hence,the signal power is: (. The interference power: -.w小m However,we haveE 9
9 4.6 Continuing Mapping Theorem Hence, the signal power is: 2 1 2 1 K H H k i i N k i h h h I h The interference power: 1 1 2 2 1 1 1 1 | K K K K H H H H k i i N i i i i i i N k i i i i i k i k E s s h h h I h h h h I h H However, we have 1 0 i j i j E s s i j

4.6 Continuing Mapping Theorem Hence,the interference power can be written as 含a+s,)2u2+, Here,we know that 立a=[乞+,1,-a The interference power can be rewritten as -空j-[+mj八
10 4.6 Continuing Mapping Theorem Hence, the interference power can be written as 1 1 2 2 1 1 K K K H H H H k i i N i i i i N k i i k i h h h I h h h h I h Here, we know that 2 2 1 K K H H H i i i i N N k k i k i h h h h I I h h The interference power can be rewritten as 2 1 2 1 2 2 2 2 1 1 1 K K K H H H H H H k i i N k k i i N k k i i N k i i i h h h I h h h h I h h h h I h