
4.Asymptotic Spectrum Theorems 1
1 4. Asymptotic Spectrum Theorems
Overview Apply central limiting theorem into multi-user and multi-cell MIMO system ·MF detector in uplink MF and ZF precoding in downlink MF and ZF precoding with imprefect CSI ·Multi--cell system 2
2 Overview • MF detector in uplink • MF and ZF precoding in downlink • MF and ZF precoding with imprefect CSI • Multi-cell system Apply central limiting theorem into multi-user and multi-cell MIMO system
4.4 Central Limiting Theorem User User User Base station User Consider a MU-MIMO system with a single cell,where has K single-antenna users and one BS with N antennas. 3
3 4.4 Central Limiting Theorem Consider a MU-MIMO system with a single cell, where has K single-antenna users and one BS with N antennas
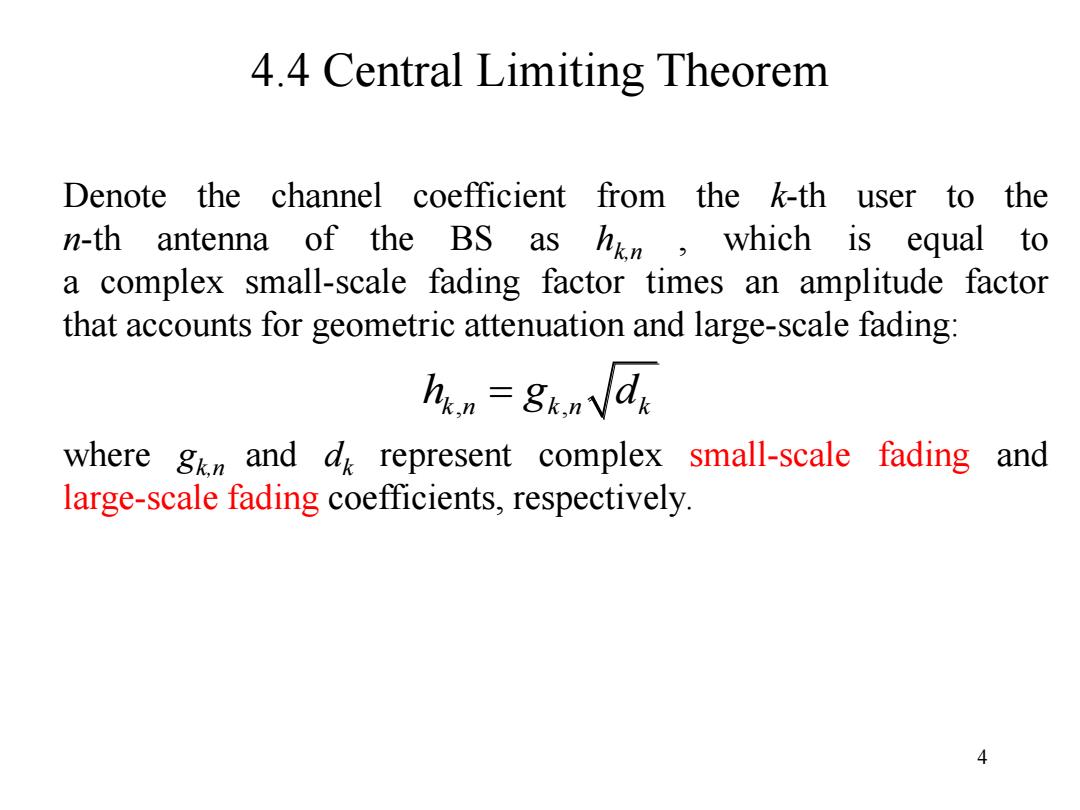
4.4 Central Limiting Theorem Denote the channel coefficient i from the k-th user to the n-th antenna of the BS as hk.n,v which is s equal to a complex small-scale fading factor times an amplitude factor that accounts for geometric attenuation and large-scale fading: hn =gknYdk where gkm and d represent complex small-scale fading and large-scale fading coefficients,respectively. 4
4 4.4 Central Limiting Theorem Denote the channel coefficient from the k-th user to the n-th antenna of the BS as hk,n , which is equal to a complex small-scale fading factor times an amplitude factor that accounts for geometric attenuation and large-scale fading: where gk,n and dk represent complex small-scale fading and large-scale fading coefficients, respectively. k n k n k , , h g d
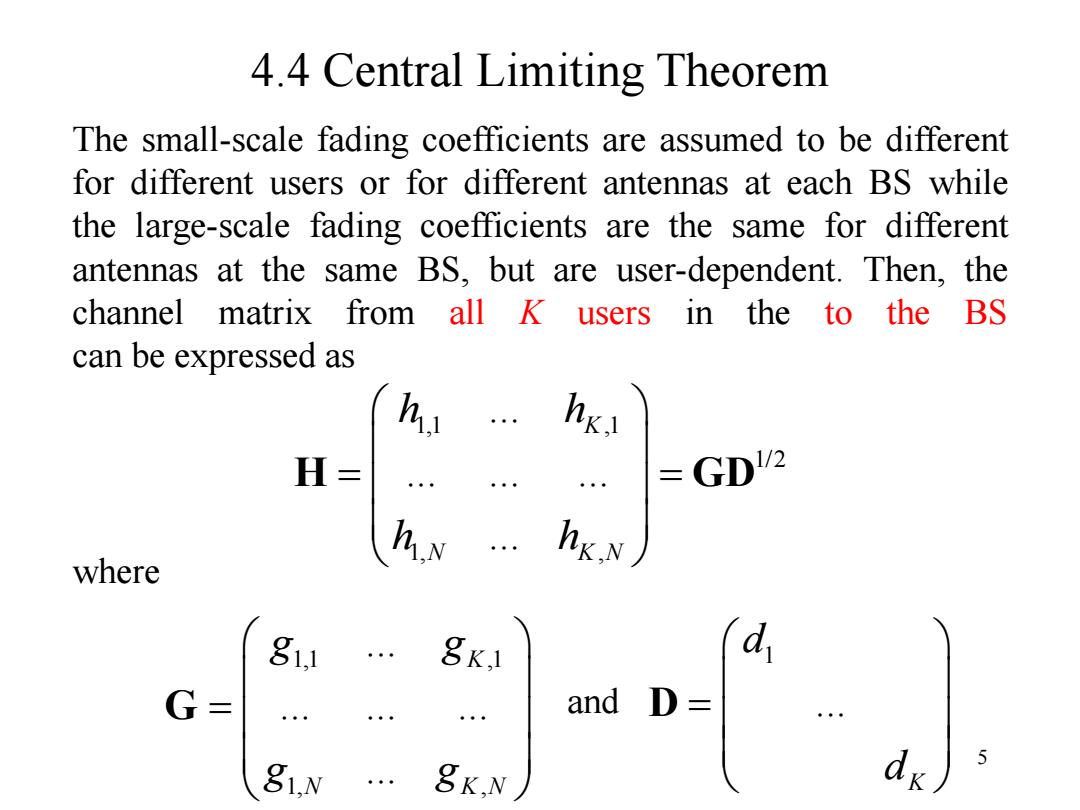
4.4 Central Limiting Theorem The small-scale fading coefficients are assumed to be different for different users or for different antennas at each BS while the large-scale fading coefficients are the same for different antennas at the same BS,but are user-dependent.Then,the channel matrix from all K users s in the to the BS can be expressed as H= h.N where hi.N g1,1 8K,1 G and D 81,N 8K.N
5 4.4 Central Limiting Theorem The small-scale fading coefficients are assumed to be different for different users or for different antennas at each BS while the large-scale fading coefficients are the same for different antennas at the same BS, but are user-dependent. Then, the channel matrix from all K users in the to the BS can be expressed as where and 1,1 ,1 1/2 1, , ... ... ... ... ... K N K N h h h h H GD 1,1 ,1 1, , ... ... ... ... ... K N K N g g g g G 1 ... K d d D
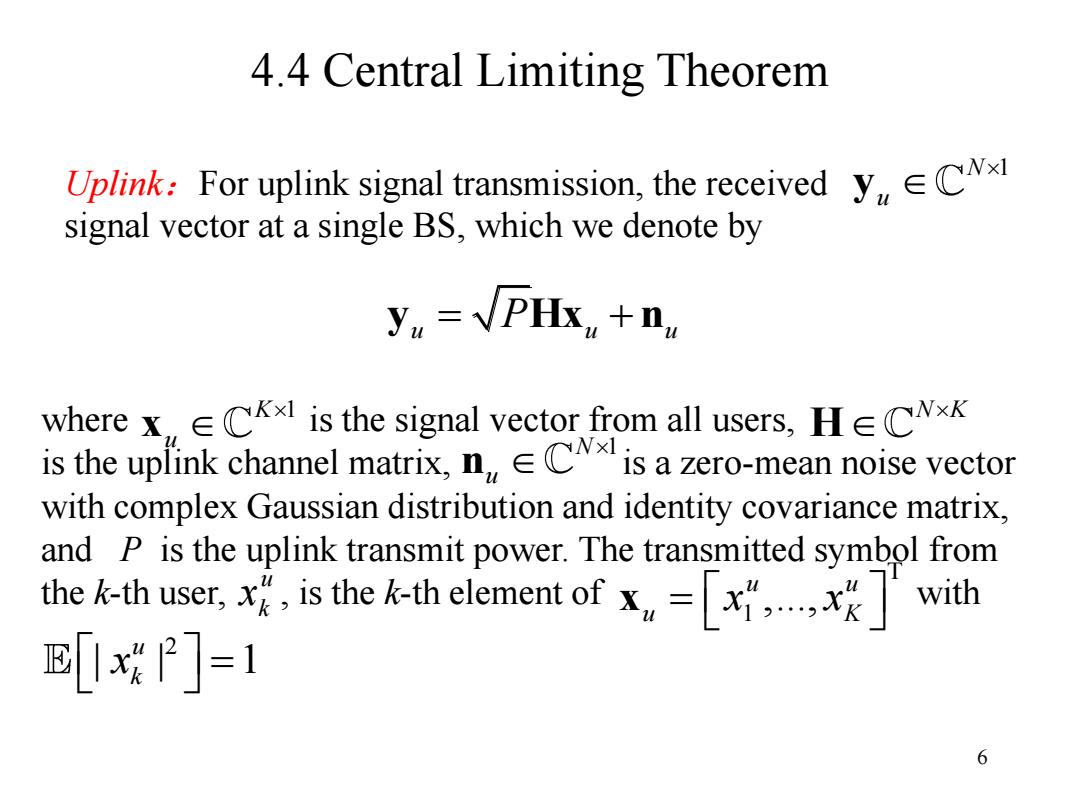
4.4 Central Limiting Theorem Uplink:For uplink signal transmission,the received y signal vector at a single BS,which we denote by y =PHx+n where x CK is the signal vector from all users,HECNxK is the uplink channel matrix,is a zero-mean noise vector with complex Gaussian distribution and identity covariance matrix, and P is the uplink transmit power.The transmitted symbol from the k-th user,is the k-th element ofwith [x门=1 6
6 4.4 Central Limiting Theorem Uplink:For uplink signal transmission, the received signal vector at a single BS, which we denote by N 1 u y y Hx n u u u P where is the signal vector from all users, is the uplink channel matrix, is a zero-mean noise vector with complex Gaussian distribution and identity covariance matrix, and P is the uplink transmit power. The transmitted symbol from the k-th user, , is the k-th element of with K 1 u x N 1 u n N K H T 1 ,..., u u u K x x x u k x 2 | | 1 u k x

4.4 Central Limiting Theorem Based on the assumption that the small-scale fading coefficients for different users are independent,the column channel vectors from different users are asymptotically orthogonal as the number of antennas at the BS,N,grows to infinity.Then, we have HHH-DIGHGD2 NDIID2 =ND Based on the result in,the achievable rate of the multi-user MIMO system becomes C=log2 det(I+PHH ≈log2det(I+WPD) =∑1og2(1+NPdx) k 7
7 4.4 Central Limiting Theorem Based on the assumption that the small-scale fading coefficients for different users are independent, the column channel vectors from different users are asymptotically orthogonal as the number of antennas at the BS, N , grows to infinity. Then, we have H 1/2 H 1/2 1/2 1/2 H H D G GD D I D D N N K Based on the result in, the achievable rate of the multi-user MIMO system becomes H 2 2 2 1 log det log det log 1 K k k C P NP NPd I H H I D
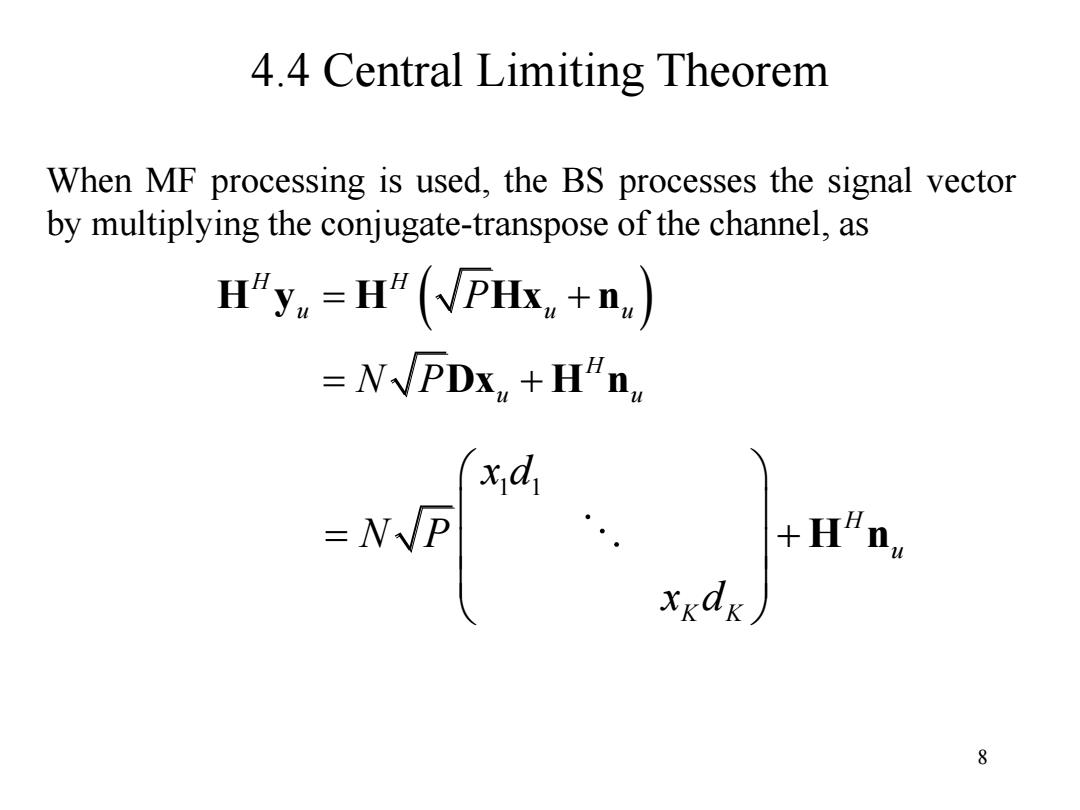
4.4 Central Limiting Theorem When MF processing is used,the BS processes the signal vector by multiplying the conjugate-transpose of the channel,as H"y,=H"PHx+n) =N√PDx+HnW xd =NP +Hn xkdk 8
8 4.4 Central Limiting Theorem When MF processing is used, the BS processes the signal vector by multiplying the conjugate-transpose of the channel, as 1 1 H u K K x d N P x d H n H H u u u H u u P N P H y H Hx n Dx H n
4.4 Central Limiting Theorem Since D is a diagonal matrix,the MF processing separates the signals from different users into different streams and there is asymptotically no inter-user interference.The signal transmissions from each user can thus be treated as if originating from a SISO channel. 9
9 4.4 Central Limiting Theorem Since D is a diagonal matrix, the MF processing separates the signals from different users into different streams and there is asymptotically no inter-user interference. The signal transmissions from each user can thus be treated as if originating from a SISO channel
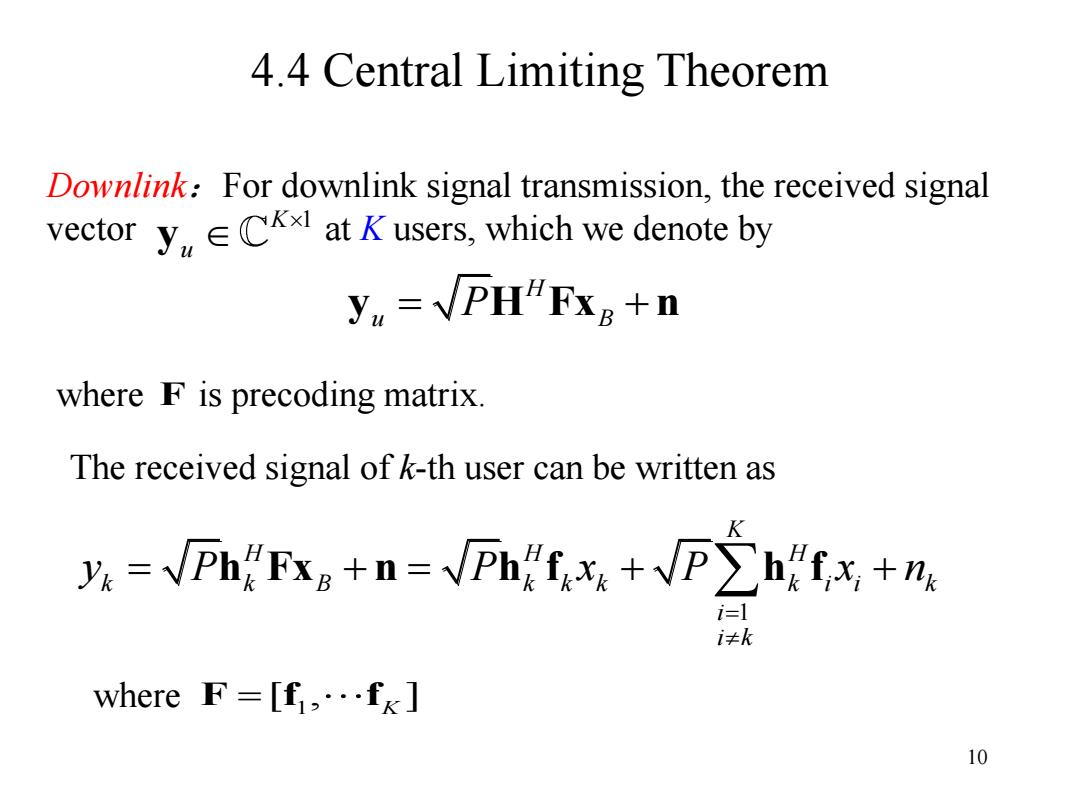
4.4 Central Limiting Theorem Downlink:For downlink signal transmission,the received signal vector y at Kusers,which we denote by y=PH"Fx8+n where F is precoding matrix. The received signal of k-th user can be written as y=PhgFxg+n=Pnf+Phfx+ng i=l i≠k where F=[f,…fk] 10
10 4.4 Central Limiting Theorem Downlink:For downlink signal transmission, the received signal vector at K users, which we denote by K 1 u y H y H Fx n u B P where is precoding matrix. F The received signal of k-th user can be written as 1 K H H H k k B k k k k i i k i i k y P P x P x n h Fx n h f h f where 1 [ , ] F f f K