3,Transforms 1
3. Transforms 1

Overview We will introduce the most useful transforms which are similar to Fourier transform in signal processing,including: ▣Stieltjes transform ▣Shannon transform ▣R transform ▣S transform ▣n-transform These transforms will form a strong basis to be able to understand the extensions discussed in the subsequent Chapters 2
Overview 2 We will introduce the most useful transforms which are similar to Fourier transform in signal processing, including: Stieltjes transform Shannon transform R transform S transform η-transform These transforms will form a strong basis to be able to understand the extensions discussed in the subsequent Chapters
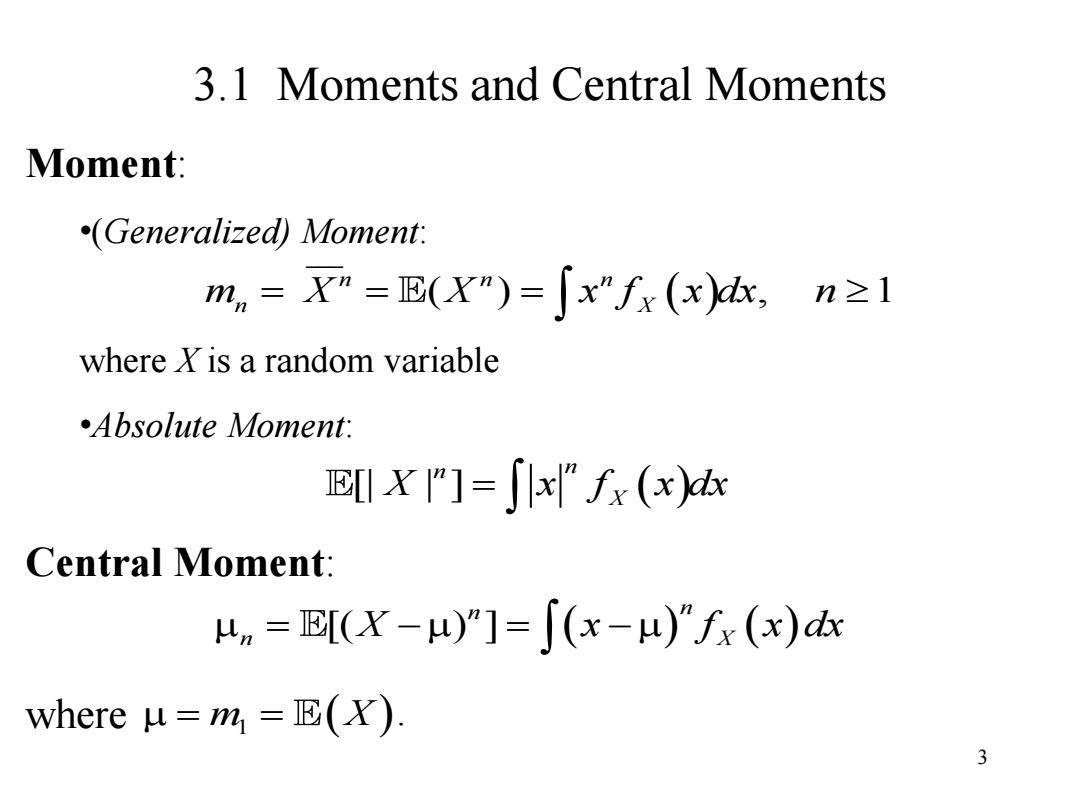
3.1 Moments and Central Moments Moment: (Generalized)Moment: mn=X”=E(X")=∫x”fx(x),n≥1 where X is a random variable Absolute Moment: EX]=Jlx”fx(x) Central Moment: un=E(X-)”]=J(x-u)”fx(x)d where u=m=(). 3
3 3.1 Moments and Central Moments Moment: •(Generalized) Moment: where X is a random variable •Absolute Moment: Central Moment: where ___ ( ) , 1 n n n m X X x f x dx n n X [| | ] n n X x f x dx X [( ) ] n n n X X x f x dx m X 1

3.1.1 Moments for Gaussian Random Variable If X N(u,o2),then u=E(X),o2=E(X-)) and 现x-1-h9w.日n n odd, 13…(n-1)o”, n even, X-u)F2k1o2元,n=(2k+0,odd 4
4 3.1.1 Moments for Gaussian Random Variable If then and ~ ( , ), 2 X N 2 2 X X , 0, odd, [ ] 1 3 ( 1) , even. n n n X n n 2 1 1 3 ( 1) , even, (| | ) 2 ! 2 / , (2 1), odd. n n k k n n X k n k

3.2 Characteristic Function 3.2 Definition: Φx(o)=E(eo)=∫ehof(x) For diserete r. Φx(o)=∑eoP(X=k) Properties: (iΦx(o)=1andΦx(o≤1. mm.=129(x)1-2m m-(-o (n≥1) 0=0 5
5 3.2 Characteristic Function 3.2 Definition: For discrete r.v., Properties: (i) and (ii) ( ) ( ) . jX jx X X e e f x dx k jk X ( ) e P(X k). X (0) 1 () 1. X 1 1 0 ( ) 1 1 ! ! 1 ( ) ( 1) n n n X n n n n n X n n n j j X m n n d m X n j d

3.3 Stieltjes Transform History:Stielyjes transform is proposed by Thomas Stieltjes in 1885, which is used in the study of nonnegative complex number.In 1967, Russian mathematician Marcenko and Pastur exploited Stieltjes transform to derive the limiting eigenvalues distribution of large dimension Wishart random matrix which is called as MP law. Definition 3.3.1:Let X be a real random variable with distribution Fx).Its Stieltjes transform is defined as: m.a)-画x1) Theorem 3.3.1:For all F which admits a Stieltjes transform,the inverse transformation exists and formulates as follows F)-元m广mm,(x+刚]k 6
6 3.3 Stieltjes Transform Definition 3.3.1: Let X be a real random variable with distribution FX (·). Its Stieltjes transform is defined as: 1 1 ( ) [ ] ( ) F X X m z dF X z z Theorem 3.3.1: For all F which admits a Stieltjes transform, the inverse transformation exists and formulates as follows 0 1 ( ) lim Im ( ) X x F y F x m x iy dx History: Stieltjes transform is proposed by Thomas Stieltjes in 1885, which is used in the study of nonnegative complex number. In 1967, Russian mathematician Marcenko and Pastur exploited Stieltjes transform to derive the limiting eigenvalues distribution of large dimension Wishart random matrix which is called as MP law
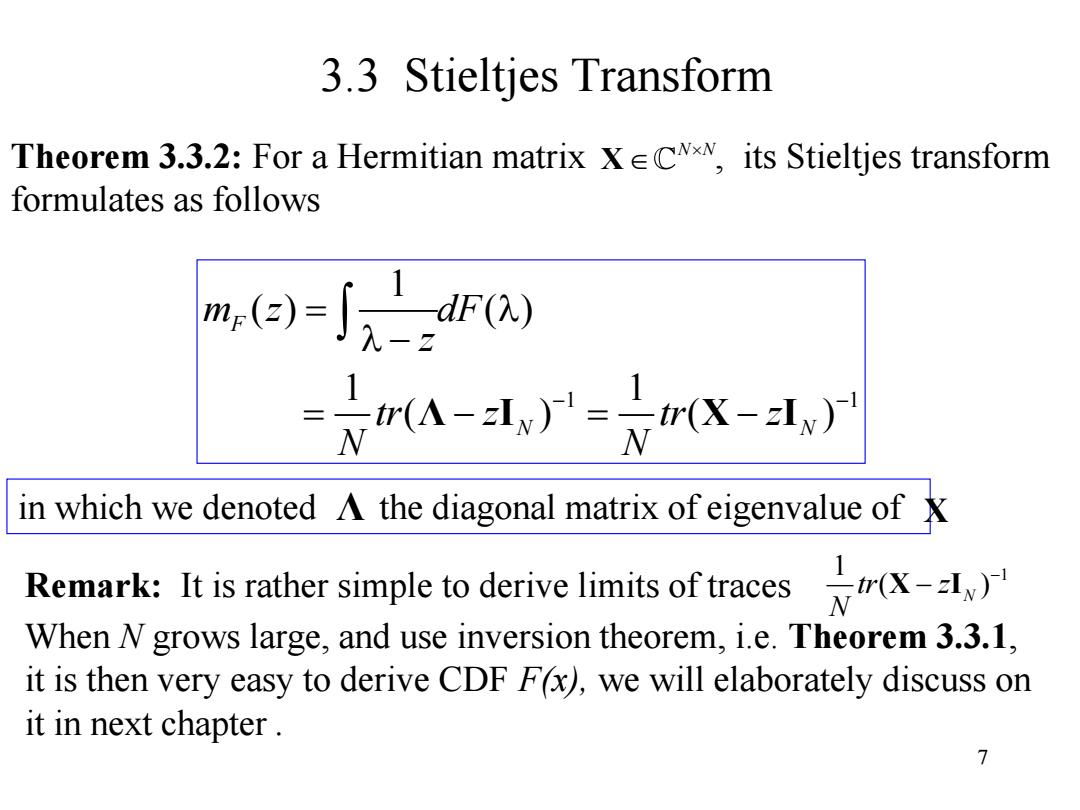
3.3 Stieltjes Transform Theorem 3.3.2:For a Hermitian matrix XeCNxM,its Stieltjes transform formulates as follows ma)-2 =T(A-:Iy)=Tm(X-2Iy) 1 N in which we denoted A the diagonal matrix of eigenvalue of Remark:It is rather simple to derive limits of traces x-1,y When N grows large,and use inversion theorem,i.e.Theorem 3.3.1, it is then very easy to derive CDF F),we will elaborately discuss on it in next chapter. 7
7 3.3 Stieltjes Transform Theorem 3.3.2: For a Hermitian matrix , its Stieltjes transform formulates as follows N N X 1 1 1 ( ) ( ) 1 1 ( ) ( ) F N N m z dF z tr z tr z N N Λ I X I Remark: It is rather simple to derive limits of traces 1 1 ( ) N tr z N X I When N grows large, and use inversion theorem, i.e. Theorem 3.3.1, it is then very easy to derive CDF F(x), we will elaborately discuss on it in next chapter . in which we denoted the diagonal matrix of eigenvalue of Λ X
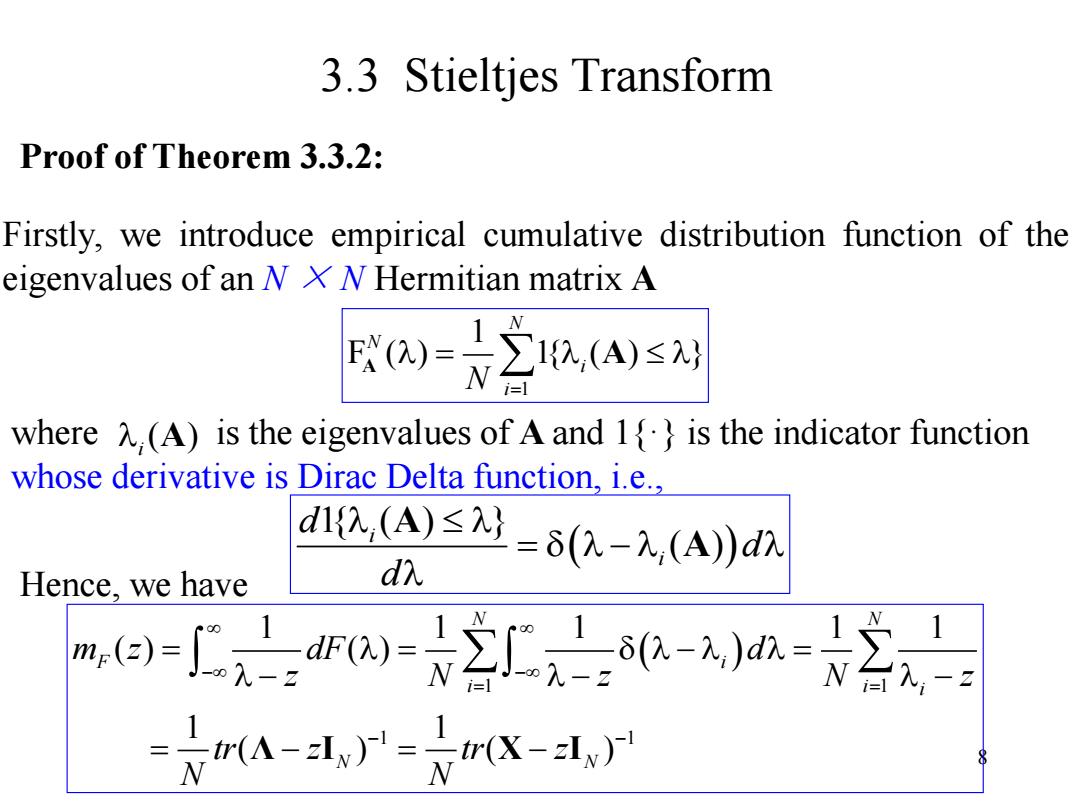
3.3 Stieltjes Transform Proof of Theorem 3.3.2: Firstly,we introduce empirical cumulative distribution function of the eigenvalues of an N X N Hermitian matrix A =之,(a)s刘 where (A)is the eigenvalues of A and 1 is the indicator function whose derivative is Dirac Delta function,i.e., d1{2,(A)≤入 =δ(-,(A)dn Hence,we have dλ m日--m)2-8c-h2- 是-l厂=rx-厂
8 3.3 Stieltjes Transform Proof of Theorem 3.3.2: 1 1 1 1 1 1 1 1 1 ( ) ( ) 1 1 ( ) ( ) N N F i i i i N N m z dF d z N z N z tr z tr z N N Λ I X I Firstly, we introduce empirical cumulative distribution function of the eigenvalues of an N × N Hermitian matrix A 1 1 F ( ) 1{ ( ) } N N i N i A A where is the eigenvalues of A and 1{·} is the indicator function whose derivative is Dirac Delta function, i.e., ( ) i A 1{ ( ) } ( ) i i d d d A A Hence, we have
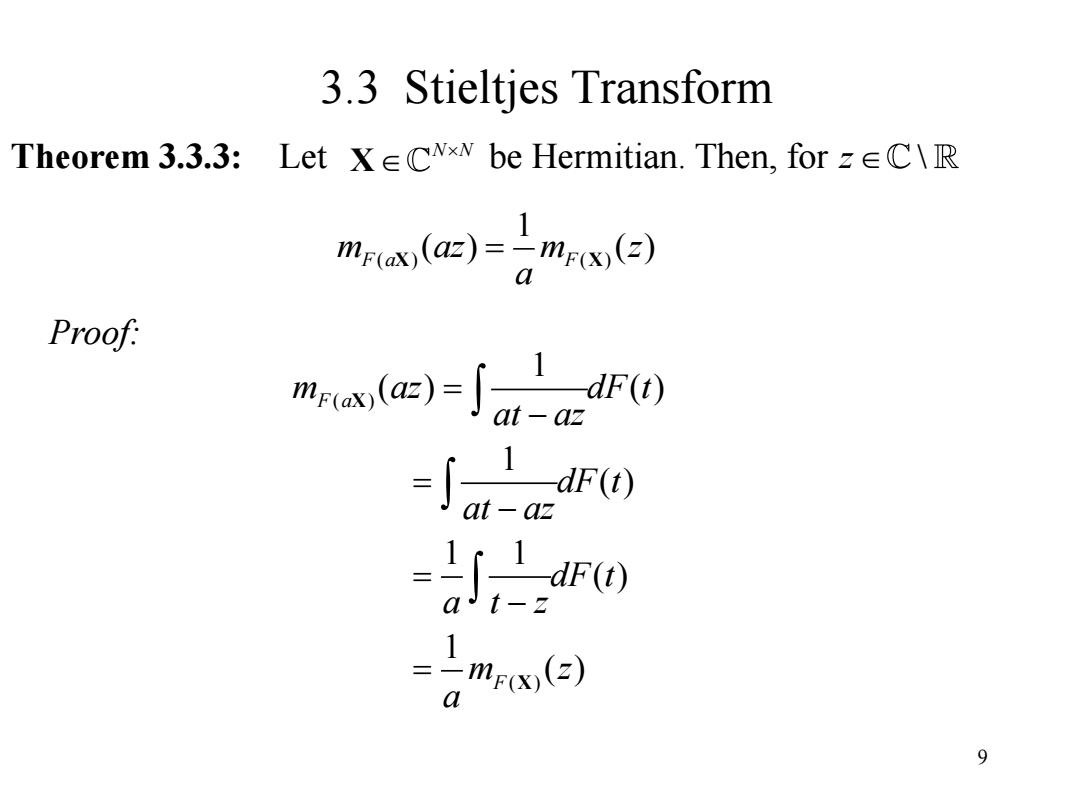
3.3 Stieltjes Transform Theorem 3.3.3:Let XeCNxN be Hermitian.Then,for zC\R mru(z)=m( Proof: mae)-a'正F0 ao =,20 -1m ( 9
9 3.3 Stieltjes Transform Theorem 3.3.3: Let be Hermitian. Then, for N N X z \ ( ) ( ) 1 ( ) ( ) m az m z F a F a X X Proof: ( ) ( ) 1 ( ) ( ) 1 ( ) 1 1 ( ) 1 ( ) F a F m az dF t at az dF t at az dF t a t z m z a X X
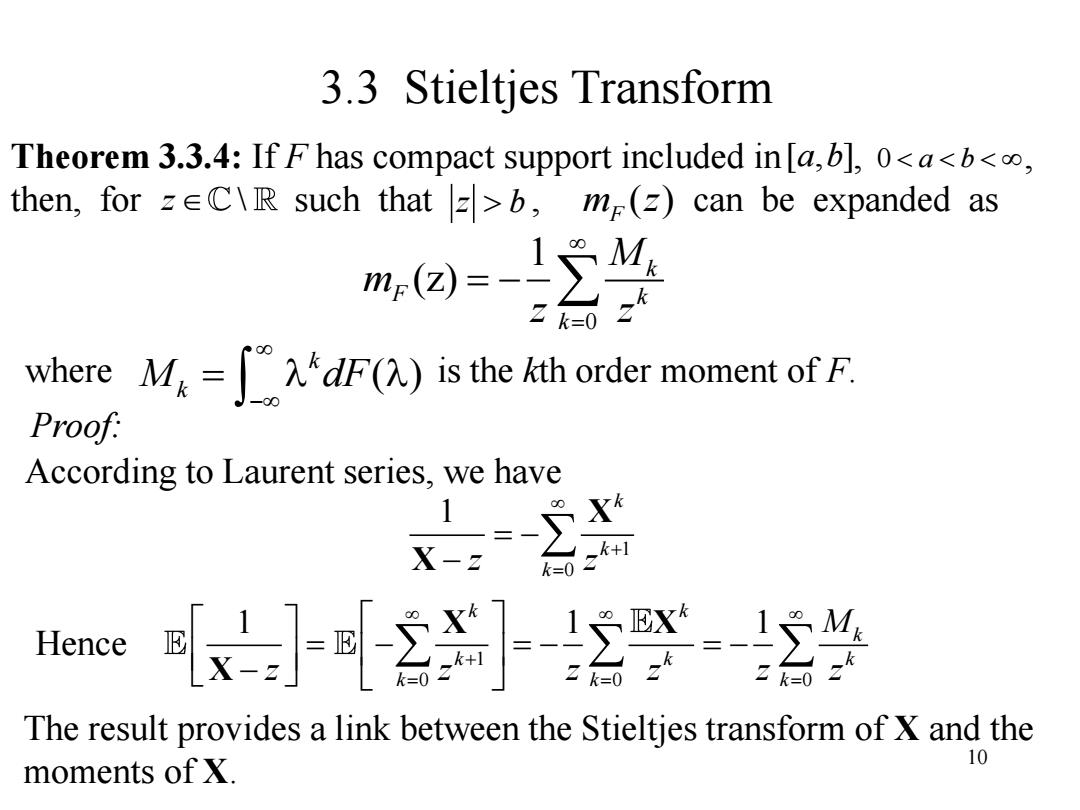
3.3 Stieltjes Transform Theorem 3.3.4:If F has compact support included in [a,b],0b,m(z)can be expanded as m=-2兰 Zk=0 where M=dF()is the kth order moment of F. Proof: According to Laurent series,we have X-z k=02 州会空 Zk=0 The result provides a link between the Stieltjes transform of X and the 10 moments of X
10 3.3 Stieltjes Transform Theorem 3.3.4: If F has compact support included in , , then, for such that , can be expanded as z \ z b ( ) m z F [ , ] a b 0 a b 0 1 (z) k F k k M m z z ( ) k M dF k where is the kth order moment of F. The result provides a link between the Stieltjes transform of X and the moments of X. Proof: According to Laurent series, we have 1 0 1 k k z z k X X Hence 1 0 0 0 1 1 1 k k k k k k k k k M z z z z z z X X X