
上游充通大¥ SHANGHAI JIAO TONG UNIVERSITY Engineering Thermodynamics I Lecture 12 Spring,2018 Prof.,Dr.Yonghua HUANG 强 A几nn是n http://cc.sjtu.edu.cn/G2S/site/thermo.html 1日G
Engineering Thermodynamics I Lecture 12 Spring, 2018 Prof., Dr. Yonghua HUANG http://cc.sjtu.edu.cn/G2S/site/thermo.html
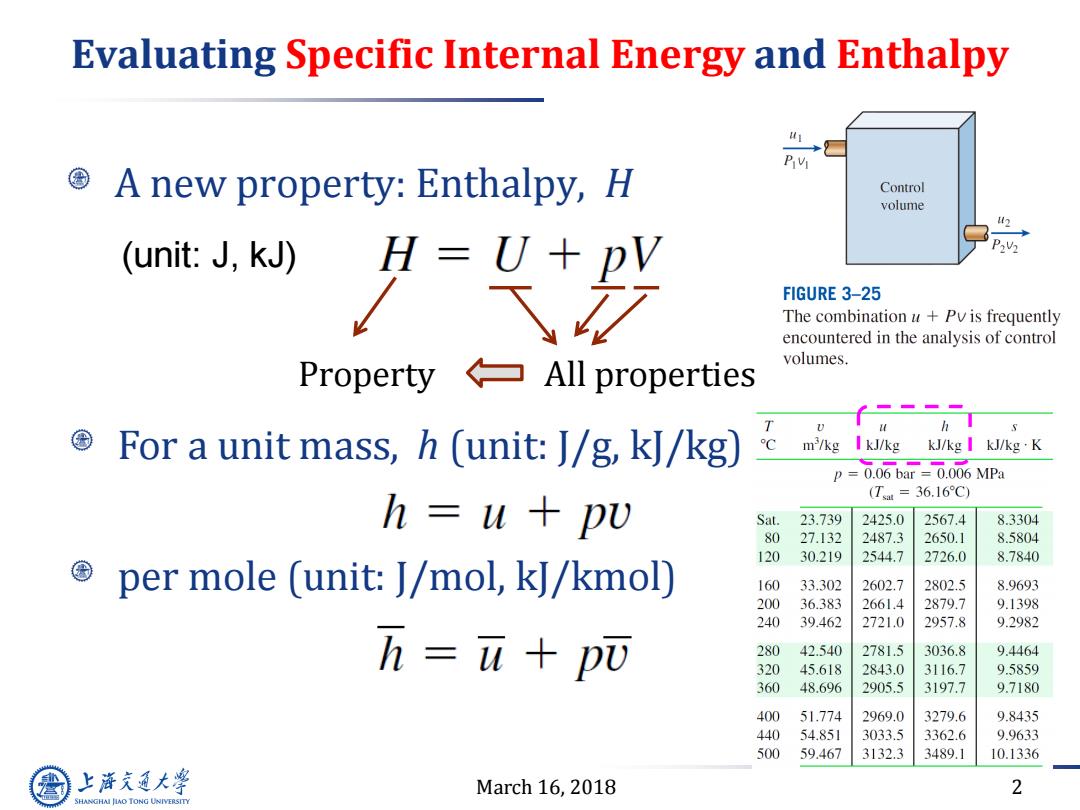
Evaluating Specific Internal Energy and Enthalpy A new property:Enthalpy,H Control volume (unit:J,KJ) ”=光 FIGURE 3-25 The combination u+Pv is frequently encountered in the analysis of control volumes. PropertyAll properties 0 For a unit mass,h (unit:J/g,kJ/kg) m'/kg I kJ/kg kJ/kg I kJ/kg K p=0.06bar=0.006MPa (T=36.16C) h u+pu Sat. 23.739 2425.0 2567.4 8.3304 80 27.132 2487.3 2650.1 8.5804 120 30.219 2544.7 2726.0 8.7840 per mole (unit:J/mol,kJ/kmol) 160 33.302 2602.7 2802.5 8.9693 200 36.383 2661.4 2879.7 9.1398 240 39.462 2721.0 2957.8 9.2982 h=u+p而 280 42.540 2781.5 3036.8 9.4464 320 45.618 2843.0 3116.7 9.5859 360 48.696 2905.5 3197.7 9.7180 400 51.774 2969.0 3279.6 9.8435 440 54.851 3033.5 3362.6 9.9633 500 59.467 3132.3 3489.1 10.1336 上游通大学 March 16,2018 2 SHANGHAI JLAO TONG UNIVERSITY
March 16, 2018 2 Evaluating Specific Internal Energy and Enthalpy A new property: Enthalpy, H Property All properties For a unit mass, h (unit: J/g, kJ/kg) per mole (unit: J/mol, kJ/kmol) (unit: J, kJ)

u and h in two-phase region The same way as the specific volume v Tables for sat.properties quality x u=(1-x)4r+g=4+x(ug-4) ↓ Sat.liquid Sat.vapor h (1 -x)hy xhg hr x(hg -h) L hie Latent heat,vaporization heat 上游究通大学 March 16,2018 3 SHANGHAI JLAO TONG UNIVERSITY
March 16, 2018 3 u and h in two-phase region The same way as the specific volume v Tables for sat. properties + quality x Sat. liquid Sat. vapor Latent heat, vaporization heat + pv
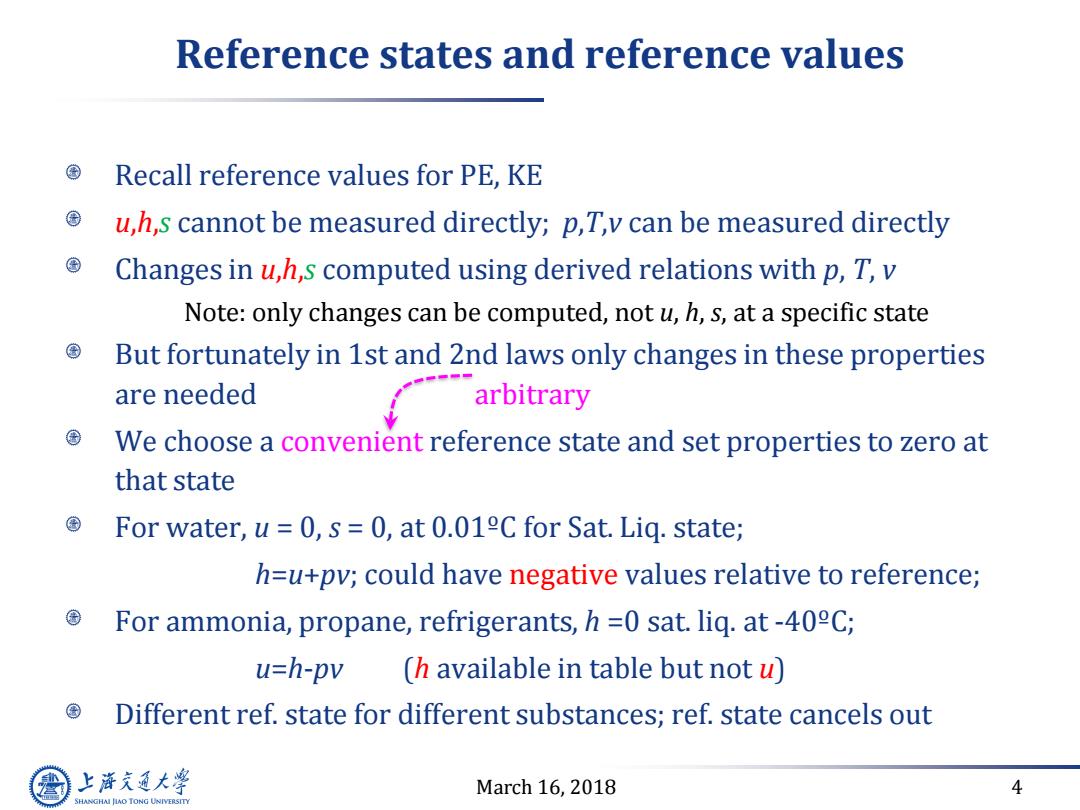
Reference states and reference values Recall reference values for PE,KE u,h,s cannot be measured directly;p,T,v can be measured directly Changes in u,h,s computed using derived relations with p,T,v Note:only changes can be computed,not u,h,s,at a specific state But fortunately in 1st and 2nd laws only changes in these properties are needed arbitrary 国 We choose a convenient reference state and set properties to zero at that state ● For water,u=0,s=0,at 0.01C for Sat.Liq.state; h=u+pv;could have negative values relative to reference; For ammonia,propane,refrigerants,h =0 sat.liq.at-40C; u=h-pv Ch available in table but not u) Different ref.state for different substances;ref.state cancels out 上游通大学 March 16,2018 4 SHANGHAI JLAO TONG UNIVERSITY
March 16, 2018 4 Reference states and reference values Recall reference values for PE, KE u,h,s cannot be measured directly; p,T,v can be measured directly Changes in u,h,s computed using derived relations with p, T, v Note: only changes can be computed, not u, h, s, at a specific state But fortunately in 1st and 2nd laws only changes in these properties are needed We choose a convenient reference state and set properties to zero at that state For water, u = 0, s = 0, at 0.01ºC for Sat. Liq. state; h=u+pv; could have negative values relative to reference; For ammonia, propane, refrigerants, h =0 sat. liq. at -40ºC; u=h-pv (h available in table but not u) Different ref. state for different substances; ref. state cancels out arbitrary

Example12.1 Stirring water at constant volume T1=100℃ Sat.vapor Water 1.5 bars stir 0.25m3 1.014 bars P2=1.5 bar 100C Boundary insulated Find:(a)T2;(b)W Assumptions: 1.5 bars 1.The water is a closed system. 2.The initial and final states are at equilibrium. 1.014 bars There is no net change in kinetic or potential energy. 100°C 3.There is no heat transfer with the surroundings. 4.The tank volume remains constant. 上游充通大 March 16,2018 5 SHANGHAI JLAO TONG UNIVERSITY
March 16, 2018 5 Example12.1 Stirring water at constant volume insulated 0.25m3 p2=1.5 bar T1=100 ̊C Sat. vapor stir Find: (a) T2 ; (b) W
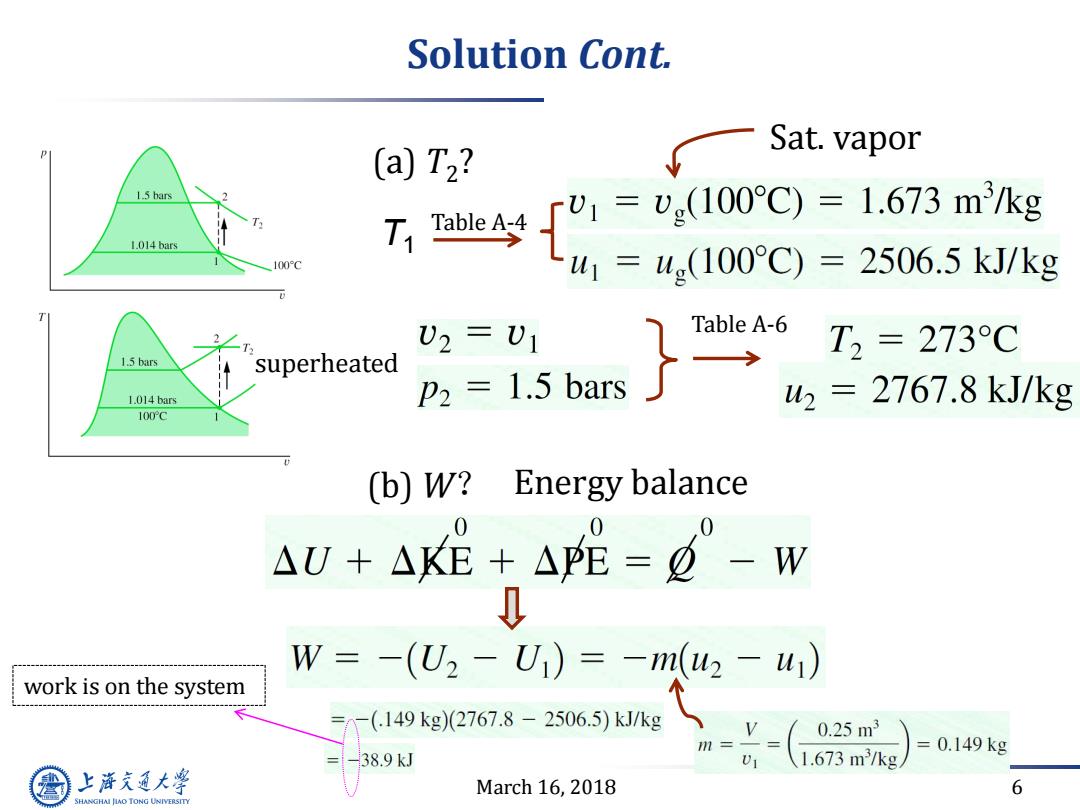
Solution Cont. Sat.vapor (a)T2? 1.5 bars 1.014 bars 74[%-,10r0=L.673m水g 100C -u =u(100°C)=2506.5kJ/kg U2=U1 Table A-6 2 T, T2=273C 1.5 bars superheated 1.014 bars P2 1.5 bars 2=2767.8kJ/kg 100fC (b)W? Energy balance AU+AKE+A呢=-w W=-(U2-Ui)=-mu2-u1) work is on the system -(149kg)(2767.8-2506.5)kJ/kg v( 0.25m3 0.149kg 38.9kJ 1.673m3/kg 上游充通大 March 16,2018 6 SHANGHAI JIAO TONG UNIVERSITY
March 16, 2018 6 Solution Cont. Table A-4 Sat. vapor Table A-6 superheated (a) T2 ? (b) W? Energy balance work is on the system T1

Example 12.2 Analyzing two processes in series Not insulated P1=10 bar Process 1-2:water cooled as compressed at a constant pressure of 10 bar to the saturated vapor state. T1=400°℃ Process 2-3:water cooled at constant volume to 150C. -Boundary T1=400°C>Tsat(p1=10bar)=179.9℃ Find: D (a)Sketch both processes on T-v State 1:superheated 10 bar and p-v diagrams. 400C 400°C (b)For the overall process 10bar 179.9C determine the work,in kJ/kg. 179.9C 4.758bar (c)For the overall process 4.758bar 150°C 150°C determine the heat transfer,in kJ/kg. 上降文通大学 March 16,2018 7 SHANGHAI JIAO TONG UNIVERSITY
March 16, 2018 7 Example 12.2 Analyzing two processes in series Not insulated p1=10 bar T1=400 ̊C Find: (a) Sketch both processes on T–v and p–v diagrams. (b) For the overall process determine the work, in kJ/kg. (c) For the overall process determine the heat transfer, in kJ/kg. Process 1–2: water cooled as compressed at a constant pressure of 10 bar to the saturated vapor state. Process 2–3: water cooled at constant volume to 150 ̊C. T1=400 ̊C > T p1=10 bar sat( )= 179.9 ̊C State 1: superheated
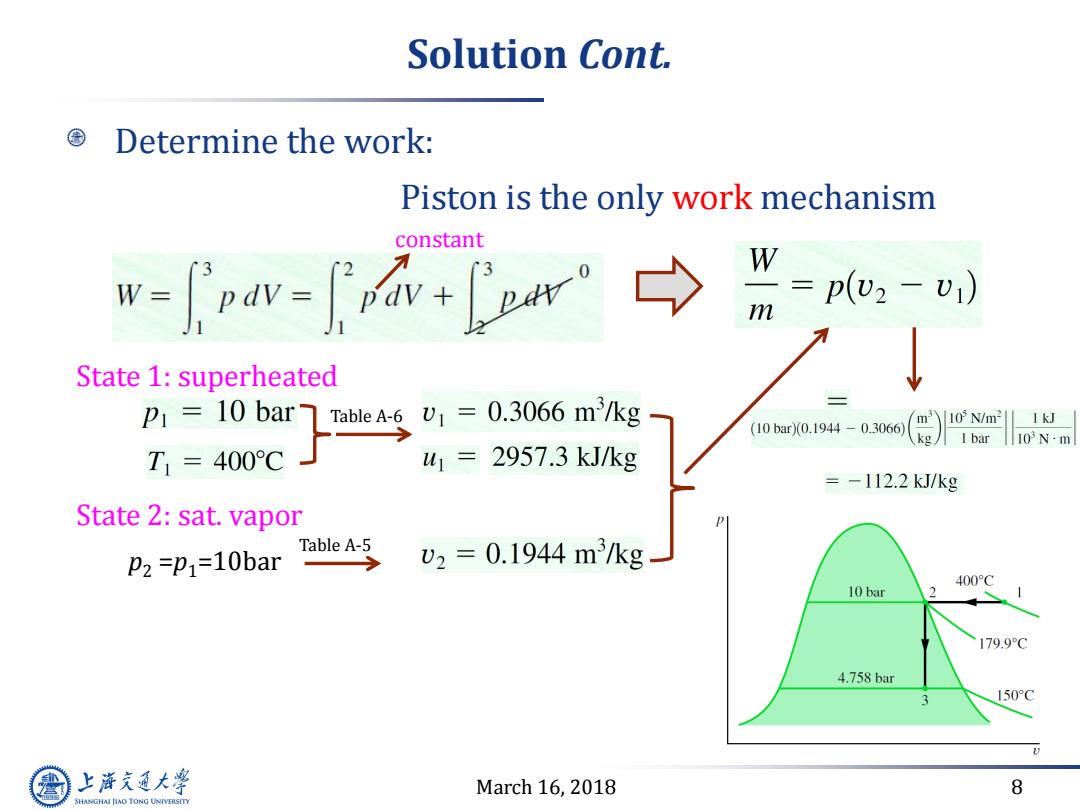
Solution Cont. Determine the work: Piston is the only work mechanism constant w-frav-Frivtpow W =p(2-v1) m State 1:superheated PI=10 bar Table A-6 v1 =0.3066 m3/kg (10bar0.1944-0.3066) m10 N/m2 1 kJ kg I bar 10'N-m T1=400C w1=2957.3kJ/kg =-112.2kJ/kg State 2:sat.vapor D2=p:=10bar TableAS v2=0.1944m3/kg 400C 10 bar 179.9C 4.758bar 150°C 上游充通大学 March 16,2018 8 SHANGHAI JIAO TONG UNIVERSITY
March 16, 2018 8 Solution Cont. Determine the work: Piston is the only work mechanism constant Table A-6 State 1: superheated State 2: sat. vapor p2 =p1=10bar Table A-5
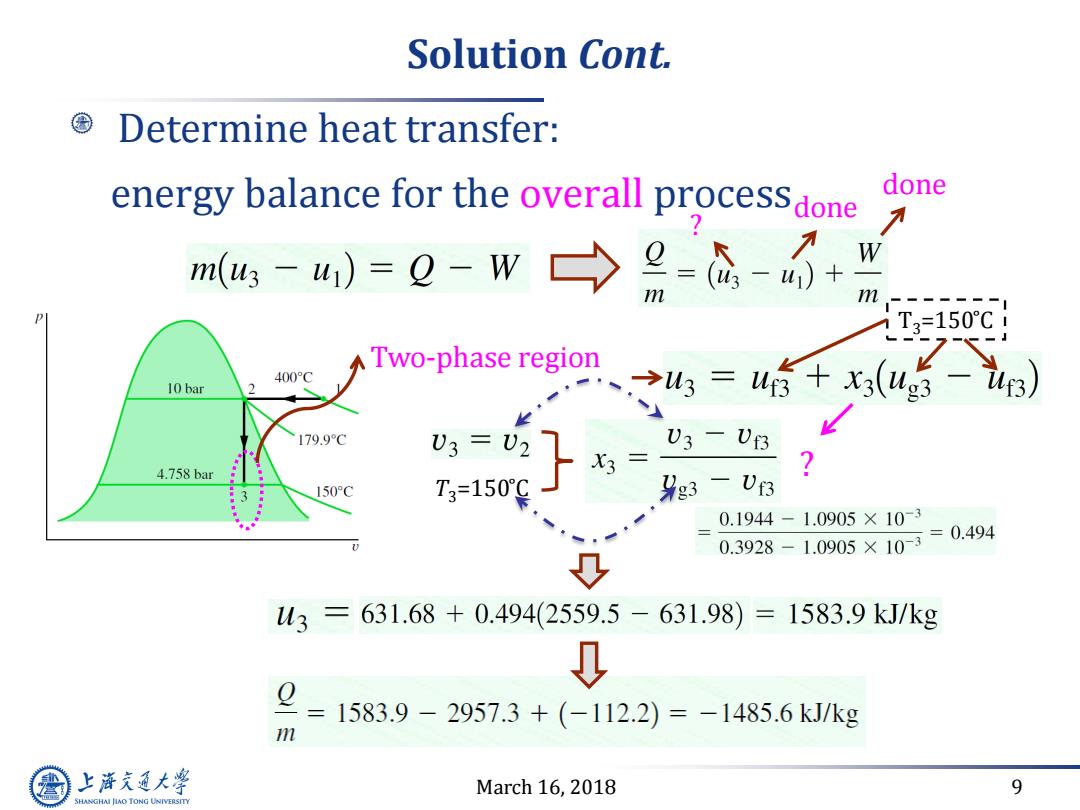
Solution Cont. Determine heat transfer: energy balance for the overall processdone done m(g3-u)=Q-W→ 9- W m T3=150C ATwo-phase region 400°C 10bar 、34=4+x,(u5) 179.9C 3= U3-U3 4.758bar 150°C T3=150C 9g3-U3 0.1944-1.0905×10-3 0.3928-1.0905×10=0.494 3=631.68+0.494(2559.5-631.98)=1583.9kJ/kg Q 1583.9-2957.3+(-112.2)=-1485.6kJ/kg m 上游充通大学 March 16,2018 9 SHANGHAI JIAO TONG UNIVERSITY
March 16, 2018 9 Solution Cont. Determine heat transfer: energy balance for the overall processdone done ? Two-phase region T3=150 ̊C ? T3=150 ̊C

Evaluating Specific Heats c,and cp specific heats (or specific heat capacities) defined for pure,simple compressible substances Ou(T,v) @h(T,p) Spectroscopic C,= ∂T Cp= ot measurement SI units are kJ/kg·K or kJ/kmol·K. intensive properties Specific heat data are available for common gases, liquids,and solids. Cp specific heat ratio k= Cv 上游通大学 March 16,2018 10 SHANGHAI JLAO TONG UNIVERSITY
March 16, 2018 10 Evaluating Specific Heats cv and cp defined for pure, simple compressible substances intensive properties Specific heat data are available for common gases, liquids, and solids. specific heat ratio ( , ) ( , ) v p v p u T v h T p c c T T Spectroscopic measurement specific heats (or specific heat capacities)