
上游充通大学 SHANGHAI JIAO TONG UNIVERSITY Engineering Thermodynamics I Lectures 16 Chapter 5 Mass and Energy Analysis of Control Volume Analysis Spring,3/28/2018 Prof.,Dr.Yonghua HUANG 强 。 http://cc.sjtu.edu.cn/G2S/site/thermo.html 1日
Engineering Thermodynamics I Lectures 16 Spring, 3/28/2018 Prof., Dr. Yonghua HUANG Chapter 5 Mass and Energy Analysis of Control Volume Analysis http://cc.sjtu.edu.cn/G2S/site/thermo.html

Closed system/Open system Closed system(CM) Open system (CV) P2,T2,V2 T State 1 ---> pT,v inlet exit Pr Ty Vi m Besides 0&W,energy accompanying State 2 T2 mass as it enters or exits. Objective of Chap.5:develop illustrate the use of the CV forms of the conservation of mass and conservation of energy 上游究通大学 March 28,2018 2 SHANGHAI JLAO TONG UNIVERSITY
March 28, 2018 2 Closed system/Open system Closed system(CM) Open system (CV) State 1 State 2 p1 , T1 , v1 p2 , T2 , v2 m p1 , T1 , v1 p2 , T2 , v2 inlet exit Objective of Chap. 5: develop & illustrate the use of the CV forms of the conservation of mass and conservation of energy Q W Besides Q & W, energy accompanying mass as it enters or exits. pi , Ti , vi pe , Te , ve
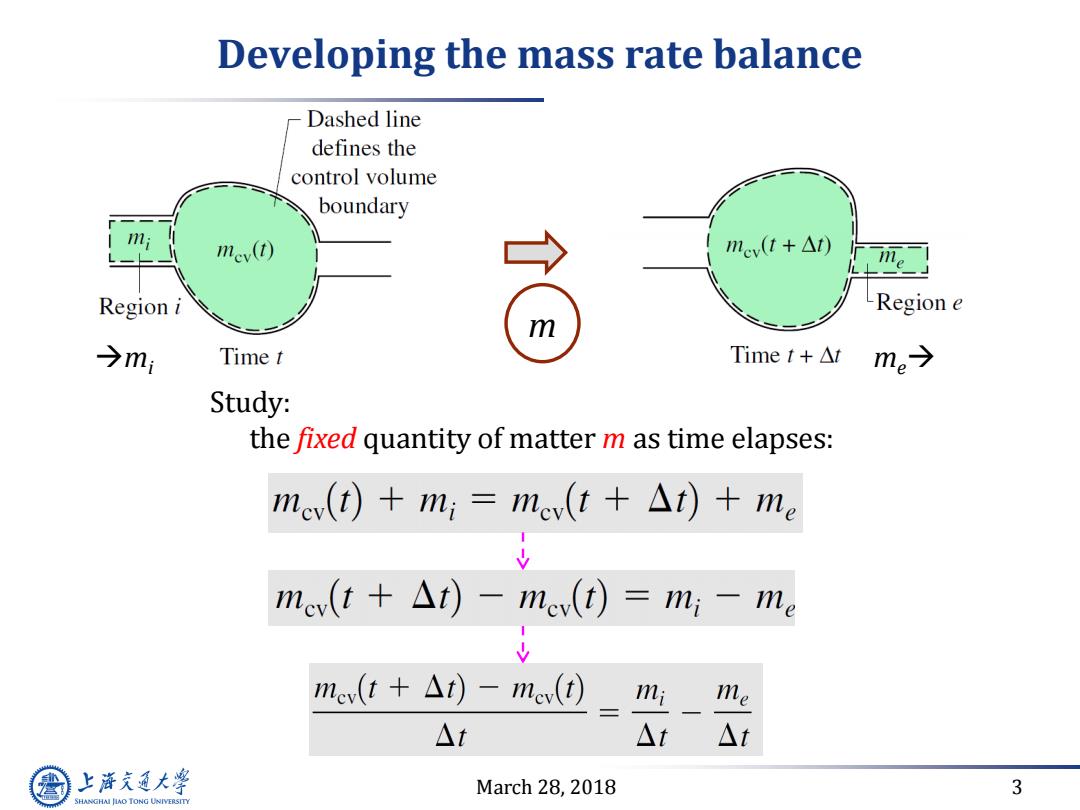
Developing the mass rate balance Dashed line defines the control volume boundary mi nev(①) nev(t+△) Region i LRegion e m →m; Time t Time t+△t me→ Study: the fixed quantity of matter m as time elapses: mev(t)+mi=mev(t At)+me mev(t At)-moy(t)=mi-me me(t+△t)-nev(t) mi me △t △t△t 上游充通大 March 28,2018 3 SHANGHAI JIAO TONG UNIVERSITY
March 28, 2018 3 Developing the mass rate balance Study: the fixed quantity of matter m as time elapses: mi me m

conservation of mass principle mn=0 Dashed line defines the control volume mi boundary One-inlet,one-exit control volume system mev(t) > me Inlet i mcv(t+△t)-mev(t) mi me Exit e △t △t △t At time t time rate of change of time rate of flow time rate of flow mass contained within of mass in across of mass out across the control volume at time t inlet i at time t exit e at time t Multi-streams dmev At time t: dt mi me → Mass flow rate (SI units:kg/s) 上游充通大 March 28,2018 4 SHANGHAI JIAO TONG UNIVERSITY
March 28, 2018 4 conservation of mass principle At time t mcv(t) mi me At time t: Mass flow rate (SI units: kg/s) Multi-streams One-inlet, one-exit control volume system

Evaluating the mass flow rate mi velocitys、 time interval dm dA Volume Control volume (CV) of matter Incremental area Control surface (CS) amount of mass A:area crossing dA during =pVn△t)dA the time interval△t Divided by△t ≥(N.a) Muti-streams instantaneous rate Integration over A of mass flow =pVn dA 防= PVn dA across dA 上究大学 March 28,2018 5 SHANGHAI JIAO TONG UNIVERSITY
March 28, 2018 5 Evaluating the mass flow rate m velocity time interval : area Incremental area Divided by ∆t Integration over A Muti-streams
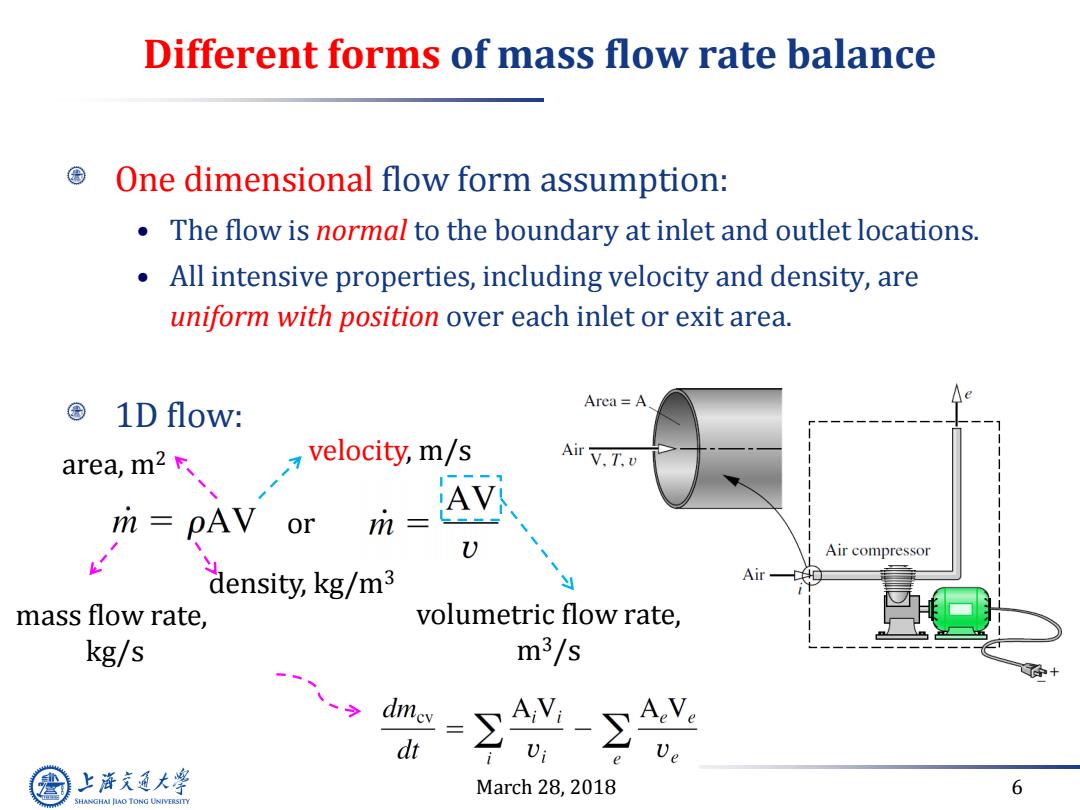
Different forms of mass flow rate balance One dimensional flow form assumption: The flow is normal to the boundary at inlet and outlet locations. All intensive properties,including velocity and density,are uniform with position over each inlet or exit area. 1 D flow: Area=A area,m2、 velocity,m/s Air V.T.v m=pAV or Air compressor density,kg/m3 Air mass flow rate, volumetric flow rate, kg/s m3/s dt U: 上游充通大学 March 28,2018 6 SHANGHAI JLAO TONG UNIVERSITY
March 28, 2018 6 Different forms of mass flow rate balance One dimensional flow form assumption: • The flow is normal to the boundary at inlet and outlet locations. • All intensive properties, including velocity and density, are uniform with position over each inlet or exit area. 1D flow: or velocity, m/s area, m2 density, kg/m3 mass flow rate, kg/s volumetric flow rate, m3/s
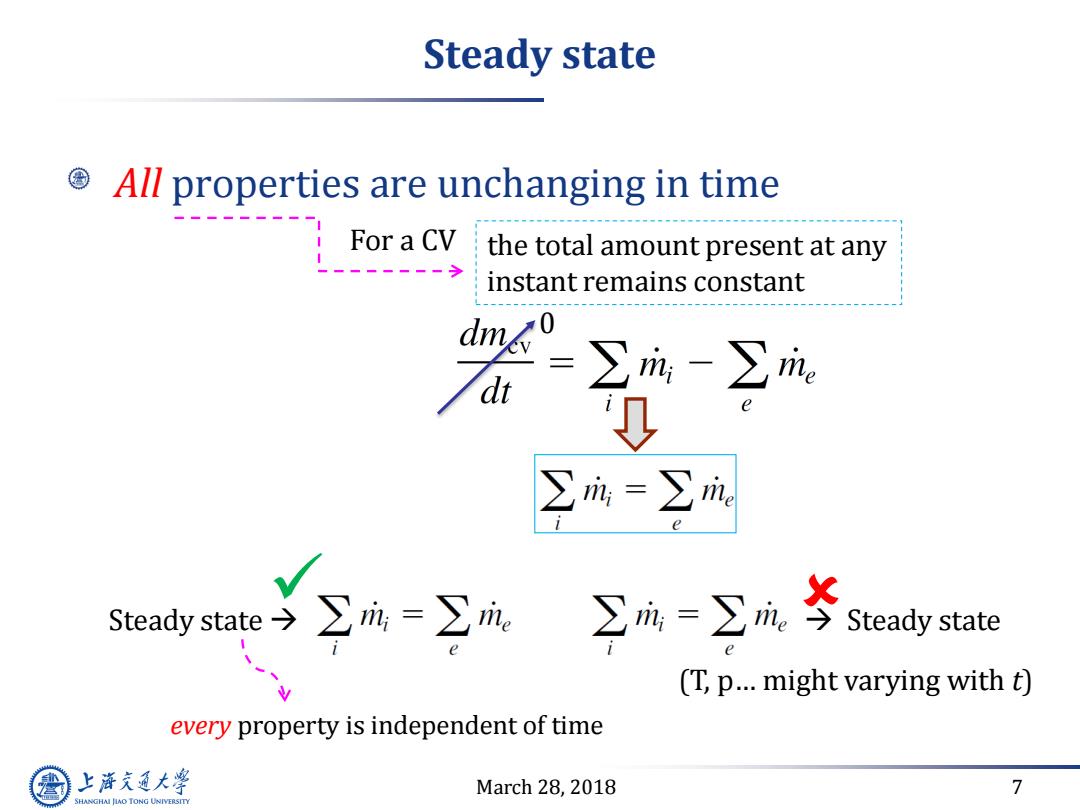
Steady state All properties are unchanging in time For a CV the total amount present at any instant remains constant ∑=∑m Steady sate今∑m=∑m】 ∑m=∑成芩Sady stae (T,p...might varying with t) every property is independent of time 上游充通大 March 28,2018 7 SHANGHAI JLAO TONG UNIVERSITY
March 28, 2018 7 Steady state All properties are unchanging in time For a CV the total amount present at any instant remains constant Steady state Steady state (T, p… might varying with t) every property is independent of time 0

Example 16.1:Steady flow feedwater heater,Steady State (SS) b Find:Determine mz,m,and the velocity V2. A2=25cm2 T1=200C T2=40C P1=7 bar rin =40 kg/s Solution: (AV)3 0.06m3/s P2=7 bar m3= (1.108×10-3m3/kg) =54.15kg/s 3 个 Control volume boundary Saturated liquid P3 =7bar,@sat.liq.>Table A-5 P3 7 bar (AV)3=0.06m3/s =∑m一∑m: dt 0 given dm dt m1+m2一m3 ? m2=m3-m1=54.15-40=14.15kg/s 上游充通大 March 28,2018 8 SHANGHAI JIAO TONG UNIVERSITY
March 28, 2018 8 Example 16.1: Steady flow feedwater heater, Steady State (SS) Find: Determine , , and the velocity V2 . m2 m3 Solution: ? p3 =7bar, @sat. liq. Table A-5 given
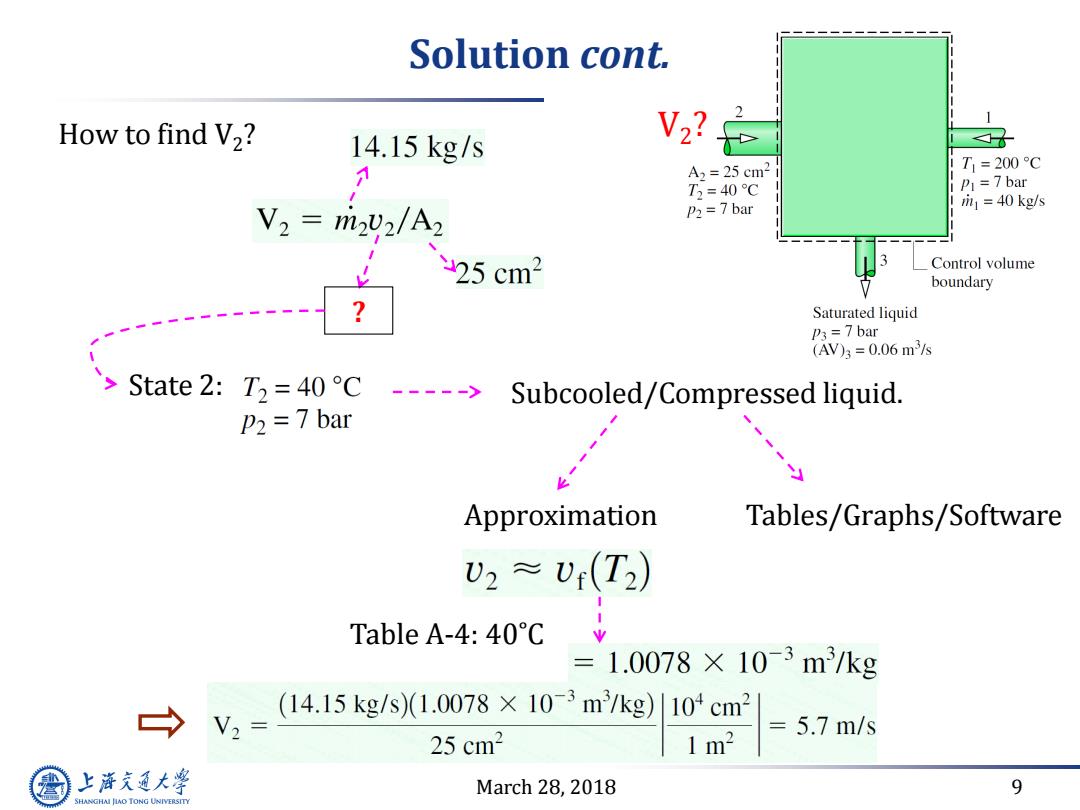
Solution cont. How to find V2? 14.15kg/s T=200C 1 A2=25cm2 T2=40C P1=7bar P2=7bar rin =40 kg/s V2=m22/A2 25cm2 Control volume boundary Saturated liquid P3=7bar (AV)3=0.06m3/s > State2:T2=40C----> Subcooled/Compressed liquid. P2 7 bar Approximation Tables/Graphs/Software U2≈U(T2) Table A-4:40°C =1.0078×10-3m3/kg (14.15kg/s)(1.0078×10-3m/kg)104cm2 V2= 5.7m/s 25cm2 1m2 上游充通大 March 28,2018 9 SHANGHAI JIAO TONG UNIVERSITY
March 28, 2018 9 Solution cont. ? State 2: Subcooled/Compressed liquid. Approximation Tables/Graphs/Software Table A-4: 40˚C How to find V2 ? V2 ?
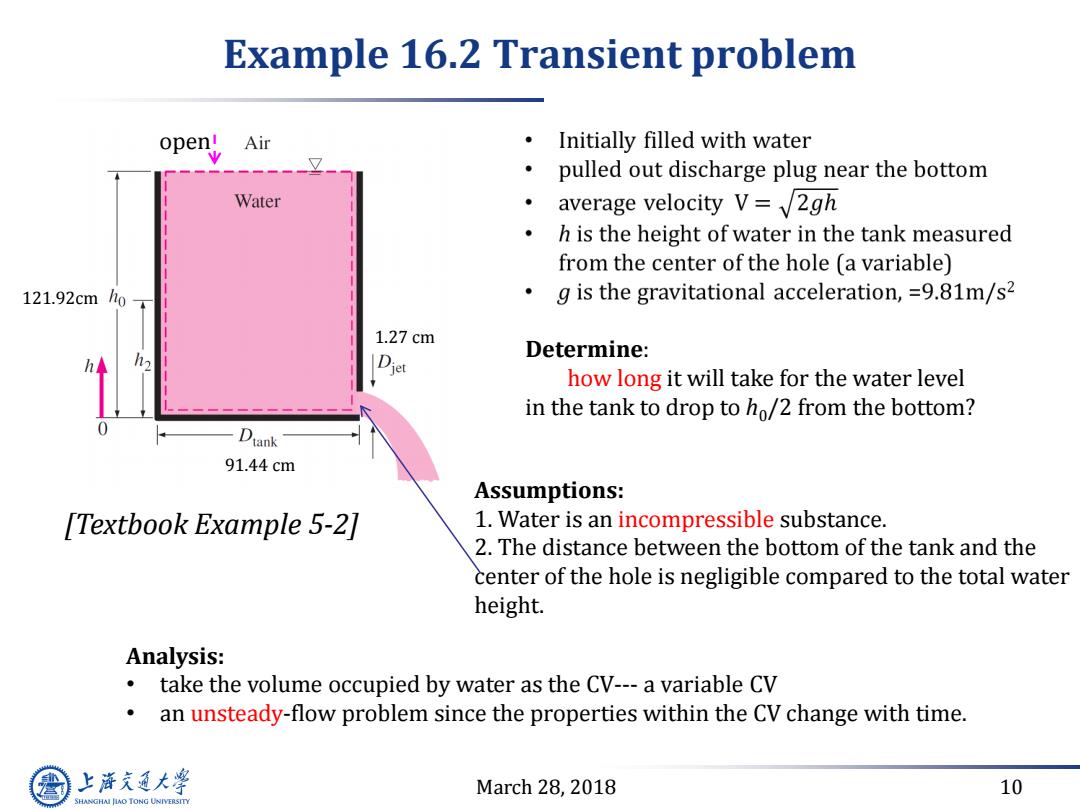
Example 16.2 Transient problem open! Air Initially filled with water pulled out discharge plug near the bottom Water ·average velocity V=√2gh h is the height of water in the tank measured from the center of the hole (a variable) 121.92cmh07 g is the gravitational acceleration,=9.81m/s2 1.27cm Determine: e how long it will take for the water level in the tank to drop to ho/2 from the bottom? 0 Dtank 91.44cm Assumptions: [Textbook Example 5-2] 1.Water is an incompressible substance. 2.The distance between the bottom of the tank and the center of the hole is negligible compared to the total water height. Analysis: take the volume occupied by water as the CV---a variable CV an unsteady-flow problem since the properties within the CV change with time. 上降文通大学 March 28,2018 10 SHANGHAI JIAO TONG UNIVERSITY
March 28, 2018 10 Example 16.2 Transient problem 121.92cm 91.44 cm open 1.27 cm Determine: how long it will take for the water level in the tank to drop to h0/2 from the bottom? Assumptions: 1. Water is an incompressible substance. 2. The distance between the bottom of the tank and the center of the hole is negligible compared to the total water height. Analysis: • take the volume occupied by water as the CV--- a variable CV • an unsteady-flow problem since the properties within the CV change with time. [Textbook Example 5-2]