
Chapter 10 Multirate Digital Signal Processing
Chapter 10 Multirate Digital Signal Processing
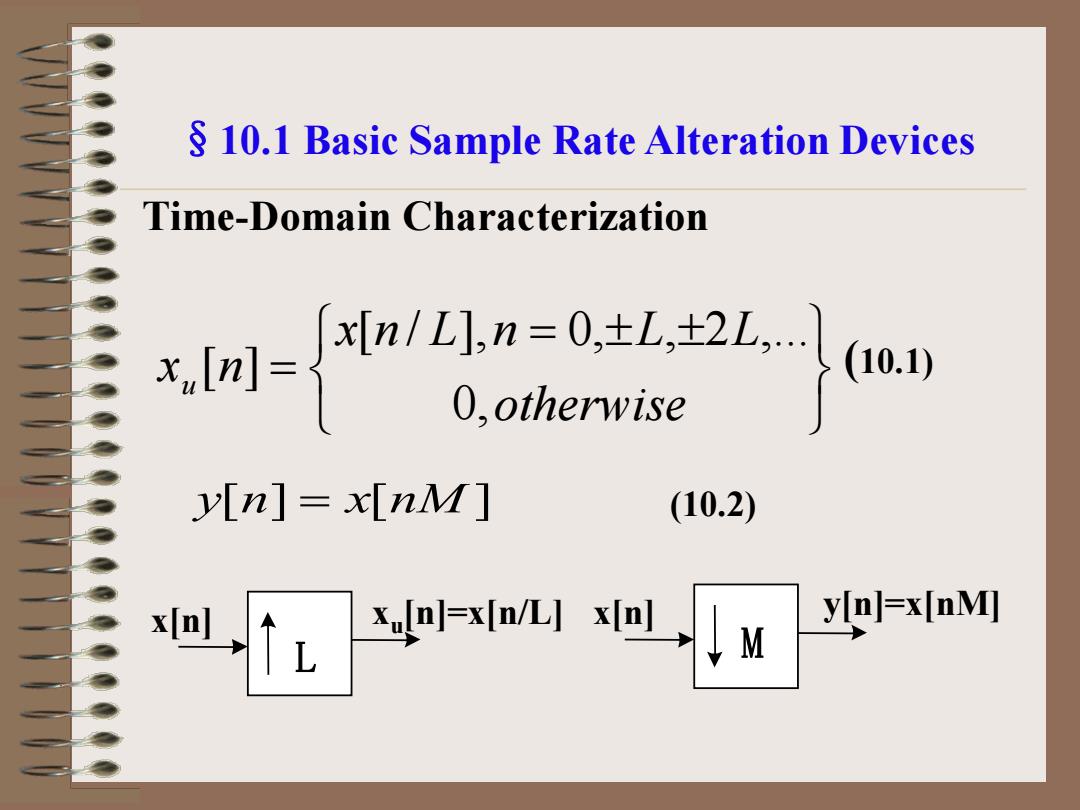
S 10.1 Basic Sample Rate Alteration Devices Time-Domain Characterization 内-2n 0,otherwise y[n]=x[nM] (10.2) u↓M yln]=x[nM]
§10.1 Basic Sample Rate Alteration Devices = ± ± = otherwise x n L n L L xu n 0, [ / ], 0, , 2 ,... [ ] (10.1) y[n] = x[nM ] (10.2) Time-Domain Characterization L xu x[n] [n]=x[n/L] M x[n] y[n]=x[nM]
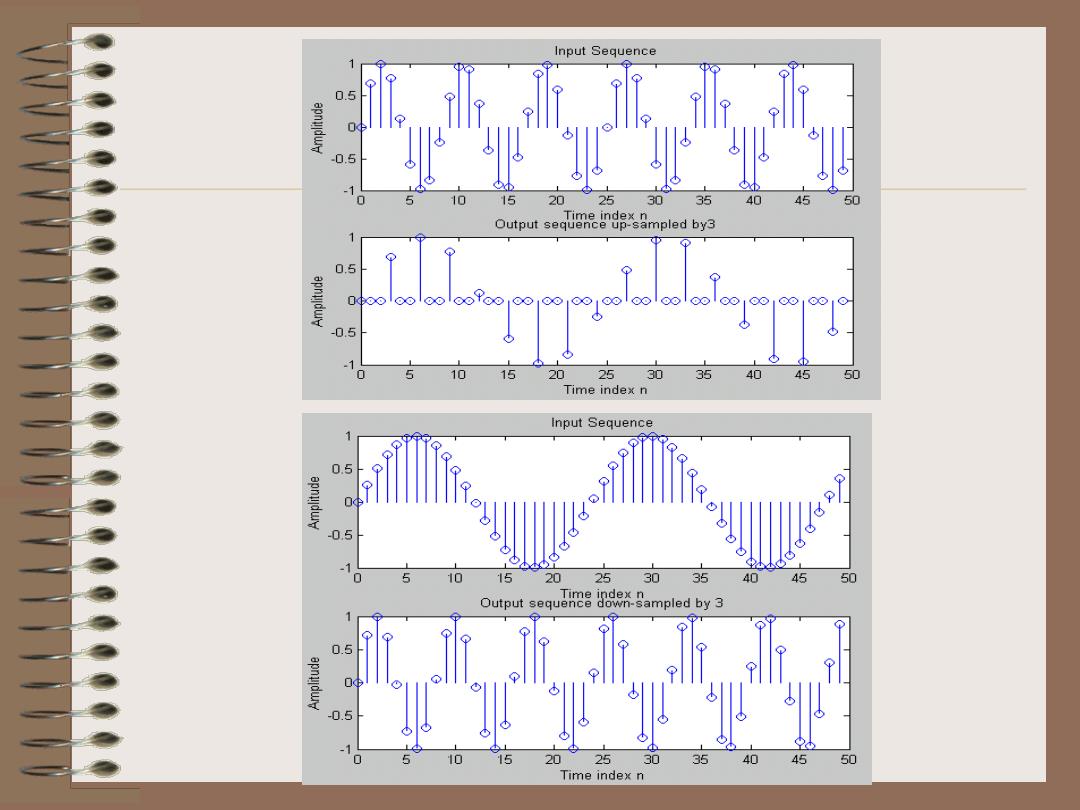
Input Sequence 派 业 20 25 30 35 Time index n. Output sequence up-sampled by3 001 5 10 15 20 25 30 35 40 45 50 Time index n Input Sequence epntdu 15 20 25 30 35 40 50 Time index n Output sequence down-sampled by 3 it I Tr 20 35 50 Time index n
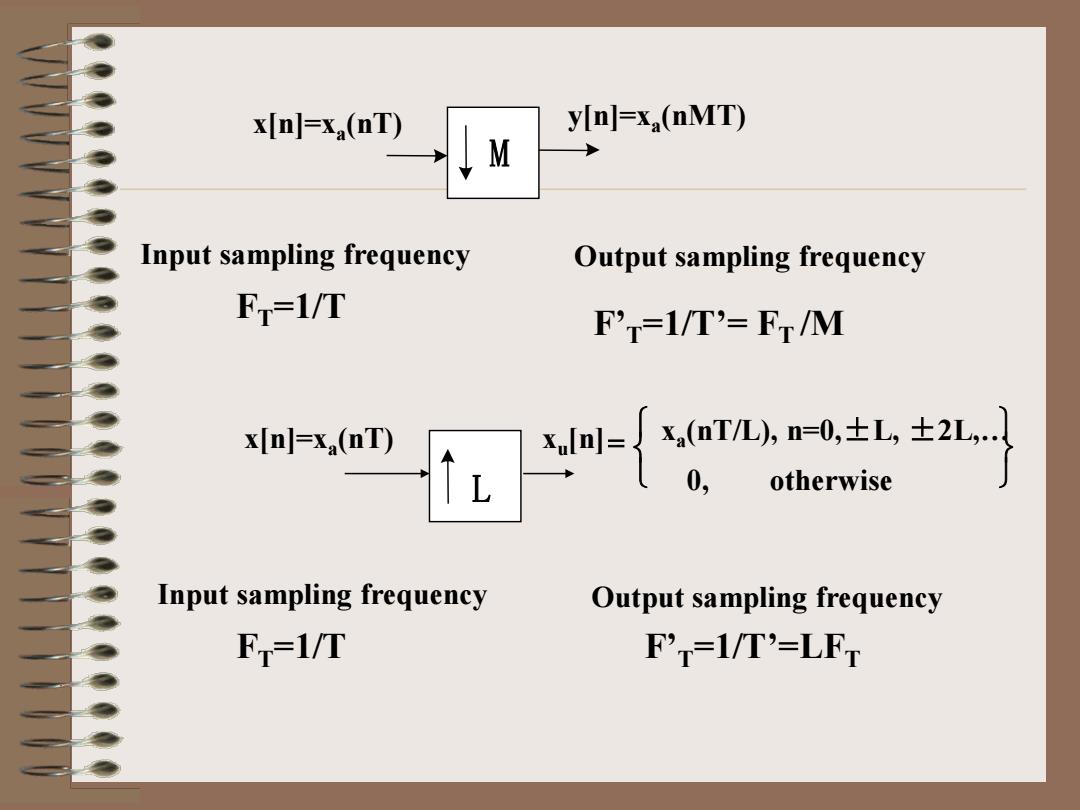
x[n]=xa(nT) y[n]=x(nMT) M Input sampling frequency Output sampling frequency FT=1/T F'T=1/T'=FT/M x[n]=xa(nT) 0. Input sampling frequency Output sampling frequency F=1/T F'T=1/T'=LFT
Input sampling frequency FT=1/T Output sampling frequency F’T=1/T’=LFT L = xa(nT/L), n=0,±L, ±2L,… 0, otherwise xu x[n]=x [n] a(nT) FT=1/T Input sampling frequency F’T=1/T’= FT /M Output sampling frequency M x[n]=xa(nT) y[n]=xa(nMT)
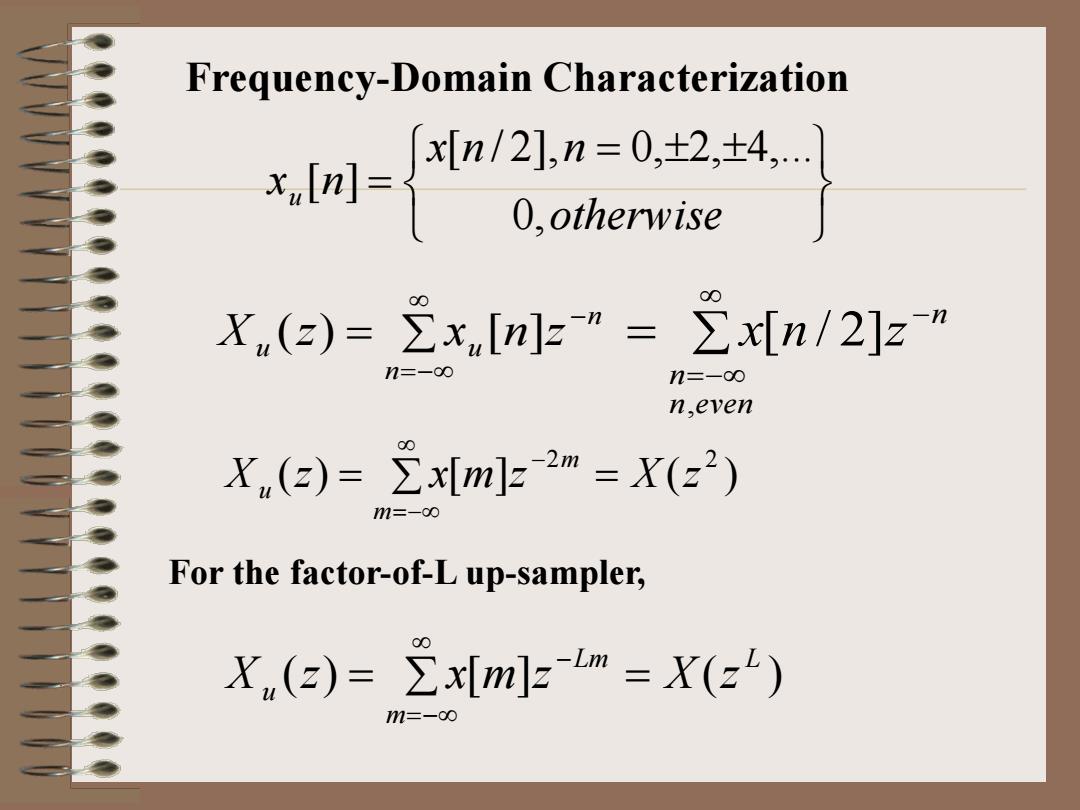
Frequency-Domain Characterization x[n/2],n=0,+2,+4,… 5.0 0,otherwise ● 00 Xu(z)=∑x[n]z"=∑x[n/2]z" n=-o0 n=-00 n,even X(z)=∑x[m]z2m=X(z2) m=-00 For the factor-of-L up-sampler, Xu(z)=∑x[m]zm=X(z) m=-o0
Frequency-Domain Characterization = ± ± = otherwise x n n xu n 0, [ / 2], 0, 2, 4,... [ ] = ∑ ∞ =−∞ − n n u u X (z) x [n]z = ∑ ∞ =−∞ − n even n n x n z , [ / 2] ( ) [ ] ( ) 2 2 X z x m z X z m m u = ∑ = ∞ =−∞ − For the factor-of-L up-sampler, ( ) [ ] ( ) L m Lm u X z = ∑ x m z = X z ∞ =−∞ −
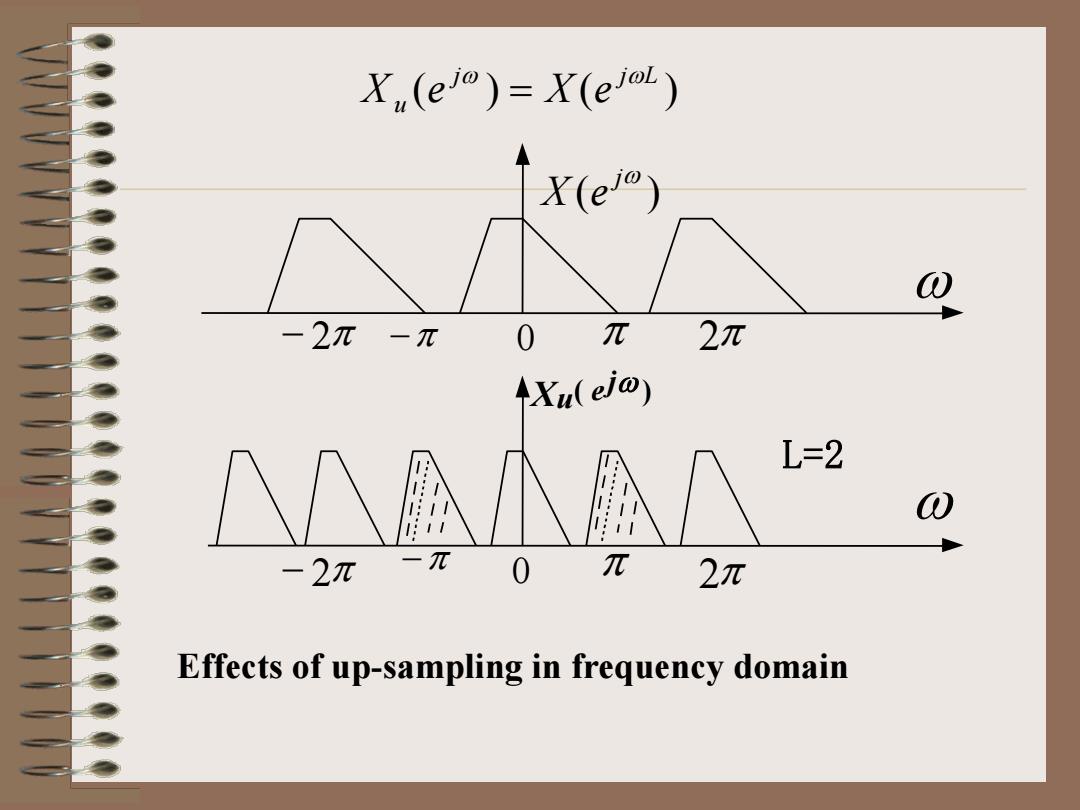
X(e)=X(ej) X(e) 0 -2π-元 0 π 2π AXu(ej@) L=2 -2π 0 π 2π Effects of up-sampling in frequency domain
( ) ( ) j j L u X e X e ω ω = Effects of up-sampling in frequency domain ( ) jω X e ω − 2π −π 0 π 2π ( ω ) j u X e L=2 ω 0 π 2π −π − 2π
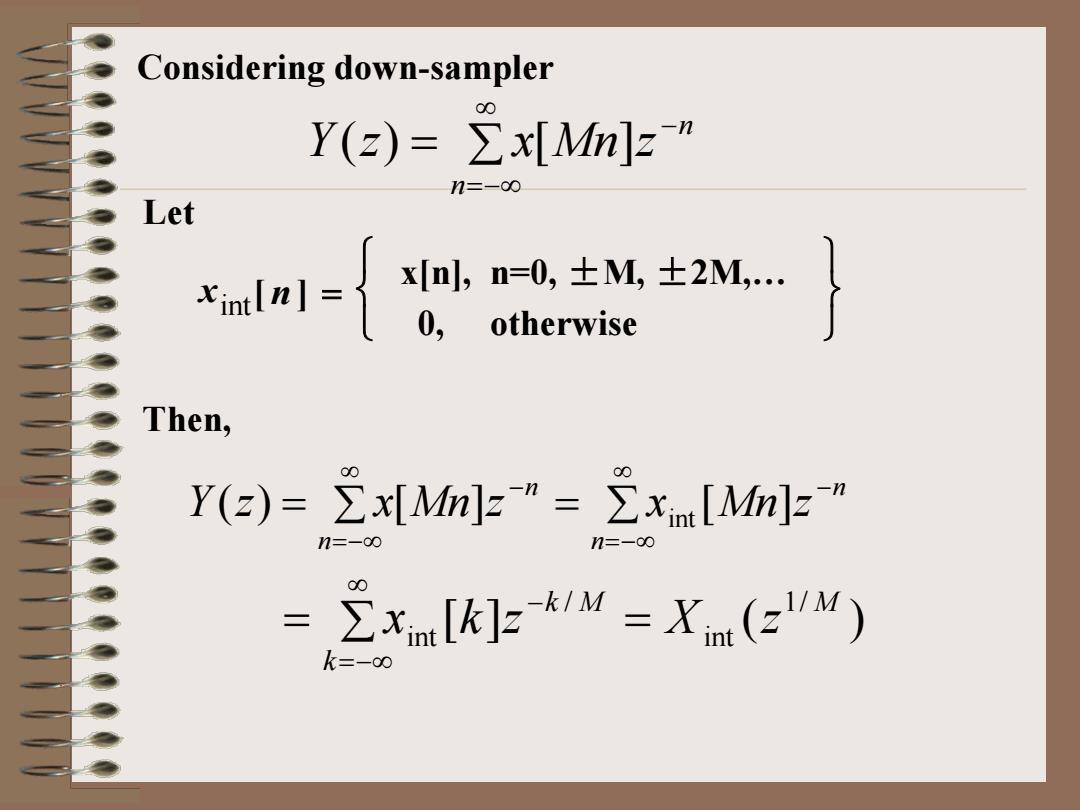
Considering down-sampler Y(z)=∑x[Mm]z” n=-00 Let Then, 00 00 Y(e)=∑x[M例]z"=∑xn[Mim]z" n=-o0 00 = xt[k]z/M=Xn(zM) k=-00
Considering down-sampler = ∑ ∞ =−∞ − n n Y(z) x[Mn]z Let Then, = ∑ = ∑ ∞ =−∞ − ∞ =−∞ − n n n n Y(z) x[Mn]z x [Mn]z int [ ] ( ) 1/ int / int M k k M = ∑ x k z = X z ∞ =−∞ − x [ n ] = int x[n], n=0, ±M, ±2M,… 0, otherwise
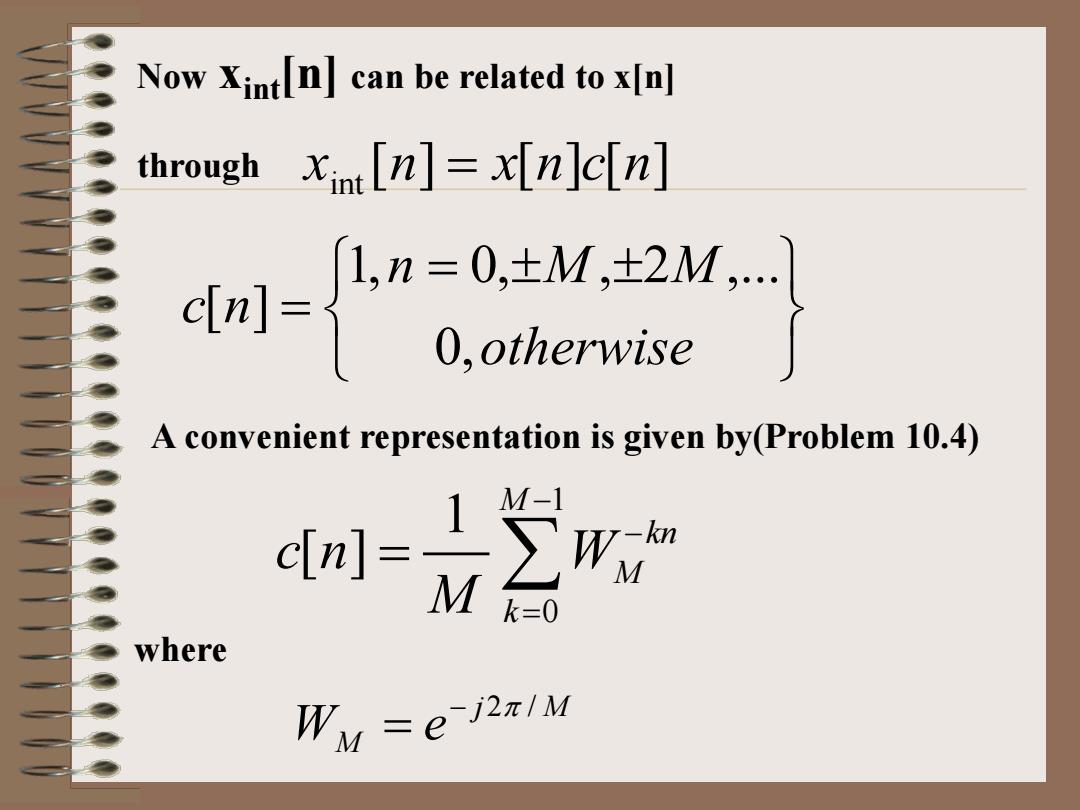
Now Xint n]can be related to x[n] through xint[n]=x[n]c[n] [1,n=0,±M,+2M,… 0,otherwise A convenient representation is given by(Problem 10.4) 时2r M-1 k=0 where Wy =e-i2zIM
Now xint[n] can be related to x[n] through [ ] [ ] [ ] xint n = x n c n = ± ± = otherwise n M M c n 0, 1, 0, , 2 ,... [ ] A convenient representation is given by(Problem 10.4) 1 0 1 [ ] M kn M k cn W M − − = = ∑ where j M M W e− 2π / =
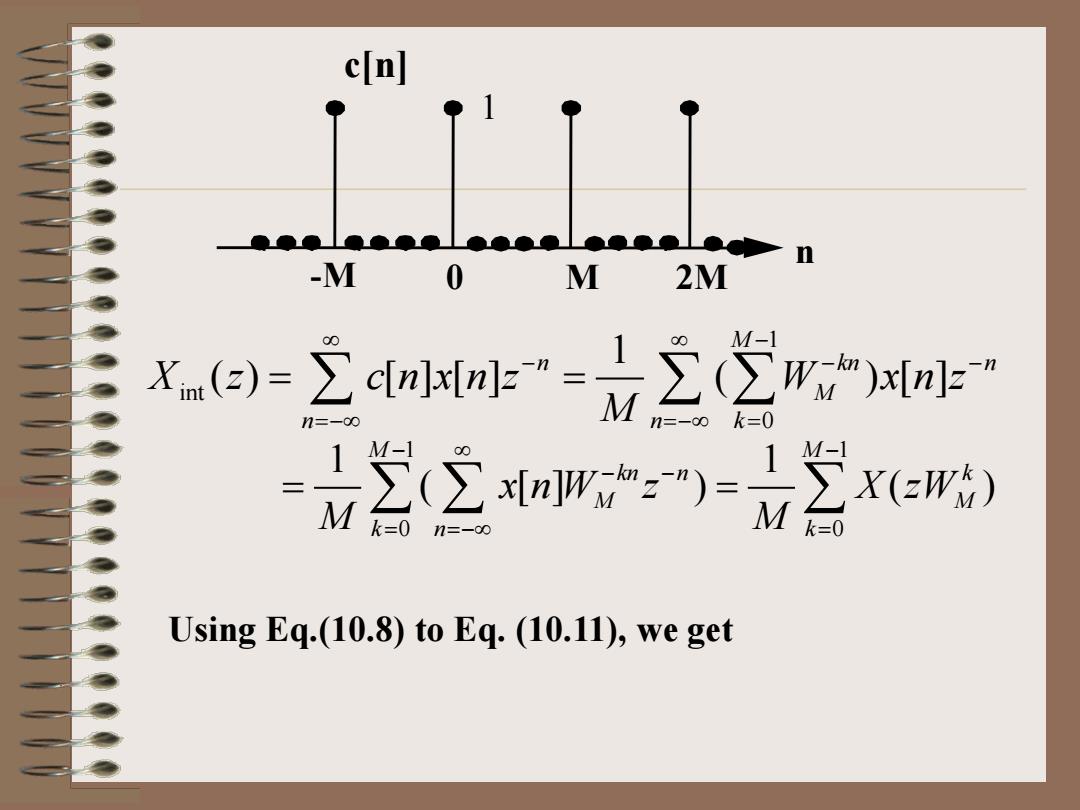
c n -M 0 M 2M X.e)立eaa啡立2m,a 00 M-1 n=-00 n=-o0k=0 22r*) M ∑X(zW) k=0 n=-00 =0 Using Eq.(10.8)to Eq.(10.11),we get
1 int 0 1 () [ ][ ] ( )[ ] M n kn n M n n k X z cnxnz W xnz M ∞ ∞ − − − − =−∞ =−∞ = = ∑ ∑∑ = 1 1 0 0 1 1 ( [] ) ( ) M M kn n k M M k n k x n W z X zW M M − ∞ − − − = =−∞ = = ∑∑ ∑ = Using Eq.(10.8) to Eq. (10.11), we get -M 0 M 2M 1 c[n] n
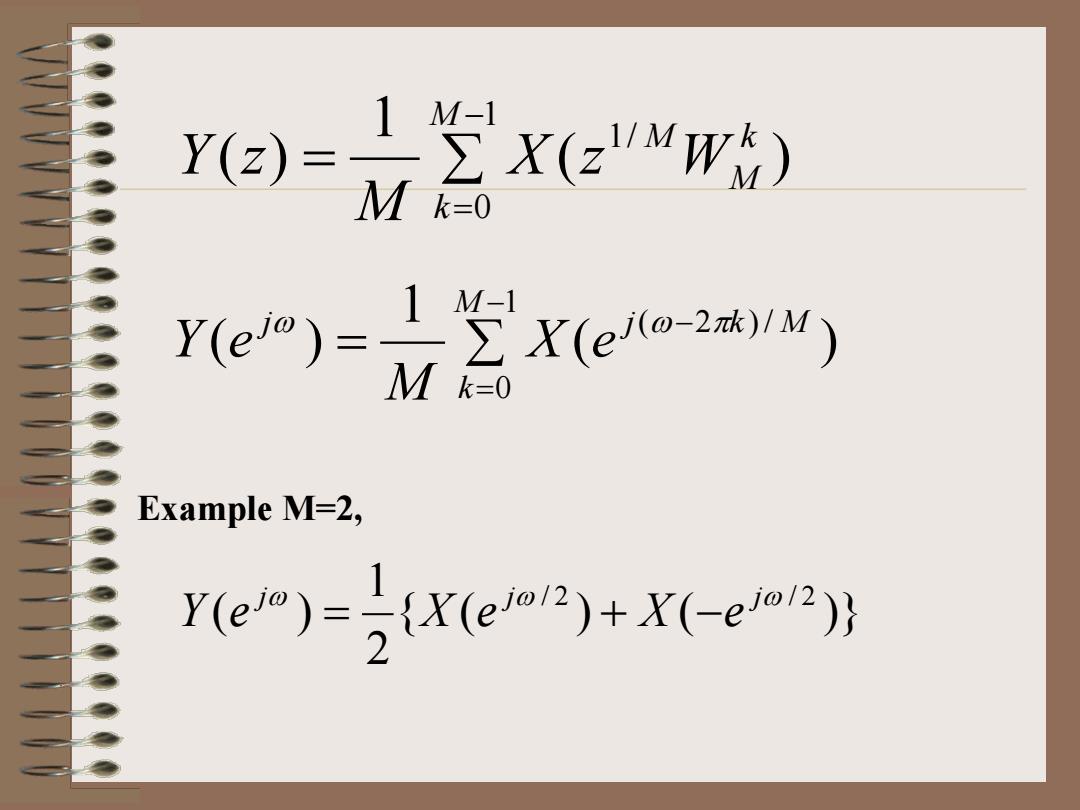
1 M-1 Y(z)=∑X(zMW) M=0 e)点ewsM Example M=2, e)-Ke)+x-emy
= ∑ − = 1 0 1/ ( ) 1 ( ) M k k M M X z W M Y z = ∑ − = − 1 0 ( 2 )/ ( ) 1 ( ) M k j j k M X e M Y e ω ω π Example M=2, { ( ) ( )} 2 1 ( ) jω jω / 2 jω / 2 Y e = X e + X −e