Chapter 6 Digital transmission through band-limited AWGn channels by Prof.XIAOFENG LI SCIE,UESTC UESTC-Digital transmission thro BL AWGN channels Prof李晓峰 1
Contents 5 Hrs ISI and zero-ISI condition (Refp380-381,p393-394) Design of BL signals for zero-ISI (Refp396-399) OFDM UESTC-Digital transmission thro BL AWGN channels Prof李晓峰 3
6.1 ISI and zero-ISI condition Consider the general block diagram as follows.Without loss of generality,we consider baseband (BB)case. C(f)=0,f八≤B。 G(f) c(f) G(f)) Det. a. y() {a} 8r() n(t) gx(1) Sym-timing Tx Channel Rx where, GT(f)represents the freq-characteristic of the transmission signal,which is determined by the pulse shape. C(f)the freq-response of the channel. GR(f)the freq-response of the receiving filter.(eg.the MF) The corresponding time representations are gr(t),c(t)and gR(t) respectively. UESTC-Digital transmission thro BL AWGN channels Prof李晓峰 5
6.1 ISI and zero-ISI condition C(f)=0,f≤B。 G(f) c(f) Gx(f) Det. a. y(t) {a} 8r() gx() Sym-timing Chanpel Rx Trans.Signal:s(1)>ag() Filtered sig: y(t)=r(t)*gx(1) agr(t-kT) =∑ag(-kT)*c()*g.)+n)*g() ∑ah(t-kT)+v() Received sig:r()=>ag(1-kT)*c(1) where,h(t)=g(t)*c()*gt)is the overall resp. k=00 +n(t) UESTC-Digital transmission thro BLAWGN channels Prof李晓峰 6

6.1 ISI and zero-ISI condition C(f)=0,f≤B。 G,(f) C() G:(f) Det. {a} y() {a} g(0 n(t)月 gR()) Sym-timing Tx ChanneL Rx Observation: The 1st term on RHS provides the nth symbol an. =y(nT)=>ah(nT-kT)+v(nT) The 3rd term represents additive noise. The 2nd term provides no info on an,and is an …+am-2h(2T)+abE)tah明 harmful interference,called Inter-Symbol +ah(T)ah(-2T) ..+v(nT Interference (ISI) =anh(0)+IsI+v(mT) UESTC-Digital transmission thro BLAWGN channels Prof李晓峰 9

6.1 ISI and zero-ISTii (t) G,(f) {a} gr(1) Tx The tails on both sides of h(7)out of the symbol interval cause the ISI. Observation: The 1st term on RHS provides the nth symbol an. n=y(nI)=∑ah(nI-kT)+v(nZ The 3rd term represents additive noise. The 2nd term provides no info on an,and is an …+am-2h(2T)+abE)tah明 harmful interference,called Inter-Symbol +ah(T)ah2T) ..+v(nT Interference (ISI) =anh(0)+Isi+v(mT) UESTC-Digital transmission thro BLAWGN channels Prof李晓峰 10
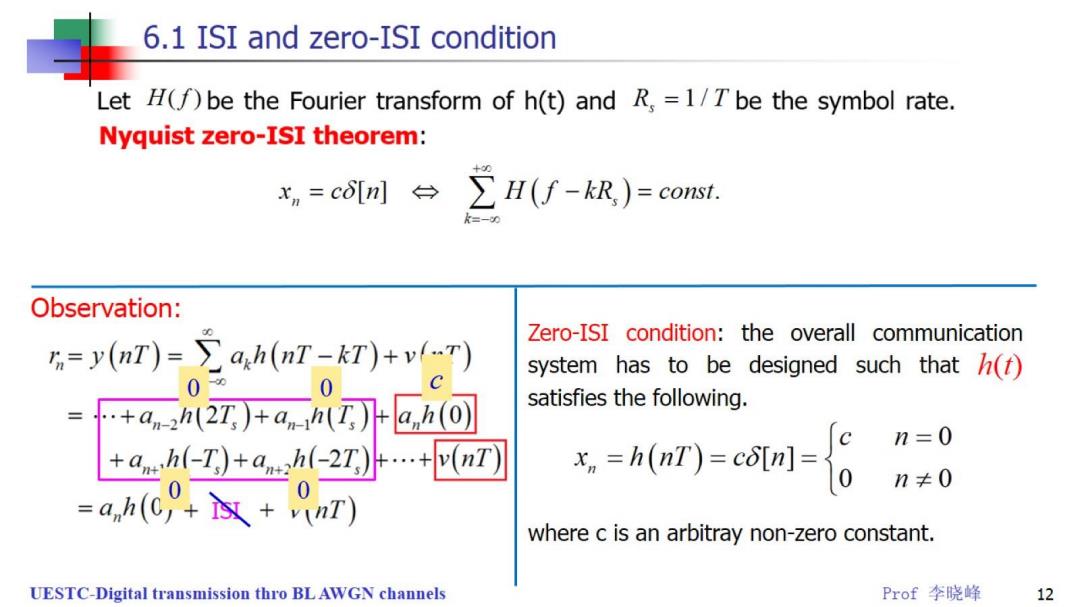
6.1 ISI and zero-ISI condition Let H()be the Fourier transform of h(t)and R,=1/T be the symbol rate. Nyquist zero-ISI theorem: xn=c6[n台 ∑H(f-kR)=cosi. Observation: Zero-ISI condition:the overall communication =y(nT)=>agh(nT-kT)+v(-) system has to be designed such that h() 0 0 ..+a2h(2T)+ah()+a,h(0) satisfies the following. n=0 +ah(-T)+amh(-2T)+... v(nT =ah00+汉+,9mn) 0 &=lwn=a5-6 n≠0 where c is an arbitray non-zero constant. UESTC-Digital transmission thro BLAWGN channels Prof李晓峰 12

6.1 ISI and zero-ISI condition Let H()be the Fourier transform of h(t)and R,=1/T be the symbol rate. Nyquist zero-ISI theorem: xn=co[n川台 ∑H(f-kR)=const. As shown in fig,the condition requires the summation yields a flat spectrum. Foris periodic with Zero-ISI condition:the overall communication Rs,we pay attention to the interval system has to be designed such that h() of(-R/2,+R,/2). satisfies the following. &=wn=a5-日 n=0 n≠0 where c is an arbitray non-zero constant. -R R UESTC-Digital transmission thro BLAWGN channels Prof李晓峰 13