Statistical Signal Processing Detection Estimation Theory Wenhui Xiong NCL UESTC
Statistical Signal Processing Detection & Estimation Theory Wenhui Xiong NCL UESTC
Outline ●Introduction Administration Information What is the Detection Estimation Problem? Applications of Detection Estimation Theory ●Varming Up--Review Vector Representation of Signal 。Stochastic Process 。Probability
Introduction Administration Information What is the Detection & Estimation Problem? Applications of Detection & Estimation Theory Warming Up---Review Vector Representation of Signal Stochastic Process Probability Outline 2
Admin.Info.(continue) 。Textbook: Steven M.Kay,Statistical Signal Processing vol.I, Steven M.Kay,Statistical Signal Processing vol.Il Prerequisite Probability ■Stochastic process ·Linear Algebra Signal System
Textbook: Steven M.Kay, Statistical Signal Processing vol. I, Steven M.Kay, Statistical Signal Processing vol. II Prerequisite Probability Stochastic process Linear Algebra Signal & System Admin. Info. (continue) 4
●Contents: Fundamentals on Detection Theory ·Hypothesis test Deterministic Random signal detection Signal with unknown parameters detection Fundamentals on Estimation Theory Deterministic unknown parameter estimation Random unknown parameter estimation
Contents: Fundamentals on Detection Theory • Hypothesis test • Deterministic & Random signal detection • Signal with unknown parameters detection Fundamentals on Estimation Theory • Deterministic unknown parameter estimation • Random unknown parameter estimation 5

Outline Introduction Administration Information What is the Detection Estimation Problem? Applications of Detection Estimation Theory 。Warming Up--Review Vector Representation of Signal 。Stochastic Process 。Probability
Introduction Administration Information What is the Detection & Estimation Problem? Applications of Detection & Estimation Theory Warming Up---Review Vector Representation of Signal Stochastic Process Probability Outline 6
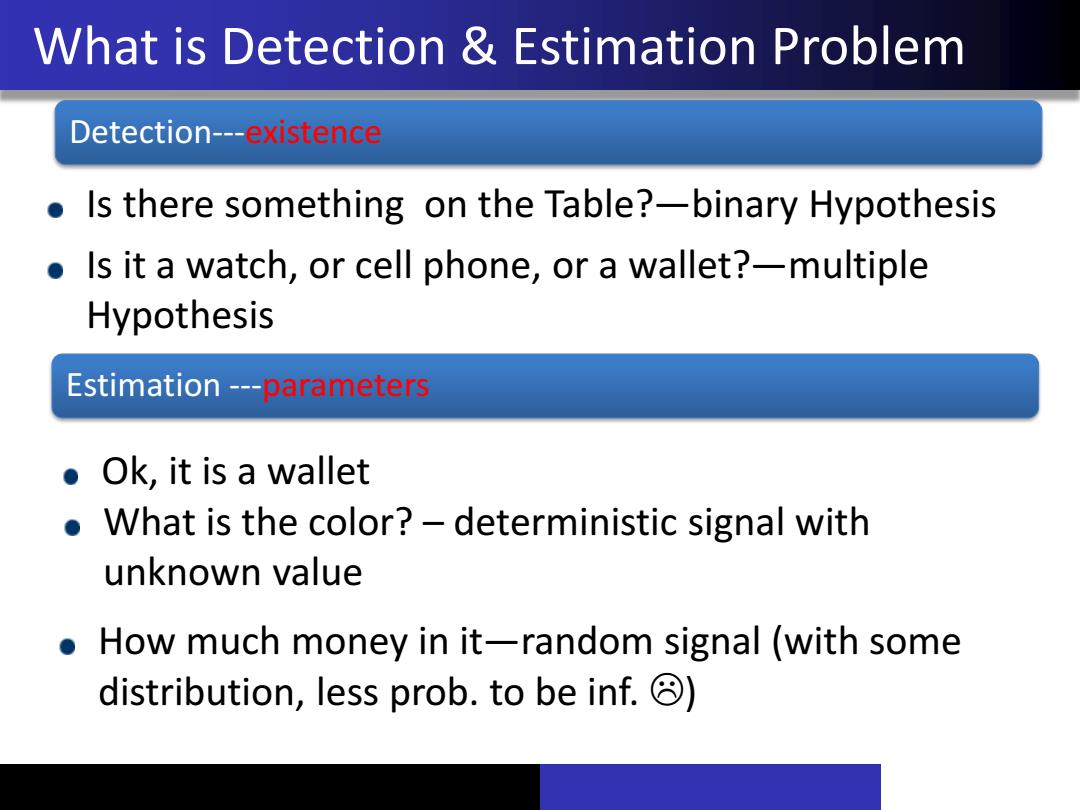
What is Detection Estimation Problem Detection---existence Is there something on the Table?-binary Hypothesis Is it a watch,or cell phone,or a wallet?-multiple Hypothesis Estimation ---parameters Ok,it is a wallet What is the color?-deterministic signal with unknown value How much money in it-random signal (with some distribution,less prob.to be inf
Is there something on the Table?—binary Hypothesis Is it a watch, or cell phone, or a wallet?—multiple Hypothesis What is Detection & Estimation Problem 7 Detection---existence Estimation ---parameters Ok, it is a wallet What is the color? – deterministic signal with unknown value How much money in it—random signal (with some distribution, less prob. to be inf. )
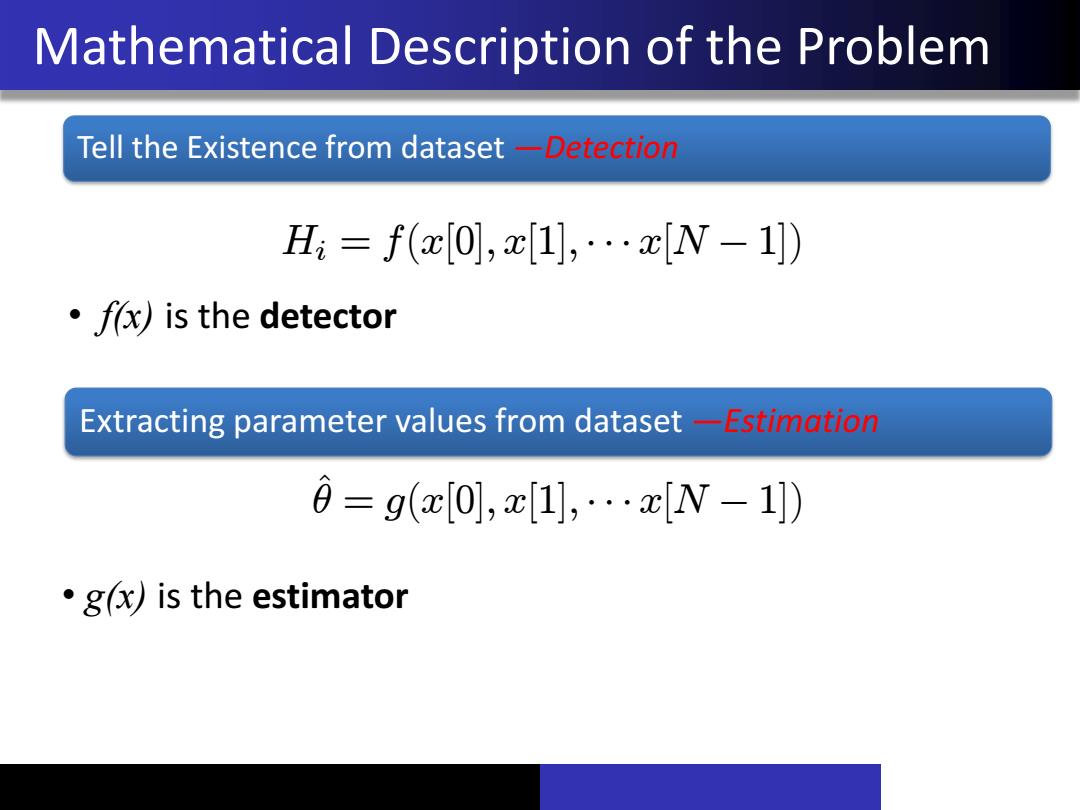
Mathematical Description of the Problem Tell the Existence from dataset-Detection H=f(x[0],c[1],…x[N-1]) ·fx)is the detector Extracting parameter values from dataset-Estimgtion 0=g(x[0],x[1],…x[N-1) .g(x)is the estimator
Mathematical Description of the Problem 8 Tell the Existence from dataset —Detection Hi = f(x[0];x[1]; ¢¢¢x[N ¡ 1]) • f(x) is the detector µ ^ = g(x[0]; x[1]; ¢¢¢x[N ¡ 1]) • g(x) is the estimator Extracting parameter values from dataset —Estimation

Applications ●Radar 。Communication ●Speech recognition ●Face Recognition 。Etc
Radar Communication Speech recognition Face Recognition Etc… Applications 9

Outline Introduction 。Admin.lnfo. What is the Detection Estimation Problem? Application of Detection Estimation Theory ●Warming Up-Review Vector Representation of Signal 。Stochastic Process 。Probability Theory
Introduction Admin. Info. What is the Detection & Estimation Problem? Application of Detection & Estimation Theory Warming Up---Review Vector Representation of Signal Stochastic Process Probability Theory Outline 10
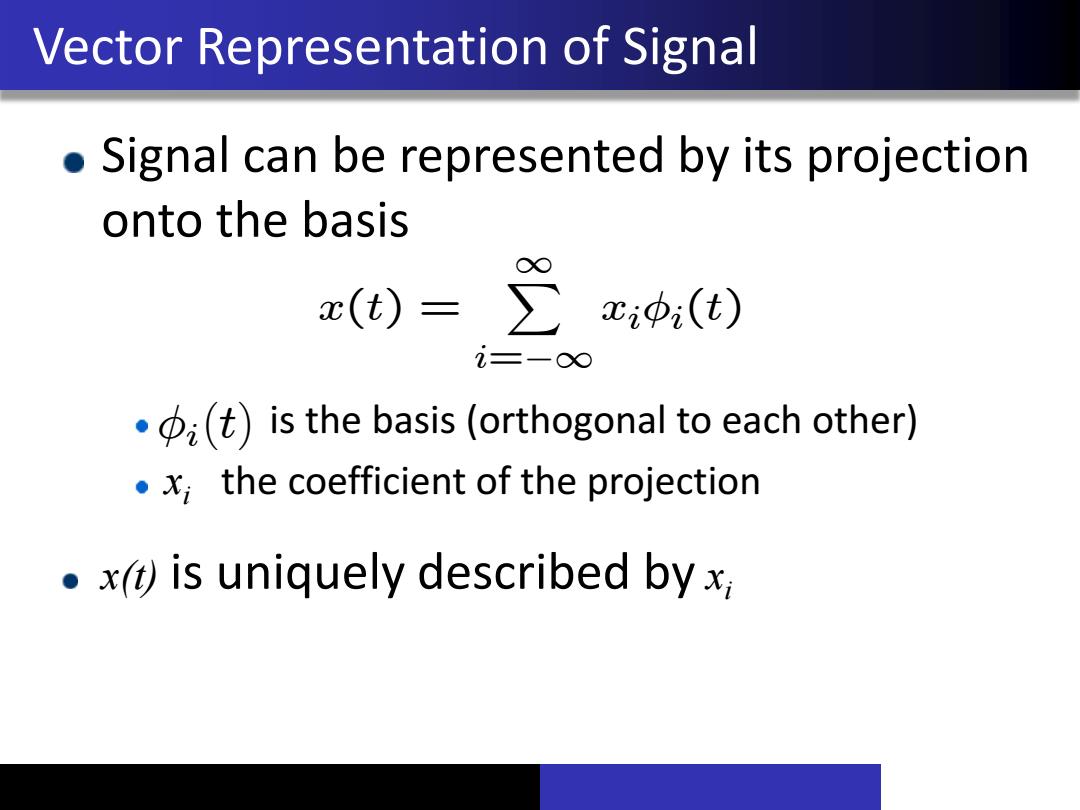
Vector Representation of Signal Signal can be represented by its projection onto the basis x(t)=∑r,(t) ·中,(t)is the basis(orthogonal to each other) .x the coefficient of the projection .x(is uniquely described by x
Vector Representation of Signal x(t) = X 1 i=¡1 xiÁi (t) 11 Signal can be represented by its projection onto the basis is the basis (orthogonal to each other) xi the coefficient of the projection Ái (t) x(t) is uniquely described by xi