Statistical Detection Theory Random Signal Wenhui Xiong NCL UESTC whxiong@uestc.edu.cn
whxiong@uestc.edu.cn Statistical Detection Theory Random Signal Wenhui Xiong NCL UESTC

Outline Signal Model Estimator-correlator whxiong@uestc.edu.cn 2
whxiong@uestc.edu.cn Signal Model Estimator-correlator Outline 2

System Model Binary Hypo.Test:Ho or H Ho xIn]wln] n=0,1..N-1 H cIn]sIn]+wln] whxiong@uestc.edu.cn 3
whxiong@uestc.edu.cn System Model 3 H0 Binary Hypo. Test: H0 or H1 H0 x[n] = w[n] H1 x[n] = s[n] + w[n] n=0,1…N-1

System Model Binary Hypo.Test:Ho or Hi Ho xIn]wln] n=0,1..N-1 H cln]=sIn]+wlnl 。Assumption: s[n]is WGN with zero mean and known variance .wIn]:awgn rww[k]=E(w[njwin+k)=026(k) whxiong@uestc.edu.cn 4
whxiong@uestc.edu.cn System Model 4 Assumption: s[n] is WGN with zero mean and known variance w[n]: awgn H0 Binary Hypo. Test: H0 or H1 H0 x[n] = w[n] H1 x[n] = s[n] + w[n] n=0,1…N-1 rww[k] = E(w[n]w[n + k]) = ¾ 2 ±(k)

System Model Binary Hypo.Test:Ho or Hi Ho xIn]wln] n=0,1..N-1 H cln]=sIn]+wlnl 。Assumption: s[n]is WGN with zero mean and known variance .wIn]:awgn rww[k]=E(w[njwin+k)=026(k) P(x;H1) L(x)=Px;Ho) whxiong@uestc.edu.cn 5
whxiong@uestc.edu.cn System Model 5 Assumption: s[n] is WGN with zero mean and known variance w[n]: awgn H0 Binary Hypo. Test: H0 or H1 H0 x[n] = w[n] H1 x[n] = s[n] + w[n] n=0,1…N-1 rww[k] = E(w[n]w[n + k]) = ¾ 2 ±(k) L(x) = P(x; H1) P(x; H0) > °

Estimater-correlator L(x)= 2 i!te p{o4可∑2oj} 2m2 ex{-∑2(mj月 whxiong@uestc.edu.cn 6
whxiong@uestc.edu.cn Estimater-correlator 6 L(x) = 1 [2¼(¾2 s +¾2)] N=2 exp n ¡ 1 2(¾2 s +¾2) Px 2 (n) o 1 (2¼¾2) N=2 exp © ¡ 1 2¾2 Px 2(n) ª

Estimater-correlator L(x)= 2so+o7rep{-2o∑2(mj} 2 X{-》2(nj月 L(x)= ()(+)∑w whxiong@uestc.edu.cn
whxiong@uestc.edu.cn Estimater-correlator L(x) = 1 [2¼(¾2 s +¾2)] N=2 exp n ¡ 1 2(¾2 s +¾2) Px 2 (n) o 1 (2¼¾2) N=2 exp © ¡ 1 2¾2 Px 2(n) ª L(x) = N 2 ln µ ¾2 ¾2 s + ¾2 ¶ ¡ 1 2 µ 1 ¾2 s + ¾2 ¡ 1 ¾2 ¶ Xx 2 (n)

Estimater-correlator L(x)= 2soo7rep{0o4o∑a20j} 2rexp{-》2(nj月 L(x)= ()(动)X The detection statistics T()=2(n) whxiong@uestc.edu.cn 8
whxiong@uestc.edu.cn Estimater-correlator 8 L(x) = 1 [2¼(¾2 s +¾2)] N=2 exp n ¡ 1 2(¾2 s +¾2) Px 2 (n) o 1 (2¼¾2) N=2 exp © ¡ 1 2¾2 Px 2(n) ª L(x) = N 2 ln µ ¾2 ¾2 s + ¾2 ¶ ¡ 1 2 µ 1 ¾2 s + ¾2 ¡ 1 ¾2 ¶ Xx 2 (n) The detection statistics T(x) = Xx 2 (n)

Estimater-correlator L(x)= 2 xp{0o4o∑a20j} 2wexp{-∑r2(mj月 L(x)= ()()∑ The detection statistics T()=2(n) We decide H]if T()=>22(n)>Y whxiong@uestc.edu.cn 9
whxiong@uestc.edu.cn Estimater-correlator 9 L(x) = 1 [2¼(¾2 s +¾2)] N=2 exp n ¡ 1 2(¾2 s +¾2) Px 2 (n) o 1 (2¼¾2) N=2 exp © ¡ 1 2¾2 Px 2(n) ª L(x) = N 2 ln µ ¾2 ¾2 s + ¾2 ¶ ¡ 1 2 µ 1 ¾2 s + ¾2 ¡ 1 ¾2 ¶ Xx 2 (n) The detection statistics T(x) = Xx 2 (n) We decide H1 if T(x) = Xx 2 (n) > ° 0
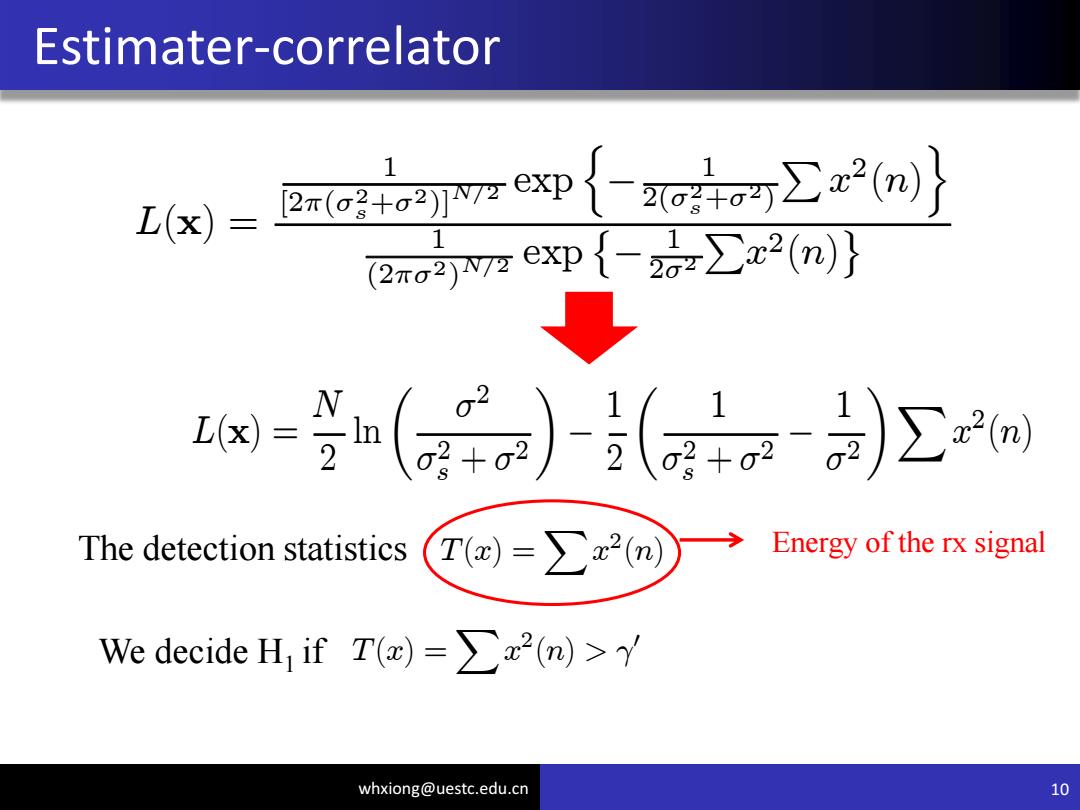
Estimater-correlator L(x)= 2 xp{zo4可∑r2(mj} 2 ara exp{-2o∑2(0m刃 L(x)= ()∑测 The detection statistics T(=∑a2(n→ Energy of the rx signal We decide H if T()=>22(n)>Y whxiong@uestc.edu.cn 10
whxiong@uestc.edu.cn Estimater-correlator 10 L(x) = 1 [2¼(¾2 s +¾2)] N=2 exp n ¡ 1 2(¾2 s +¾2) Px 2 (n) o 1 (2¼¾2) N=2 exp © ¡ 1 2¾2 Px 2(n) ª L(x) = N 2 ln µ ¾2 ¾2 s + ¾2 ¶ ¡ 1 2 µ 1 ¾2 s + ¾2 ¡ 1 ¾2 ¶ Xx 2 (n) The detection statistics T(x) = Xx 2 (n) We decide H1 if T(x) = Xx 2 (n) > ° 0 Energy of the rx signal