
ENGINEERING SOCIETIES MONOGRAPHS THEORY OF Bakhmetef:Hydrauiics of Open Channeis Bleich:Buckling Strength of Metal Structures ELASTIC STABILITY Crandall:Engineering Analysis Elevatorski:Hydraulic Energy Dissipators Leontovich:Frames and Arches Nadai:Theory of Flow and Fracture of Solids Timoshenko and Gere:Theory of Elastic Stability Timoshenko and Goodier:Theory of Elasticity Timoshenko and Woinowsky-Krieger:Theory of Plates and Shells STEPHEN P.TIMOSHENKO Professor Emeritus of Engineering Mechanica American In urgical,and P tro Stanford Uniseraity snd the American Institute of Chemieal Engineers,have an arrangement with the McGraw-Hill Book Company,Ine.,for the production of a serics of selected books usefulness for engineers and industry. of thia are:to provide monographa of high technical IN COLLABORATION WITH manuricmisht not be publiahed sommercially because of too limited aale of engineeri ortant technical JAMES M.GERE without pecialirodtio to developmnrptolgaps nexi sting literature; to collect into one volume scattered informstion of especial timeliness on a given Associate Professor of Civil Engineering ocieties sasume no responsibility for any statementa made in these books. Stanford Unipersily xamined by one or more representa- tives of the societies competent to expressan opinion on the merita of the manuaeript. Ralph H.Phelps,CHAIRMAN Engineering Societies Library New York SECOND EDITION ENGINEERING SOCIETIES MONOGRAPHS COMMITTEE INTERNATIONAL STUDENT EDITION A.8.C.E. 且oward T.Critchlow H.Alden Foster EERING A.I.M.E. Nathanicl Arbiter John F.Elliott A.8.M.E. Calvin 8.Cronan Raymond D.Mindlin A.I.E.E. F.Maloolm Farmer Royal W.Sorensen MCGRAW-HILL INTERNATIONAL BOOK COMPANY A.I.Ch.E. Auckland Bogota Guatemala Hamburg Johannesburg Lisbon Joseph F.Skelly London Madrid Mexico New Delhi Panama Paris San Juan Charles E.Reed Sao Paulo Singapore Sydney Tokyo

PREFACE TO THE BECOND EDITION Numerous footnote references are given throughout the text as an aid to the student who wishes to pursue some aspect of the subject further. The authors take this opportunity to thank Mrs.Thor H.Sjostrand and Mrs.Richard E.Platt for assistance in preparing the manuscript and reading the proofs for this second edition. Stephen P.Timoshenko James M.Gere PREFACE TO THE FIRST EDITION The modern use of steel and high-strength alloys in engineering struc- tures,especinlly in bridges,ships,and aireraft,has made elastic insta- bility a problem of great importance.Urgent practical requirements have given rise in recent years to extensive investigations,both theo- retical and experimental,of the conditions governing the stability of such structural elements as bars,plates,and shells.It seems that the time has come when this work,recorded in various places and languages, often difficult of access to engineers who need it for guidance in design, should be brought together and put in the form of a book. The first problems of elastic instability,concerning lateral buckling of compressed members,were solved about 200 years ago by L.Euler.1 At that time the principal structural materials were wood and stone. The relatively low strength of these materials necessitated stout struc- tural members for which the question of elastic stability is not of primary importance.Thus Euler's theoretical solution,developed for slender bars,remained for a long time without practical application.Only with the beginning of extensive construction of steel railway bridges during the latter half of the past century did the question of buekling of com- pression members become of practical importance.The use of.steel led naturally to types of structures embodying slender compression mem- bers,thin plates,and thin shells. Experience showed that such struc- tures may fail in some cases not on account of high stresses,surpassing the strength of material,but owing to insufficient elastic stability of slender or thin-walled members. Under pressure of practical requirements,the problem of lateral buckling of columns,originated by Euler,has been extensively investi- gated theoretically and experimentally and the limits within which the theoretical formulas can be applied have been established.However, lateral buckling of compressed members is only a particular case of elaatie instability.In the modern design of bridges,ships,and aircraft we are confronted by a variety of stability problems.We encounter there not only solid struts,but built-up or "lattice-work"columns,and 1Loonard Euler's"Elnstie Curves,"tranalated and annotated by W.A.Oldfather, C.A.Ellis,and D.M.Brown,1933. vii

vi道 PREFACE TO THE FIRST EDITION PREPACE TO THE FIRST EDITION ix tubular members,where there is the possibility of local buckling,as well as buckling as a whole.In the use of thin sheet material,as in plate To the University of Michigan the author is grateful for financial girders and airplane structures,we have to keep in mind that thin plates support obtained from a research fund and used in the preparation of may prove unstable under the action of forces in their own planes,and numerical tables and diagrams for this book.He also takes this oppor- fail by buckling sideways.Thin cylindrical shells,such as vacuum tunity to extend thanks to Dr.D.H.Young who read over the complete vessels,which have to withstand uniform external pressure,may exhibit manuscript and made many valuable suggestions and corrections,to instability and collapse at a relatively low stress if the thickness of the Professors G.H.MacCullough and H.R.Lloyd who read some portions shell is too small in comparison with the diameter.The thin cylindrical of the manuscript,to Dr.I.A.Wojtaszak and Mr.S.H.Fillion for the shell may buckle also under axial compression,bending,torsion,or com- checking of equations and numerical tables,to Dr.Wojtaszak for reading binations of these.All such problems are of the utmost importance proofs,to Miss Reta Morden for the typing of the manuscript,and to in the design of airplanes of the modern monocoque type. Mr.L.S.Veenstra for the preparation of the drawings. S.Timoshenko In the discussion of these problems and their solutions,it has not been deemed necessary to include an account of the general theory of elastic stability,which finds its appropriate place in books on the theory of elasticity.This book proceeds directly to particular problems showing in each case under what conditions the question of stability calls for consideration.The various methoda of solution are presented in con- neetion with the types of problem to which they are best suited.The solutions have in most cases been supplemented by tables and diagrams which furnish values of critical loads and stresses for each particular case. While all available information relevant to a prescribed problem has been given,no attempt has been made to go beyond this into actual design,since it is a field in which other considerations besides rational theory and testing play their parts. The preliminary knowledge of mathematics and strength of materials taken for granted is that usually covered by our schools of engineering. Where additional mathematical equipment has been found necessary, it is given in the book with the appropriate explanations.To simplify the reading of the book,problems which,although of practical import- ance,are such that they can be omitted during a first reading are put in small type.The reader may return to the study of such problems after finishing the more essential portions of the book. Numerous references to papers and books treating stability problems are given in the book.These references may be of interest to engineers who wish to study some special problems in more detail.They give also a picture of the modern development of the theory of elastie sta- bility and may be of some use to graduate students who are planning to take their work in this field. In the preparation of this book the contents of a previous book dealing with stability problems and representing a course of lectures on the theory of thin plates and shells,as given in several Russian engi- neering schools,have been freely drawn upon. 1"Theory of Elasticity,"vol.II,St.Petersburg,Rusis,1916

CONTENTS Preface to the Second Edition. Preface to the First Edition Netations.. Chapter 1.Beam-columns..... 1 1.1 Introduction 12 Differentinl Equstions for Beam-oolumns 4 1 1.3 Beam-column with Concentrated Lateral Load 1 1.4 Several Coneentrated Loads 3 1.5 Continuous Lateral Losd. 4 79 1.6 Bending of s Beam-column by Couples 1.7 Approximate Formula for DeBections. 、 2 1.8 Beam-columns with Built-in Ends. 4 4 1.9 Beam-columns with Elastie Reatrainta 15 1.10 Continuous Beams with Axial Loads 1.11 Application of Trigonometrie Beries 1.12 The Effect of Initial Curvature on Deflections 4 ” 1.13 Determination of Allowable Stresses .. 3 Chapter 2.Elastic Buckling of Bars and Frames 6 2.1 Euler's Column Formuls 2.2 Alternate Form of the Differential Equntion for Determining Critical 6 Loads. 51 The Use of Beam-column Theory in Caleulating Critieal Loads 2.4 Buekling of Frames. 2.5 Buckling of Continuoun Beams 2.6 2.7 Buckling of Continuous Beams on Elastie Supporta 70 2.8 Large Defleetions of Buckled Bara(the Elnstics). The Energy Method. 2,9 Approximate Caleulntion of Critical Loads by the Energy Metbod 2.10 Buekling of a Bar on an Elastie Foundstion.. 2.11 Buckling of a Bar with Intermediate Compressive Forees. 86 2.12 Buckling of a Bar under Distributed Axinl Loads. 2.13 Buekling ofa Bar on an Elastic Foundation under Distributed Axial 100 2.14 Buekling of Bars with Changes in Croes Section 107 2.15 The Determination of Critical Loads by Buceesive Approximations. 113 2.16 Bars with Continuously Varying Crons Section. 116 2.17 The Effeet of Sbearing Force on the Critical Load. 132

CONTENTS 出 2.18 Buckling of Built-up Columns. 4 4 135 7.0 Buckling of Bimetallie Strip 310 2.19 Buckling of Helical Springs 4 7.10 Lateral Buckling of a Curved Bar with Cireular Axis 4 。4 313 2.20 Stability of n System of Bar8 2.21 The Case of Nonconservative Forees. Chapter 8.Bending of Thin Plates... 319 2.22 Stability of Priamatic Bars under Varying Axial Foreea 4 8.1 Pure Bending of Plates 4 319 82 Bending of Plates by Distributed Lateral Load. 163 326 Chapter 3.Inelastic Buckling of Bars 8.3 Combined Bending and Tension or Compression of Plates. 332 3.1 Inelastic Bending 163 8.4 Strain Energy in Bending of Plates 335 3.2 Inelastic Bending Combined with Axial Lond 8.5 Deflections of Rectangular Plntes with Simply Supported Edges. 340 3.3 Inelastic Buekling of Bars (Fundamental Case). g 8.6 Bending of Plates with a Small Initinl Curvature.. 844 3.4 Inelastie Buekling of Bars with Other End Conditions 182 8.7 Large Deflections of Plates. 346 Chapter 4.Experiments and Design Formulas 185 Chapter 9. Buckling of Thi扣ates, 348 4.I Column Tests 185 91 Methods of Caleulation of Critical Loads 348 4.2 Ideal-column Formulas as a Baais of Column Design 92 Buckling of Simply Bupported Rectangular Plates Uniformly Compreed 4.3 Empirical Formulas for Column Deaign. 路 in One Direction. 351 4.4 Ansumed Insecuracies as a Basis of Column Deaign 197 93 Buckling of Simply Supported Rectangular Plates Compreased in Two 4.5 Various End Conditions 202 Perpendieular Directions 4 a56 4.6 The Desigh of Built-up Columns. 206 9.4 Buckling of Uniformly Compreesed Reetangular Plates Simply Supported 212 along Two Opposite Sides Perpendicular to the Direetion of Compreasion Chapter 5.Torsional Buckling. and Having Various Edge Conditions along the Other Two Sides 4 360 5.1 Introduetion.. 212 9.5 Buekling of a Rectangular Plate Simply Supported along Two Opposite 52 Pure Torsion of Thin-walled Bars of Open Cross Section. 、 212 Sides and Uniformly Compressed in the Direction Parallel to Those Sides. 370 5.3 Nonuniform Torion of Thin-walled Bars of Open Croms Seetion.. 218 9.6 Buckling of a Simply Supported Rectangular Pinte under Combined 5.4 Torsional Buekling. 225 Bending and Comprension. 373 5.5 Buckling by Torsion and Flexure. 229 9,7 Buckling of Rectangular Plates under the Action of Shearing Btreascs 379 5.6 Combined Torsional and Flexural Buckling of a Bar with Continuous 9.8 Other Cnses of Buckling of Rectangular Plates 385 Elastic8 upporte,· 237 9.9 Buckling of Circular Platee. 380 5.7 Torsional Buckling under Thrust and End Moments 244 9.10 Buckling of Plates of Other Shapes 392 9.11 Stability of Plates Reinforeed by Ribs 39H Chapter 6. Lateral Buckling of Beams 4 251 9.12 Buckling of Plstes beyond Proportional Limit 408 6.1 Differential Equations for Lateral Buckling 251 9.13 Large Deflections of Buckled Plates 41 6.2 Lateral Buckling of Beams in Pure Bending 4 253 9.14 Ultimate Strength of Buckled Plates 418 6.3 Lateral Buckling of a Cantilever Beam 257 9.15 Experiments on Buckling of Platee 42 6.4 Lateral-Buckling of Simply Supported I Beams 262 9.16 Practical Applications of the Theory of Buckling of Piatee 29 6.5 Lateral Bucklins of Simply Supported Beam of Narrow Rectangular Chapter 10. Bending of Thin Shells.. 440 Cross Section. 268 6.8 Other Cases of Lateral Buekling. 270 10.1 Deformation of an Element of a Shell 440 6.7 Inelastie Lateral Buckling of I Beams 272 10.2 Symmetrical Deformation of a Cireular Cylindricnl Shell 10.3 Inextensional Deformation of Cireular Cylindrical Shell Chapter 7. Buckling of Rings,Curved Bars,and Arches. 278 445 10.4 General Case of Deformation of a Cylindrical Shell. 4M8 7.1 Bending of a Thin Curved Bar with a Circular Axis 278 10.5 Symmetrical Deformation of a Bpberical Shell 4 453 72 Application of Trigonometrie Series in the Anslysis of a Thin Circular Chapter 11.Buckling of Shells 457 ing· 44 ” 282 73 Effect of Uniform Preasure on Bending of a Cireulnr Ring.. 287 111 Symmetrical Buekling of a Cylindrienl Shell under the Action of Uniform 7.4 Buckling of Cireular Rings and Tubes under Uniform External Proasure 289 Axial Compression 457 7.5 The Design of Tubee under Uniform External Pressure on the Basis of 11.2 Inextensional Forms of Bending of Cylindrical Shells Due to Instability 461 A题mmed Inaecuracies + 294 11.3 Buekling of a Cylindrical Shell under the Action of Uniform Axial Pressure 462 7.6 Buckling of a Uniformly Compressed Cireular Arch ▣44 11.4 Experimenta with Cylindrical Shells in Axial Compreasion 488 7,7 Arebes of Other Forms. 11.5 Buckling of a Cylindrical Shell under the Action of Uniform Externsl 7.8 Buekling of Very Flnt Curved Bars .... 。4 305 Lateral Preeeure. 474

xiv CONTENTS 11.6 Bent or Eooentrically Compressed Cylindrical Shells. 482 11:7 Axial Compression of Curved Sheet Panels 485 11.8 Curved Sheet Panels under Shear or Combined Shear and Axial Stree 11.9 Buckling ofStitfened Cylindricnl Shell under Axial Compreesion.. 48 11.10 Buekling of a Cylindrical Shell under Combined Axial and Uniform Lateral Pressure . 495 11.11 Buckling of a Cylindrical Shell Bubjeeted to Torsion.. 500 11.12 Buckling of Conical Shells . 509 11.13 Buckling of Uniformly Compreed Spherical Sbeils 612 NOTATIONS pnh,,。。。,,+。··,。····”· 521 Table A-1.Table of the Funetions),),x(). 4 521 a,b,c,d Numerical coefficienta,distances Table A-2.Table of the Functiona()and () 629 A Cross-nectional ares Table A-3.Properties of8 ections,,,·· 530 Distance from neutral axis to extreme fiber of beam 631 C Toreional rigidity (C-GJ) Name Index.‘.,',,-. C Warping rigidity (C BC.) Subject Index 4 535 C. Warping constant D Flexural rigidity of plate or shell [D Eh/12 (1-) Eocentricity,distance from centroid to shear center B.E,E Modulus of elaaticity,roduced modulus,tangent modulus Streas function Acceleration of gravity Modulus of clasticity in shear Thickness of plate or shell,height,distance Polar moments of inertia of a plane ares with respect to centroid and ahear center I Iy I Moments of inertis ofa plane area with respect to ,and axe Produet of inertia of a plane ares with respeet to a and y axes Torsion constant Axial losd faetor for beam-columns (k-P/BI),modulus of clastic foundation,numerieal faetor 【c知gth,8pBD L Redueed length ,作 Integers,numerical factors Intenity of torque per unit distance alongaxis Bending moment,couple M. Twisting couple or torque M.My Mev Bending and twisting momenta per unit distance in plate or shell Faetor of safety Shearing force in beam,normal force N.Nu N. Normal snd shearing forces per unit distance in middle surface of plate or shell 月9 Intenaity of distributed load,preasure P Concentrated foree,axial foree in beam-column Critical buckling load Q Shearing force in beam,conoentrated foree 0Q Sbearing forees per unit distance in plate or shell Radius of gyration,radius of curvature of shell,radius R Radins,reactive foree 8 Core radius (s -2/A),distanee 8 Axial force W

xvi NOTATIONB Thiekness,time,temperature Work,tenaile foree Axisl load factor for beam-columns (u/2) ,, Displacementa in多,,and z directions U Btrain energy 可,0 Displacements in tangential and radial directions CHAPTER 1 y Shearing foree in beam Warping displacement in beam BEAM-COLUMNS ,多 Rectangular coordinates Section modulus (2=I/e) 1.1.Introduction.In the elementary theory of bending,it is found that stresses and deflections in beams are directly proportional to the restraint applied loads.This condition requires that the change in shape of the y Shearing unit strain,weight per unit volume,spring conatant, beam due to bending must not affect the action of the applied loads. numerical factor For example,if the beam in Fig.1-la is subjected to only lateral loads, 8 Defection Unit normal strain,coefficient of thermal expansion such as Q amd Qa,the presence of the small deflections 61 and and slight , Unit normal strains in z,y,and s directionn changes in the vertical lines of action of the loads will have only an insig- Amplifcation factors for beam-columns nificant effect on the moments and shear forces.Thus it is possible to Angle,angulnr coordinate,angle of twist per unit length make calculations for deflections,stresses,moments,ete.,on the basis of Distance,numerical factor Poisson's ratio the initial configuration of the beam.Under these conditions,and also 名男下 Reetangular coordinates if Hooke's law holds for the material,the deflections are proportional to Radius of curvature the acting forces and the principle of superposition is valid;Le.,the final Unit normal streas deformation is obtained by summation of the deformations produced by Ua dn ds Unit normal stresses in z,y,and directions the individual forces. 4 Average compresaive unit atreas for columns Compreesive unit streas at critical load Conditions are entirely different when both axial and lateral loads act olt Unit streas at ultimate load simultaneously on the beam (Fig.1-16).The bending moments,shear Working unit streas forces,stresses,and deflections in the beam will not be proportional to Yield-point streas the magnitude of the axial load.Furthermore,their values will be Unit shear stres dependent upon the magnitude of the deflections produced and will be Te,T南Tu Unit shear stresses on planes perpendicular to the馬,斯,and&axes and sensitive to even alight eccentricities in the application of the axial load. parallel to the y,美,8od¥axw Angle,angular coordinate,angle of twist of bar Beams subjected to axial compression and simultaneously supporting X Change of curvature in shell lateral loads are known as beam-columns.In this first chapter,beam- Radian froquency of vibration columns of symmetrical cross section and with various conditions of Warping function support and loading will be analyzed. 1.2.Differential Equations for Beam-columns.The basic equations for the analyais of beam-columns can be derived by considering the beam in Fig.1-2a.The beam is subjected to an axial compressive force P and to a distributed lateral load of intensity g which varies with the dis- tance z along the beam.An element of length dz between two cross sections taken normal to the original (undeflected)axis of the beam is 1 For an analysis of beama subjected to axial tension see Timoshenko,"Strength of Materials,"3d od.,part II,p.41,D.Van Nostrand Company,Ine.,Princeton,N.J. 1966
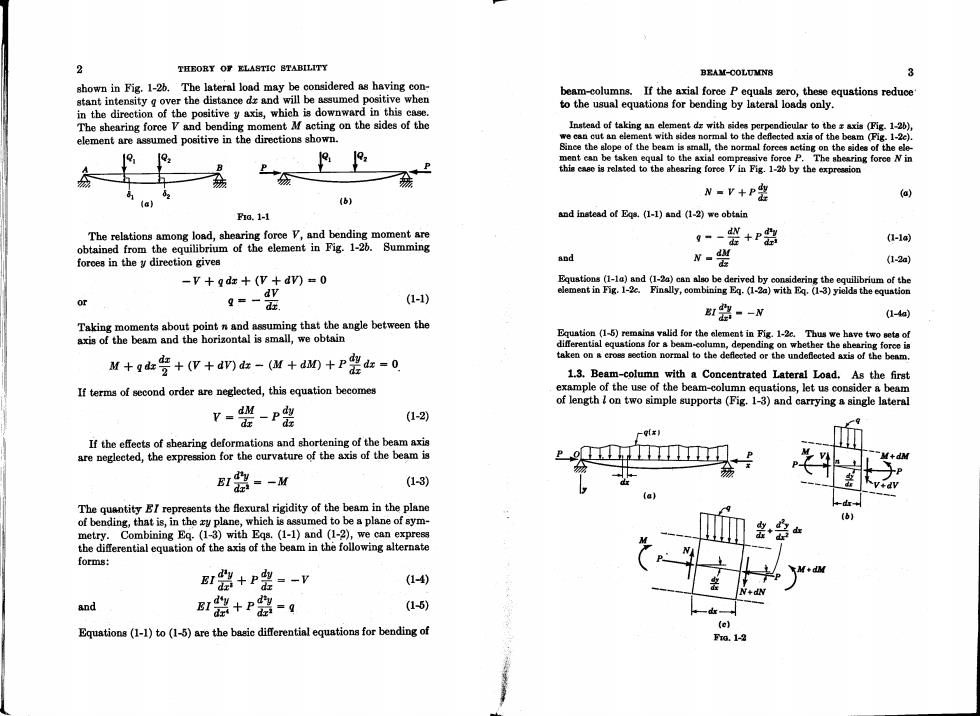
% THEORY OF ELASTIC STABILITY BEAM-COLUMNS shown in Fig.1-2b.The lateral load may be considered as having con- stant intensity g over the distance da and will be assumed positive when beam-columns.If the axial force P equals zero,these equstions reduce in the direction of the positive y axis,which is downward in this case. to the usual equations for bending by lateral loads only. The shearing force V and bending moment Mf acting on the sides of the Instead of taking an element d=with sides perpendicular to the axis (Fig.1-2), element are assumed positive in the directions shown. we can cut an element with sides normal to the deflected axis of the beam (Fig.1-2c). Since the slope of the beam is small,the normal forees neting on the sides of the ele- 12, ment can be taken equal to the axial compressive foree P.The shearing force N in this cae is relsted to the ahearing force V in Fig.1-26 by the expresaion (b) N-r+P器 @) (a) hg.1-1 nd instead of Egs.(1-1)and (1-2)we obtain The relations among load,shearing foroe V,and bending moment are (-1a obtained from the equilibrium of the element in Fig.1-20.Summing g-器+P器 forces in the y direction gives and N- (1-2a) -v+qdz+(V+dv)=0 Equstions(1-1a)and(1-2)can also be derived by considering the equilibrium of the element in Fig.1-2c.Finally,combining Eq.(1-2a)with Eq.(1-3)yields the equstion or 9=- dt. (1-1) 数尝-N (1-4aj Taking moments about point n and assuming that the angle between the axis of the beam and the horizontal is small,we obtain Equstion (1-5)remains valid for the element in Fig.1-2c.Thus we have two seta of differential equations for s beam-column,depending on whether the shearing force is M+ga警+(v+am-(M+d0+P费-0 taken on s eross section normal to the deflected or the undeflected axis of the beam. 1.3.Beam-column with a Concentrated Lateral Load.As the first If terms of second order are neglected,this equation becomes example of the use of the beam-column equations,let us consider a beam of length I on two simple supports(Fig.1-3)and carrying a single lateral v-器-P器 (1-2) If the effects of shearing deformations and shortening of the beam axis are neglected,the expression for the curvature of the axis of the beam is 1碧=-M (1-3) The quantity BI represents the fexural rigidity of the beam in the plane of bending,that is,in the ry plane,which is assumed to be a plane of sym- metry.Combining Eq.(1-3)with Eqs.(1-1)and (1-2),we can express the differential equation of the axis of the beam in the following alternate forms: B路+P鼎=-V (1-4) and BI器+P器-g (1-5) (e) Equations(1-1)to (1-5)are the basie differential equations for bending of Fro.1-2

THEORY OF ELASTIC BTABILITY BEAM-COLUMNB 5 load Q at distance c from the right end.The bending moments lue to the mon tangent.These conditions give lateral load Q,if acting alone,could be found readily by statics.How- ever,in this case the axial force P causes bending moments which can- B血机-0-%a-g not be found until the deflections are determined.The beam-column =Dsn机-)-tan-l-号Q-0 is therefore statically indetermi- nate,and it is necessary to begin by B张cosk机-)- solving the differential equation for Fg.1-3 the deflection curve of the beam. -DHtcos k(-)tan kl sin k()) P The bending momenta in the left-and right-hand portions of the beam from which Q sin ke in Fig.1-3 are,respectively, B=Pkim对 D=-Qink0-c) Pk tan ) M-号:+mM-90-9Q-9+Pm Substituting into Egs.(c)and (d)the values of the constants from (e) and,therefore,using Eq.(1-3)we obtain and (f),we obtain the following equations for the two portions of the deflection curve: B1---Pm (a) 1路--0-0-丑-Pm y=领血u-资:0sz≤1-0 (1-7) () y-Q血地-d血h机--0-e0-91-6≤x≤1 Pk sinl For simplification the following notation is introduced: (1-8) P k= (1-6) It is seen that Eq.(1-8)can be obtained from Eq.(1-7)by substituting -c for c and -x for z. and then Eq.(a)becomes By differentiation of Eqs.(1-7)and (1-8)the following formulas,useful 器+灼一品: Qc in later calculations,are obtained: dy Q sin ke The general solution of this equation is dz 品经mu-器 = 0≤x≤1-c(1-9) y=Ac在十B面好一气: (c) =-Qk:9osk0-)+g001-c≤z≤1 dz P sin kl (1-10) In the same manner the general solution of Eq.(6)is dy i=- 华血a 0≤x≤1-e(1-11) y=Coos缸十D gin kx- Q0-c0- Pl (④ dy dz Qk sink(-)sin k(-到 P ainl 1-c≤x≤1(1-12) The constants of integration A,B,C,and D are now determined from the conditions at the ends of the beam and at the point of applieation of In the particular case of a load applied at the center of the beam,the the load Q.Since the deflections at the ends of the bar are zero,we deflection curve is symmetrical and it is necessary to consider only the conclude that portion to the left of the load.The maximum deflection in this case is A =0 C=-D tan kl (e) obtained by subatituting x=c=1/2 in Eq.(1-7),which gives At the point of applieation of the load Q the two portions of the defleetion curve,as given by Eqs.(c)and (d),have the same deflection and a com- )

BEAM-COLUMNS 6 THEORY OF ELASTIC BTABILITY 7 To simplify this equation the following additional notation will be used: Again,the first factor is the slope produced by the lateral lond Q acting alone at the center of the beam and the second factor represents the effect 以1P (1-13) of the axial load P.Values of the factor A(u)are given in Table A-2 “=2=2VE7 of the Appendix. Then Eq.(g)becomes By using Eq.(1-11)we obtain the maximum bending moment as follows: 8贺7--%7x侧 &=481 (1-140 M--EI dy八 k以QI tan u dr* 2Ptan2-4 (1-18) The first factor on the right-hand side of this equation represents the deflection which is obtained if the lateral load Q acts alone.The second The maximum bending moment is obtained in this case by multiplying factor,x(u),gives the influence of the longitudinal force P on the deflec- the bending moment produced by the lateral load by the factor (tan u)/u. tion 8.Numerical values of the factor x(u)for various values of the The value of this factor,as well as the previous trigonometric factors quantity tare given in Table A-1 in the Appendix.By using this table, A()and x(u),approaches unity as the compressive force becomes the deflections of the bar can be calculated readily in each particular case smaller and smaller and increases indefinitely when the quantity u from Eq.(1-14). approaches r/2,that is,when the compressive foroe approaches the When P is small,the quantity u is also small [see Eq.(1-13)]and the critical value given by Eq.(1-15). factor x(u)approaches unity.This can be shown by using the series 1.4.Several Concentrated Loads.The results of the previous article will now be used in the more general case of several lateral loads acting on 如=+号+答+… the compressed beam.Equations (1-7)and (1-8)show that for a given longitudinal force the deflections of the bar are proportional to the and retaining only the first two terms of this series.It is seen alao that lateral load Q.At the same time the relation between defections and x(u)becomes infinite when u approaches x/2.When u=x/2,we find the longitudinal force P is more complicated,sinee this force enters into from Eq.(1-13) the trigonometric functions containing k.The fact that deflections are p linear functions of Q indicates that the principle of superposition,which (1-15) is widely used when lateral loads act alone on a beam,can also be applied in the case of the combined action of lateral and axial loads,but in a some- Thus it can be concluded that when the axial compressive force what modified form.It is seen from Eqs.(1-7)and (1-8)that,if we approaches the limiting value given by Eq.(1-15),even the smallest increase the lateral load Q by an amount Q,the resultant deflection is lateral load will produce considerable lateral deflection.This limiting obtained by superposing on the deflections produced by the load Q the value of the compressive force is called the critical load and is denoted deflections produced by the load Q,provided the same axial force acta by P By using Eq.(1-15)for the critical value of the longitudinal on the bar. force,the quantity u[see Eq.(1-13)]can be represented in the following It can be shown that the method of superposition can be used also if form: several lateral loads are acting on the compressed bar.The resultant (1-16) deflection is obtained by using Eqs.(1-7)and (1-8)and superposing the separate deflections produced by each lateral load aeting in combination with the total axial force.Take the case of two lateral loads Q1 Thus u depends only on the magnitude of the ratio P/P To find the slope of the deflection curve at the end of the beam,we and Q at the distances c and cs from the right support (Fig.1-4). Proceeding as in the previous article,we find that the differential equation substitute c =1/2 and z=0 into Eq.(1-9),which gives of the deflection curve for the left portion of the beam(sI-ca)is Q1 r路=-9z-9a-Py (a) Q2(1-c0s4) Q =16E7w2co8“ =16E7A (1-170 Now consider the loads Q,and Q:acting separately on the compressed