同济大学：《结构力学》课程教学资源（教案讲稿，打印版）16 Dynamic analysis of structures

2016/4/6 Chapter 16 Dynamic analysis of structures @问济大学 目土本2程季悦 Why Dynamics? Statics vs.Dynamics F=ma @同冷大学 目士本红程学悦 1

2016/4/6 1 1 Chapter 16 Dynamic analysis of structures Why Dynamics? F  ma Statics vs. Dynamics

2016/4/6 Contents 1 Introduction 2 Undamped free vibration(无阻尼自由振动)of one-degree systems 3 Undamped forced vibration(无阻尼强迫振动)of one-degree systems 4 Damped vibration(阻尼振动)of one degree systems 5 Free vibration(自由振动)of multi-.degree systems 6 Orthogonality(正交性)of normal modes 7 Forced vibration(受迫振动)of multi--degree systems 8 Free vibration of undamped(无阻尼)systems with distribution mass 9 Approximate procedures for determining circular frequency @问冷大学目土本2程季乾 16.1 Introduction ·Dynamic load(动荷载) Dynamic degree of freedom ·D'Alembert's principle(达朗贝尔原理 ·Dynamic equilibrium ·Equation of motion @同冷大学 目士本工相幸悦 2

2016/4/6 2 3 Undamped forced vibration（无阻尼强迫振动）of one-degree systems 1 Introduction 2 Undamped free vibration（无阻尼自由振动）of one-degree systems 4 Damped vibration（阻尼振动）of one degree systems 5 Free vibration（自由振动）of multi-degree systems 6 Orthogonality（正交性）of normal modes 7 Forced vibration（受迫振动）of multi-degree systems 8 Free vibration of undamped（无阻尼）systems with distribution mass 9 Approximate procedures for determining circular frequency Contents 16.1 Introduction • Dynamic load（动荷载） • Dynamic degree of freedom • D’Alembert’s principle（达朗贝尔原理 ） • Dynamic equilibrium • Equation of motion

2016/4/6 Dynamic load Periodic Unbalanced rotating machine (周期性) p(t) 2T。 3订。 Nonperiodic Bomb blast pressure-impulsive ( (非周期性) p(t) Earthquake-long duration 同桥大学 Dynamic degrees of freedom Number of dynamic DOF -the number of displacement components which must be considered in order to represent the effects of all significant inertial forces(惯性力)ofa structure m 1 DOF 2DOFs Infinite DOFs @同冷大学 3

2016/4/6 3 Dynamic load Periodic （周期性） Nonperiodic （非周期性） Unbalanced rotating machine Bomb blast pressure - impulsive（脉冲） Earthquake – long duration p t p(t) t o t po To To 2 To 3 p(t) t 1 DOF 2 DOFs m Infinite DOFs Dynamic degrees of freedom Number of dynamic DOF – the number of displacement components which must be considered in order to represent the effects of all significant inertial forces（惯性力）of a structure

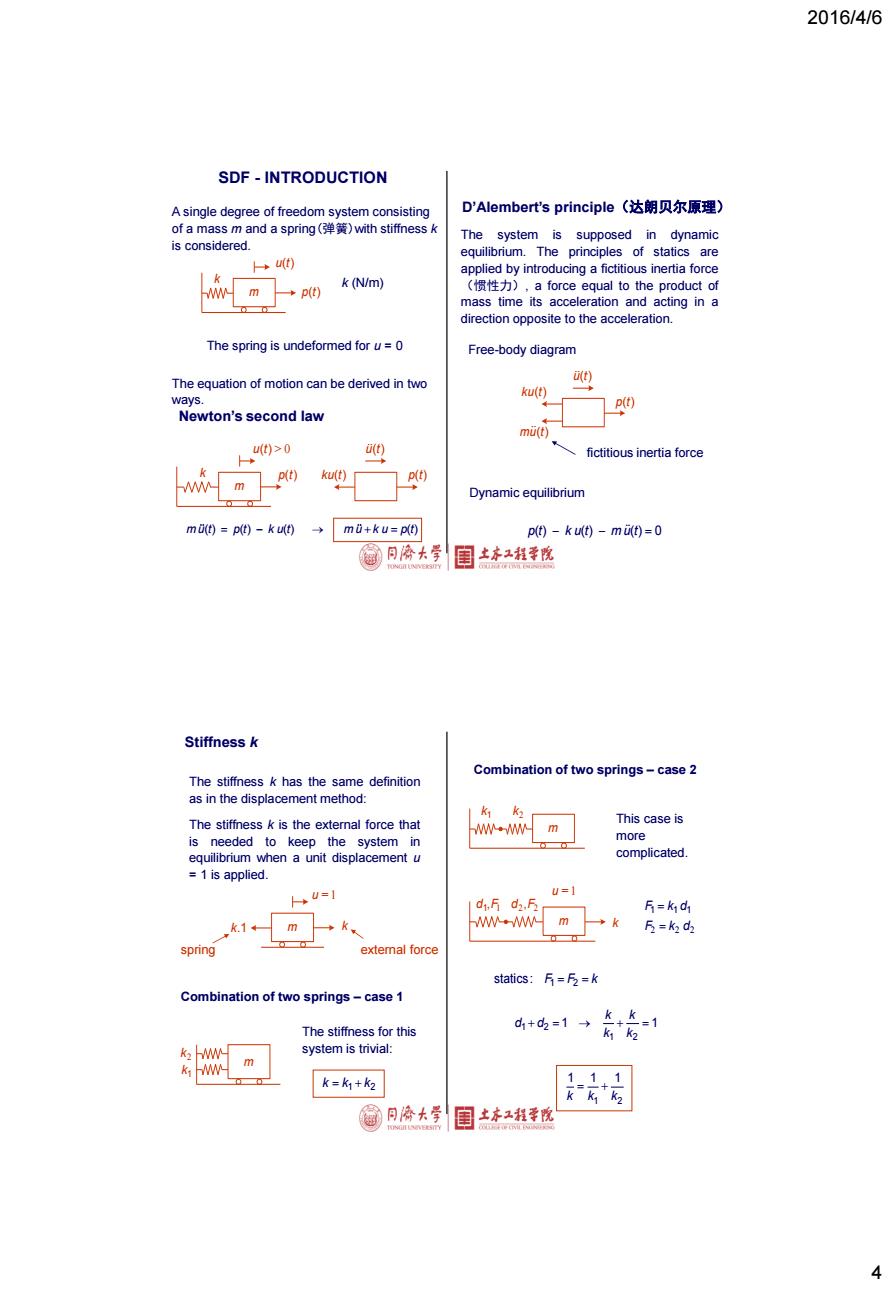
2016/4/6 SDF-INTRODUCTION A single degree of freedom system consisting D'Alembert's principle(达朗贝尔原理) of a mass m and a spring(弹簧)with stiffness k The system is supposed in dynamic is considered. equilibrium.The principles of statics are applied by introducing a fictitious inertia force k(N/m) m p(t) (惯性力)，a force equal to the product of mass time its acceleration and acting in a direction opposite to the acceleration. The spring is undeformed for u=0 Free-body diagram The equation of motion can be derived in two i(t) ku(t) ways. Newton's second law mi(t) u(t)>0 i(t) fictitious inertia force o(t) ku(t) p(t) M Dynamic equilibrium m(t)=p(t)-ku(t) mu+ku=p(t) p(t)-ku(t)-mi(t)=0 目土永2程学院 Stiffness k Combination of two springs-case 2 The stiffness k has the same definition as in the displacement method: k The stiffness k is the extemnal force that This case is W is needed to keep the system in more equilibrium when a unit displacement u complicated. 1 is applied. U= U=1 di.F d2.F F=k d W m 5=k2d spring extemal force statics:F=F2=k Combination of two springs-case 1 The stiffness for this d1+d2=1→ kK=1 K2 FM system is trivial: 名m k=k+k2 11,1 k k3 K2 同橋大学 士本工相学院 4

2016/4/6 4 A single degree of freedom system consisting of a mass m and a spring(弹簧)with stiffness k is considered. SDF - INTRODUCTION The spring is undeformed for u = 0 k (N/m) Newton’s second law m u (t)  p(t)  k u(t)  m u   k u  p(t) D’Alembert’s principle（达朗贝尔原理） p(t)  k u(t)  m u (t)  0 fictitious inertia force Dynamic equilibrium The equation of motion can be derived in two ways. The system is supposed in dynamic equilibrium. The principles of statics are applied by introducing a fictitious inertia force （惯性力）, a force equal to the product of mass time its acceleration and acting in a direction opposite to the acceleration. Free-body diagram k m u(t) p(t) k m u(t) > 0 p(t) ku(t) p(t) u(t) p(t) ku(t) u(t) mu(t) Stiffness k The stiffness k has the same definition as in the displacement method: The stiffness k is the external force that is needed to keep the system in equilibrium when a unit displacement u = 1 is applied. Combination of two springs – case 1 The stiffness for this system is trivial: 1 2 k  k  k Combination of two springs – case 2 m k u = 1 k.1 1k m 2 k 1k m 2 k d F1 ,1 m u = 1 k d2 F2 , This case is more complicated. 2 2 d2 F k F k d  1  1 1 F  F  k 1 2 statics: 1 1 1 2 1  2     k k k k d d 1 2 1 1 1 k k k   spring external force

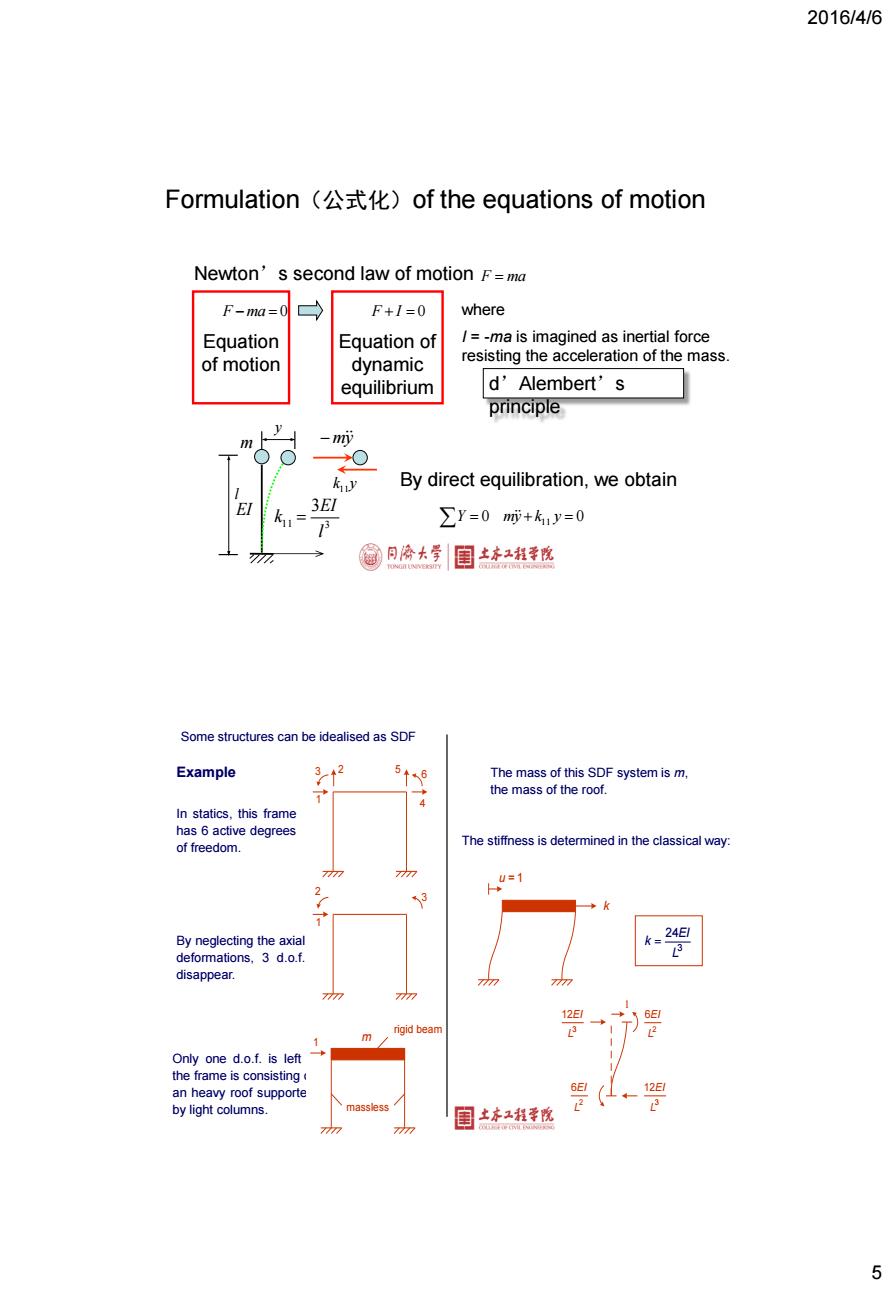
2016/4/6 Formulation(公式化)of the equations of motion Newton's second law of motion F=m F-ma=0 F+1=0 where Equation Equation of /=-ma is imagined as inertial force of motion dynamic resisting the acceleration of the mass. equilibrium d'Alembert's principle By direct equilibration,we obtain 3El 3 ∑Y=0m+k1y=0 同桥大学 士齐工程季晚 Some structures can be idealised as SDF Example 3 *2 The mass of this SDF system is m, the mass of the roof. In statics,this frame has 6 active degrees of freedom. The stiffness is determined in the classical way: 777 By neglecting the axial 24E k= deformations,3 d.o.f. 3 disappear 7770 7777 12E rigid beam Only one d.o.f.is left the frame is consisting an heavy roof supporte 6E 12E by light columns. massless 12 1土本工程学院 t导 5

2016/4/6 5 Equation of dynamic equilibrium Formulation（公式化）of the equations of motion Newton’s second law of motion F  ma F ma  0 F  I  0 I = -ma is imagined as inertial force resisting the acceleration of the mass. where d’Alembert’s principle Equation of motion m EI y l k y 11  m  y  11 3 3 l EI k  0 0 Y  m  y   k11 y  By direct equilibration, we obtain Example 3 24 L EI k  Only one d.o.f. is left if the frame is consisting of an heavy roof supported by light columns. 1 2 6 5 4 3 1 2 3 1 m rigid beam massless u = 1 k 1 3 12 L EI 2 6 L EI 3 12 L EI 2 6 L EI Some structures can be idealised as SDF In statics, this frame has 6 active degrees of freedom. By neglecting the axial deformations, 3 d.o.f. disappear. The mass of this SDF system is m, the mass of the roof. The stiffness is determined in the classical way:

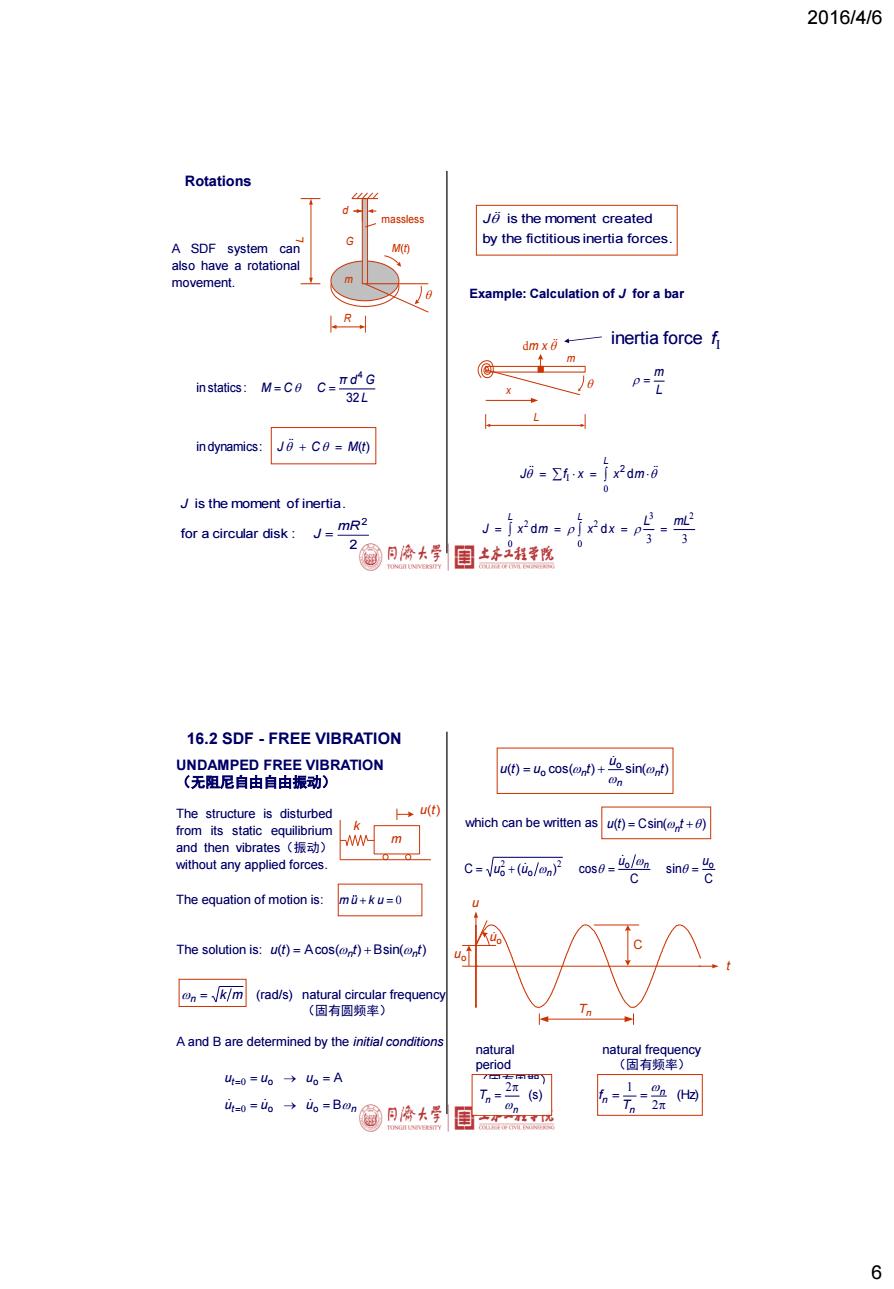
2016/4/6 Rotations J6 is the moment created by the fictitious inertia forces A SDF system can M(t) also have a rotational movement. Example:Calculation of J for a bar LR dmx6 inertia force f in statics: M=C0 C=md'G 32L in dynamics: J6+C0=M(t) Ji-∑所x=jx2dm-8 J is the moment of inertia. J=mR2 3_ml2 for a circular disk: 2 J-jxam-pixdx- 3 同桥大学 国土本相幸院 0 16.2 SDF-FREE VIBRATION UNDAMPED FREE VIBRATION u(t)=u cos(+osin() (无阻尼自由自由振动) n The structure is disturbed +u(t) from its static equilibrium which can be written as u(t)=Csin(t+) m and then vibrates(振动) without any applied forces C=√6+(/on2 cos8=6色2 sin-号 The equation of motion is: m0+ku=0 The solution is:u(t)=Acos(@t)+Bsin(@t) on=k/m (rad/s)natural circular frequency (固有圆频率) A and B are determined by the initial conditions natural natural frequency period (固有频率) t=0=u0→U0=A 1 4o=i。→=B@n盒同将大学 (s) n（H n 2 6

2016/4/6 6 Rotations indynamics: J   C   M(t)  2 for a circular disk : is the moment of inertia. 2 mR J J        L J f x x m 0  I    d 2 3 3 2 0 0 3 2 2 mL L J x m x x L L   d    d    L m   I inertia force f  massless m L R dG M(t)  m x  dm x L A SDF system can also have a rotational movement. L π d G M C C 32 in statics: 4    by the fictitious inertia forces. J  is the moment created Example: Calculation of J for a bar 16.2 SDF - FREE VIBRATION UNDAMPED FREE VIBRATION （无阻尼自由自由振动） u(t) Acos( t) Bsin( t)  n  n n  k m t n t u u u u u u B A o o o o            0 0 u(t)  Csin( t ) n (Hz) T f n n n    2 1  (rad/s) A and B are determined by the initial conditions which can be written as natural circular frequency （固有圆频率） The structure is disturbed from its static equilibrium and then vibrates（振动） without any applied forces. The equation of motion is: m u   k u  0 The solution is: ( ) cos( ) sin( ) o o t u u t u t n n n       k m u(t) C sin C C ( ) cos o o o o u u u u n   n         2 2 natural period （固有周期） natural frequency （固有频率） uo uo  Tn u t C T (s) n n    2

2016/4/6 Energy in undamped free vibration At each instant of time,the total energy E is made of two parts,the kinetic energy Ek and the strain energy Es. E()-ku) Ex(t)=mu(t) E。=max E.=0 Ek=0 Ek=max E(0=Ex)+E,() -mCnCOs(t++kCsint+ Remark:the conservation of energy can be used to derive the differential equation. -mC2cos(@nt+0)+kC2sin(nt+0) E0)-m+k -KC (k=m2)） conservation of energy dE =0 dt E(is constant,which implies conservation of energy. dE=m00+ku=0→m0+k知=0 d 同桥大学 国土本2程学院 16.3 Damped vibration(阻尼振动)of one degree systems A and B are determined by the initial conditions Friction in the structure is u(t) idealized by a linear viscous u(t)=-ore[Acos(@,t)+Bsin(t)] damper(线性粘滞阻尼器 )which develops a force +enL-Aa。sin(o)+Ba。cos(o, proportional to the velocity 6=-ca) 4=0=u0→6=A 4=0=i。→。=-nA+Bam。 The equation of motion is: mi+cǜ+ku=0 U(t)=e-iet u。coso,+。+sino.】 if c<c=2km (criticaldamping)the solutionis u(t)=e [Acos()+Bsin(t)] The solution can also be written as u(t)=Ce sin(t+0) sine=o damping ratio damped pulsation c Cr 2√km 0。=a,V-a C= 6+ cos9=4。+in46 Cap 同大学 士本工程学院 7

2016/4/6 7 Energy in undamped free vibration At each instant of time, the total energy E is made of two parts, the kinetic energy Ek and the strain energy Es. 2 2 1 E (t) m u(t) k   2 2 1 E (t) k u(t) s      C ( ) C cos ( ) C sin ( ) C cos( ) Csin( ) ( ) ( ) ( ) k k m m t k t m t k t E t E t E t n n n n n n n k s 2 2 2 2 2 2 2 2 2 2 1 2 1 2 1 2 1 2 1                        E(t) is constant, which implies conservation of energy. Remark : the conservation of energy can be used to derive the differential equation. 2 2 2 1 2 1 E(t)  m u  k u   0 t E d d conservation of energy  muu  kuu  0  mu  ku  0 t E    d d u t = max = 0 k E s E = 0 = k E s E max = 0 = k E s E max mu   c u   k u  0 if c c 2 km (criticaldamping) the solutionis  r  u(t) Acos( t) Bsin( t) D D t n      e km c c c r 2    2   1 D ωn    A sin( ) B cos( ) ( ) Acos( ) Bsin( ) t t u t t t D D D D D D t t n n n                  e  e t n D t u u u u u u ξω A Bω A 0 o o 0 o o                        ( ) cos( ) sin( ) o o o t u u u t u t D D D n t n        e ( ) C sin( )     u t t D t n e D u nu ω ξω θ C cos o  o   Friction in the structure is idealized by a linear viscous damper(线性粘滞阻尼器 )which develops a force proportional to the velocity f c u(t) D    A and B are determined by the initial conditions. The solution can also be written as The equation of motion is: damping ratio damped pulsation 2 2            D u u u n ω o ξω o C o  C sin uo θ  k m u(t) c 16.3 Damped vibration（阻尼振动）of one degree systems

2016/4/6 Ce-fai! p periods between two maximal points un and un+p tntp=in+pTo Un Ce-ontn sin(n+) Unsp Cewn(n+pTo)sin(o(n+pTo)+0) 2元 T。= =efenTo 2π Decay of motion nun=5anpT。=5n Un+p Onv1-52 A free vibration test can be used to determine experimentally the natural Un frequency(固有频率)and the damping 51(c>2mo) overdamped(过阻尼) C,=2m0 c.2mo =5 8

2016/4/6 8 Decay of motion pTD t t np  n  D D D D D pT n (t pT ) n t n p n n n n n n ( (t pT ) ) ( t ) u u ξω ξω ξω e e ω θ e ω θ          C sin C sin 2 n n n n p n 1 pT p u u D            2 ln n p n u u p         ln 2 1  0.1 1  1  2 n un p u and p points  periods between two maximal A free vibration test can be used to determine experimentally the natural frequency（固有频率）and the damping of a structure. D TD  2  t e C n n t n p t + un un+p u t m  y  cy   k11y  0 m y(t) m  y (t) ( ) 11 k y t cy (t)   c / 2m 2 0 2  y   y   y  t y t Ae ( )  2 0 2 2      Proper equation root 2    i 1  1(c  2m) Let 2  D  1 0 0 y(0)  y , y (0)  y  1 0 0 2 0 c (y y )/ ,c y     D  ( ) sin( ) D D t y t Ae  t      ( ) ( sin cos ) 1 2 y t e c t c t D D t       2 0 2 0 ( ) D y y A y      tan /( ) 0 0 0 y y y  D   D    1(c  2m) t y t c c t e  ( )  (  ) 1 2 cr  2m     m c c c r 2  1(c  2m) underdamped(欠阻尼) overdamped（过阻尼） Critical damping (临界阻尼)

2016/4/6 Example:Give the mass an initial displacement of 2cm by applying a force of 16.4kN.The amplitude reduces to 1 cm after 4 cycles within 2 seconds.Determine the parameters of the system. Solution: 2cm 1.Damping ratio(阻尼比)5= -In =0.0276 16.4kN 2π×41 2.Stiffness coefficient(刚度系数) 飞，=164x10 =8.2×105(N/m) 0.02 3.Undamped period(无阻尼周期) T。=2/4=0.5(s) T=ToV1-52=0.4998(s) 4.Weight 0=27-=12.5711s) 5.Damping coefficient(阻尼系数) T m=k1/o2=5190kg) c=2mo5=3601(N.s/m) W=-mg=50.86(kN)@向海大学 目土水工程学院 16.4 Undamped forced vibration of one-degree systems SDF-HARMONIC EXCITATIONS Example 2 y A harmonic load →U(t) is applied to the W m P。sin(et) m structure. y=u+ug k12 Without damping,the equation of motion is my=-ku 才7977777 mi+k u=po sin(ot) Ug(t)=Ugo sin(ot) Example 1 If u is to be studied (e.g.earthquake) The system consisting m(u+Ug)=-ku of the mass m and the excentric mass-m is =rsin(ot mi+ku =mugo @2 sin(at) considered for writing Newton's equation. 多k12k12 If y is to be studied(e.g.floor isolation) 7777 (m-而)ū+而(v+ū)=-ku my =-k(y-ug) mu+ku mro-sin(ot) my+k y =k uoo sin(ot) 同海大学 国士本工程学悦 9

2016/4/6 9 Example: Give the mass an initial displacement of 2cm by applying a force of 16.4kN. The amplitude reduces to 1 cm after 4 cycles within 2 seconds. Determine the parameters of the system. 2cm 16.4kN Solution: 1.Damping ratio（阻尼比） 0.0276 1 2 ln 2 4 1      2.Stiffness coefficient（刚度系数） 8.2 10 ( / ) 0.02 16.4 10 5 3 k1 1   N m   3.Undamped period（无阻尼周期）  2/ 4  0.5(s) TD 1 0.4998(s) 2 T  TD   4.Weight 12.57(1/s) 2   T   / 5190(kg) 2 m  k11   W  mg  50.86(kN) 5.Damping coefficient（阻尼系数） c  2m  3601(Ns/m) SDF - HARMONIC EXCITATIONS sin( ) o m u   k u  p t Example 1 sin( ) ( ) ( ) m u k u m r t m m u m v u k u   2            m u k u m u sin( t) m u u k u 2 go g          (   ) A harmonic load is applied to the structure. Without damping, the equation of motion is The system consisting of the mass m and the excentric mass m is considered for writing Newton’s equation. v = r sin(t) m m k / 2 k / 2 u k m u(t) sin( ) o p t u (t) u sin( t) g = go  k / 2 k / 2 u m Example 2 y y  u  ug m y   k u If u is to be studied (e.g. earthquake) m y k y k u sin( t) m y k y u go g          ( ) If y is to be studied (e.g. floor isolation) 16.4 Undamped forced vibration of one-degree systems

2016/4/6 WITHOUT DAMPING u(t) C and 6 are determined by the initial conditions Po sin(ot) u(t)is a summation of two sinus and is not mu+k u=po sin(oxt) defined for = In reality,the damping implies that u(t) The solution u(t)of the differential disappears after some time.Then the equation is the sum of two parts up(f)and solution steady state response )is U(⑨ u(t)=Un(t)+Up(t) u(t)=Up(t)=-Po/k (/sin(ot) homogeneous solution u(t)=Csin(t+6) conclusions particular solution up(t)=Asin(ot) After some time,the structure vibrates with Up(t)=-Ao2sin(ot) the same frequency as the applied force The amplitudes of the vibration are infinite →-mAo2+kA=P%→A= Po Polk I-(ofon)2 when =cn- k-ma u,()is the transient response(瞬态响应) u(t)=Csin(cnt+0)+ 6/k sin(@t) -(@ln)f u,(0 is the steady state response(稳态响应) 大学 闺土水2程学悦 NITH VISCOUS DAMPING(粘性阻尼) after calculations(see the book),it is obtained u(t) po sin(ot) m Po/k -M sin(ot-) V-(olonY]+25(oloaT 25(@l@d mu+cu+k u=po sin(ot) tan= 0<p<180 1-(@lon月 The homogeneous solution u(f)(transient response)disappears after some time. Remarks Un(t)=Cessin(+0) The total response is u(f)=un(f)+up(t).But after some time un(t)disappears and u(t)= The particular solution u(f)(steady state p(f)(steady state response) response)is of the form: After some time,the structure vibrates with the same frequency as the applied force. Up(t)=Asin(ot-) 土本工程学院 10

2016/4/6 10 WITHOUT DAMPING sin( ) o m u   k u  p t u (t)  Csin( t ) h ωn homogeneous solution particular solution u (t) Asin( t) p   2 2 1 ( ) / A A o o o n 2 p k k m p m kA p ω  ω ω          u (t) A sin( t) p   2    C and  are determined by the initial conditions u(t) is a summation of two sinus and is not defined for  = n. In reality, the damping implies that uh(t) disappears after some time. Then the solution ( steady state response ) is sin( ) ( ) ( ) ( ) o t p k u t u t n p    2 1   conclusions • After some time, the structure vibrates with the same frequency as the applied force. • The amplitudes of the vibration are infinite when  = n. up (t) is the steady state response(稳态响应) uh (t) is the transient response(瞬态响应) u(t) u (t) u (t)  h  p The solution u(t) of the differential equation is the sum of two parts uh (t) and up (t). k m u(t) sin( ) o p t sin( ) ( ) ( ) Csin( ) t p k u t t n o n ω 2 1         WITH VISCOUS DAMPING(粘性阻尼) sin( ) o m u   c u   k u  p t ( ) C sin( )     u t t D t h n e The homogeneous solution uh (t) (transient response) disappears after some time. The particular solution up (t) (steady state response) is of the form: u (t)  Asin(t ) p after calculations (see the book), it is obtained     sin( ) ( ) ( ) ( ) o            t p k u t n n p 2 2 2 1 2  0 180 1 2 2            n n ( ) ( ) tan Remarks • The total response is u(t) = uh(t) + up(t). But after some time uh(t) disappears and u(t) = up(t) (steady state response). • After some time, the structure vibrates with the same frequency as the applied force. k m u(t) c sin( ) o p t