
ENGINEERING SOCIETIES MONOGRAPHS Bakhmeteff:Hydraulics of Open Channels THEORY Bleich:Buckling Strength of Metal Structures Crandall:Engineering Analysis Elevatorski:Hydraulic Energy Dissipators OF Leontovich:Frames and Arches Nadai:Theory of Flow and Fracture of Solids Timoshenko and Gere:Theory of Elastic Stability ELASTICITY Timoshenko and Goodier:Theory of Elasticity Timoshenko and Woinowsky-Krieger:Theory of Plates and Shells Five national engineering societies,the American Society of Civil Engineers,the Amcrican Institute of Mining,Metallurgical,and Petroleum Engineers,the American By S.TIMOSHENKO Society of Mechanical Engineers,the Amcrican Institute of Electrical Engincers, and the American Institute of Chemical Engineers,have an arrangement with the MeGraw-Hill Book Company,Inc.,for the produetion of a series of selected books And J.N.GOODIER adjudged to possess usefulness for engineers and industry. The purposes of this arrangement are:to provide monographs of high technical Professors of Engineering Mechanics quality within the field of engineering;to rescue from obseurity important technical Stanford Universit到 manuscripts which might not be published commercially because of too limited sale e e ocere nfe subject. The societies assume no responsibility for any statements made in these books. Each book before publication has,however,been examined by one or ore representa- tives of the societics competent to express an opinion on the merits of the manuseript. Ralph H.Phelpe,CHAIRMAN Engineering Societies Library New York IBLIOT含CAC客TAi UNIVERSITATRA .POLITERNICA ENGINEERING SOCIETIES MONOGRAPHS COMMITTEE TI红8OARA A.S.C.E. Howard T.Critehlow 00128156 H.Alden Foster A.I.M.E. IHST门TUJuloP%L驿IC TIMIS0ARA Nathaniel Arbiter John F.Elliott B:F A A.8.M.E. Calvin S.Cronan Raymond D.Mindlin Yolunu' 39.121 Culap A.I.E.E. 又02.D F.Maleolm Farmer NEW YORK TORONTO LONDON Royal W.Sorensen McGRAW-HILL BOOK COMPANY,INe. A.I.Ch.E. Joseph F.Skelly 1951 Charles E.Reed

PREFACE TO THE SECOND EDITION The many developments and clarifications in the theory of elasticity and its applications which have occurred since the first edition was written are reflected in numerous additions and emendations in the present edition.The arrangement of the book remains the same for the most part. The treatments of the photoelastic method,two-dimensional problems in curvilinear coordinates,and thermal stress have been rewritten and enlarged into separate new chapters which present many methods and solutions not given in the former edition.An appendix THEORY OF ELASTICITY on the method of finite differences and its applications,including the relaxation method,has been added.New articles and paragraphs Copyright,1934,by the United Engineering Trustees,Ine.Copyright,1951,by the MeGraw- incorporated in the other chapters deal with the theory of the strain Hill Book Company,Ine.Printed in the United gauge rosette,gravity stresses,Saint-Venant's prineiple,the components States of America.All rights reserved.This book, of rotation,the reciprocal theorem,general solutions,the approximate or parts thereof,may not be reproduced in any character of the plane stress solutions,center of twist and center of form without permission of the publishers. shear,torsional stress concentration at fillets,the approximate treat- XIIL ment of slender (e.g.,solid airfoil)sections in torsion and bending, and the cireular cylinder with a band of pressure. 6M719 Problems for the student have been added covering the text as far as the end of the chapter on torsion. It is a pleasure to make grateful acknowledment of the many helpful suggestions which have been contributed by readers of the book. S.TIMOSHENKO J.N.GooDIER PALO ALTO,CALIF February,1951 THE MAPLE PRESS COMPANY,TORK,PA

PREFACE TO THE FIRST EDITION During recent years the theory of elasticity has found considerable application in the solution of engineering problems.There are many cases in which the elementary methods of strength of materials are inadequate to furnish satisfactory information regarding stress distri- bution in engineering structures,and recourse must be made to the more powerful methods of the theory of elasticity.The elementary theory is insufficient to give information regarding local stresses near the loads and near the supports of beams.It fails also in the cases when the stress distribution in bodies,all the dimensions of which are of the same order,has to be investigated.The stresses in rollers and in balls of bearings can be found only by using the methods of the theory of elasticity.The elementary theory gives no means of investigating stresses in regions of sharp variation in cross section of beams or shafts.It is known that at reentrant corners a high stress concentration occurs and as a result of this eracks are likely to start at such corners,especially if the structure is submitted to a reveraal of stresses.The majority of fractures of machine parts in service can be attributed to such cracks. During recent years considerable progress has been made in solving such practically important problems.In cases where a rigorous solu- tion cannot be readily obtained,approximate methods have been developed.In some cases solutions have been obtained by using experimental methods.As an example of this the photoelastic method of solving two-dimensional problems of elasticity may be mentioned.The photoelastic equipment may be found now at universities and also in many industrial research laboratories.The results of photoelastic experiments have proved especially useful in studying various cases of stress concentration at points of sharp variation of cross-sectional dimensions and at sharp fillets of reentrant corners.Without any doubt these results have considerably influ- enced the modern design of machine parts and helped in many cases to improve the construction by eliminating weak spots from which cracks may start. Another example of the successful application of experiments in the solution of elasticity problems is the soap-film method for deter- mining stresses in torsion and bending of prismatical bars.The vii

PREPACE T0 THE FIRST EDITION PREFACE TO THE FIRST EDITION ix vi进 difficult problems of the solution of partial differential equations with To simplify the presentation,the book begins with the discussion of given boundary conditions are replaced in this case by measurements two-dimensional problems and only later,when the reader has familiar. of slopes and deflections of a properly stretched and loaded soap film. ized himself with the various methods used in the solution of problems The experiments show that in this way not only a visual picture of of the theory of elasticity,are three-dimensional problems discussed. the stress distribution but also the necessary information regarding The portions of the book that,although of practical importance,are magnitude of stresses can be obtained with an accuracy sufficient for such that they can be omitted during the first reading are put in small practical application. type.The reader may return to the study of such problems after Again,the electrical analogy which gives a means of investigating finishing with the most essential portions of the book. torsional stresses in shafts of variable diameter at the fillets and The mathematical derivations are put in an elementary form and grooves is interesting.The analogy between the problem of bending usually do not require more mathematical knowledge than is given in of plates and the two-dimensional problem of elasticity has also been engineering schools.In the cases of more complicated problems all successfully applied in the solution of important engineering problems. necessary explanations and intermediate calculations are given so In the preparation of this book the intention was to give to engi- that the reader can follow without difficulty through all the deriva- neers,in a simple form,the necessary fundamental knowledge of the tions.Only in a few cases are final results given without complete theory of elasticity.It was also intended to bring together solutions derivations.Then the necessary references to the papers in which the of special problems which may be of practical importance and to derivations can be found are always given. describe approximate and experimental methods of the solution of In numerous footnotes references to papers and books on the theory elasticity problems. of elasticity which may be of practical importance are given.These Having in mind practieal applications of the theory of elasticity, references may be of interest to engineers who wish to study some matters of more theoretical interest and those which have not at special problems in more detail.They give also a picture of the present any direct applications in engineering have been omitted in modern development of the theory of elasticity and may be of some favor of the discussion of specific cases.Only by studying such cases use to graduate students who are planning to take their work in this with all the details and by comparing the results of exact investigations field. with the approximate solutions usually given in the elementary books In the preparation of the book the contents of a previous book on strength of materials can a designer acquire a thorough under- ("Theory of Elasticity,"vol.I,St.Petersburg,Russia,1914)on standing of stress distribution in engineering structures,and learn to the same subject,which represented a course of lectures on the theory use,to his advantage,the more rigorous methods of stress analysis. of elasticity given in several Russian engineering schools,were used In the discussion of special problems in most cases the method to a large extent. of direct determination of stresses and the use of the compatibility The author was assisted in his work by Dr.L.H.Donnell and Dr. equations in terms of stress components has been applied.This J.N.Goodier,who read over the complete manuscript and to whom method is more familiar to engineers who are usually interested in the he is indebted for many corrections and suggestions.The author magnitude of stresses.By a suitable introduction of stress functions takes this opportunity to thank also Prof.G.H.MacCullough,Dr. this method is also often simpler than that in which equations of E.E.Weibel,Prof.M.Sadowsky,and Mr.D.H.Young,who assisted equilibrium in terms of displacements are used. in the final preparation of the book by reading some portions of the In many cases the energy method of solution of elasticity problems manuscript.He is indebted also to Mr.L.S.Veenstra for the prep- has been used.In this way the integration of differential equations is aration of drawings and to Mrs.E.D.Webster for the typing of the replaced by the investigation of minimum conditions of certain inte- manuscript. grals.Using Ritz's method this problem of variational calculus is reduced to a simple problem of finding a minimum of a funetion. S.TIMOSHENKO In this manner useful approximate solutions can be obtained in many UNIVERSITY OF MICHIGAN practically important cases. December,1933

CONTENTS PREFACE TO THE SKCOND EDITION.。。,。。。, PREFACE TO TE%FIRST EDETION.·,,、·,·,。·,,····,。 妙 NOTATION. CHAPTER 1.INTRODUCTION 1 2 2.Stre88.,,, 3.Notation for Forees and Stresses. 3 4.Components of Stress. 5.Components of Strain,·,·,·· G Problems······· 10 CHAPTKR 2.PLANE STRESS AND PLANE STRAIN 7.Plane Stre8s········,··· 11 8.Plane Strain,.。,.。,。.··,··· 4 11 9.Ste88 t a Point,.········· 培 10.Strain at a Point.... 11.Measurement of Surface Strains. 9 12.Construetion of Mohr Strain Cirele for Strain Rosette. l3.Differential Equations of Equilibrium.。。。.。,,· 2 14.Boundary Conditions,.·。·······,, 15.Compatibility EquationB.,。,。。,,.,,,, 23 16.8 tress Function.,,··+······+,·- Problem。··t4··”4 马 CHAPTER 3.TWO-DIMENSIONAL PROBLEMS IN RECTANGULAR COORDINATES 17.Solution by Polynomials. 39 18.Saint-Venant's Prineiple. 19.Determination of Displacements 4 20.Bending of a Cantilever Loaded at the End 3 2l,Bending of a Beam by Uniform Load.·,·, 22.Other Cases of Continuously Loaded Beams. 44 23.Solution of the Two-dimensional Problem in the Form of s Fourier Series. 46 24.Other Applicntions of Fourier Series.Gravity Loading...... 53 Problems,,,,···· xi INSTITUTUL POLITEHN. TIMISOARA OTECA CENTRALA

xii CONTENTS CONTENTS xii CHAPTER 4.TWO-DIMENSIONAL PROBLEMSIN POLAR COORDINATES GL.Stress Components in Curvilinear Coordinates,····,·,,·lg5 25.General Equations in Polar Coordinates.. 55 Problems,。·····‘ ··.197 4,44■▣,44 26.Stress Distribution Symmetrical about an Axis, 58 62.Solutions in Elliptie Coordinates. 197 27.Pure Bending of Curved Bar8.··..·······,,·+· 61 63.Elliptic Hole in a Plate under Simple Tension·,,·,,··.- 201 204 28.Strain Components in Polar Coordinates. 65 G4 Hyperbolic Boundaries,Notches。.,.,,,,,·。。.,. 206 29.Displacementa for Symmetrieal Stress Distributions. 66 65.Bipolar Co0 rdinate8.·.·。。,--·········-- 30.Rotating Disk3.· 69 GG.Solutions in Bipolar Coordinates.。.·,.,,·····。。. 208 31.Bending of a Curved Bar by a Force at the End . 73 Other Curvilinear Coordinates,,,·,···· ,,212 32.The Effect of Cireular Holes on Stress Distributions in Plates .. 33.Coneentrated Foree at a Point of a Straight Boundary 85 CHAPTER 8.ANALYSIS OF STRESS AND STRAIN IN THREE DIMEN. 34.Any Vertical Loading of a Straight Boundary 81 SIONS 35.Force Acting on the End of a Wedge...,:,···. 6 67.Specification of8 tress at a Point,。·。,,,,·,······衫 213 36.Concentrated Force Aeting on a Beam. 09 68.Principal Stresses. 214 37.Streases in a Cireular Disk. 44 107 69.Stress Ellipeoid and Stress-direetor Surface........... 215 38.Force at a Point of an Infinite Plate. 112 70,Determination of the Prineipal Stres8cw,。,,,+,····。, 217 39.General Solution of the Two-dimensional Problem in Polar Coordi- 71.Determination of the Maximum Shearing Stress 218 nates. ···。。116 72.Homogeneou3 Deformation,··········· 219 40.Applications of the General Solution in Polar Coordinates.....121 73.Strain at a Point......... 221 41.A Wedge1 oaded along the Faces,·,····。。·,,,·,,123 74.Principal Axes of Strain.... 4 224 Problem8,·。,,···,····125 75.otat50n..。·.。··,· 225 Problem. 227 CHAPr&R 5.THE PHOTOELASTIC METHOD 42.Photoelastic Stress Measurement..... 131 CHAPTER 9.GENERAL THEOREMS 43.Circular Polariscope. 135 76.Differential Equntion3 of Equilibrium,,,,,,。,·。。。·, 228 44.Examples of Photoelastic Stress Determination 138 77.Conditions of Compatibility.. 220 45.Determination of the Principal Stresscs.,,..,+···,·, 142 78.Determination of Displncementa 232 48.Three-dimensional Photoelasticity,,····。,,,,,..。l43 79.Equations of Equilibrium in Terms of Displacements 233 80.General Solution for the Displacements,,+.·。。。,·,,t 235 CHAPTER 6.STRAIN ENERGY METHODS 8l.The Principle of Superposition,,,············· 235 82.Uniqueness of Solution,,,·····。···,·,· 236 47.Strain Energy.· 48.Principle of Virtual Work 151 83.The Reciprocal Theorem. 239 49.Castigliano's Theorem. 162 84.Approximate Character of the Plane Stress Solutions. 241 50.Prineiple of Least Work 160 Problem8···.。 244 51.Applications of the Principle of Least Work-Rectangular Plates..167 52.Effective Width of Wide Beam Flanges··,·········,1T1 CHAPTER 10.ELEMENTARY PROBLEMS OF ELASTICITY IN THREE 53.Shear Lag。·。.,。,,,。。。。。。,,。。·。。·。。·177 DIMENSIONS Probiems......... 177 85.Uniform Stress..,,,·· 245 8G.Stretching of a Prismatical Bar by Tts Own Weight.。。。.,,,246 CHAPTER 7.TWO-DIMENSIONAL PROBLEMS IN CURVILINEAR COOR- 87.Twist of Circular Shafts of Constant Crogs Section...... 249 DINATES 88.Pure Bending of Prismatical Bars. 54.Functions of a Complex Variable................179 8般.Pure Bending of Plates··,··· ,+·。255 55.Analytie Funetions and Laplace's Equation ,......... 181 Problems. .··182 CHAPTER 11.TORSION OF PRISMATICAL BARS 56.Stress Functions in Terms of Harmonic and Complex Functions..183 90.Torgion of Prismatical Bars,.,,,,:·,.,。。···· 258 87.Displacement Corresponding to a Given8 tress Funetion,·,···1l86 91.Bars with Elliptical Cross Section................ 263 58.Stress and Displacement in Terms of Complex Potentials.....187 92.Other Elementary Solutions.................. 265 59.Resultant of Stress on a Curve.Boundary Conditions......190 g3.Membrane Analogy,。·,···· 268 60.Curvilinear Coordinates. --4192 94.Torsion of a Bar of Narrow Reetangular Cross Seetion..... 272

XV xiv CONTENTS CONTENTS 276 135.The Long Cireular Cylinder·,··· 408 95.Torsion of Rectangular Bara................. 410 96.Additional Results. 278 136.The Sphere·,··· g7.Solution of Torsional Probleme by Energy Method,.·,··· 280 137.General Equations 、 421 98.Torsion of Rolled Profile Seetions. .287 138.Initial Stresses.. 425 99.The Uae of Soap Films in Solving Torsion Problems.. ··.289 139.Two-dimensional Problems with Steady Heat Flow 427 100.Hydrodynamical Analogies... 140.Solutions of the General Equations 44 433 10l.Torsion of Hol0 w Shafts:.,,··········,···· 294 102.Torsion of Thin Tubes . 298 CHAPTER 15. THE PROPAGATION OF WAVES IN ELASTIC SOLID MEDIA 103.Torsion of a Bar in which One Cross Section Remains Plane. 302 438 104.Torsion of Circular Shafta of Variable Dinmeter.........304 141..·· Problem···············。·,,313 142.Longitudinal Waves in Prismatical Bars. ···。38 143.Longitudinal Impact of Bars... 444 CHAPTER 12.BENDING OF PRISMATICAL BARS 144.Waves of Dilatation and Waves of Distortion in Isotropic Elastie Media.· 452 454 105.Bending of n Cantilever···,············316 145.Plane Waves....... ·,318 146.Propagation of Waves over the Surfnce of an Elnstie Solid Body... 456 106.Stress Funetion,.·.·, 107.Cireular Cross Section. 319 108.Elliptie Cross Seetion 321 APPENDIX. THE APPLICATION OF FINITE DIFFERENCE EQUATIONS 109.Rectangular Cross Section 323 IN ELASTICITY 110.Additional Results .. 329 l,Derivation of Finite Difference Equations,,·,,·····, 461 111.Nonsymmetrical Cross Sections......... ······831 2.Methods of8 uccessive Approximation,.·······.。,.. 465 1l2.8 henr Center,.....:,,·····,, 333 113.The Solution of Bending Problems by the Soap-film Method.... 336 3.Relaxation Method..... 468 4.Triangular and Hexagonal Nets 473 t 340 5.Block and Group Relaxation..... 477 115.Further Investigations of Bending. 341 6.Torsion of Bars with Multiply-conneeted Cross Sections. 479 7.Points Near the Boundary.... 480 CHAPTER 18.AXIALLY SYMMETRICAL STRESS DISTRIBUTION IN A 8.Biharmonic Equation,·· 483 g.Toraion of Cireular8 hafts of Variable Diameter,·+·,,··· 400 SOLID OF REVOLUTION 116.General Equation8,:,·············348 495 1l7.Solution by Polynomials.····,,。。·。。。,,。·..347 AUTHOR INDEX. 118.Bending of a Circular Plate. 349 499 119.The Rotating Disk as a Three-dimensional Problem. 352 120.Force at a Point of an Indefinitely Extended Solid........ 354 121.Spherical Container under Internal or External Uniform Pressure.. 356 122.Local Stresses around a Spherieal Cavity:··,·,····,,,359 l23.Force on Boundary of a Semi-infinite Body.,,,·。·. ,362 124.Load Distributed over a Part of the Boundary of a Semi-infinite Solid 366 125.Pressure between Two Spherieal Bodies in Contaet.. ,372 126.Preasure between Two Bodies in Contact.More General Cnse .377 127.mpact of8 pheres。·,,, ···383 128. Symmetrical Deformation of a Circular Cylinder......... 384 l29.The Circular Cylinder with a Band of Pressure,,.·.····- 388 l30.Twist of a Cireular Ring Sector,,,·.,.·.。··,,.+ 391 l31,Pure Bending of a Circular Ring8 ector.,.·····,··· 395 CHAPTER 14.THERMAL STRESS 132.The Simplest Cases of Thermal Streas Diatribution ·······898 133.Some Problems of Plane Thermal Stress... 404 134.The Thin Circular Disk:Tempersture Symmetrical about Center.. 406

NOTATION 宝,h,2 Rectangular coordinates. 7,9 Polar coordinates. 志,”Orthogonal curvilinear coordinates;8 ometimes rec- tangular coordinates. R Spherical coordinates. N Outward normal to the surface of a body. 马,,n Direction cosines of the outward normal. A Cross-seetional area. I Iy Moments of inertia of a eross section with respeet t切nd珍ax线, Ip Polar moment of inertia of a cross seetion. Gravitational acceleration. Density. Intensity of a continuously distributed load. Pressure. X,Y.Z Components of a body force per unit volume. £,亚,2 Components of a distributed surface force per unit area. M Bending moment. Me Torque. 0!d3a Normal components of stress parallel to ,and -axe8. Normal component of stress parallel to n. es Radial and tangential normal stresses in polar coordinates. Normal stress components in curvilinenr co- ordinates. ns,Normal stress components in eylindrical co- ordinates. 0=ge十gy十gg=g十g十g Shearing stress. Try:Tan Yut Shearing-stress components in reetangular co- ordinates. Shearing stress in polar coordinates. Shearing stress in curvilinear coordinates. Trd:Tpry Trt Shearing-stress components in cylindrical co- ordinates. 8 Total stress on a plane. u,v,w Components of displacements. Unit elongation. Unit elongations in,and direetions. xvii

xvi进 NOTATION eRadial and tangential unit elongations in polar coordinates. e+Volume expansion. Unit shear. Yeur Yau Yw Shearing-strain components in rectangular co- CHAPTER 1 ordinates. Trd,ThY和 Shearing-strain components in eylindrical co- INTRODUCTION ordinates. E Modulus of elasticity in tension and compression. G Modulus of elasticity in shear.Modulus of 1.Elasticity.All structural materials possess to a certain extent rigidity. the property of elasticity,i.e.,if external forces,producing deformation Poisson's ratio of a structure,do not exceed a certain limit,the deformation disappears E with the removal of the forces.Throughout this book it will be “=G,A=0+0-2 Lamd's constants. assumed that the bodies undergoing the action of external forces are φStreas funetion. perfectly elastic,i.e.,that they resume their initial form completely after ()x(s)Complex potentials;funetions of the complex removal of forces. variable a =x+iy. The conjugate complex varinble z -iy. The molecular structure of elastic bodies will not be considered here. C Torsional rigidity. It will be assumed that the matter of an elastic body is homogeneous and Angle of twist per unit length. continuously distributed over its volume so that the smallest element P2G0 Used in torsional problems. cut from the body possesses the same specific physical properties as the y Strain energy. body.To simplify the discussion it will also be assumed that the body Ve Strain energy per unit volume. is isotropic,i.e.,that the elastic properties are the same in all directions. Time. T Certain interval of time.Temperature. Structural materials usually do not satisfy the above assumptions. a Coefficient of thermal expansion. Such an important material as steel,for instance,when studied with a microscope,is seen to consist of crystals of various kinds and various orientations.The material is very far from being homogeneous,but experience shows that solutions of the theory of elasticity based on the assumptions of homogeneity and isotropy can be applied to steel struc- tures with very great accuracy.The explanation of this is that the crystals are very small;usually there are millions of them in one cubic inch of steel.While the elastic properties of a single crystal may be very different in different directions,the crystals are ordinarily dis- tributed at random and the elastic properties of larger pieces of metal represent averages of properties of the crystals.So long as the geo- metrical dimensions defining the form of a body are large in comparison with the dimensions of a single crystal the assumption of homogeneity can be used with great accuracy,and if the crystals are orientated at random the material can be treated as isotropic. When,due to certain technological processes such as rolling,a cer- tain orientation of the crystals in a metal prevails,the elastic properties of the metal become different in different directions and the condition of anistropy must be considered.We have such a condition,for instance,in the case of cold-rolled copper
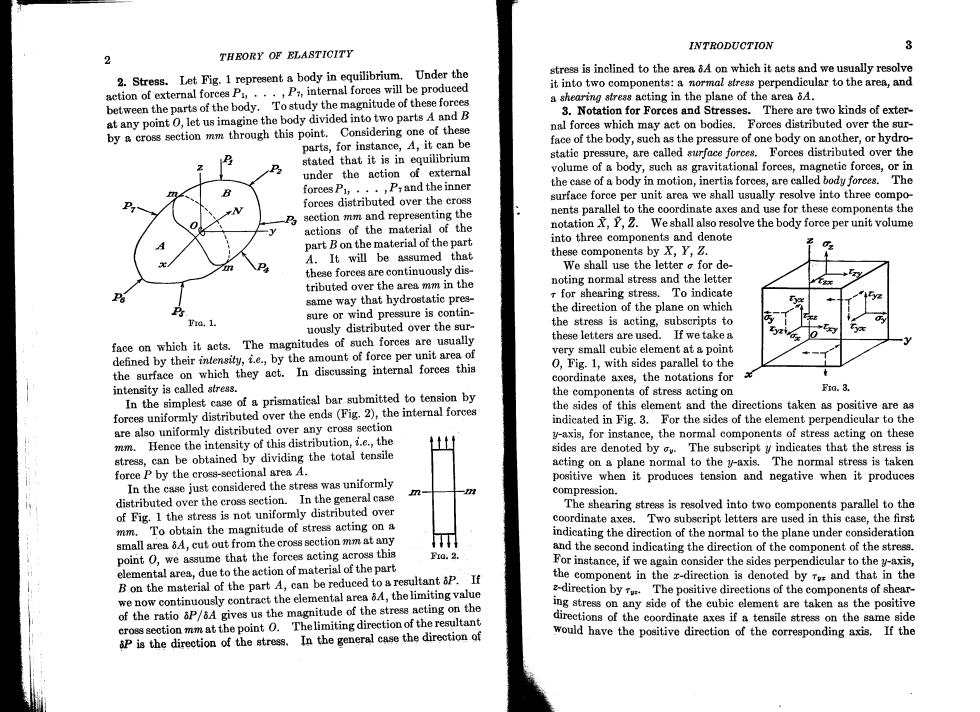
INTRODUCTION 3 2 THEORY OF ELASTICITY 2.Stress.Let Fig.1 represent a body in equilibrium.Under the stress is inclined to the area 6A on which it acts and we usually resolve action of external forces P...,P,internal forces will be produced it into two components:a normal stress perpendicular to the area,and between the parts of the body.To study the magnitude of these forces a shearing stress acting in the plane of the area 5A. at any point O,let us imagine the body divided into two parts A and B 3.Notation for Forces and Stresses.There are two kinds of exter- nal forces which may act on bodies.Forces distributed over the sur- by a cross section mm through this point. Considering one of these parts,for instance,A,it can be face of the body,such as the pressure of one body on another,or hydro- stated that it is in equilibrium static pressure,are called surface forces.Forces distributed over the under the action of external volume of a body,such as gravitational forees,magnetic forces,or in forces Pi,...,Prand the inner the ease of a body in motion,inertia forces,are called body forces.The forces distributed over the cross surface force per unit area we shall usually resolve into three compo- section mm and representing the nents parallel to the coordinate axes and use for these components the actions of the material of the notation X,F,2.Weshall also resolve the body force per unit volume part B on the material of the part into three components and denote A.It will be assumed that these components by X,Y,Z. these forees are continuously dis- We shall use the letter o for de- tributed over the area mm in the noting normal stress and the letter same way that hydrostatic pres- r for shearing stress.To indicate sure or wind pressure is contin- the direction of the plane on which Fro.1. uously distributed over the sur- the stress is acting,subscripts to face on which it acts.The magnitudes of such forces are usually these letters are used.If we take a defined by their intensity,i.e.,by the amount of force per unit area of very small cubic element at a point the surface on which they act.In discussing internal forees this 0,Fig.1,with sides parallel to the coordinate axes,the notations for intensity is called stress. In the simplest case of a prismatical bar submitted to tension by the components of stress acting on f1a.3. forces uniformly distributed over the ends (Fig.2),the internal forces the sides of this element and the directions taken as positive are as indicated in Fig.3.For the sides of the element perpendicular to the are also uniformly distributed over any croes section mm.Hence the intensity of this distribution,i.e.,the y-axis,for instance,the normal components of stress acting on these stress,can be obtained by dividing the total tensile sides are denoted by The subscript y indicates that the stress is acting on a plane normal to the y-axis.The normal stress is taken force P by the cross-sectional area A. positive when it produces tension and negative when it produces In the case just considered the stress was uniformly compression. distributed over the cross section.In the general case of Fig.I the stress is not uniformly distributed over The shearing stress is resolved into two components parallel to the coordinate axes.Two subscript letters are used in this case,the first mm.To obtain the magnitude of stress acting on a indicating the direction of the normal to the plane under consideration small area 6A,cut out from the croes section mm at any and the second indicating the direction of the component of the stress. point O,we assume that the forces acting across this 1a.2. elemental area,due to the action of material of the part For instance,if we again consider the sides perpendicular to the y-axis, B on the material of the part A,can be reduced to a resultant op.If the component in the r-direction is denoted by and that in the we now continuously contract the elemental area 8A,the limiting value -direction byThe positive directions of the components of shear- of the ratio p/6A gives us the magnitude of the stress acting on the ing stress on any side of the cubic element are taken as the positive eross section mm at the point O.Thelimiting direction of the resultant directions of the coordinate axes if a tensile stress on the same side 6P is the direction of the stress.In the general case the direction of would have the positive direction of the corresponding axis.If the