
2016/4/6 Chapter 6 Influence Lines() and Maximum Load Effects. 同大学 土工程学院 6.1 variable loadings() This old board doesn't look safe. I made it! SNAP! Mealtime' Almost! 月大学 土工程学院 2 1
2016/4/6 1 1 Chapter 6 Influence Lines(影响线) and Maximum Load Effects. 2 6.1 variable loadings(活载)

2016/4/6 6.2 variation in response function with position of load:influence line (影响线) I kip 1 kip movement - A 凡B L RA RB Ro=4 (a) (c) 1 kip I kip B ↑ R=I RB=0 RA Ri= (b) (d0 6.2 continued 1 kip B ↑ 2 R=0 RB=1 4 (e) Rg(kips) 3-4 1-2 (g) 0 Ra(kips) n @月冷大学 目土本工程季悦 2
2016/4/6 2 3 6.2 variation in response function with position of load: influence line(影响线) 4 6.2 continued
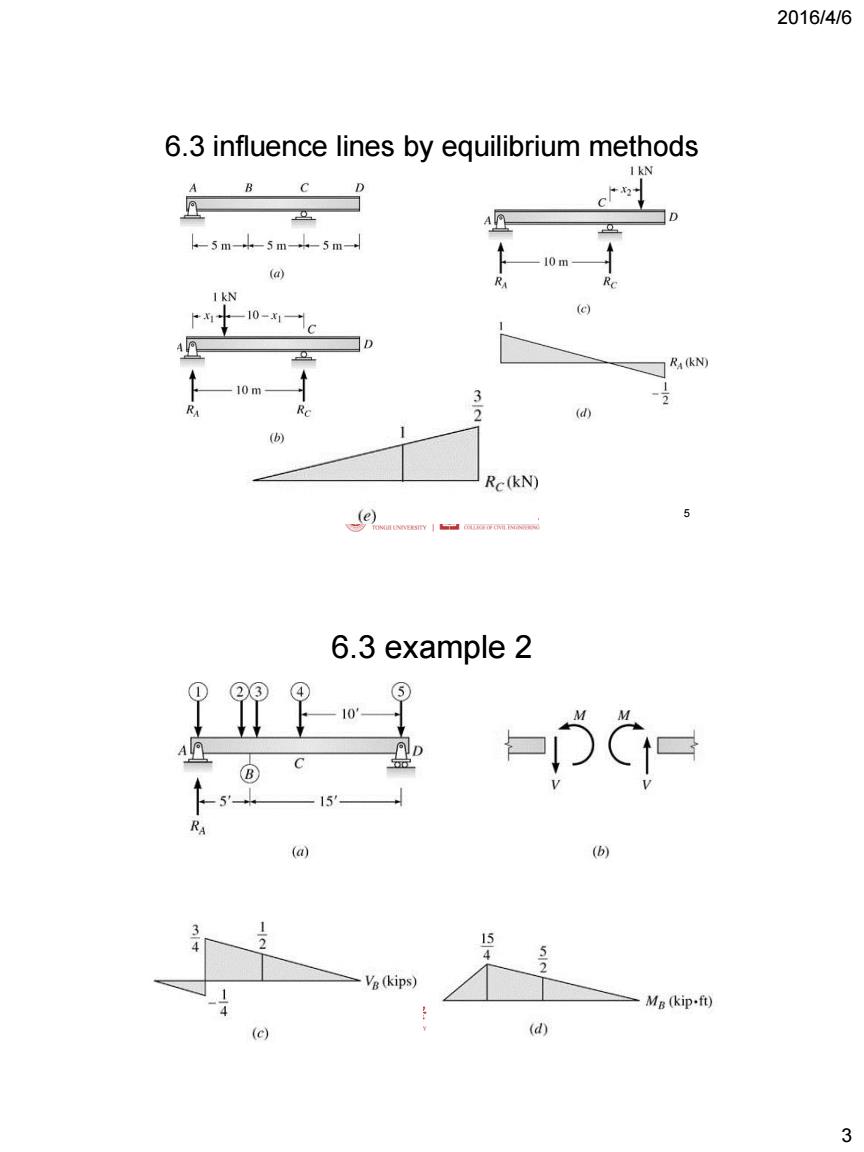
2016/4/6 6.3 influence lines by equilibrium methods kN c 5m-45m-5m- 10m (a) R Rc 1kN P-10-1一 (c) D RA(kN) -10m 3 R R 2 (d) (b) Rc(kN) 5 6.3 example 2 ②③ (4 10' =的= 5 15 RA (a) (b) 2 15 5 Ve (kips) M (kip-ft) (c) (d) 3
2016/4/6 3 5 6.3 influence lines by equilibrium methods 6 6.3 example 2
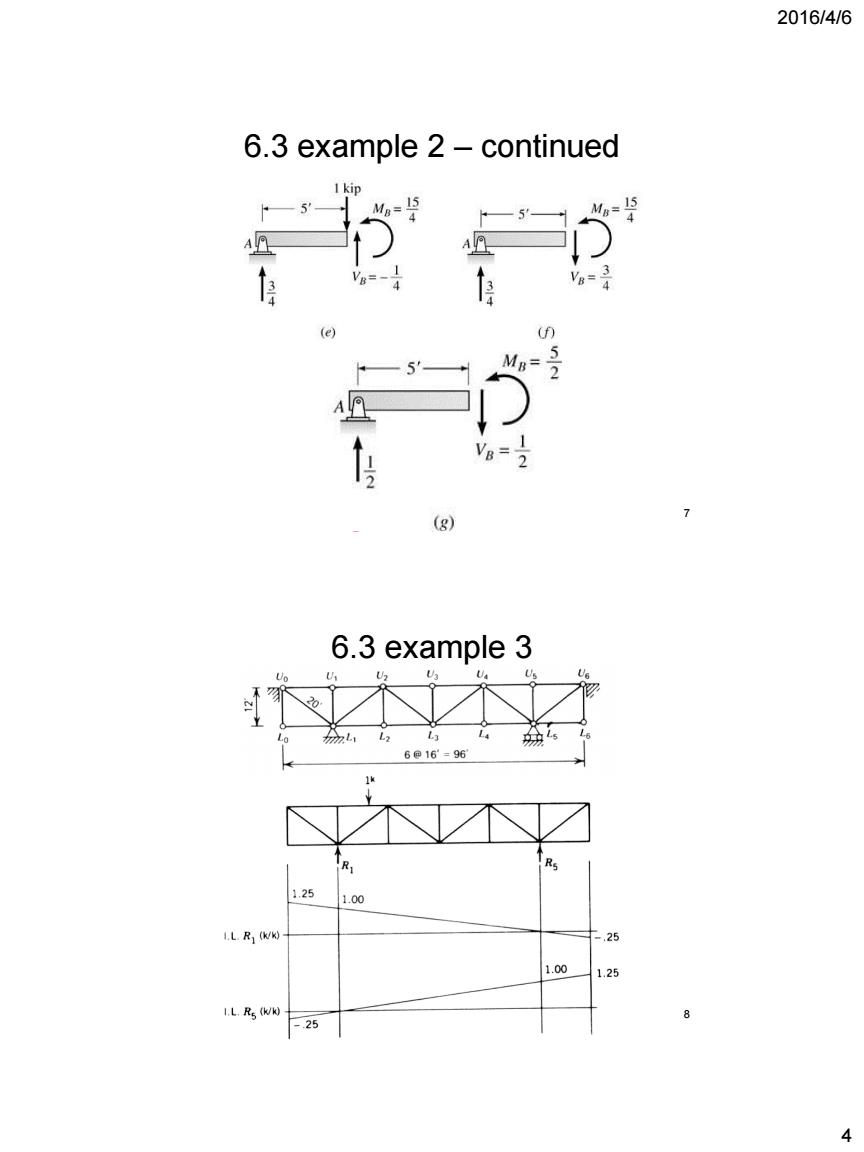
2016/4/6 6.3 example 2-continued I kip 卜5 MB= MB= A (e) (D 5 MB= 5-2 A a= (g) 7 6.3 example 3 U U3 L4 6@16°=96 R 1.25 1.00 1.L.R (k/K) -.25 1.00 1.25 1.L.Rs (k/k) 25
2016/4/6 4 7 6.3 example 2 – continued 8 6.3 example 3
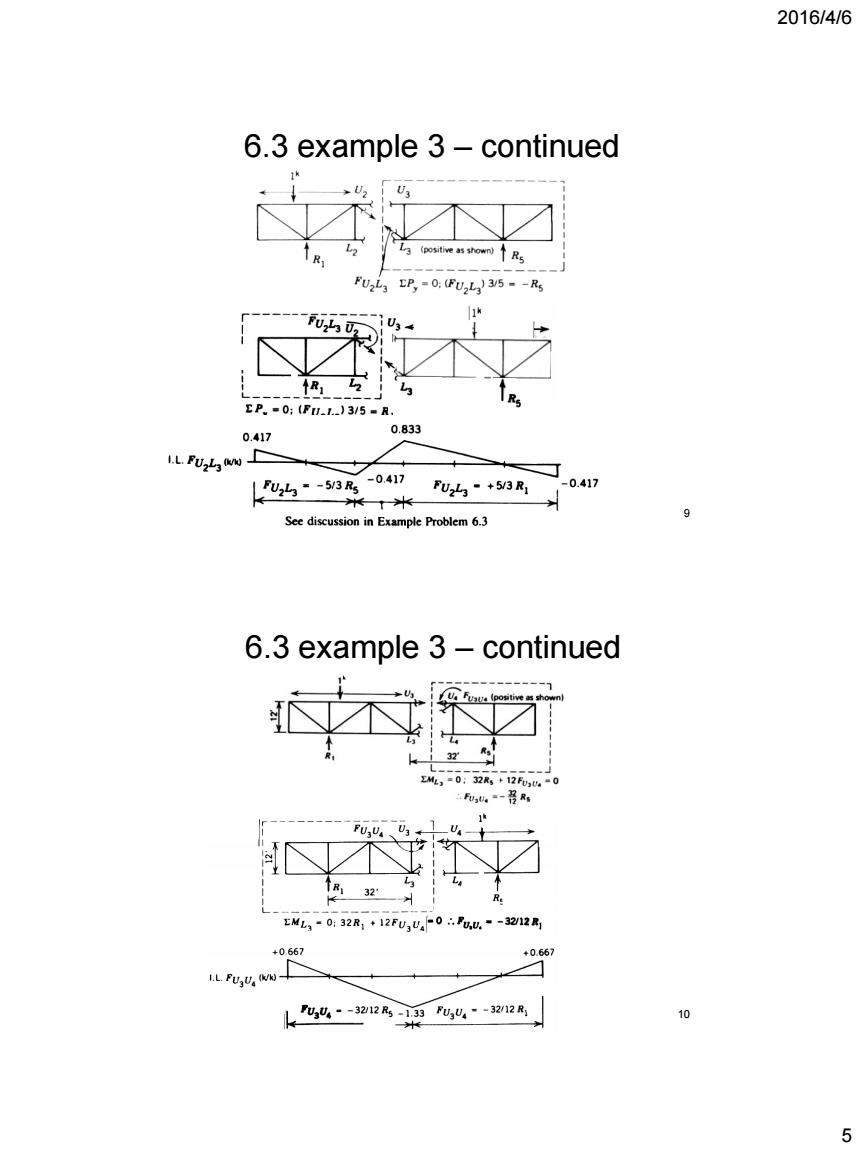
2016/4/6 6.3 example 3-continued 14 2 R L3 (positive as shown)Rs PU23cR,=0:FU235--R5 1k U202 EP.=0:(F.1.)3/5=R. 0.833 0.417 1.L.FU2Lg (w LV243·-53R -0.417 FU243·+53R1 -0.417 米十米 See discussion in Example Problem 6.3 6.3 example 3-continued 32 2M,=0:32R,+12,w ,,=-R 1 U TR132 EML30:32R1+12FU3Ua0,u."-3212 +0.667 +0.667 I.L.FUgUs (WN ku-3212R9u,u-212R 10 6
2016/4/6 5 9 6.3 example 3 – continued 10 6.3 example 3 – continued
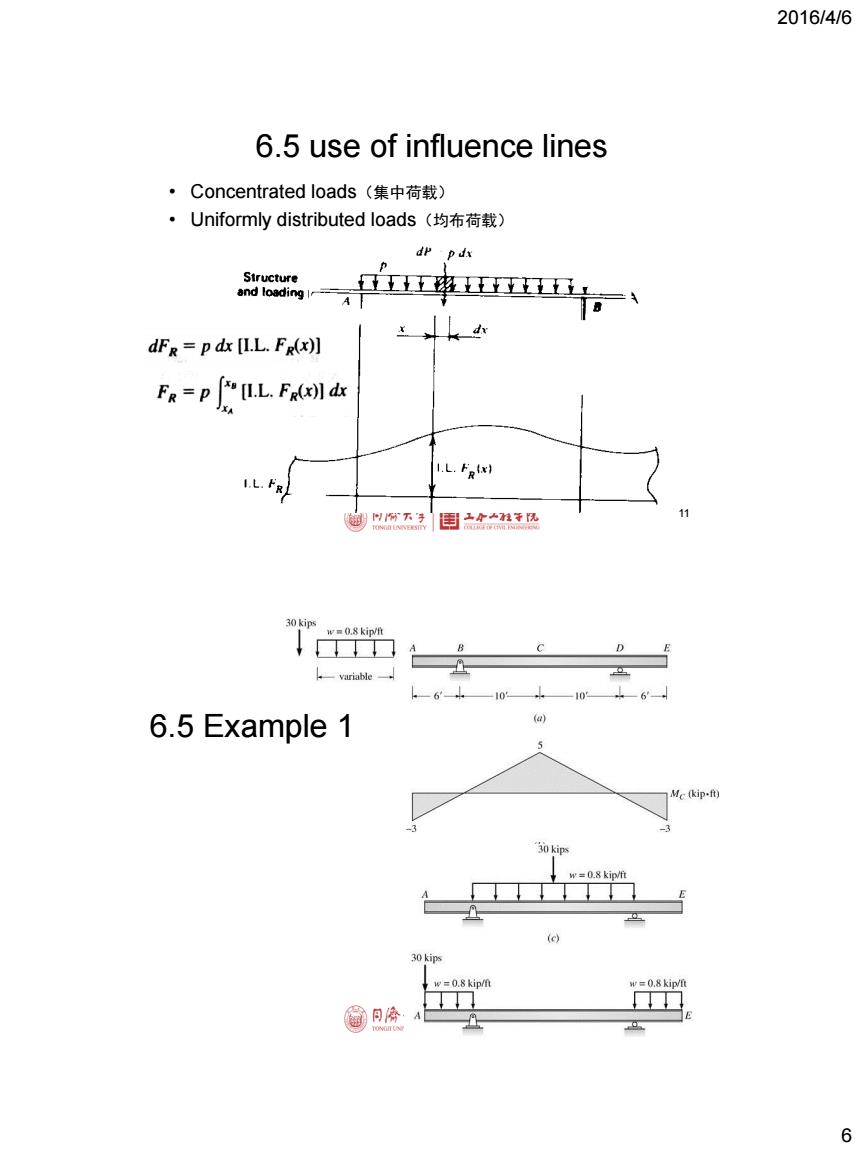
2016/4/6 6.5 use of influence lines ·Concentrated loads(集中荷载) ·Uniformly distributed loads(均布荷载) dp pdx Structure and loading 王上上11 A dFg=p dx [I.L.FR(x)] F-pF()ds 1.L.Fglx) L.L.FR 遍以阿五子 国二今柱年 15 30 kips w=0.8 kip/ft D variable 6 10 10 6 6.5 Example 1 (a) Mc (kip-ft) 30 kips w=0.8 kip/ft (c 30 kips w =0.8 kip/ft w=0.8 kip/ft 口 @月冷 6
2016/4/6 6 11 6.5 use of influence lines • Concentrated loads(集中荷载) • Uniformly distributed loads(均布荷载) 12 6.5 Example 1
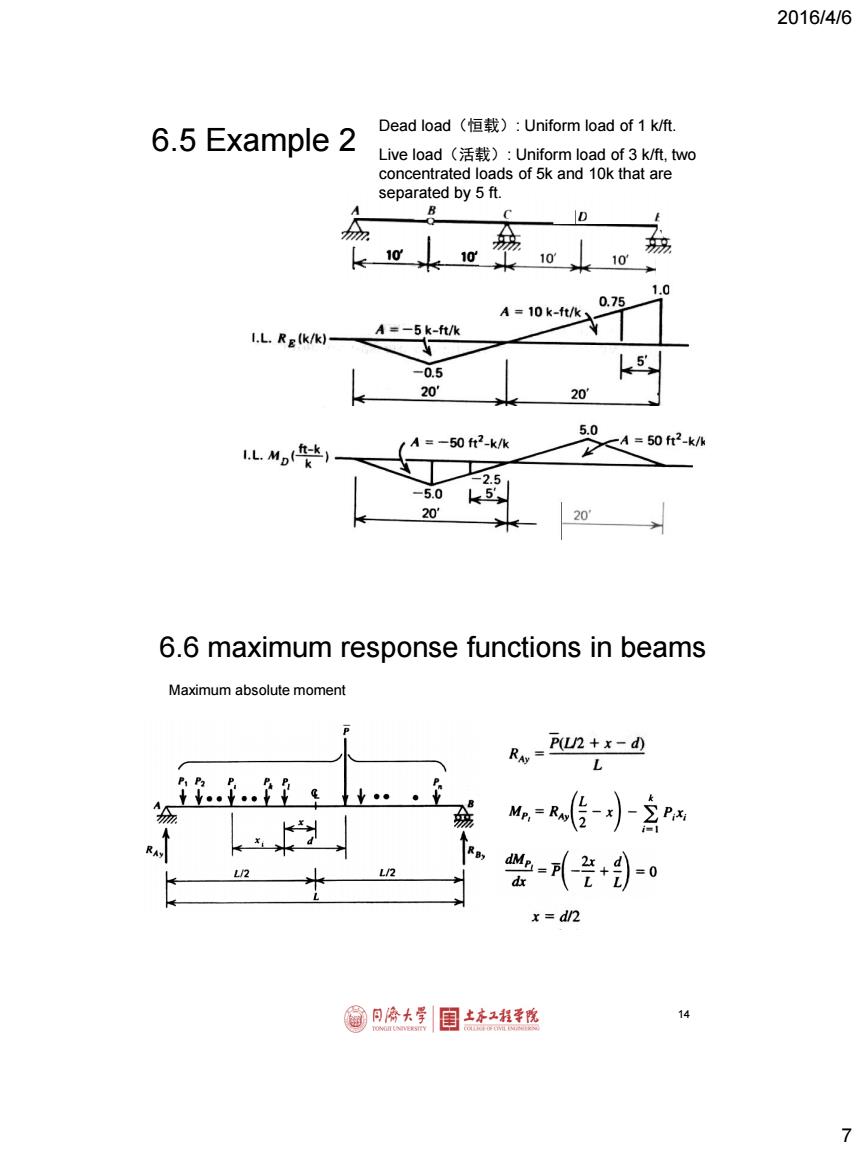
2016/4/6 Dead load(恒载):Uniform load of1k/t. 6.5 Example 2 Live load(话载):Uniform load of3k/t,two concentrated loads of 5k and 10k that are separated by 5 ft. B 【k101 10 10 1.0 0.75 A 10 k-ft/k I.L.Rg(k/k)- A=-5k-ft/k .5 -0.5 20 20 A=-50t2-k/ 5.0 A =50 ft2-k/k L.() -2.5 5.0 5 20 20 6.6 maximum response functions in beams Maximum absolute moment PUn+x-山 M=作-含两 Ra, L/2 尝-产+引-。 x=d/2 包同冷大学 目土本2相幸院 14 >
2016/4/6 7 13 6.5 Example 2 Dead load(恒载): Uniform load of 1 k/ft. Live load(活载): Uniform load of 3 k/ft, two concentrated loads of 5k and 10k that are separated by 5 ft. 14 6.6 maximum response functions in beams Maximum absolute moment
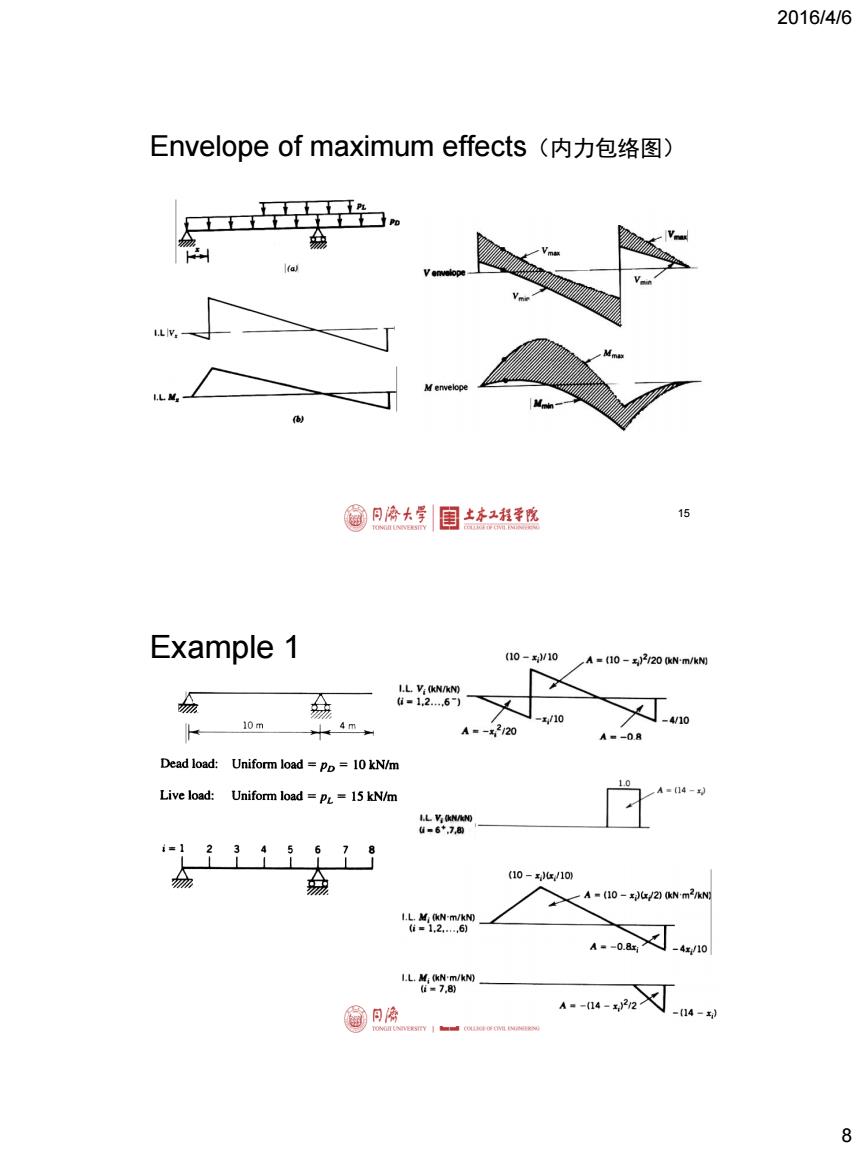
2016/4/6 Envelope of maximum effects(内力包络图) 输同海大学 15 Example 1 10-/10 A=10-2200Nm/k0 I.L.V:(kN/kN) 城 动 4=12..6 10m x/10 H 4m -410 A=-黑2120 A■-0.8 Dead load:Uniform load=Pp=10 kN/m 10 Live load:Uniform load=p =15 kN/m L.L Vi (kN/kN) 0=6*,1,0 =1 6 泳 (10-10 -A=(10-G/2kNm2N 1.L.M:(kN-m/kN) i=12.…,6) -4x/10 1.L.M:(kN'm/kN) i=78 A-14-2X -14- 8
2016/4/6 8 15 Envelope of maximum effects(内力包络图) 16 Example 1
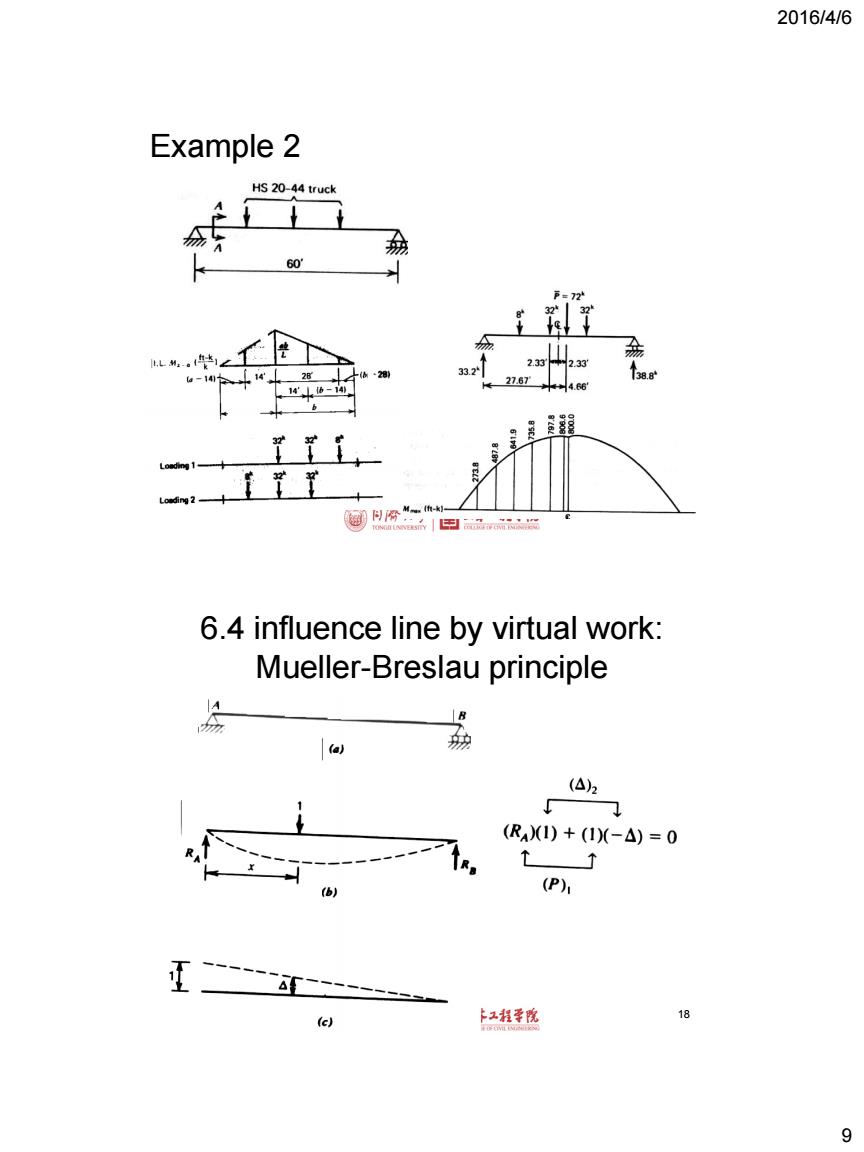
2016/4/6 Example 2 HS 20-44 truck 60 72 33.2 a-141 28 ·2 27.67 388 @“二回 6.4 influence line by virtual work: Mueller-Breslau principle 城 a (△)2 (R41)+(1(-△)=0 (P)1 (c) 上工程学院 分 9
2016/4/6 9 17 Example 2 18 6.4 influence line by virtual work: Mueller-Breslau principle
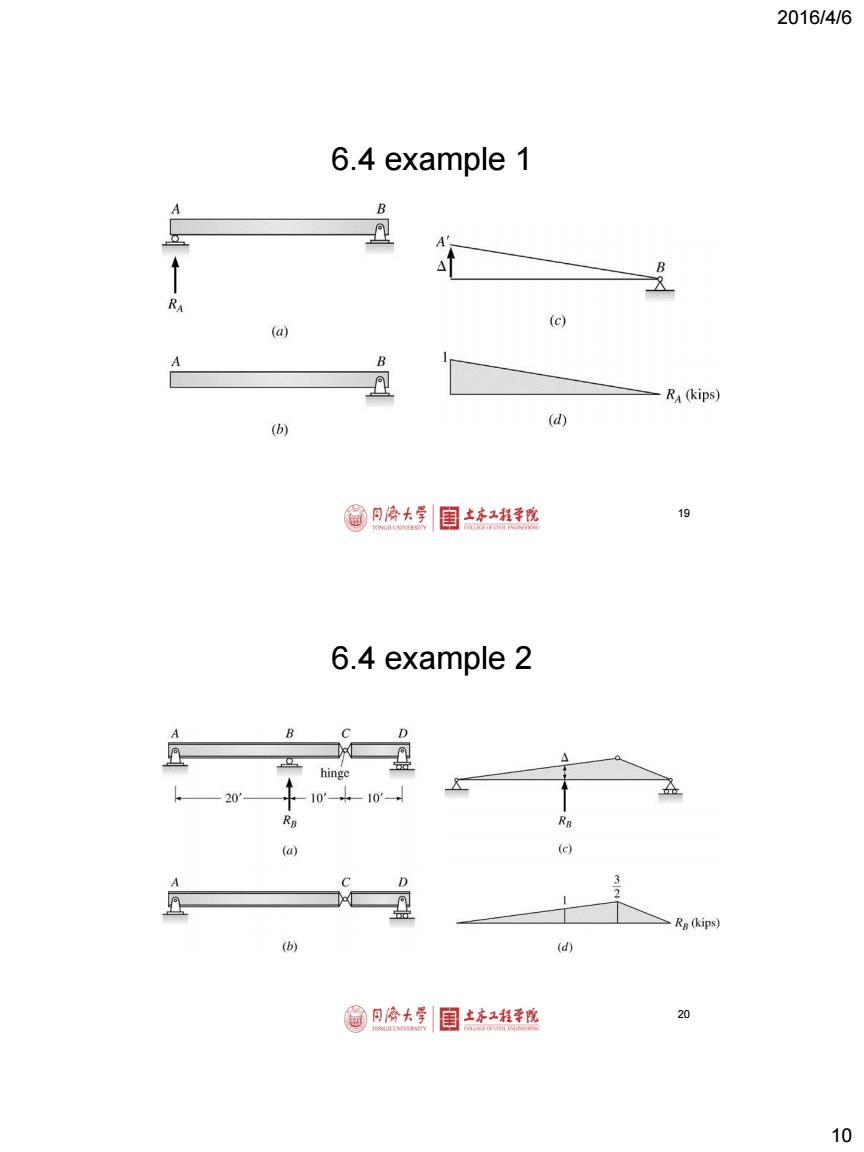
2016/4/6 6.4 example 1 B B RA (c) (a) A Ra (kips) (b) (d) 输月冷大学 目土本2程学税 19 6.4 example 2 A B C D hinge 20 -10'--10+ Rn (a) (c) Rg(kips) (b) (d) o 同降大学 目土本红相学院 20 10
2016/4/6 10 19 6.4 example 1 20 6.4 example 2