
Chapter 13 Matrix Displacement Method(矩阵位移法) 月大学 土工程学院 11 TONGI UNIVERSITY COLLGE OF CVIENGINEERING 13.1 introduction Displacement method + Matrix + Computer 月济大学 土工程学院 2 TONGJI UNIVERSITY COVEGINRNG
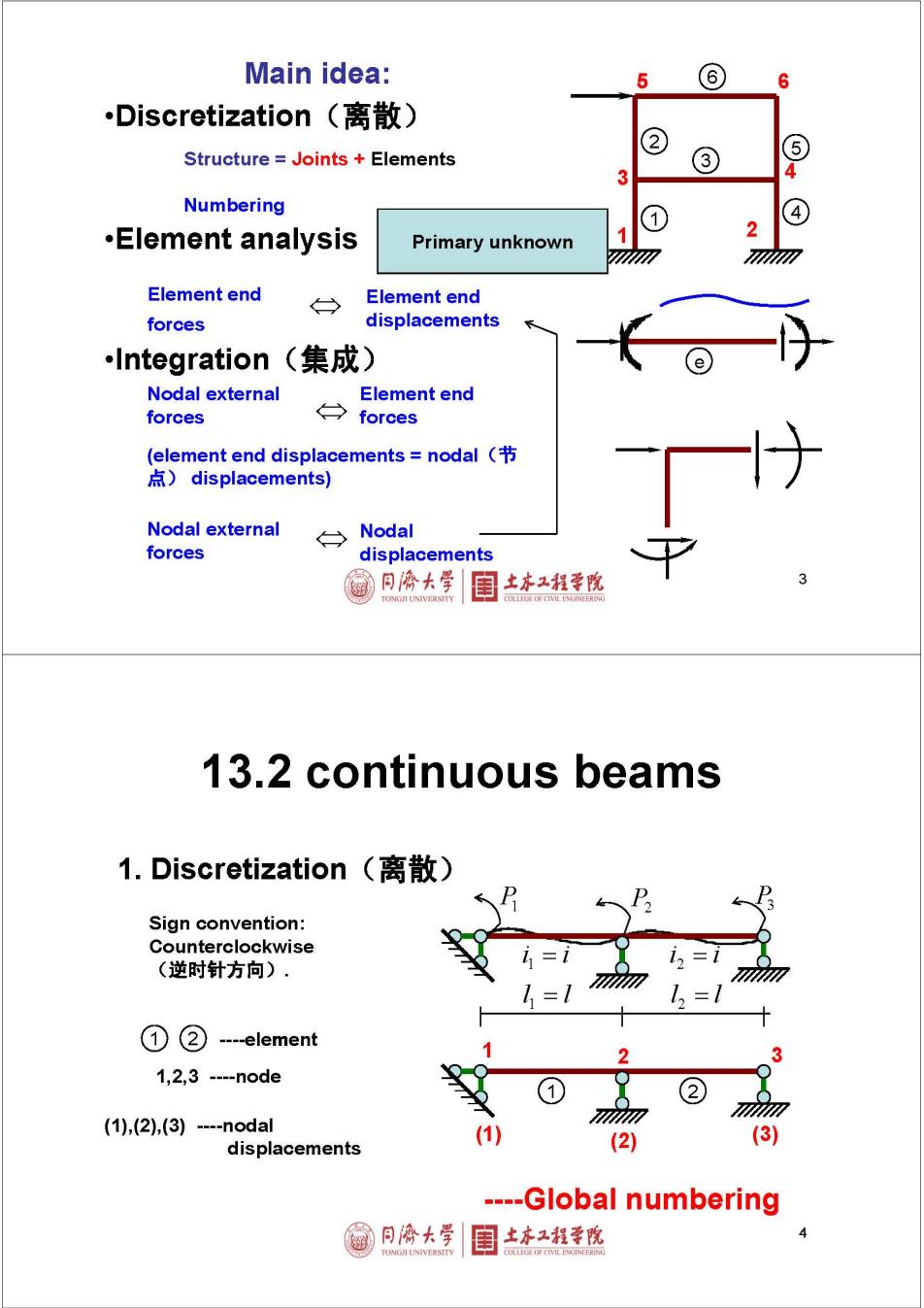
Main idea: 5 6 6 Discretization(离散) Structure Joints Elements 5 3 Numbering ·Element analysis Primary unknown Element end → Element end forces displacements .Integration (集成) Nodal external Element end forces → forces (element end displacements nodal 点)displacements) Nodal external Nodal forces displacements 同海大学 土本工程学院 TONGI UNIVERSITY 13.2 continuous beams 1.Discretization(离散) Sign convention: Counterclockwise i,= (逆时针方向), i= ,= ①② --element 2 1,2,3--node 2 (1),(2),(3)-nodal (3) displacements (2) ----Global numbering 同©大学 闺土本红程学院

2.Element analysis 1,2----local numbering FY= E --element end F forces (2 8 69 --element end (1) (2) (3) 8: displacements Sign convention: Counterclockwise (逆时针方向). 同©大学 土亦工程学院 Force-deformation relations E=4i.6+2i.δ9 41 2i, F=2i6+4i.6号 2i。41 62 Fy=k{δ --element stiffness equation [ --element stiffness matrix [- 始 「4i。2 2i K货 2i。41。 Symmetric (对称). 同像大学 土亦工程学院
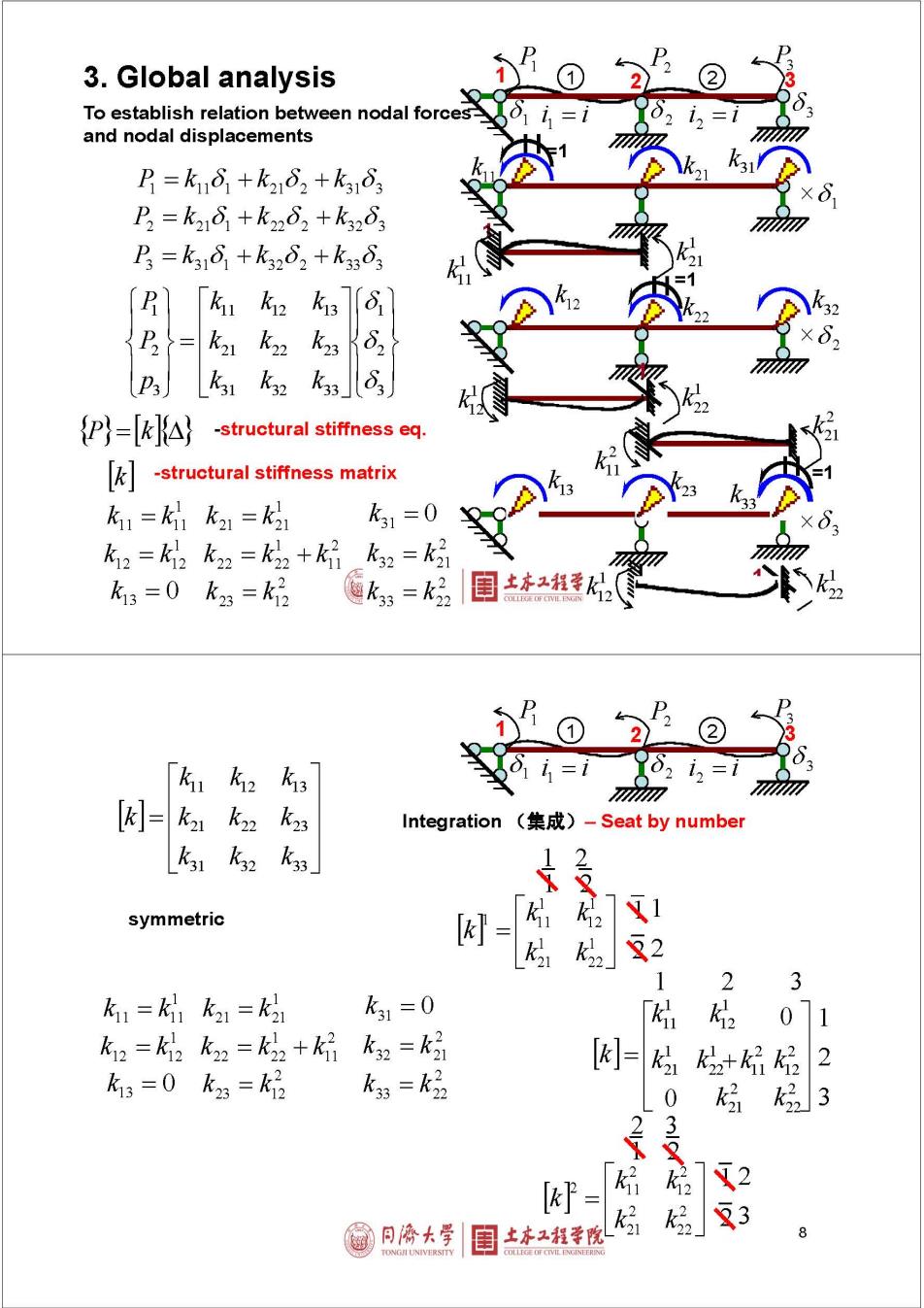
3.Global analysis To establish relation between nodal forces=i and nodal displacements B=k16+k162+k1⊙ D2=k216+k2n62+k263 =k318+k3262+k3⊙3 kat 芝31 3 飞31 P)=kIA -structural stiffness eq. [ -structural stiffness matrix k1=飞21=K21 k31=0 k2=2飞2=k32+k品k2=经 k:=0k3=k号 题k=k经 [ku δ21 =i kat k2 飞3 Integration (集成)-Seat by number ka k32 K3」 及冠 symmetric K KG」 14 2 3 k1=1k21=k21 k1=0 071 k2=2飞2=2+ k2=k号 [= k3=0 k3=品 k朗=k品 保品 2 3 [= 2 3 同降大学 土本工程学院
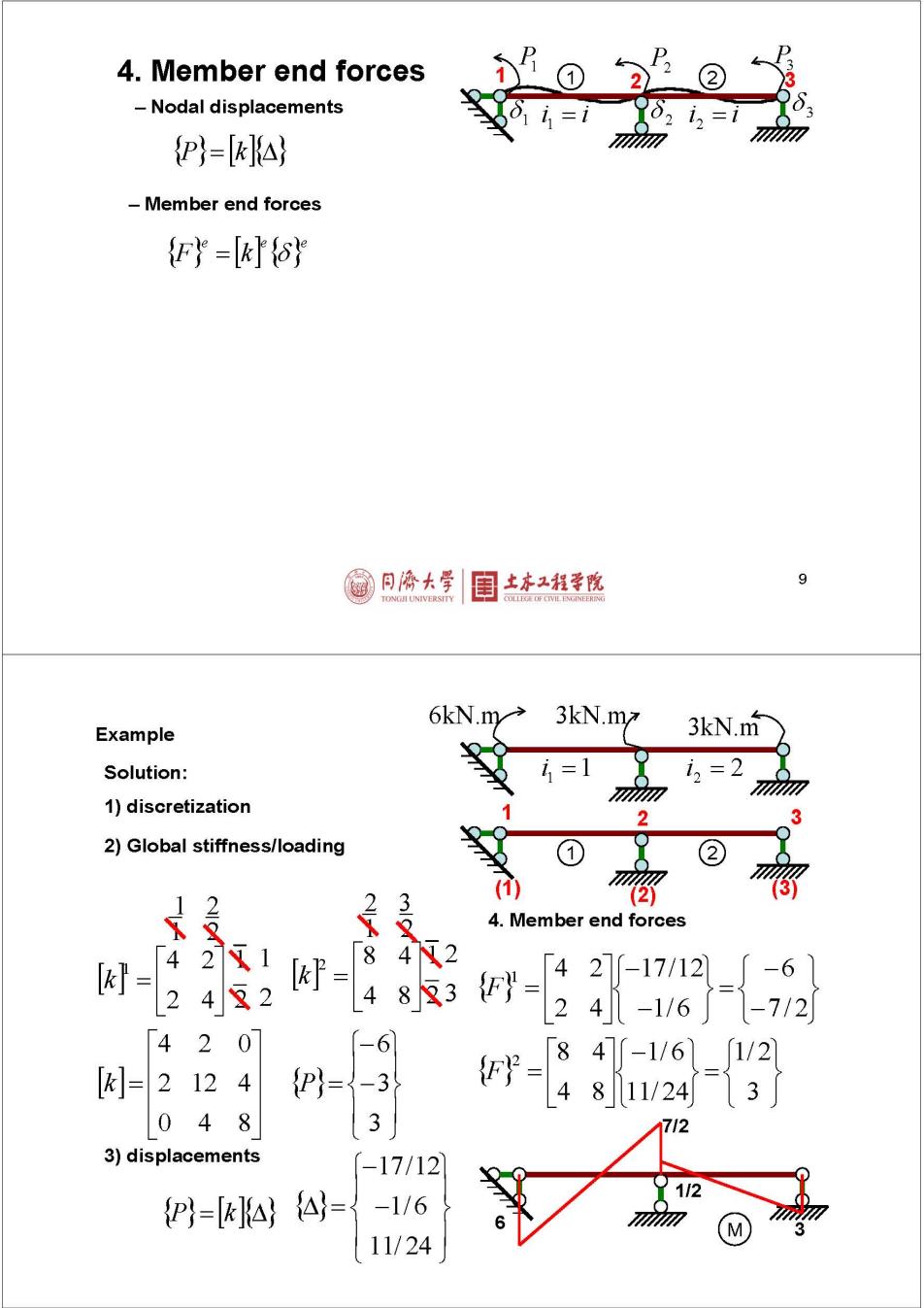
4.Member end forces -Nodal displacements P)=IklA) -Member end forces {Fy=[kδ 同源大学 土本工程学院 6kN.m> 3kN.m Example 3kN.m Solution: 1=1 i2=2 1)discretization 2 2)Global stiffness/loading ② 1 4.Member end forces = 42 1 「84N2 242 k9 区3 -%-{2 4-116j1-712 42 0 -6 [= 212 4 P)= 3 811/24 0 48 3 712 3)displacements -17/12 P)=kHA) A= -1/6 6 11/24
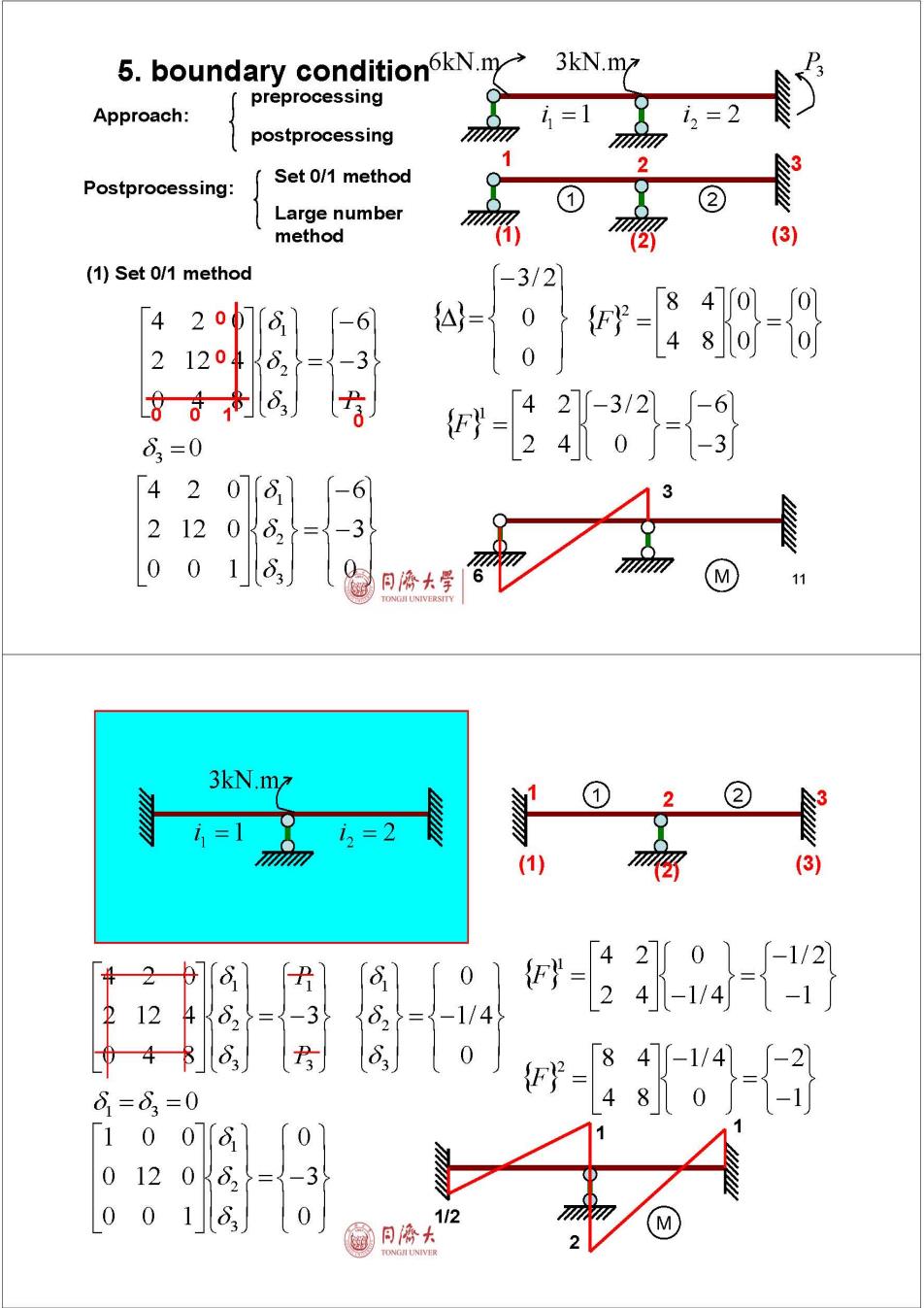
5.boundary conditionokN.m 3kN.mz preprocessing Approach: 1=1 i2=2 postprocessing 2 Postprocessing: Set 0/1 method A3 ② Large number method 2) (3) (1)Set 0/1 method [-3/2 4 200 -6 2 120 62 -3 63=0 -图 4 2 07 -6 2 12 0 62 -3 0 0 同源大学 6 TONGI UNIVERSITY 3kN.m 2 ② 3 1=1 3=2 (1) (3) 2 01 时-- 12 =-1/4 8 0 6=63=0 r-周 1 0 0 0 0 12 0 82 3 0 0 1 0 12 同源 M TONGJI UNIVER

6kN.my→ 3kN.mz (2)Large number method 1=1 i2=2 2 3 ② 4 2 3/0y -6 (2) (3) 2 12 = -3 8 4 2 0 -6 0 4 12 4 δ2 3 83=0 04 8×N63 0x6+4×62+8×N×63=P 63=(P-0×8-4×62)8×N) 8≈0 同僑大学 土本工程学院 13 6.in-span loading (I)Equivalent nodal loads(等效节点荷载) PE2 PE3 Rule:Keep the nodal displacements unchanged. 63 同僑大学 土本工程学院
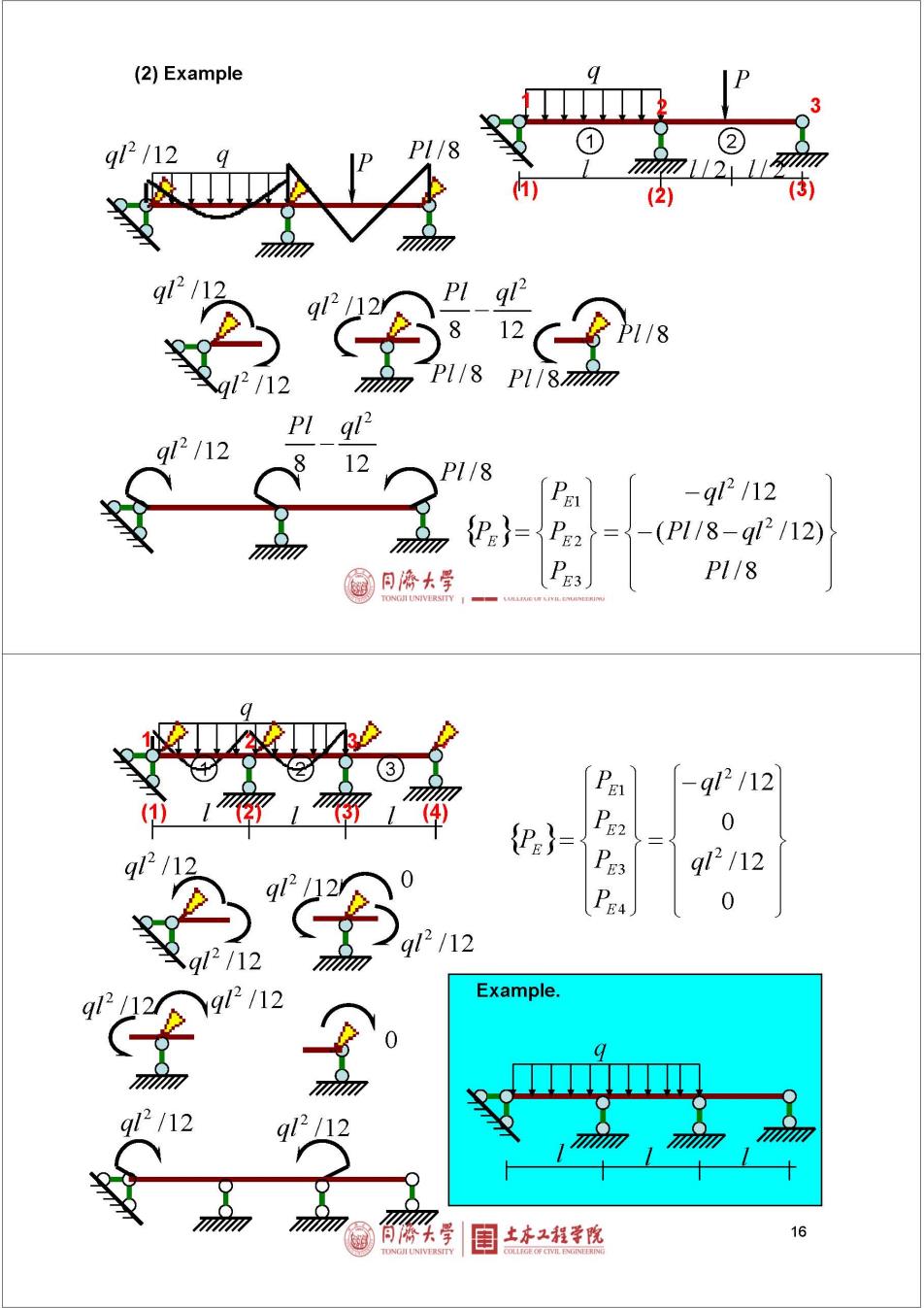
(2)Example gl2/12 ② (2) 9l2 12 P1/8 Pl18mm Pl P1/8 -gl2/12 {P}= (P1/8-ql2112) 同海大学 P1/8 2 gl2112 0 9l2 9l2/12 0 /12 N9l2/12 Example. 9/ 徐大学 土本2程学院 16
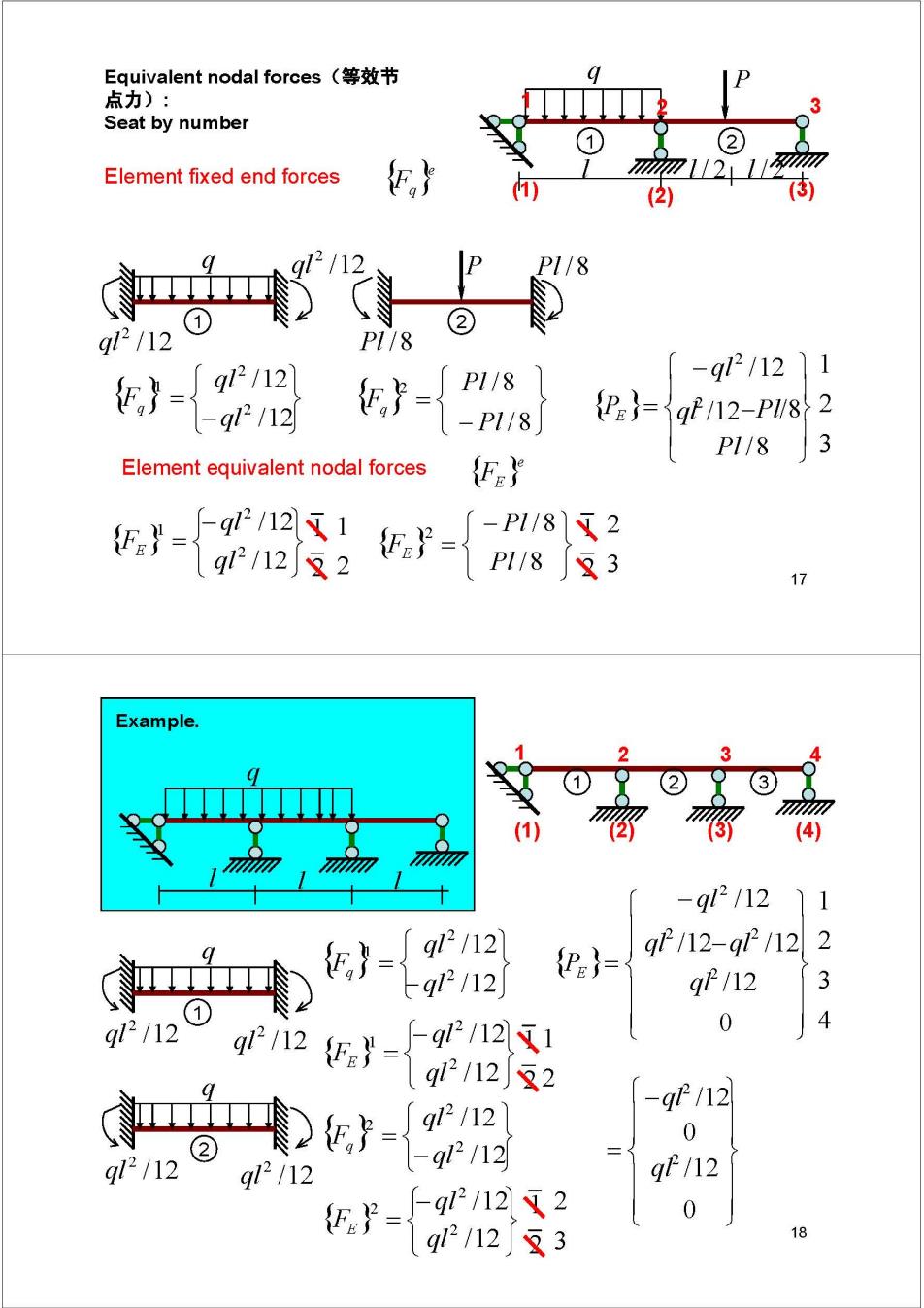
Equivalent nodal forces(等效节 点力): Seat by number Element fixed end forces (2) 2/12 P1/8 ② ql2/12 P1/8 3-图 -wg -q2/1211 }=g12-Pm82 P1/8 3 Element equivalent nodal forces Fay -{g-及 3j3 17 Example. (1) (41 -q2/12)1 g/12-g1122 q/12 3 4 gl2/12 q2112 - -{ -g/12 0 l2/12 gl2/12 =122 q112 0 q2/123 18
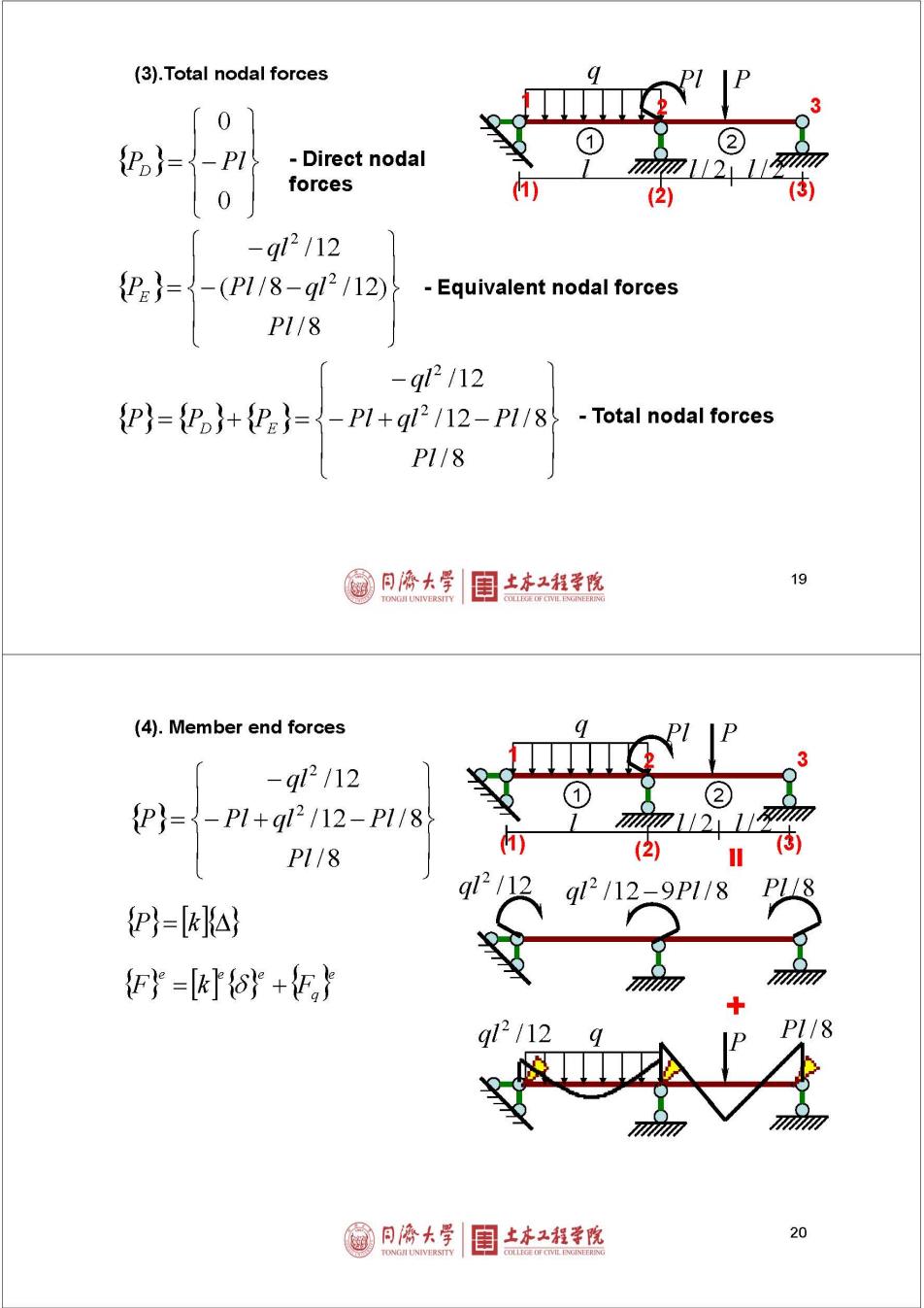
(3).Total nodal forces 0 }=-P Direct nodal forces (2 -g12112 Equivalent nodal forces -ql2/12 P}={+P=-Pm+g212-P1/8 -Total nodal forces P1/8 同源大学 土本工程学院 9 (4).Member end forces 3 -q2112 1 ② P}-=-1+12-Pm/8 P1/8 而 (2) I ql2/12 gl2/12-9P1/8 P118 P}=[k} F=8+E, gl2/12 P1/8 月傍大学 目 土亦工程学院 20