Structural Dynamics Lecture 8 Generalized SDOF Systems 闺 同桥大学 土本之程手院
Structural Dynamics Lecture 8 Generalized SDOF Systems
Contents General comments on generalized SDOF Generalized properties:assemblages of rigid bodies Generalized properties:distributed flexibility Vibration analysis by Rayleigh's method Selection of the Rayleigh's vibration shape 目 土本品程学院
Contents General comments on generalized SDOF Generalized properties: assemblages of rigid bodies Generalized properties: distributed flexibility Vibration analysis by Rayleigh’s method Selection of the Rayleigh’s vibration shape
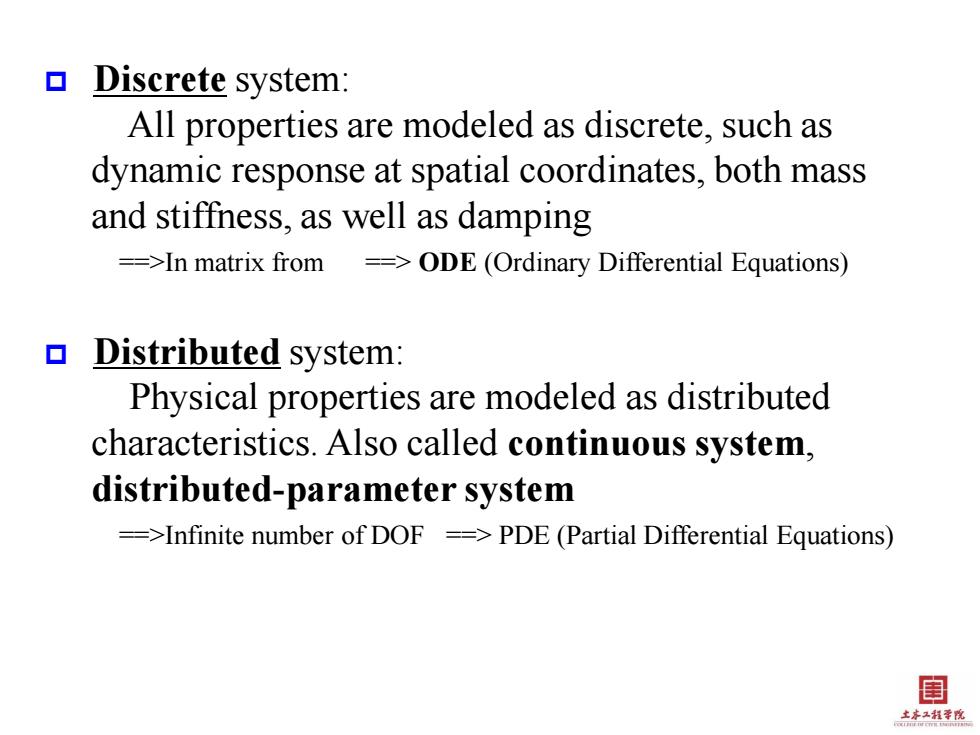
Discrete system: All properties are modeled as discrete,such as dynamic response at spatial coordinates,both mass and stiffness,as well as damping ==>In matrix from =>ODE (Ordinary Differential Equations) Distributed system: Physical properties are modeled as distributed characteristics.Also called continuous system, distributed-parameter system =>Infinite number of DOF ==>PDE(Partial Differential Equations) 目 土亦工鞋季悦
Discrete system: All properties are modeled as discrete, such as dynamic response at spatial coordinates, both mass and stiffness, as well as damping ==>In matrix from ==> ODE (Ordinary Differential Equations) Distributed system: Physical properties are modeled as distributed characteristics. Also called continuous system, distributed-parameter system ==>Infinite number of DOF ==> PDE (Partial Differential Equations)

Some complex system can be studied as SDOF systems,either exactly or under some simplifying assumption 1.SDOF rigid body assemblages. Principle of Virtual Displacements the D'Alembert Principle 2.Simple structural systems.(in an approximate manner) Assume a fixed pattern of displacement,whose amplitude(the single degree of freedom)varies with time. 目 土本2程季院
Some complex system can be studied as SDOF systems, either exactly or under some simplifying assumption 1. SDOF rigid body assemblages. Principle of Virtual Displacements the D’Alembert Principle 2. Simple structural systems. (in an approximate manner) Assume a fixed pattern of displacement, whose amplitude (the single degree of freedom) varies with time
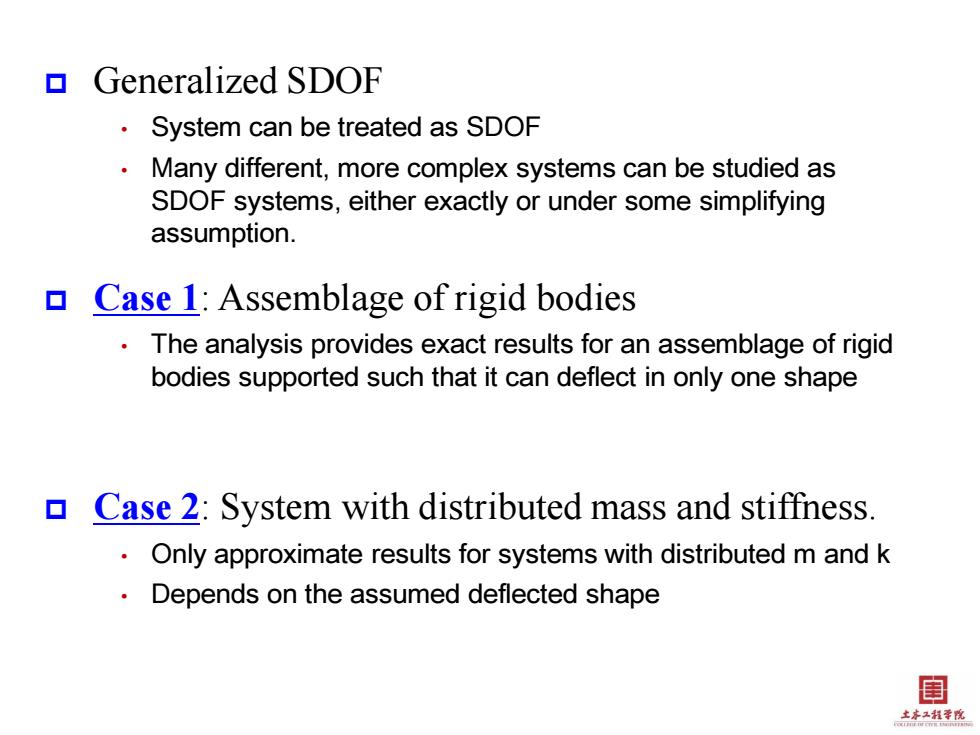
Generalized SDOF System can be treated as SDOF Many different,more complex systems can be studied as SDOF systems,either exactly or under some simplifying assumption. Case 1:Assemblage of rigid bodies The analysis provides exact results for an assemblage of rigid bodies supported such that it can deflect in only one shape Case 2:System with distributed mass and stiffness. Only approximate results for systems with distributed m and k Depends on the assumed deflected shape 闺 土本程李悦
Generalized SDOF • System can be treated as SDOF • Many different, more complex systems can be studied as SDOF systems, either exactly or under some simplifying assumption. Case 1: Assemblage of rigid bodies • The analysis provides exact results for an assemblage of rigid bodies supported such that it can deflect in only one shape Case 2: System with distributed mass and stiffness. • Only approximate results for systems with distributed m and k • Depends on the assumed deflected shape

The variable-separating method: A method that can be applied to linear partial differential equations, especially those with constant coefficients in the equation Assume the displacement can be expressed as a product of a function of x and a function of t u(x,t)=v(x)z(t) Change PDE to ODE The above expression is an assumption! 目 土茅2粗季院
The variable-separating method: • A method that can be applied to linear partial differential equations, especially those with constant coefficients in the equation • Assume the displacement can be expressed as a product of a function of x and a function of t • Change PDE to ODE • The above expression is an assumption! u x t , xzt
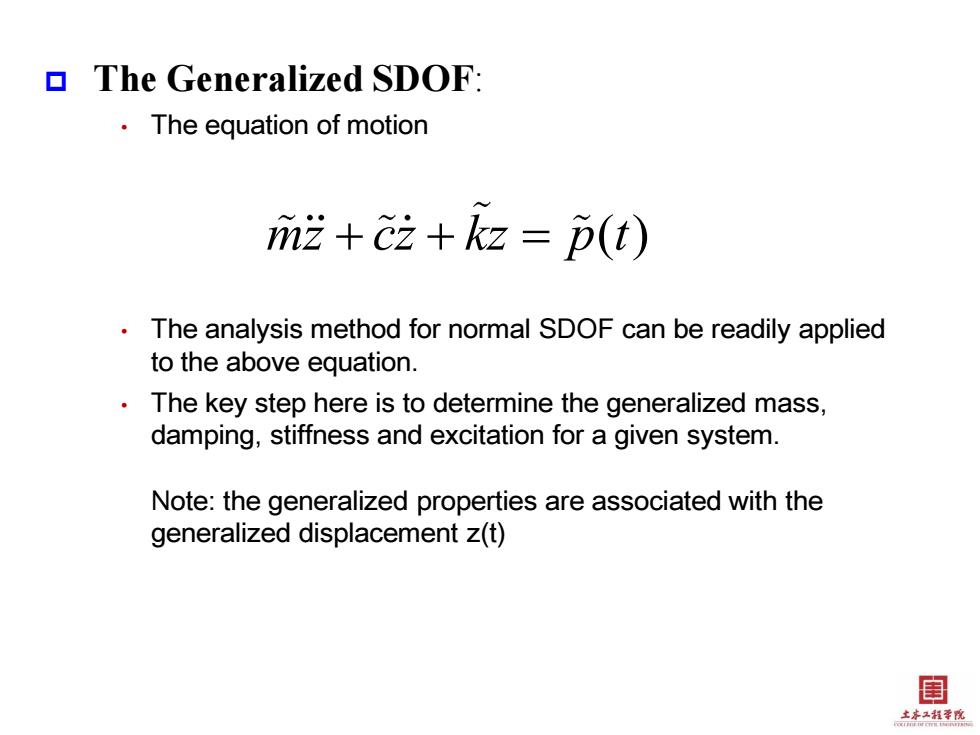
The Generalized SDOF: The equation of motion mz++kz p(t) The analysis method for normal SDOF can be readily applied to the above equation. The key step here is to determine the generalized mass, damping,stiffness and excitation for a given system. Note:the generalized properties are associated with the generalized displacement z(t) 闺 土本程李悦
The Generalized SDOF: • The equation of motion • The analysis method for normal SDOF can be readily applied to the above equation. • The key step here is to determine the generalized mass, damping, stiffness and excitation for a given system. Note: the generalized properties are associated with the generalized displacement z(t) mz cz kz p t ( )
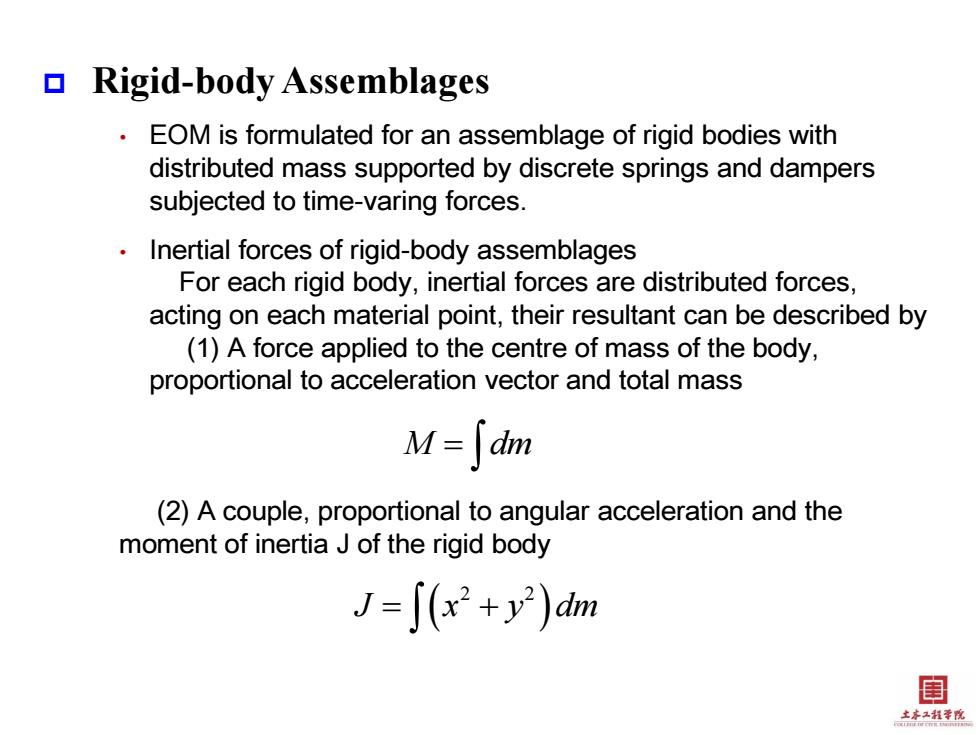
Rigid-body Assemblages EOM is formulated for an assemblage of rigid bodies with distributed mass supported by discrete springs and dampers subjected to time-varing forces. Inertial forces of rigid-body assemblages For each rigid body,inertial forces are distributed forces, acting on each material point,their resultant can be described by (1)A force applied to the centre of mass of the body, proportional to acceleration vector and total mass M=∫dm (2)A couple,proportional to angular acceleration and the moment of inertia J of the rigid body J=∫(x2+y2)dm 目 主京2相季院
Rigid-body Assemblages • EOM is formulated for an assemblage of rigid bodies with distributed mass supported by discrete springs and dampers subjected to time-varing forces. • Inertial forces of rigid-body assemblages For each rigid body, inertial forces are distributed forces, acting on each material point, their resultant can be described by (1) A force applied to the centre of mass of the body, proportional to acceleration vector and total mass (2) A couple, proportional to angular acceleration and the moment of inertia J of the rigid body M dm 2 2 J x y dm

Properties of typical rigid body:rigid bar Unit mass m=constant, Length L, Centre of Mass xG L/2, Total Mass m mL, L2 L3 Moment of Inertia J=12 12 目 土本程李悦
Properties of typical rigid body: rigid bar

Properties of typical rigid body:rigid bar Unit mass m=constant, Length L, Centre of Mass xG L/2, Total Mass m =mL, L2 Moment of Inertia J=m12 =m 12 目 土水2程学院
Properties of typical rigid body: rigid bar