
2014/5/12 Contents Structural Dynamics (earthquake excitation) Lecture2: Free vibration of SDOF Effect of gravity in EOM For this reason we usually formulate a dynami adding the corresponding static quantities tothe be obtaind by the ↓P0 ime-varyi Motion due to carthquake excitation Lincar ys.Non-linea Elastie vs.Inelastic system and e m+)++K=可 ++a-@0=- 1
2014/5/12 1 Structural Dynamics Lecture 2: Structural Dynamics: Lecture 2 1 Contents Effect of gravity force in derivation of equation of motion Equation of motion due to ground motion (earthquake excitation) Free vibration of SDOF • Solutions • Dynamic properties • Damping model Structural Dynamics: Lecture 2 2 Structural Dynamics: Lecture 2 3 Effect of gravity in EOM (a)Undeformed state (b)Static equilibrium state (c)Dynamic deformed state For this reason we usually formulate a dynamic analysis problem for a linear system with its static equilibrium position as the reference position. The total displacements and forces are obtained by adding the corresponding static quantities to the dynamic response The total response can be obtained by the Superposition Principle: • Static analysis due to dead and live load… • Dynamic analysis due to time-varying load Structural Dynamics: Lecture 2 4 Static forces (eg. gravity force) disappear from the equation of motion, if • The motion are measured from the static equilibrium state. • The system is linear. Non-linear system are usually measured from the uderformed state. Linear vs. Non-linear system & Elastic vs. Inelastic system • Linear: The relationship between the external force and the resulting deformation if linear. • Elastic: There has no unrevocerable deformation (permanent deformation) / the restoring force is a single-valued function of deformation (the loading and unloading curves are identical • Lineraly elastic systems Example: Inverted pendulum Structural Dynamics: Lecture 2 5 Structural Dynamics: Lecture 2 6 Motion due to earthquake excitation

2014/5/12 [BM]{x+[C]x+[]x)=-[M]A Free vibration of SDOF A structure is said to be undergoing free vibration x=[)间】 (0 Undamped /damped free vibration compare them: Free vibration of SDOF Undamped Free Vibration Equation of motion of SDOF system ing the vihration of the systen mii+cu+ku=f(1) mii+ku=0 he system will be disturbed by initial displacementu=(0) inial助r-i(o) REVIEW:Solution theory for non-homogeneous linear Solution of Free vibration of Undamped SDOF-Me differential eqation of second order 。em0n 少+pm+=f(t) ◆=A cos ot-+A,sinot y()=.()+y() #=w(0) initialvelociryi=ao) )=u()cos) u(1)=Acos(@t-B) “ 2
2014/5/12 2 Structural Dynamics: Lecture 2 7 Absolute vs. Relative responses x t 3 x t 2 x t 1 M X C X K X M I g x 1 2 3 , , T X x t x t x t Can we compare them? Free vibration of SDOF A structure is said to be undergoing free vibration when it is disturbed from its static equilibrium position and then allowed to vibrate without any external dynamic excitation. Undamped /damped free vibration Structural Dynamics: Lecture 2 8 Free vibration of SDOF Structural Dynamics: Lecture 2 9 • Equation of motion of SDOF system mu cu ku f t 0 0 0 f t freevibration c undamped free vibration c damped free vibration Structural Dynamics: Lecture 2 10 Undamped Free Vibration Problem statement differential equation governing the vibration of the system the system will be disturbed by mu ku 0 0 0 initial displacement initial velocity u u u u ( ) 2 2 f x dt d x Structural Dynamics: Lecture 2 11 REVIEW: Solution theory for non-homogeneous linear differential equations of second order y py qy f t y t y t c y t p yc (t) : Complementary solution, i.e. the complete solution to the homogeneous differential equation. (f(t) =0) transient vibration / eigenvibration yp (t) : Particular solution (due to f(t) steady-state vibration / stationary vibration 12 Solution of Free vibration of Undamped SDOF– Method I The general solution will have the form 1 2 u t A t A t ( ) cos sin mu ku 0 0 0 initial displacement initial velocity u u u u 0 u t t ( ) cos sin 0 u u t u t t ( ) cos A 2 2 0 0 0 arctan 0 u u A u u

2014/5/12 Solution of Free vibration of Undamped SDOF-Me The general (complementary)solution of the EOM will be mii+ku=0 u()=Aev+Ae"=+e-i A1.A2 are complex constants u(1)=Ae" Introduce Euler's pair(Euler relations),which are e=cosx+isinx e"=cosx-isinx (ms+k)Ae"=0 Notice the vibration mst be real. s2=-k1m=-o2 0=my+8m-ow时-合}my 线名a加m15后 4.Bare real-valued constants The velocity and acceleration will be u(t)=Acos创t+Bsinot i(t)=-0.Asino4+0Bsino! ii(t)=-0Acos01-0Bcoso Natural period of vibration 叫。-u(0)-A 72 i认。=a(0)=o,B The final solution is 人是 Natural cyclicfrequency of vibraion =2.225 3
2014/5/12 3 Structural Dynamics: Lecture 2 13 Solution of Free vibration of Undamped SDOF –Method II From mathematical theory, the solution to the above linear, homogeneous, second-order differential equation with constant coefficients has the following form A: constant s:unknown coefficient Bring the solution to the equation of motion leading to the characteristic equation One has two imaginary roots ( ) st u t Ae mu ku 0 2 0 st ms k Ae 2 2 / n s k m 1 2 1 n n n k s s w i i i here m Structural Dynamics: Lecture 2 14 The general (complementary) solution of the EOM will be A1, A2 are complex constants Introduce Euler’s pair (Euler relations), which are Notice the vibration must be real, A, B are real-valued constants 0 0 cos sin cos sin n n n n n u u t A t B t u t t 1 2 1 2 1 2 n n s t s t i t i t u t Ae A e Ae A e cos sin cos sin ix ix e x i x e x i x Structural Dynamics: Lecture 2 15 The velocity and acceleration will be Substitute the initial condition The final solution is 2 2 cos sin sin sin cos cos n n n n n n n n n n u t A t B t u t A t B t u t A t B t 0 0 0 0 t t n u u A u u B 0 0 cos sin cos sin n n n n n u u t A t B t u t t Structural Dynamics: Lecture 2 16 Important dynamic properties n k m Natural circular frequency of vibration 2 n n T Natural period of vibration 2 n n f Natural cyclic frequency of vibration Structural Dynamics: Lecture 2 17 F1=0.45Hz (T1=2.22s, H=100m) F1=0.16Hz (T1=6.25s, H=495m) F1=0.1475Hz, (T1=6.77s), L=1088m F1=0.1475Hz, (T1=6.77s), L=1377m Structural Dynamics: Lecture 2 18 Free vibration curve of undamped SDOF due to initial displacement and velocity
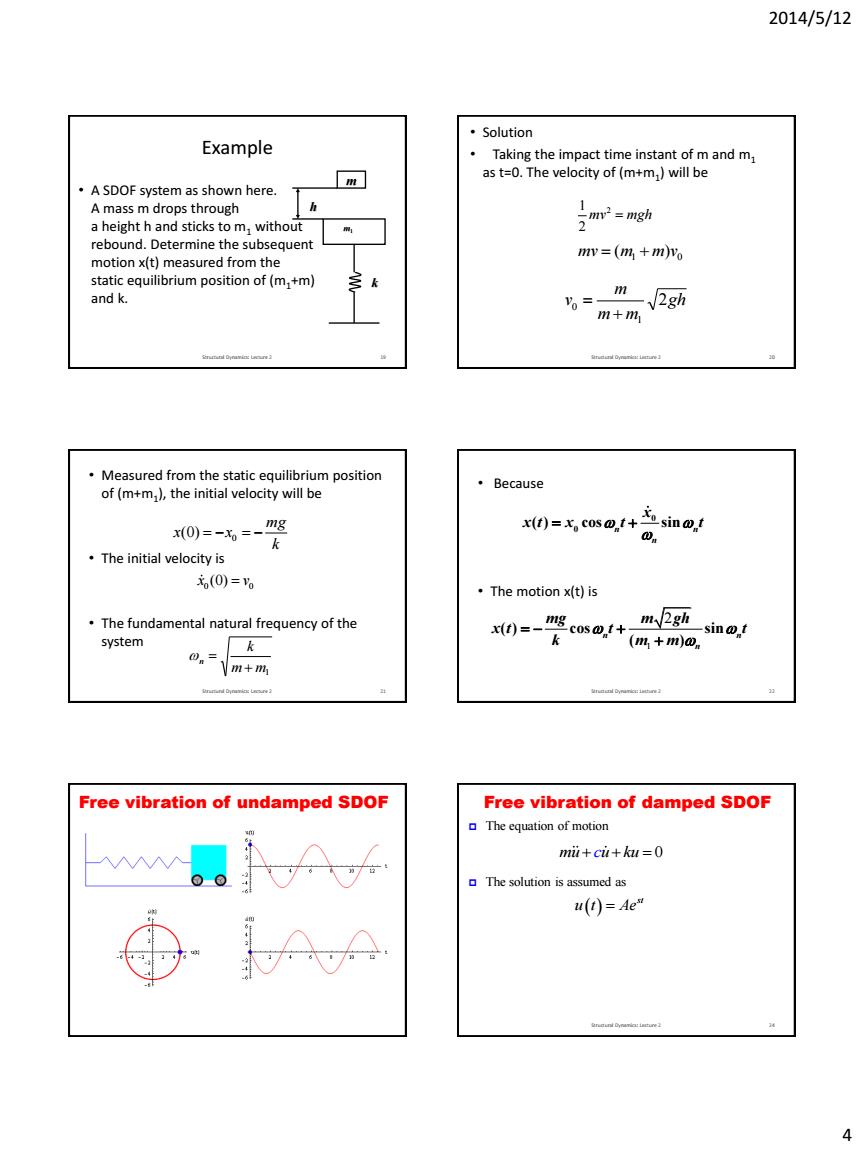
2014/5/12 Solution Example Taking the impact time instant of m and m m as t=0.The velocity of(m+m)will be A SDOF system as shown here. A mass m drops through rebound.Determine the subsequent motion x(t)measured from the w=(m,+m。 static equilibrium position of (m+m) and k. Because M0=%=-竖 0=戈m@+合如 .The initial velocity is (0)= The motion x(t)is .The fundamental natural frequency of the system k .m+m Free vibration of undamped SDOF Free vibration of damped SDOF The equation of motion ■A mit+cit+ku=0 The solution is assumed as u(t)=Ae" 4
2014/5/12 4 Example • A SDOF system as shown here. A mass m drops through a height h and sticks to m1 without rebound. Determine the subsequent motion x(t) measured from the static equilibrium position of (m1+m) and k. Structural Dynamics: Lecture 2 19 h m m1 k • Solution • Taking the impact time instant of m and m1 as t=0. The velocity of (m+m1 ) will be Structural Dynamics: Lecture 2 20 mv mgh 2 2 1 1 0 mv (m m)v gh m m m v 2 1 0 • Measured from the static equilibrium position of (m+m1 ), the initial velocity will be • The initial velocity is • The fundamental natural frequency of the system Structural Dynamics: Lecture 2 21 k mg x(0) x0 0 0 x v (0) m m1 k n • Because • The motion x(t) is Structural Dynamics: Lecture 2 22 n n n x x t x t t 0 0 ( ) cos sin 1 2 n n n mg m gh x t t t k m m ( ) cos sin ( ) Free vibration of undamped SDOF Structural Dynamics: Lecture 2 24 Free vibration of damped SDOF mu u k c u 0 The equation of motion The solution is assumed as st u t Ae
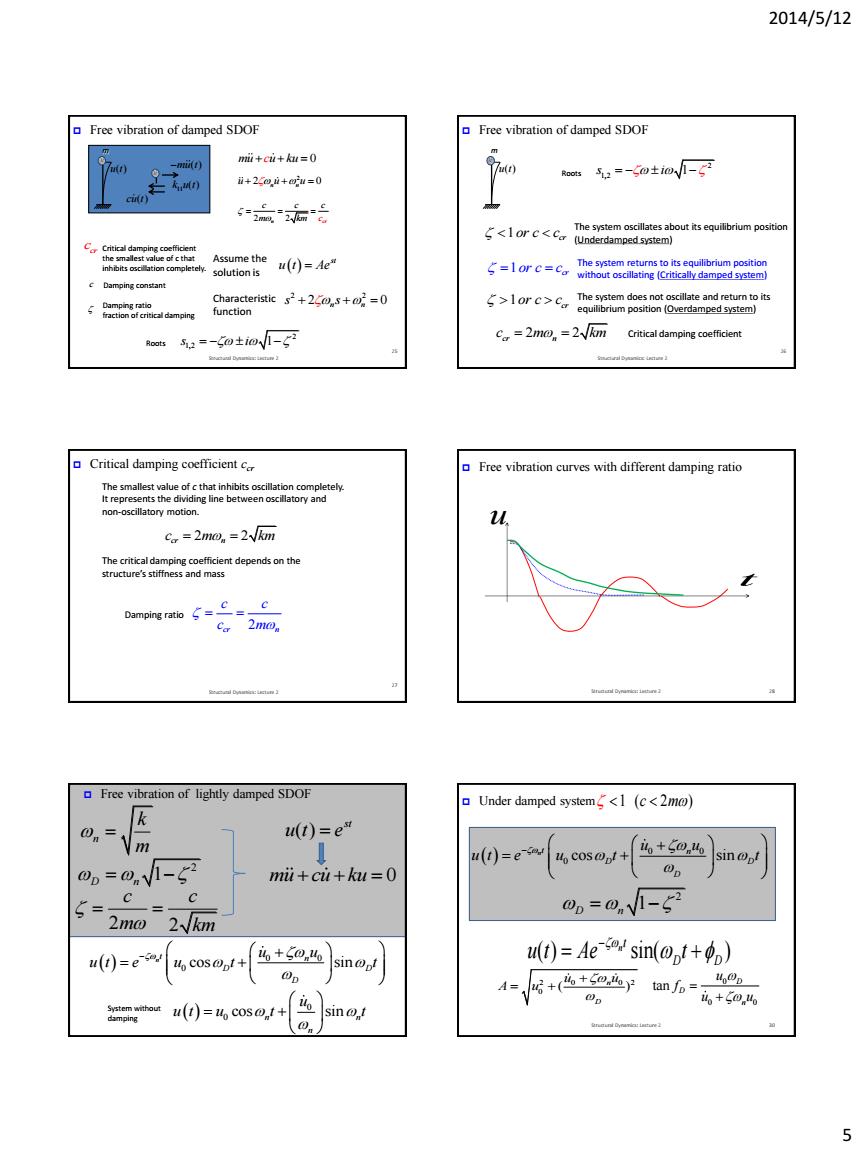
2014/5/12 Free vibration of damped SDOF Free vibration of damped SDOF m+ci+缸=0 i+2i+a-0 aa=-士i 5=1orc=c。2ecmamc5enbmecaoie Damping constant n+24+-0 oro ma=-士iofG =2m=2km Critical dampire coefficient Critical damping coefficientc Co =2m0,=2 km Free vibration of lightly damped SDOF Under damped system<(c<2m) u(t)=e" 0=0V1-2 mii+cu+ku=0 5-2mo 2km 0n=0,N1-57 l0=Aesin(o,l+4o)) 0-色} 4+229m6a 5
2014/5/12 5 Structural Dynamics: Lecture 2 25 m ut() mu t() 11 k u t() cu t() Free vibration of damped SDOF cr c Critical damping coefficient the smallest value of c that inhibits oscillation completely. Damping constant Damping ratio fraction of critical damping Assume the solution is Characteristic function mu u k c u 0 2 2 0 n n u u u 2 n 2 cr c c c m km c st u t Ae 2 2 2 0 n n s s 2 1,2 Roots s i 1 c Structural Dynamics: Lecture 2 26 m ut() Free vibration of damped SDOF 1 cr or c c The system oscillates about its equilibrium position (Underdamped system) The system returns to its equilibrium position without oscillating (Critically damped system) The system does not oscillate and return to its equilibrium position (Overdamped system) 2 2 cr n c m km Critical damping coefficient 1 cr or c c 1 cr or c c 2 1,2 Roots s i 1 Structural Dynamics: Lecture 2 27 Critical damping coefficient ccr The smallest value of c that inhibits oscillation completely. It represents the dividing line between oscillatory and non-oscillatory motion. The critical damping coefficient depends on the structure’s stiffness and mass Damping ratio 2 cr n c c c m 2 2 cr n c m km Structural Dynamics: Lecture 2 28 t u Free vibration curves with different damping ratio Free vibration of lightly damped SDOF mu cu ku 0 ( ) st u t e n k m 2 2 c c m km 0 0 0 cos sin n t n D D D u u u t e u t t 0 0 cos sin n n n u u t u t t 2 1 D n System without damping Structural Dynamics: Lecture 2 30 Under damped system 1 ( 2 ) c m 0 0 0 cos sin n t n D D D u u u t e u t t 2 1 D n ( ) sin( ) n t D D u t Ae t 2 2 0 0 0 ( ) n D u u A u 0 0 0 tan D D n u f u u
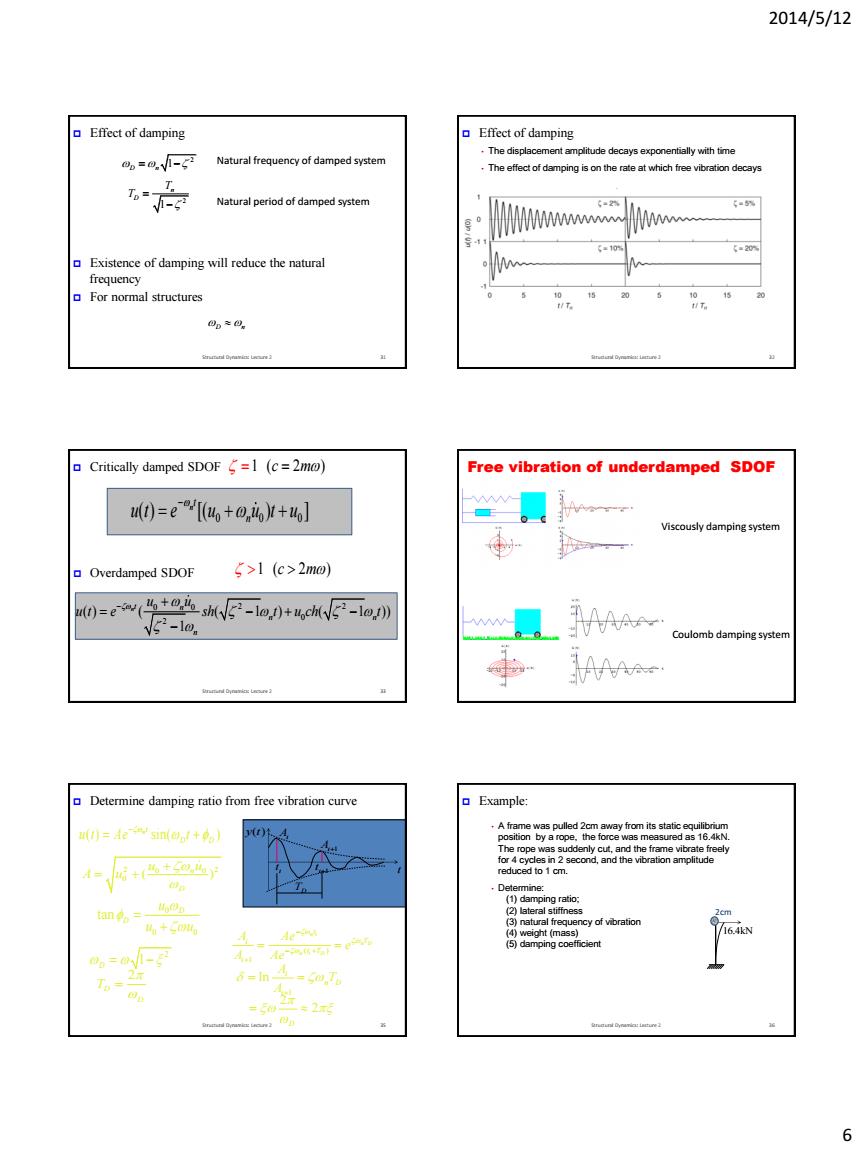
2014/5/12 Effect of damping Effect of damping %=0F Natural frequency of damped sysem To Natural period of damped system Existence of damping will reduce the natural Critically damped SDOF=1 (c=2m) Free vibration of underdamped SDOF 0=e[u+o,41+4] Viscousy damping ystem Overdamped SDOF >1 (c>2ma) )=o-iom) J2-1 AA Coulomb dampingy Determine damping ratio from free vibration curve (=e The lope 6
2014/5/12 6 Structural Dynamics: Lecture 2 31 Effect of damping Existence of damping will reduce the natural frequency For normal structures 2 1 D n Natural frequency of damped system 2 1 n D T T Natural period of damped system D n Structural Dynamics: Lecture 2 32 Effect of damping • The displacement amplitude decays exponentially with time • The effect of damping is on the rate at which free vibration decays Structural Dynamics: Lecture 2 33 Critically damped SDOF 1 ( 2 ) c m 0 0 0 ( ) [( ) ] n t n u t e u u t u Overdamped SDOF 1 ( 2 ) c m 0 0 2 2 0 2 ( ) ( ( 1 ) ( 1 )) 1 n t n n n n u u u t e sh t u ch t Free vibration of underdamped SDOF Viscously damping system Coulomb damping system Structural Dynamics: Lecture 2 35 i t i1 t TD t y(t) Ai Ai1 2 D D T 2 1 D ( ) sin( ) n t D D u t Ae t ( ) 1 n i n D n i D t i T t T i A Ae e A Ae 1 ln i n D i A T A 2 2 D Determine damping ratio from free vibration curve 2 2 0 0 0 ( ) n D u u A u 0 0 0 tan D D u u u Structural Dynamics: Lecture 2 36 Example: • A frame was pulled 2cm away from its static equilibrium position by a rope, the force was measured as 16.4kN. The rope was suddenly cut, and the frame vibrate freely for 4 cycles in 2 second, and the vibration amplitude reduced to 1 cm. • Determine: (1) damping ratio; (2) lateral stiffness (3) natural frequency of vibration (4) weight (mass) (5) damping coefficient 2cm 16.4kN

2014/5/12 Solution: aC安名立号006 a.=2”=12.571/s m=冷=510e) (间Damping c=2mg,5=3601Nsm) --子05 T.=Tov-C Coulomb damping model 1 dR d5=2x R Coulomb damping model .In the first half cycle(from right to left) u(t)=Acos0J+Bsino+ur ur=F/k u。=u(0:d。=0A=u(0)-4e,A=0 .The solution in the first half cycle is u)=[u(0)-4,]osa1+4e The maximum displacement occurs at T/2 (b)mii+ku=-F u(t)=A coso+B:sinoJ-F/k u(x/o.)=-u(0+2u:i(x/,)=0 (c)mii+ku=F u(1)=A cos0/+Bsino.+F/k 7
2014/5/12 7 1 1 2 ln ln 0.0276 2 2 4 1 i i j A j A 3 16.4 10 5 8.2 10 ( / ) st 0.02 P k N m 2 0.5sec 4 D Time T Cycle Solution: • (1) Damping Ratio • (2) Stiffness • (3) Natural frequency 2 T T n D 1 Solution: (continue) • (4) Mass • (5) Damping coefficient 2 12.57(1/ s) n T n 2 5190(kg) k m 2 3601(N s/m) n c m Structural Dynamics: Lecture 2 39 Sensitivity analysis of logarithmic decrement method 1 1 1 ln ln 2 2 i i A R A 1 2 dR d R 1 2 dR R d Relative error of damping Relative error of amplitude measurement 0.01, 16 d fo d r R R Structural Dynamics: Lecture 2 40 Coulomb damping model Motion direction Motion direction D x f N c x The direction of the friction force oppose motion, therefore the sing of the friction force will change when the direction of motion changes. Structural Dynamics: Lecture 2 41 Coulomb damping model Motion direction Motion direction ( ) ( ) b mu ku F c mu ku F 2 2 1 1 cos sin / cos sin / n n n n u t A t B t F k u t A t B t F k • In the first half cycle (from right to left) • The solution in the first half cycle is • The maximum displacement occurs at T/2 Structural Dynamics: Lecture 2 42 1 1 cos sin / k n n F F u t A t B t u u F u u u A u u B t t F 0 0 1 1 0 ; 0 0 , 0 0 cos F n F u t u u t u u u u u / 0 2 ; / 0 n F n

2014/5/12 In the second half cycle(from left to right) .In the third half cycle(from left to right) u(t)=A coso+B.sino-ur u(1)=Acos0,!+Bsino+up u(x1.)=-u(0+24:x@)=0 A■u(0)-3u:B■0 Thesolution in the third half cycle is The solution in the first half cycle is u0=[u(0)-3wr]cos-h )=[u(0)-5,]os+4 In each cycle,the vibration amplitude reduce When t=T 4u (2rla.)=u(0)-4u 1=-4u Coulomb damping model Free vibration of underdamped SDOF In each cye f en the vibration stops? Viscousy damping ystem Coulomb dampingy Homework ·Prob.2.1,2.2,2.5,2.8,2.10 8
2014/5/12 8 • In the second half cycle (from left to right) • The solution in the first half cycle is • When t=Tn Structural Dynamics: Lecture 2 43 2 2 cos sin n n F u t A t B t u 0 3 cos F n F u t u u t u u u u 2 / 0 4 n F 2 2 / 0 2 ; / 0 0 3 ; 0 n F n F u u u u A u u B • In the third half cycle (from left to right) • The solution in the third half cycle is • In each cycle, the vibration amplitude reduce 4uF Structural Dynamics: Lecture 2 44 0 5 cos F n F u t u u t u 1 1 cos sin n n F u t A t B t u 1 4 i i F u u u Structural Dynamics: Lecture 2 45 Coulomb damping model • In each cycle of motion, the amplitude is reduced by 4uf • When the vibration stops? Free vibration of underdamped SDOF Viscously damping system Coulomb damping system Homework • Prob. 2.1, 2.2, 2.5, 2.8, 2.10 • Additional problem: identify the damping and frequency of the structure using field measured data Structural Dynamics: Lecture 2 47 0 20 40 60 80 100 120 -0.003 -0.002 -0.001 0.000 0.001 0.002 0.003 frequency= ?Hz damping= ? % Amplitude (m/s/s) Time (sec) 0 20 40 60 80 100 120 -0.003 -0.002 -0.001 0.000 0.001 0.002 0.003 frequency= ?Hz damping= ? % Amplitude (m/s/s) Time (sec) Structural Dynamics: Lecture 2 48