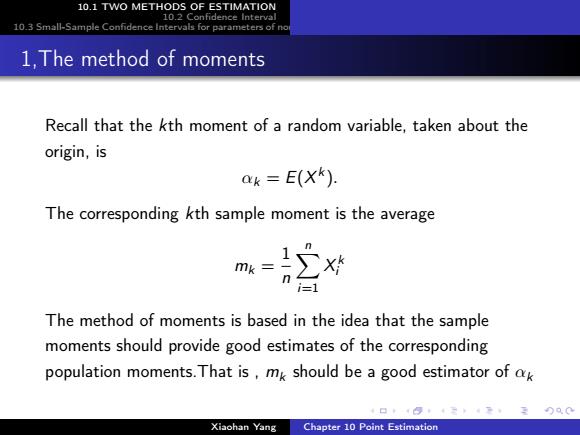
10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of n 1,The method of moments Recall that the kth moment of a random variable,taken about the origin,is ak E(Xk). The corresponding kth sample moment is the average n mk 1∑X i=1 The method of moments is based in the idea that the sample moments should provide good estimates of the corresponding population moments.That is,mk should be a good estimator of ok Xiaohan Yang Chapter 10 Point Estimation
logo 10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of normal population 1,The method of moments Recall that the kth moment of a random variable, taken about the origin, is αk = E(X k ). The corresponding kth sample moment is the average mk = 1 n Xn i=1 X k i The method of moments is based in the idea that the sample moments should provide good estimates of the corresponding population moments.That is , mk should be a good estimator of αk Xiaohan Yang Chapter 10 Point Estimation

10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of no DEFINITION Let (X1,...,Xn)is a random sample from a population X. ak=E(X*)。Suppose0=p(a1,·,am),then 0=(A1,...,Am)is the method-of-moments estimator for 0. where Ak=A∑=1X. 4口t9,18,元,2000 Xiaohan Yang Chapter 10 Point Estimation
logo 10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of normal population DEFINITION Let (X1, · · · , Xn) is a random sample from a population X. αk = E(X k )"Suppose θ = ϕ(α1, · · · , αm), then θ ˆ = ϕ(A1, · · · , Am) is the method-of-moments estimator for θ, where Ak = 1 n Pn i=1 X k i . Xiaohan Yang Chapter 10 Point Estimation

10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of ne Property Let(X1,...,Xn)is a random sample from a population X. Denoted as E(X)=u,D(X)=o2,Both of u,o2 are unknown. X is the method-of-moments estimator for u S is the method-of-moments estimator for o2 4口45,43,手·3000 Xiaohan Yang Chapter 10 Point Estimation
logo 10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of normal population Property Let (X1, · · · , Xn) is a random sample from a population X. Denoted as E(X) = µßD(X) = σ 2ßBoth of µ, σ2 are unknown. 1 X is the method-of-moments estimator for µ 2 S 2 n is the method-of-moments estimator for σ 2 Xiaohan Yang Chapter 10 Point Estimation

10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of no Property Let(X1,...,Xn)is a random sample from a population X. Denoted as E(X)=u,D(X)=02,Both of u,o2 are unknown. X is the method-of-moments estimator for u S2 is the method-of-moments estimator for o2 4口t9,18,元,2000 Xiaohan Yang Chapter 10 Point Estimation
logo 10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of normal population Property Let (X1, · · · , Xn) is a random sample from a population X. Denoted as E(X) = µßD(X) = σ 2ßBoth of µ, σ2 are unknown. 1 X is the method-of-moments estimator for µ 2 S 2 n is the method-of-moments estimator for σ 2 Xiaohan Yang Chapter 10 Point Estimation

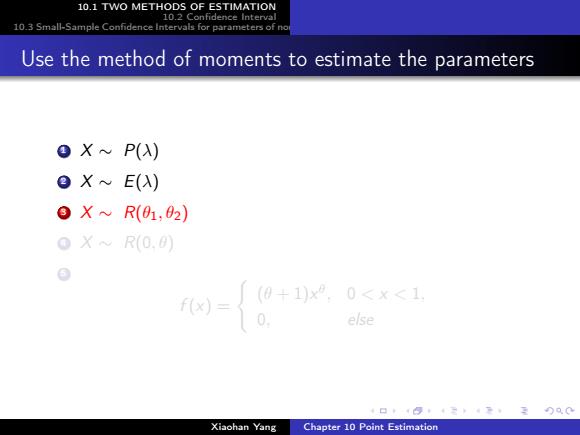
10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of no Use the method of moments to estimate the parameters X~P(A) ⊙XE 0XR(01.02 0XR0.00 f(x)= (0+1x”,0<x<1 0 else 4口·5,42手·3000 Xiaohan Yang Chapter 10 Point Estimation
logo 10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of normal population Use the method of moments to estimate the parameters 1 X ∼ P(λ) 2 X ∼ E(λ) 3 X ∼ R(θ1, θ2) 4 X ∼ R(0, θ) 5 f (x) = ( (θ + 1)x θ , 0 < x < 1, 0, else Xiaohan Yang Chapter 10 Point Estimation

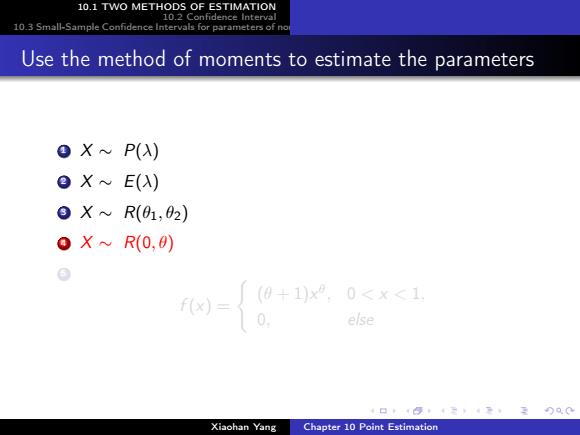
10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of no Use the method of moments to estimate the parameters X~P(A) X~E() OX心R(,02 0XR(0.0) f)= (0+1)x”,0<x<1 0 else 4口t3,t3元,王000 Xiaohan Yang Chapter 10 Point Estimation
logo 10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of normal population Use the method of moments to estimate the parameters 1 X ∼ P(λ) 2 X ∼ E(λ) 3 X ∼ R(θ1, θ2) 4 X ∼ R(0, θ) 5 f (x) = ( (θ + 1)x θ , 0 < x < 1, 0, else Xiaohan Yang Chapter 10 Point Estimation
10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interva 10.3 Small-Sample Confidence Intervals for parameters of no Use the method of moments to estimate the parameters X~P(A) 9X~E() 0X~R(0,02) 0XR(0.0 (0+1Dx”0<x<1 0 else 4日·5,421手,3000 Xiaohan Yang Chapter 10 Point Estimation
logo 10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of normal population Use the method of moments to estimate the parameters 1 X ∼ P(λ) 2 X ∼ E(λ) 3 X ∼ R(θ1, θ2) 4 X ∼ R(0, θ) 5 f (x) = ( (θ + 1)x θ , 0 < x < 1, 0, else Xiaohan Yang Chapter 10 Point Estimation
10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of no Use the method of moments to estimate the parameters ④X~P(A) X~E(A) ⊙X~R(01,2) 0X~R(0,) f(x)= (0+1)x”,0<x<1 0 else 4口13,48)4元,3000 Xiaohan Yang Chapter 10 Point Estimation
logo 10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of normal population Use the method of moments to estimate the parameters 1 X ∼ P(λ) 2 X ∼ E(λ) 3 X ∼ R(θ1, θ2) 4 X ∼ R(0, θ) 5 f (x) = ( (θ + 1)x θ , 0 < x < 1, 0, else Xiaohan Yang Chapter 10 Point Estimation
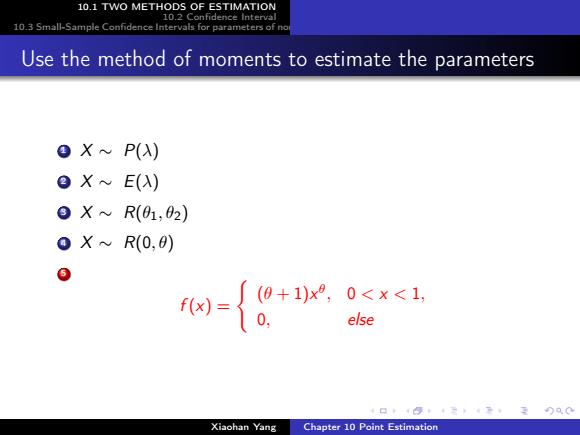
10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interva 10.3 Small-Sample Confidence Intervals for parameters of no Use the method of moments to estimate the parameters X~P(A) 9XE() oXR(,02) ⊙X~R(0,) 0 (0+1)x,0<x<1, 0 else 4口·5,43)手,3月00 Xiaohan Yang Chapter 10 Point Estimation
logo 10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of normal population Use the method of moments to estimate the parameters 1 X ∼ P(λ) 2 X ∼ E(λ) 3 X ∼ R(θ1, θ2) 4 X ∼ R(0, θ) 5 f (x) = ( (θ + 1)x θ , 0 < x < 1, 0, else Xiaohan Yang Chapter 10 Point Estimation

10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of no 2.the method of maximum likelihood (MLE) Let (X1,...,Xn)is a random sample from a population X. Suppose the probability density(mass)function of X is f(x:0),0e.We sometimes write the likelihood function as L(0)=L(0,x,…,xn)=n1f(x;) 4口t3,3元,王000 Xiaohan Yang Chapter 10 Point Estimation
logo 10.1 TWO METHODS OF ESTIMATION 10.2 Confidence Interval 10.3 Small-Sample Confidence Intervals for parameters of normal population 2. the method of maximum likelihood (MLE) Let (X1, · · · , Xn) is a random sample from a population X. Suppose the probability density(mass) function of X is f (x; θ), θ ∈ Θ. We sometimes write the likelihood function as L(θ) = L(θ, x1, · · · , xn) ˆ=Πn i=1 f (xi ; θ) Xiaohan Yang Chapter 10 Point Estimation